Sometimes you may need a quick and easy way to access Internet Explorer’s cache. Today we take a look at IECacheView which is a great application to get the job done.

有时，您可能需要一种快速简便的方法来访问Internet Explorer的缓存。 今天，我们来看一下IECacheView，它是完成工作的绝佳应用程序。

Setup

建立

There is no installation for you to worry about. Simply unzip the program zip file, place the two files inside (IECacheView.exe and IECacheView.chm) in their own folder in Program Files, and create a shortcut. Everything will be all ready to go.

没有安装让您担心。 只需解压缩程序zip文件，然后将两个文件(IECacheView.exe和IECacheView.chm)放在“程序文件”中其各自的文件夹中，然后创建快捷方式。 一切都准备就绪。

Once you open IECacheView, this is what it will look like…

一旦打开IECacheView，它的外观将是…

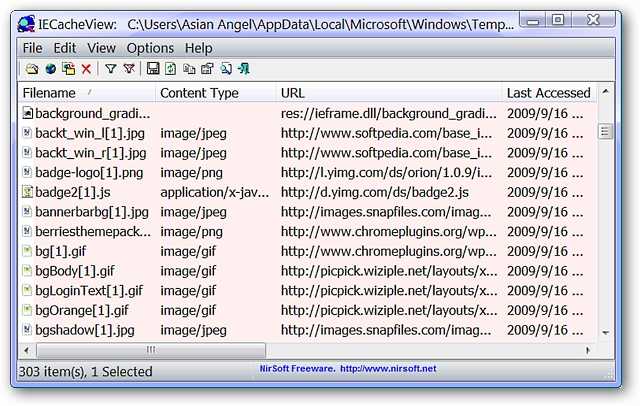
Scroll over to the right of the app and you get additional information and it’s easy to customize what columns are displayed based on your personal needs.

滚动到应用程序的右侧，您将获得更多信息，并且可以轻松地根据您的个人需求自定义显示哪些列。

Menus

选单

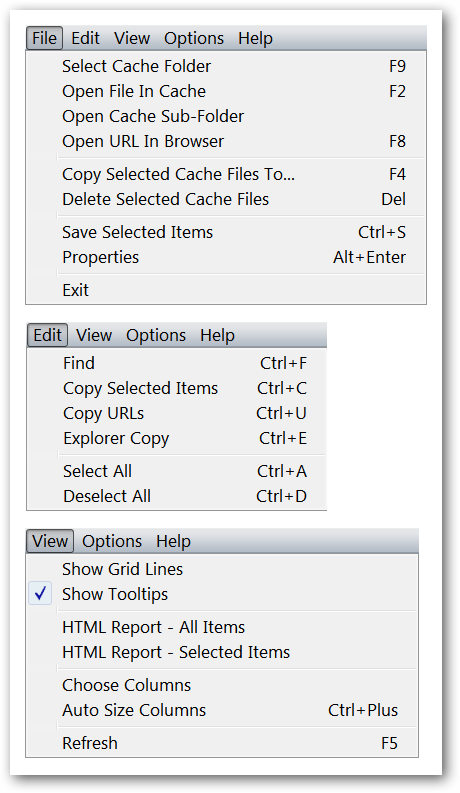
Time for a look at the menu system for IECacheView…here you can see the “File, Edit, & View Menus”. Each one provides a multitude of options that you can apply to folders, the cache, sub-folders, URL opening, saving, deleting, & copying, display (i.e. grid lines and columns), html reports, and more (very nice!).

是时候来看一下IECacheView的菜单系统了……在这里您可以看到“文件，编辑和查看菜单”。 每个选项都提供许多选项，您可以将它们应用于文件夹，缓存，子文件夹，URL打开，保存，删除和复制，显示(即网格线和列)，html报告等等(非常好！ ) 。

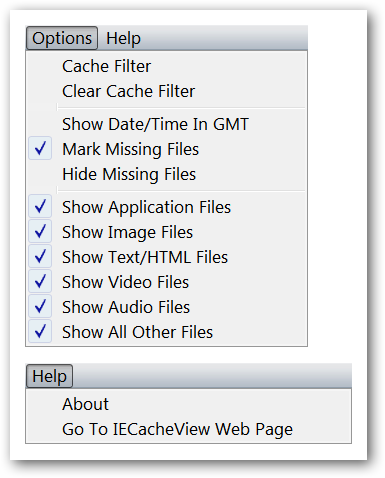
The “Options and Help Menus”. Notice that you can specify the types of files that you would like to have displayed (very convenient!).

“选项和帮助菜单”。 请注意，您可以指定要显示的文件类型(非常方便！ )。

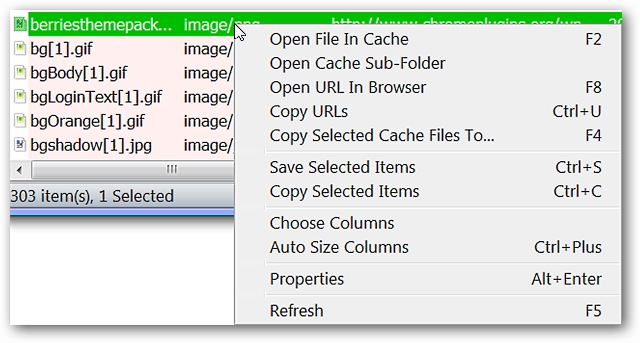
These are the options available in the “Right Click Menu”.

这些是“右键菜单”中可用的选项。

Conclusion

结论

IECacheView makes a nice little utility to have around if you ever need a quick and easy way to dig into Internet Explorer’s cache.

如果您需要一种快速简便的方法来挖掘Internet Explorer的缓存，IECacheView会提供一个不错的小实用程序。

Links

链接

Download IECacheView (version 1.31)

下载IECacheView(1.31版)

Note: Download link is at the bottom of the page.

注意：下载链接位于页面底部。

Works On: Windows 98 – Vista, Internet Explorer 6 – 8

适用于： Windows 98 – Vista，Internet Explorer 6 – 8

Installed On: Windows Vista (32 bit), Service Pack 2

安装于： Windows Vista(32位)，Service Pack 2

翻译自: https://www.howtogeek.com/howto/4592/view-internet-explorer-cache-files-the-easy-way/