前言
多相滤波器解决的是:多速率问题。
通过降采样、插值来改变信号的输出速率(主要利用Nyquist采样定理,保证不混叠),从而降低数据率,多相滤波器为这类操作提供了实现框架。在满足采样定理的前提下,内插/抽取并配合滤波器使用(防止混叠),可以改变数据的速率。
多相滤波的结构也多用在信道化中(即构建滤波器组),固化系数借助硬件实现快速运算。
一、信道化实现思路
信道化的基本思路为:
clc;clear all;close all
N = 256;
h = firls(N, [0 .2 .25 1], [1 1 0 0],[.001 .0001]);
D = 8;
% PolyPhase Componets
i = 1:length(h);
h_channel = zeros(D,length(h));
im = sqrt(-1);
for j = 1:Dh_channel(j,:) = h.*exp((-im*2*pi*((j-1)*(i-1)))/D);
end
figure(1)
for i = 1:D+1switch icase 1subplot (2,1,1);plot(abs(fft(h)));title('Orignal LPF ')otherwisesubplot (2,1,2)plot(abs(fft(h_channel(i-1,:))));hold on;title('channelize')end
end
对应频谱图:
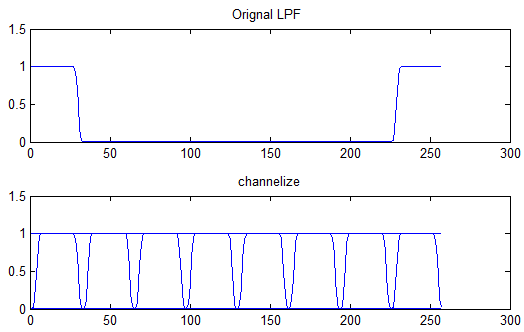
即将频带切分成若个个区域。基于该特性,有信道化的基本思路:
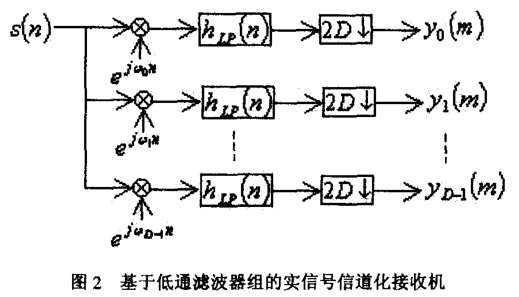
如果是实信号,D信道化之后进行D倍抽取仍然不会有频谱混叠,而经过本振以及低通滤波器之后的信号为复信号,故可以进行2D倍抽取。
可以看出,信道化接收机的抽取器位于滤波器之后,当抽取率D很大或者滤波器阶数较高,计算效率将难以提升,这时候借助多相滤波器便可以优化。
假设LPF:

并取Q=N/D,N为滤波器阶数,D为抽取率。则滤波器可重写为:
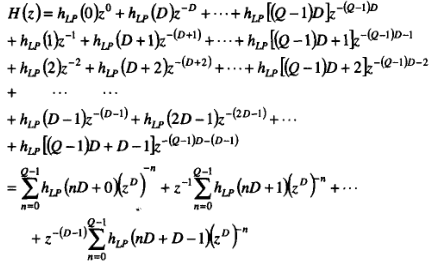
定义:
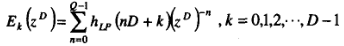
滤波器可重写为:
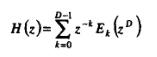
其中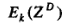 为多相分量,这也是多相滤波器说法的缘由。
为多相分量,这也是多相滤波器说法的缘由。
第k个信道可写为:
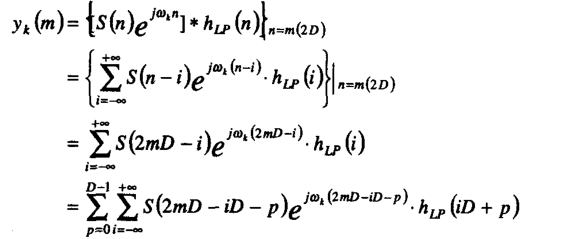
记

有
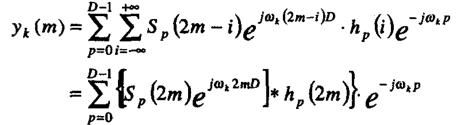
令
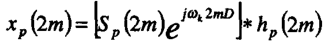
则

进一步取
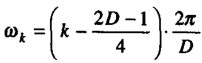
从而有
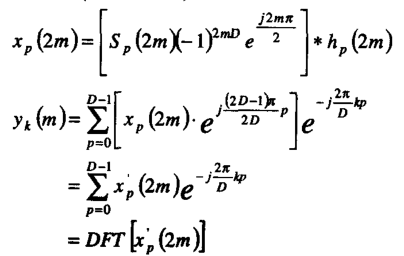
根据这一系列推导,即可得出实信号多相滤波器信道化的实现思路:
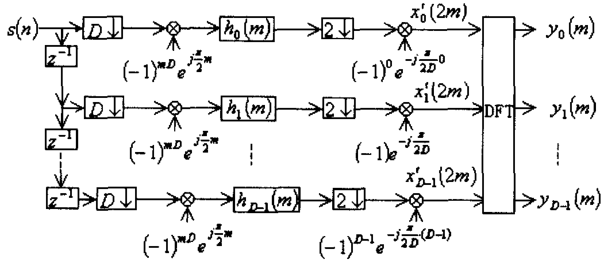
两处相位相乘分别为: ,p = 0,1,...,D-1
,p = 0,1,...,D-1
二、多相滤波器设计步骤
- 根据指标,确定滤波器类型以及阶数N
- 求解h(n)
- 根据信道个数,并用下式确定多相滤波器:
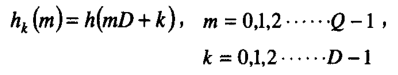
三、复杂度简要分析
以滤波器阶数N=256,抽取率(信道个数)D=16,每个信道输出1个数据,则:
- 基于低通滤波器组的信道化需要乘法:M1 = D x (1+N) = 4112次;
- 基于多相滤波器的信道化需要乘法:M2 = N+2*D+D*log2D = 352次;
多相滤波器的实现思路节约了资源,且便于硬件实现。
四、应用实例
考虑带宽1GHz的情况,如果信道个数为16,则每一个的宽度为1e9/16=62.5e6,考虑到滤波器为实数的共轭对称性,故LPF构造依据:
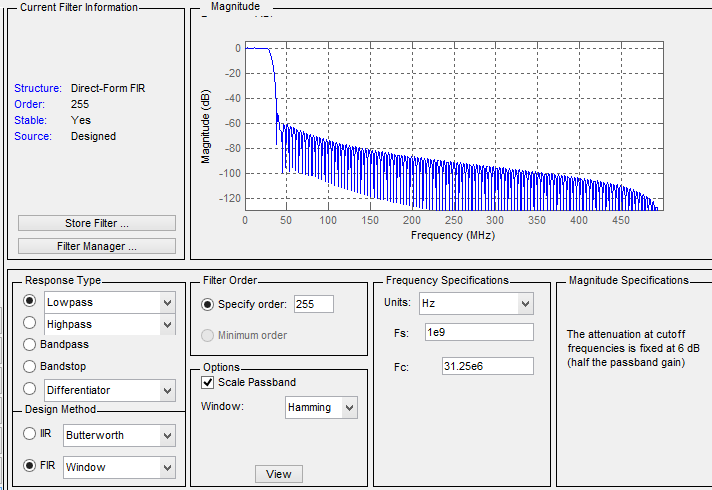
对其进行仿真验证:
输入信号为70MHz的1)余弦信号;2)复指数信号,对于1)理论上应落在2、16两个信道,2)只落在2信道。
clc;clear all;close all
load coef_lpf.mat;
fs = 1e9;
t = 0:1/fs:1e-6;
f0 = 70e6;
sig = sin(2*pi*t*f0);
len = length(sig);
h = coef_lpf;
N = length(h);
D = 16;
y = zeros(D,len);
% PolyPhase Componets
i = 1:length(h);
h_channel = zeros(D,length(h));
im = sqrt(-1);
x_fre = linspace(0,fs,N);
for j = 1:Dh_channel(j,:) = h.*exp((-im*2*pi*((j-1)*(i-1)))/D);y(j,:) = filter(h_channel(j,:),1,sig);
end
figure()
for i = 1:D+1switch icase 1subplot (2,1,1);plot(x_fre,abs(fft(h)));title('Orignal LPF ')otherwisesubplot (2,1,2)plot(x_fre,abs(fft(h_channel(i-1,:))));hold on;title('channelize')end
end
x_fre1 = linspace(0,fs,len);
figure()
plot(x_fre1,abs(fft(sig)));
for iter = 1:Dif mod(iter,4) ==1figure()j = 1;endsubplot(4,1,j)j = j+1;plot(x_fre1,abs(fft(y(iter,:))));title(['信道',num2str(iter)]);
end
仿真结果:
信道化结果:

情形一:
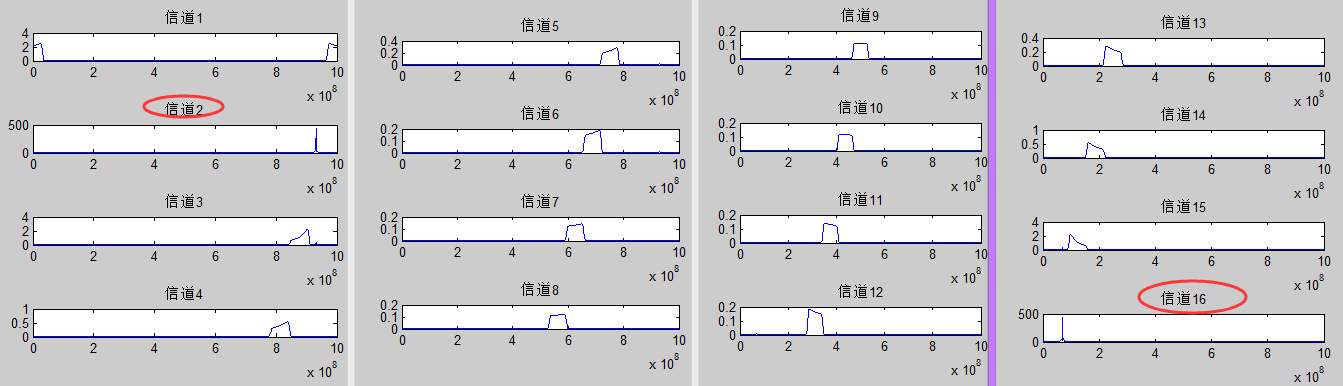
与理论分析相符。
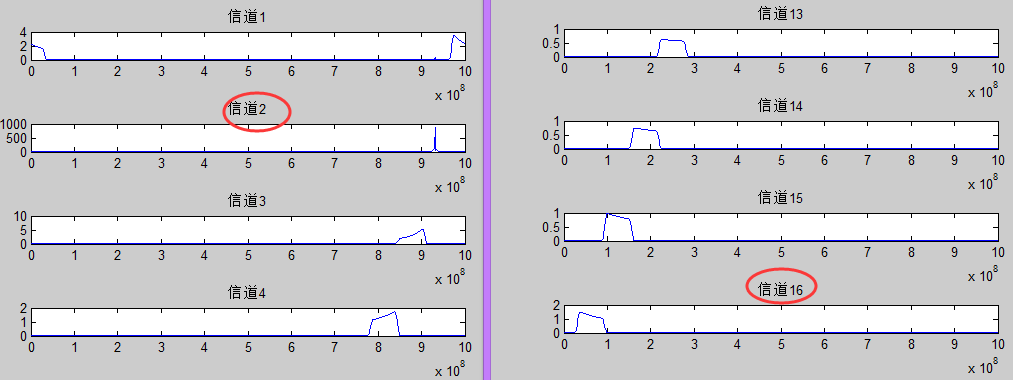
情形二:
sig = exp(-1j*2*pi*t*f0);
信号只在信道2出现:
多相滤波的实现思路也非常多,这里仅列举一种:

clc;clear all;close all; load coef_lpf.mat; h = coef_lpf; %%产生信号 fc = 70e6; c = 3.0e8; fs = 1e9; theta = 30/180*pi; lambda = c/fc; d = lambda/4; len = 2048; t = 0:1/fs:1/fs*(len-1); st = exp(-1j*2*pi*t*fc); Phase = 2*pi*d*sin(theta)/lambda; st = st/trace(st*st'); Interformer01 = st; %%信道化 D = 16; si = 1:length(h); h_channel = zeros(D,length(h)); im = sqrt(-1); for j = 1:Dh_channel(j,:) = downsample(h.*exp((im*2*pi*((si-1)*(j-1)))/D),1); end figure() for i = 1:Dplot(abs(fft(h_channel(i,:))));hold on; end Interformer_channel = zeros(D,len/D); for i = 1:DInterformer_channel(i,:) = downsample(filter(h_channel(i,:),1,Interformer01),D); end figure() for i = 1:Dsubplot(4,4,i)plot(real((Interformer_channel(i,:)))); end %%信道化实现思路 si_new = 1:length(h)/D; Interformer01_py = (reshape(Interformer01,D,len/D)); % Interformer01_py = Interformer01_py.*repmat((exp(1j/2*pi*si_new)),length(h)/D,1); % h_py = reshape(h,D,length(h)/D); h_py = fliplr(reshape(h,D,length(h)/D));%.*repmat((exp(1j/2*pi*si_new)),D,1); Interformer02_channel = zeros(D,len/D);for i = 1:DInterformer02_channel(i,:) = downsample(filter(h_py(i,:),1,Interformer01_py(i,:)),1); % Interformer02_channel(i,:) = Interformer02_channel(i,:)*(-1)^(i-1)*exp(-1j*pi/2/D*(i-1)); end Interformer02_channel = fft(Interformer02_channel); figure() for i = 1:Dsubplot(4,4,i)plot(real((Interformer02_channel(i,:)))); end
信道化结果:
直接信道化:
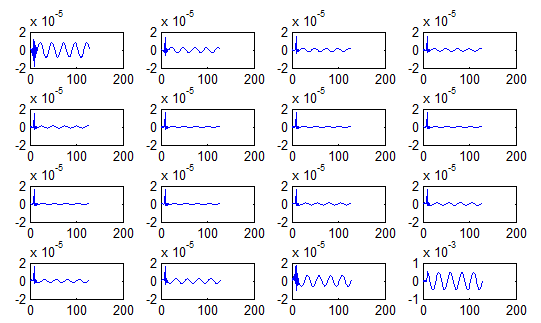
多相实现:
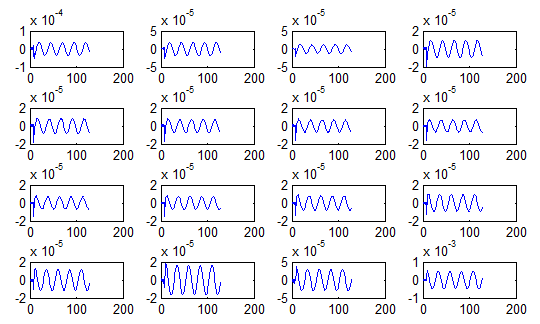
该方式比直接实现,兔耳效应更明显。



















