125. 验证回文串

原题链接 125. 验证回文串

双指针，一前一后，遇到非数字字母跳过即可

class Solution {
public:bool isPalindrome(string s) {int i = 0, j = s.size()-1;while(i < j) {while(i<j && !isalnum(s[i])) i++;while(i<j && !isalnum(s[j])) j--;if(tolower(s[i]) != tolower(s[j])) {return false;}i++, j--;}return true;}
};

28. 找出字符串中第一个匹配项的下标

原题链接 28. 找出字符串中第一个匹配项的下标

子串匹配问题，有很多算法，其中最著名的是KMP算法，时间复杂度是$O(M+N)$。之前短暂的理解过，现在又不明白了。贴一个讲解 可能是全网最清晰的KMP算法讲解
理解不了就硬背

class Solution {
public:int strStr(string haystack, string needle) {int len_n = needle.size();int next[len_n];next[0] = -1;for(int i = 1, j = -1;i < len_n;i++){while(j > -1 && needle[i] != needle[j+1]) j = next[j];if(needle[i] == needle[j+1]) j++;next[i] = j;}for(int i = 0, j = -1;i < haystack.size();i++) {while(j > -1 && haystack[i] != needle[j+1]) j = next[j];if(haystack[i] == needle[j+1]) j++;if(j == len_n-1) {return i-len_n+1;}}return -1;}
};

找到一个好理解的Sunday算法
算法仍然包括两步：预处理和移动模式串
基本流程是：

• 左对齐，开始进行匹配
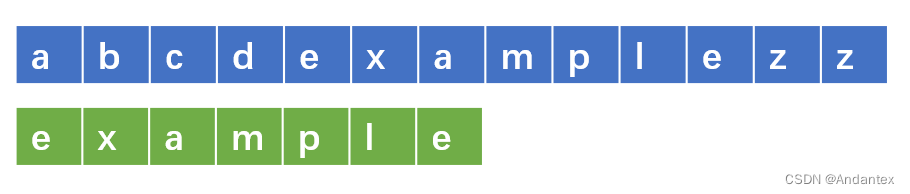
• 一旦匹配失败，对“下一位”进行检查
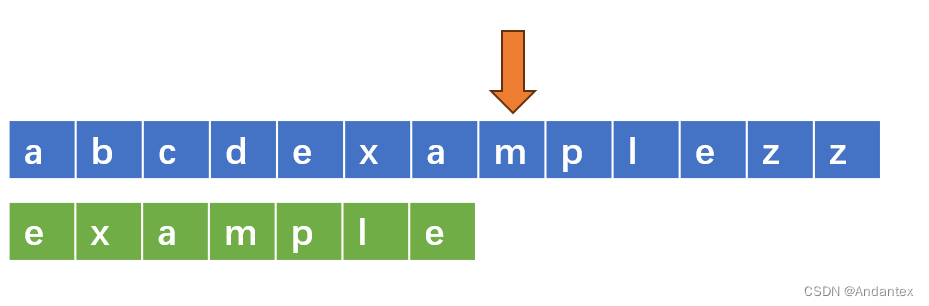
• 找到模式串中该字母最后出现的位置，然后对齐，开启下一轮匹配
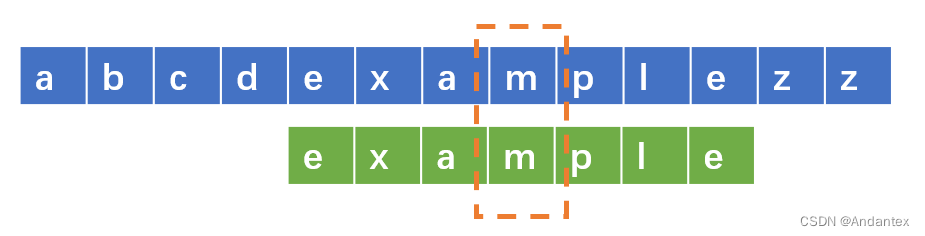
  为了加速这个过程，我们预处理出一个键值对，键即字母表中的各个字母，对应的值是该字母在模式串中出现的位置（事实上，我们使用一个数组进行模拟；并且处理值，使得其意味着“此时模式串应该向后平移几位”）

class Solution {
public:int strStr(string haystack, string needle) {int n = haystack.size(), m = needle.size();if(m > n) return -1;vector<int> shifts(26,m+1);for(int i = 0;i < m;i++) {shifts[needle[i]-'a'] = m-i;}int s = 0, i;while(s <= n-m) {i = 0;while(haystack[s+i] == needle[i]) {i++;if(i == m) {return s;}}if(s+m >= n) break;s += shifts[haystack[s+m]-'a'];}return -1;}
};

151. 反转字符串中的单词

原题链接 151. 反转字符串中的单词

• 第一步，去掉前后的空格
• 第二步，双指针，均从串末尾开始遍历，一个指向单词的开头，一个指向单词的结尾
  • 交替地判断正在被遍历的是字母还是空格

class Solution {
public:string reverseWords(string s) {int i,j;int len = s.size();for(i = 0;i < len && s[i] == ' ';i++);for(j = len-1;j >= 0 && s[j] == ' ';j--);s = s.substr(i,j-i+1);i = s.size()-1;j = s.size()-1;string result = "";while(i >= 0) {while(i >= 0 && s[i] != ' ') i--;result += s.substr(i+1,j-i) + " ";while(i >= 0 && s[i] == ' ') i--; j = i;}result = result.substr(0,result.size()-1);return result;}
};

6. Z 字形变换

原题链接 6. Z 字形变换

直接分类讨论，设我们讨论在Z字形中的第i行第j个字母，其在原串中的位置是index

• 每一行的index都从i开始
• 对于第一行和最后一行，j每增加1，index增加2*(numRows-1)
• 对于中间
  • j是偶数，则index增加2*(numRows-i-1)
  • j是奇数，则index增加2*i

class Solution {
public:string convert(string s, int numRows) {int len = s.size();if(numRows == 1 ||  len <= numRows) return s;string result;int t = 2*(numRows-1);for(int i = 0;i < numRows;i++) {int j = 0;int index = i;while(index < len) {result.push_back(s[index]);if(i == 0 || i == numRows-1) {index += t;} else if(j%2 == 0) {index += t-2*i;} else {index += 2*i;}j++;}}return result;}
};

68. 文本左右对齐

原题链接 68. 文本左右对齐

做的我有点脑溢血了，大致分三步

• 第一步，将原单词列分成连续的区间，每个区间中的单词应该以左右对齐放在一行中
  • 设单词数量是$n$，单词总长度是$sum$，则满足$sum+n-1\le maxWidth$的尽可能长的一组单词应该放到一行（即，最少在每个单词之间放一个空格）

    • 这一步我脑残突然发了，死活写不出来。其实简化一下问题就是：有一个数组，一个最大和maxSum。写一个函数，将数组分成几个尽可能长的连续片段，使得在每个连续片段内，数组元素的和都小于等于maxSum。代码应该是：

      vector<vector<int>> splitArray(const vector<int>& nums, int maxSum) {vector<vector<int>> result;int currentSum = 0;vector<int> currentSegment;for (int num : nums) {if (currentSum + num > maxSum) {// 当前片段的和超过maxSum，将当前片段加入结果，并开始新的片段result.push_back(currentSegment);currentSegment.clear();currentSum = 0;}currentSegment.push_back(num);currentSum += num;}// 将最后一个片段加入结果result.push_back(currentSegment);return result;
      }
      

      • 应该是 执行循环判断是否下标越界–>再循环内先判断是否已经超出最大和–>构造序列
• 第二步，对每组单词，执行左右对齐。假设需要$s$个空格放到$n$个间隔之中去，思路是先给每个间隔分配$\lfloor \frac{s}{n}\rfloor$个空格，再给前$s\mathrm{mod}n$个间隔多分配上一个空格
• 第三步，给剩下的单词分配左对齐。这个好说。

class Solution {
public:struct Line {int st;int ed;int lw;};string space_factory(int n) {return string(n,' ');}vector<string> fullJustify(vector<string>& words, int maxWidth) {int len = words.size();int wordsize[len];for(int i = 0;i < len;i++) {wordsize[i] = words[i].size();}vector<Line> lines;int start = 0, count = 0, sum = 0;while(start < len) {count = 0;sum = 0;while(start+count < len) {if(sum+count+wordsize[start+count]>maxWidth) {lines.push_back({start,start+count-1,sum});break;}sum += wordsize[start+count];count++;}start += count;}start -= count;vector<string> res;for(int i = 0;i < lines.size();i++) {auto line = lines[i];int sum_spaces = maxWidth-line.lw;string new_line = "";new_line += words[line.st];if(line.st == line.ed) {new_line += space_factory(sum_spaces);} else {int sigle_spaces = (sum_spaces)/(line.ed-line.st);int remain_spaces = (sum_spaces)%(line.ed-line.st);for(int j = 1;line.st+j<=line.ed;j++) {if(j-1 < remain_spaces) {new_line += space_factory(sigle_spaces+1);} else {new_line += space_factory(sigle_spaces);}new_line += words[line.st+j];}}res.push_back(new_line);}string new_line = "";new_line += words[start];sum = wordsize[start];for(int i = start+1;i < len;i++) {new_line += " ";new_line += words[i];sum +=  wordsize[i];}new_line += space_factory(maxWidth-sum-(len-start-1));res.push_back(new_line);return res;}
};