计数器数组

Problem statement:

问题陈述：

Given an array of N positive integers a₁, a₂,  ..., a_n. The value of each contiguous subarray of a given array is the maximum element present in that subarray. The task is to return the number of subarrays having value strictly greater than K.

给定N个正整数数组a ₁ ，a ₂ ，...，a _n 。 给定数组的每个连续子数组的值是该子数组中存在的最大元素。 任务是返回值严格大于K的子数组数。

Input:

输入：

The first line contains two space-separated positive integers N and K denoting the size of the array and the value of K. The second line contains N space-separated positive integers denoting the elements of the array.

第一行包含两个空间隔开的正整数N，和K表示的阵列的尺寸和K值。 第二行包含N个以空格分隔的正整数，它们表示数组的元素。

Output:

输出：

Output the number of subarrays having value strictly greater than K.

输出值严格大于K的子数组数。

Constraints:

限制条件：

1 <= T <= 50
1 <= N <= 100
1 <= a[i] <= 105

Example:

例：

Test case: 1
Input: 
5 3
3 4 5 2 7
Output:
13
Test case: 2
4 1
1 2 3 4
Output:
9

Explanation:

说明：

Test case 1: 
All possible subarrays are listed below 
with their respective value (maximum in the subarray)
3 -> 3
4 -> 4
5 -> 5
2 -> 2
7 -> 7
3, 4 -> 4
4, 5 -> 5
5, 2 -> 5
2, 7 -> 7
3, 4, 5 -> 5
4, 5, 2 -> 5
5, 2, 7 -> 7
3, 4, 5, 2 -> 5
4, 5, 2, 7 -> 7
3, 4, 5, 2, 7 -> 7
So, number of valid subarrays is 13

Solution Approach:

解决方法：

1. Create dp[n][n] to store value (maximum) of subarray;

   创建dp [n] [n]来存储子数组的值(最大值)；

2. Initialize count = 0 which will be our final result;

   初始化计数= 0 ，这将是我们的最终结果；

3. Base case computation (single length subarrays),

   基本案例计算(单长度子数组)，

   for i=0 to n
   // since only one element in single length subarray, 
   // just check for that element
   if(a[i]>k) 
   dp[i][i]=a[i];
   count++;
   else
   dp[i][i]=-1; // or arr[i] itslef
   end for
   
   

4. Computing all length subarray cases,

   计算所有长度的子阵列案例，

   for subarray length,len=2 to n
   for start=0 to n-len
   end=start+len-1; // last element of subarray
   if(a[end]>k || dp[start][end-1]>k)
   dp[start][end]=std::max(a[end],dp[start][end-1]);
   count++;
   else
   dp[start][end]=-1;  
   end for
   end for
   
   

Okay, so the strategy is to compute for subarray a[start..end] with help of already computed one a[start..end-1].

好的，因此该策略是在已经计算的a [start..end-1]的帮助下为子数组a [start..end]计算。

Subarray a[start..end] will only make a count if a[start..end-1] already makes a count ( yes because, it's part of the subarray so, anything max in it would be max in the parent one too) Or if a[end]>k.

子数组a [start..end]仅在a [start..end-1]已经计数的情况下才会计数(是的，因为它是子数组的一部分，所以其中的任何最大值在父数组中也是最大值)或者a [end]> k 。

In both the cases maximum of the subarray, a[start..end] is greater than K

在这两种情况下，子数组的最大值a [start..end]都大于K

That's what we have done.

那就是我们所做的。

Below is illustration for our test case 1,

下面是我们的测试用例1的说明，

Input array: [3, 4, 5, 2, 7] and K=3.

输入数组： [3，4，5，2，7]和K = 3 。

So, let's compute the basic test case first

因此，让我们先计算基本测试用例

We need a 5X5 DP array for this

为此，我们需要一个5X5 DP阵列

Starting to compute for other values.

开始计算其他值。

Len=2

Len = 2

Start=0, end=1

开始= 0，结束= 1

Start=1, end=2

开始= 1，结束= 2

Start=2, end=3

开始= 2，结束= 3

Start=3, end=4

开始= 3，结束= 4

Len=3

Len = 3

Start=0, end=2

开始= 0，结束= 2

Start=1, end=3

开始= 1，结束= 3

Start=2, end=4

开始= 2，结束= 4

Len=4

Len = 4

Start=0, end=3

开始= 0，结束= 3

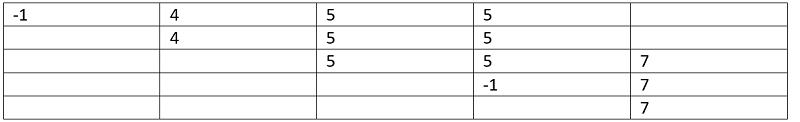
Start=1, end=4

开始= 1，结束= 4

Len=5

Len = 5

Start=0, end=3

开始= 0，结束= 3

Done!

做完了！

Count is 13 (count of positive values in the array).

Count是13(数组中正值的数量)。

C++ Implementation:

C ++实现：

#include <bits/stdc++.h>
using namespace std;
int maximum(int a, int b)
{
return (a > b) ? a : b;
}
int subArray(vector<int> a, int n, int k)
{
int dp[n][n];
int count = 0;
for (int i = 0; i < n; i++) {
if (a[i] > k) {
dp[i][i] = a[i];
count++;
}
else
dp[i][i] = -1; //or arr[i] itslef
}
for (int len = 2; len <= n; len++) {
for (int start = 0; start <= n - len; start++) {
int end = start + len - 1;
if (a[end] > k || dp[start][end - 1] > k) {
dp[start][end] = std::max(a[end], dp[start][end - 1]);
count++;
}
else
dp[start][end] = -1;
}
}
return count;
}
int main()
{
int n, item, k;
cout << "Input size of array\n";
cin >> n;
cout << "Input k\n";
cin >> k;
cout << "Add the array elements\n";
vector<int> a;
for (int j = 0; j < n; j++) {
scanf("%d", &item);
a.push_back(item);
}
cout << "Total count of valid subarray is " << subArray(a, n, k) << endl;
return 0;
}

Output:

输出：

Input size of array
5
Input k
3
Add the array elements
3 4 5 2 7
Total count of valid subarray is 13

翻译自: https://www.includehelp.com/icp/count-of-subarrays.aspx

计数器数组