Codeforces Round #693 (Div. 3)A~G解题报告

A Cards for Friends

原题信息

http://codeforces.com/contest/1472/problem/A

解题思路

本题就是一个找 $x/2^i=old,y/2^j=old$, 返回 $2^i\ast 2^j>=n$
一般这样的题目都需要注意使用 LL

AC代码

#include <cstdio>
#include <cstring>
#include <algorithm>
#include <iostream>
#include <fstream>
using namespace std;typedef long long LL;bool inline Check(LL x, LL y, LL n)
{LL ret = 1;while ((x & 1) == 0){ret <<= 1;x >>= 1;if (ret >= n)return true;}while ((y & 1) == 0){y >>= 1;ret <<= 1;if (ret >= n)return true;}if (ret >= n)   return true;else    return false;
}int main()
{int T;  cin >> T;while (T -- ){static LL x, y, n;cin >> x >> y >> n;if (Check(x, y, n))cout << "YES\n";elsecout << "NO\n";}return 0;
}

---

---

---

B Fair Division

原题信息

http://codeforces.com/contest/1472/problem/B

解题思路

能否将糖果平均分，直接分类讨论
首先统计 $1gram$ 数量为$cnt1$,$2gram$数量为$cnt2$下面对$cnt1,cnt2$进行分类讨论
$cnt1偶数,cnt2偶数$
————可以直接进行平均分
$cnt1偶数,cnt2奇数$
————当$cnt1=0$时，不可以平均分
————当$cnt1!=0$时，可以平均分，只需要将$cnt1-=2,cnt2++$即可
$cnt1奇数,cnt2偶数或偶数$
————因为$cnt1$为奇数，导致总的grams根本就是个奇数，是不可能平均分的。

AC代码

#include <cstdio>
#include <cstring>
#include <algorithm>
#include <iostream>
#include <fstream>
using namespace std;typedef long long LL;const int N = 200010;
int a[N], n;bool inline Check()
{static int cnt1, cnt2, sum;cnt1 = cnt2 = sum = 0;for (int i = 1; i <= n; i ++ ){sum += a[i];cnt1 += (a[i] == 1);cnt2 += (a[i] == 2);}if (sum & 1)   return false;else if (cnt1 == 0 && cnt2 % 2 == 1)    return false;else    return true;
}int main()
{int T;  cin >> T;while (T -- ){cin >> n;for (int i = 1; i <= n; i ++ ){scanf("%d", &a[i]);}if (Check())cout << "YES\n";elsecout << "NO\n";}return 0;
}

---

---

---

C Long Jumps

原题信息

http://codeforces.com/contest/1472/problem/C

解题思路

一个较为简单的dp，需要注意的是数组下标的特判，防止越界，最好开LL
$f_i$表示选择$i$做为$StartPosition$的游戏结果
那么，我们可以得到递归关系式为
————$f_i=a_i+f_{i+a_i}$ 条件为当 $i+a_i<=n$
————否则$f_i=a_i$
上述的这个讨论就是为了避免数组的越界

AC代码

#include <cstdio>
#include <cstring>
#include <algorithm>
#include <iostream>
#include <fstream>
using namespace std;typedef long long LL;const int N = 200010;
int a[N], n;
LL res = 0;
LL f[N];int main()
{int T;  cin >> T;while (T -- ){cin >> n;for (int i = 1; i <= n; i ++ ){scanf("%d", &a[i]);}memset(f, 0, sizeof f);res = 0LL;for (int i = n; i >= 1; i -- ){f[i] = a[i] + (i + a[i] <= n ? f[i + a[i]] : 0);res = max(res, f[i]);}/// printf("###########\n");cout << res << endl;}return 0;
}

---

---

---

D Even-Odd Game

原题信息

http://codeforces.com/contest/1472/problem/D

解题思路

题目大意是给定一个数组，$AliceBob$轮流在里面选一个数, $Alice$选择偶数，那么加和偶数一样大的分， 选择奇数不加分；$Bob$选择奇数，那么加和奇数一样大的分，选择偶数不加分。
从题意出发，我们不难想到下面这种求解方法，将奇数、偶数分开，两个数组分别进行非递增排序，每次选择的时候从两个数组选取最大的，进行拿出。（好像不分开也行）
对于$Alice$来讲，选取当前最大的数是偶数，那么加分最多，选取最大的数是奇数，是为了防止$Bob$加分过多！同理，对于$Bob$也是如此，下面我们来证明他的合法性。

• 直接严格证明是很难的，我们不妨辅助的思想来看这个问题，对于人物A来说，他可以选择自己可以加分的数字，也可以选择不让别人加分的数（自己不加分），不让别人加分，就类似于让自己加分（因为让别人加分少了）
• 也就是说让自己的得分减去另一个人得分更大，自己的赢面更大。因此对于博弈双方，我们只需要选择当前最大数即可。
• 记得开LL

AC代码

#include <cstdio>
#include <cstring>
#include <algorithm>
#include <iostream>
#include <fstream>
using namespace std;typedef long long LL;const int N = 200010;
int a[N], n;
int even_a[N], old_a[N], idx_e, idx_o;
LL score_a, score_b;
int cur_o = 1, cur_e = 1;
bool cmp(int a, int b){ return a > b; }bool Check()
{if (cur_o > idx_o)  return false;if (cur_e > idx_e)  return true;if (old_a[cur_o] >= even_a[cur_e])  return true;else    return false;
}
int main()
{int T;  cin >> T;while (T -- ){cin >> n;score_a = score_b = 0LL;idx_e = idx_o = 0;for (int i = 1; i <= n; i ++ ){scanf("%d", &a[i]);if (a[i] & 1)   old_a[++ idx_o] = a[i];else    even_a[++ idx_e] = a[i];}sort(even_a + 1, even_a + idx_e + 1, cmp);sort(old_a + 1, old_a + idx_o + 1, cmp);cur_o = 1, cur_e = 1;for (int i = 1; i <= n; i ++ ){if (Check())    // 奇数{if (i & 1){cur_o ++;}else{score_b += old_a[cur_o ++];}}else    // 偶数{if (i & 1){score_a += even_a[cur_e ++];}else{cur_e ++;}}}if (score_a == score_b) puts("Tie");else if (score_a > score_b) puts("Alice");else    puts("Bob");}return 0;
}

---

---

---

E Correct Placement

原题信息

解题思路

对于每一个$(h,w)$我们需要找到$(h_i,w_i),h_i<h,w_i<w$的下标，至于另一种情况我们直接 $swap(h,w)$即可，
主要是先对$(w,h)$进行排序二分查询 $h_i<h$的最大下标，找他的前缀最小值$w$(可以预处理)

AC代码

#include <bits/stdc++.h>
using namespace std;const int N = 200010;
const int INF = 0x3f3f3f3f;
class Node
{
public:int h, w;int idx;void operator=(const Node &t1){h = t1.h, w = t1.w, idx = t1.idx;}
}a[N], q[N];
int pre_w[N], pre_idx[N];
int n;bool cmp(const Node &t1, const Node &t2)
{if (t1.h == t2.h)return t1.w < t2.w;elsereturn t1.h < t2.h;
}int Cal2(int h, int w)
{int l = 1, r = n, mid;if (h <= q[l].h)  return -1;while (l < r){mid = l + r + 1 >> 1;if (q[mid].h < h){l = mid;}else{r = mid - 1;}}if (w > pre_w[l])   // 找到了一个高度小，宽度也小的return pre_idx[l];else    // 没有宽度小的return -1;
}int Cal(int h, int w)
{int res1 = Cal2(h, w), res2 = Cal2(w, h);if (res1 == -1 && res2 == -1)return -1;elsereturn max(res1, res2);
}int main()
{int t;  cin >> t;while (t -- ){scanf("%d", &n);for (int i = 1; i <= n; i ++ ){scanf("%d%d", &a[i].h, &a[i].w);a[i].idx = i;q[i] = a[i];}sort(q + 1, q + 1 + n, cmp);pre_w[0] = INF, pre_idx[0] = -1;for (int i = 1; i <= n; i ++ ){if (q[i].w < pre_w[i - 1]){pre_w[i] = q[i].w, pre_idx[i] = q[i].idx;}else{pre_w[i] = pre_w[i - 1], pre_idx[i] = pre_idx[i - 1];}}/*for (int i = 1; i <= n; i ++ )printf("h=%d, w=%d, idx=%d, pre_w=%d, pre_idx=%d\n", q[i].h, q[i].w, q[i].idx, pre_w[i], pre_idx[i]);*/printf("%d", Cal(a[1].h, a[1].w));for (int i = 2; i <= n; i ++ ){printf(" %d", Cal(a[i].h, a[i].w));}puts("");}return 0;
}

---

---

---

F New Year’s Puzzle

原题信息

http://codeforces.com/contest/1472/problem/F

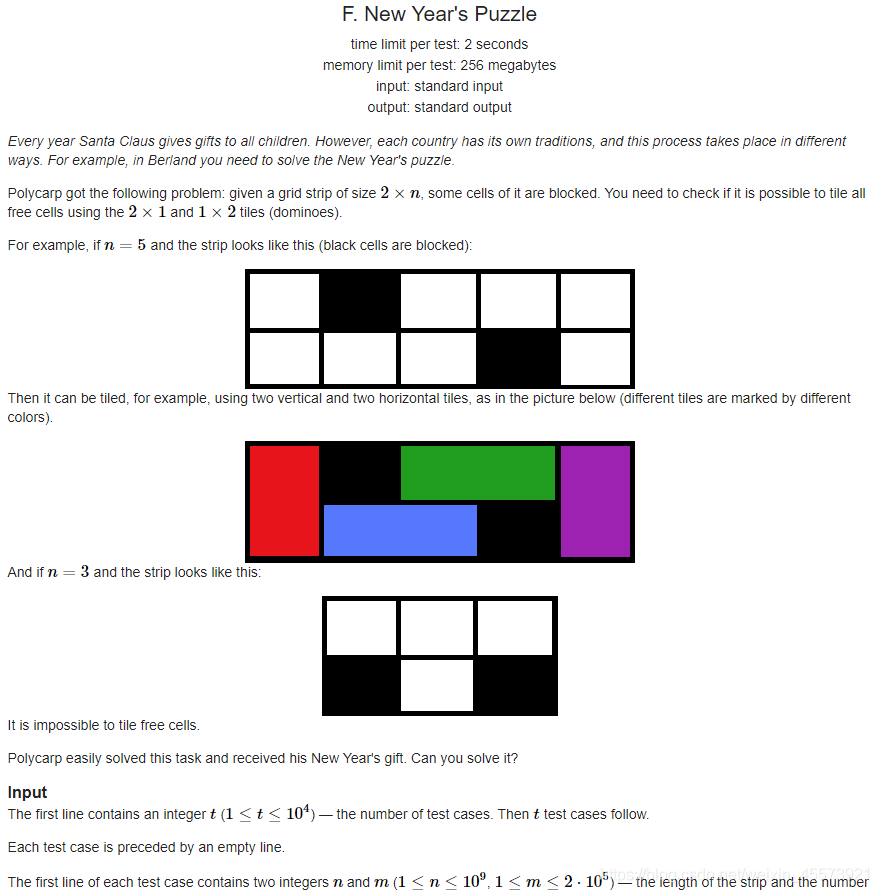
解题思路

首先第一个想到的就应该是对这些点按照列编号进行排序，然后我们逐个点进行处理。
用$cur1,cur2$表示当前第一行，第二行当前可以放置的最小列编号。下面我们对$BlockedCell$进行讨论

• $r1=r2$，即两个$BlockedCell$将一整列堵住，那么要求$abs(cur1-cur2)$%$2=0$，最后更新$cur1=cur2=r1+1$即可，这一次处理了两个$BlockedCell$问题
• $r1!=r2$,我们处理$BlockedCell(r1,c1)$，假设这个$BlockedCell$在$cur1$那一列。首先倘若$r1<cur1$指定不行，因为小于$cur1$的区域已经使用完毕
  那么如果$(r1-cur2)$%$2=0$，那么就说明这一行是可以自己解决的。
  即使是使用了下面的那一行，也会是成对的使用，与另外一列自己解决一样
  倘若$(r1-cur2)$%$2=1$，也就是说他需要另外一列的帮助，加奇数的竖线排列，下面我们只需考虑是否可以加（至少）一个竖线，在这里只需要考虑加一个就行，因为多加了也只能是奇数个，奇数个竖线，两两可以合并为横线,因为是加入一个，我们考虑一下最晚加入（在$cur-1$的位置）。
  为什么是最晚在$cur-1$？因为最晚就是在 BlockedCell之前加入！
  那么如何判断是否可以，那么只需在最晚加入的话，另一个列前方时候可以组成成对个二元组即可
  最后$cur1,cur2$注意更新，一个为$c1$，一个为$c1+1$
• 最后，我们还需考虑满足了$BlockedCell$之后的$cur1,cur2$是否可以在结尾处ok，因此检验一下
  $(cur1-cur2)$%$2=0$->成立，否则不成立

AC代码

#include <bits/stdc++.h>
using namespace std;const int M = 200010, INF = 0x3f3f3f3f;
int n, m;class Node
{
public:int r, c;
}q[M];bool cmp(const Node &t1, const Node &t2)
{if (t1.c == t2.c)return t1.r < t2.r;elsereturn t1.c < t2.c;
}bool Check()
{// if (m & 1)  return false;sort(q + 1, q + 1 + m, cmp);
/*for (int i = 1; i <= m; i ++ )printf("I=%d, r=%d, c=%d\n", i, q[i].r, q[i].c);
*/int r1, r2, c1, c2;int cur1 = 1, cur2 = 1;q[m + 1].c = INF;for (int i = 1; i <= m; i += 1){/// printf("I=%d, cur1=%d, cur2=%d\n", i, cur1, cur2);c1 = q[i].c, r1 = q[i].r, c2 = q[i + 1].c, r2 = q[i + 1].r;if (c1 == c2){/// puts("Equal");i ++;if (abs(cur1 - cur2) & 1)   // 口封不上return false;cur1 = cur2 = c1 + 1;}else{if (r1 == 1)    // 上面{/// puts("Up");if (cur1 > c1)  // 当前这个地方不能堵上return false;else{if ((c1 - cur1) % 2 == 0)   // 偶数，可以自行解决{cur1 = c1 + 1;}else    // 需要下面协助一个竖着的{cur1 = c1 - 1;if (cur1 - cur2 >= 0 && (cur1 - cur2) % 2 == 0)  // 下面可以跟上{cur1 = c1 + 1;cur2 = c1;}else{return false;}}}}else    // 下面{/// puts("Down");if (cur2 > c1)  // 当前这个地方不能堵上return false;else{if ((c1 - cur2) % 2 == 0)   // 偶数，可以自行解决{cur2 = c1 + 1;}else    // 需要上面协助一个竖着的{cur2 = c1 - 1;/*printf("Down, cur1=%d, cur2=%d\n", cur1, cur2);printf("%d\n", cur2 - cur1 >= 0);printf("%d\n", ((cur2 - cur1) % 2 == 0));*/if ((cur2 - cur1 >= 0) && ((cur2 - cur1) % 2 == 0))  // 上面可以跟上{cur2 = c1 + 1;cur1 = c1;}else{//puts("FALSE");return false;}}}}}}if (abs(cur1 - cur2) & 1)return false;elsereturn true;
}int main()
{int t;  cin >> t;while (t -- ){scanf("%d%d", &n, &m);for (int i = 1; i <= m; i ++ )scanf("%d%d", &q[i].r, &q[i].c);if (Check())puts("YES");elseputs("NO");}return 0;
}
/*
1
4 2
1 2
2 2
YES
*/
/*
1
4 3
1 1
1 2
2 2
NO
*/
/*
1
4 2
1 1
2 3
YES
*/
/*
3
5 2
2 2
1 4
3 2
2 1
2 3
6 4
2 1
2 3
2 4
2 6
*/

---

---

---

G Moving to the Capital

原题信息

http://codeforces.com/contest/1472/problem/G

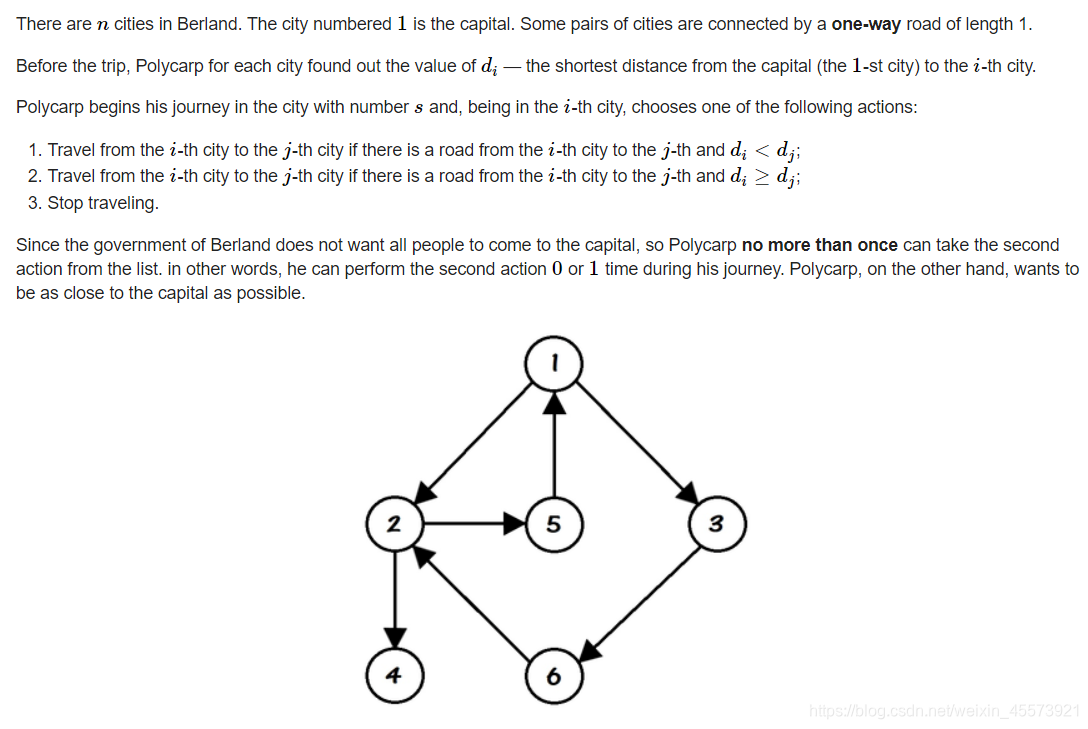
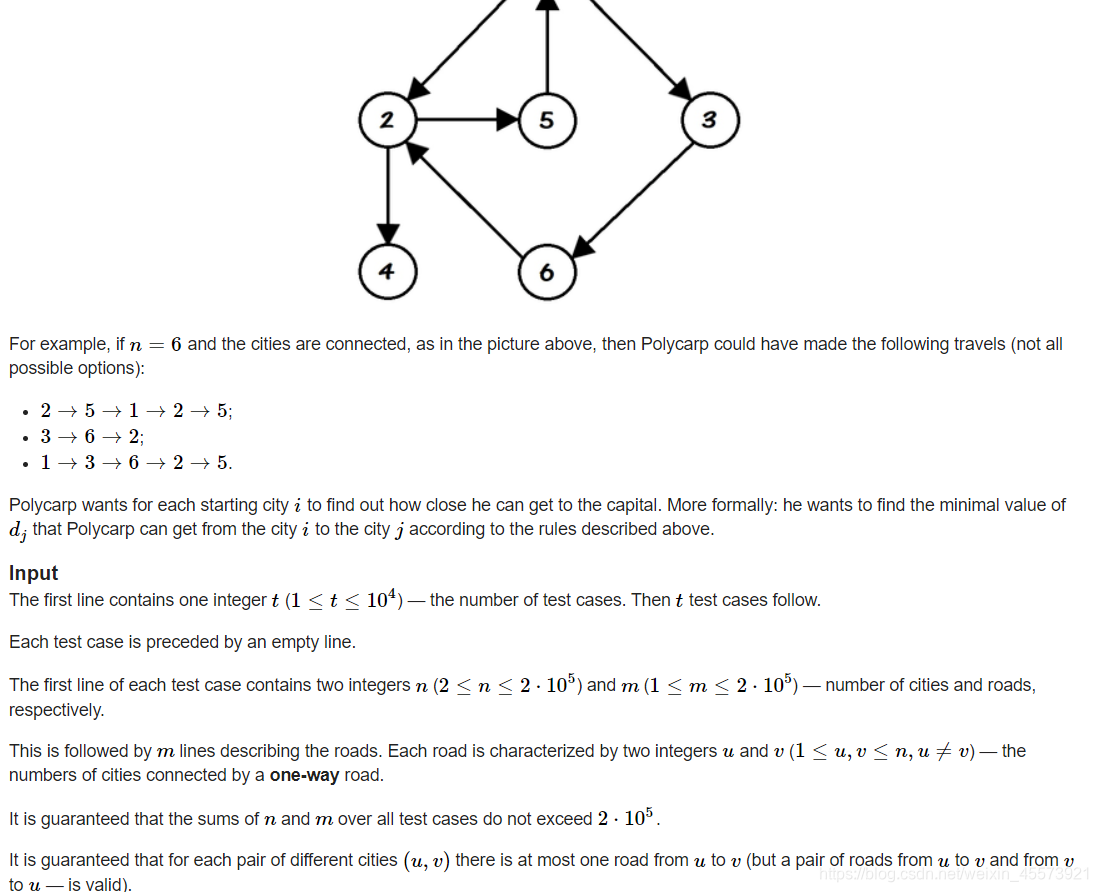
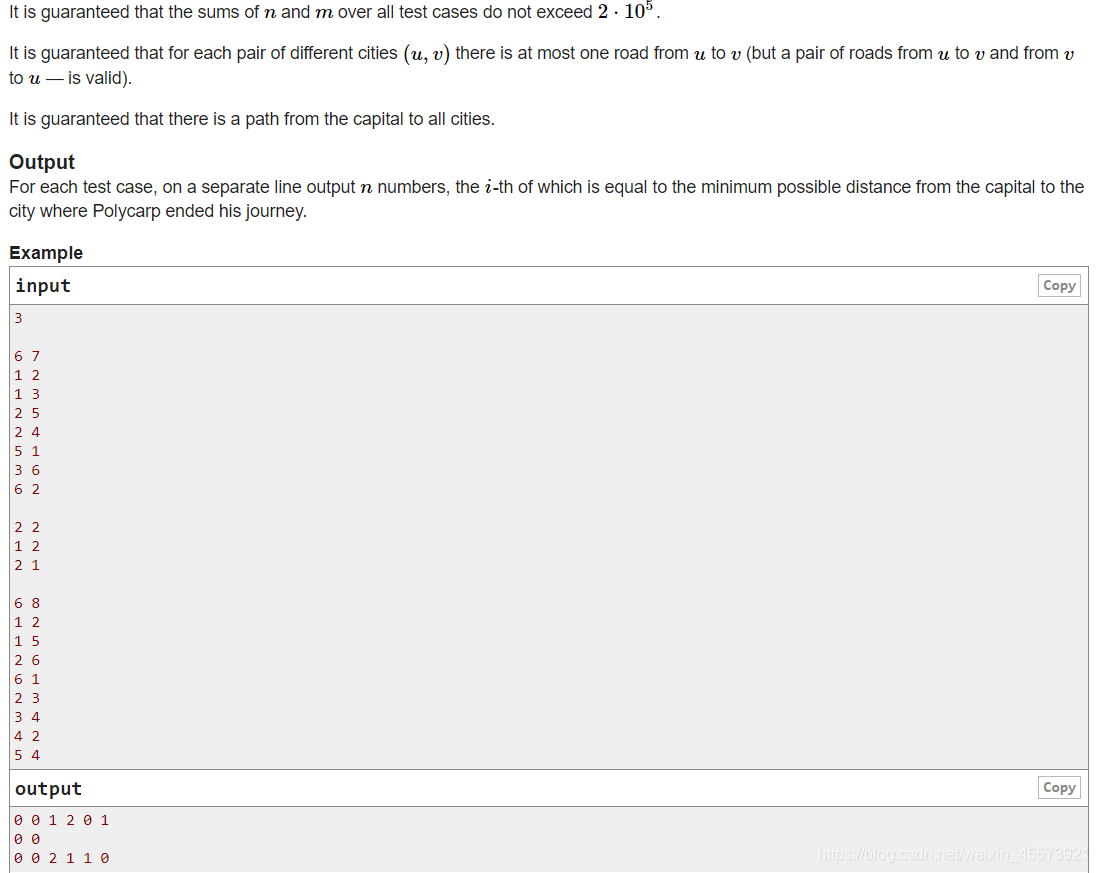
解题思路

一个很有意思的dp问题
首先我们先利用bfs求出起点到其它任意各点的举例，然后我们进行dp求解。

• dp思路一
  $f_{i,0}$表示从$i$点开始，使用0次规则2可以达到的最小$d$,(其实就是自身的$d$)
  $f_{i,1}$表示从$i$点开始，使用1次规则2可以达到的最小$d$
  下面我们来分析一下$f_{i,1}$如何进行更新，
  $$f_{i,1}=\{\begin{array}{ll}{f}_{j,0}& \exists i\Rightarrow j边且d_i\ge d_j\\ f_{j,1}& \exists i\Rightarrow j边且d_i<d_j\end{array}$$
  $f_{j,0}$容易保证，因为直接就是$d_j$，但是我们如何保证使用的$f_{j,1}$是更新完毕的最终结果呢？ 下面我们考虑$f_{j,1}$如何使其尽可能早的确定，而且不会使用到未确定的$f_{j,1}$，我们从$d_i,d_j$在分段函数的关系中，就可以发现，倘若我们对$d$的距离进行降序排序，即先进性距离更大的$dp$，那么就可以是得使用到的$f_{j,1}$都会是前面进行处理完毕的，因此进行$dp$的算法如下代码

• Algo

• 首先$bfs$处理出各点的距离$d_i$

• 处理出$d_i$之后，我们就可以进行按照距离进行非递增排序。

• 按照分段函数进行$dp$

AC代码

#include <bits/stdc++.h>
using namespace std;const int N = 200010, INF = 0x3f3f3f3f;
int f[N];
int h[N], e[N], ne[N], idx;
int n, m;
bool st[N];
int dist[N];
vector<int> order;void Initial()
{// edge initialmemset(h, -1, sizeof h);idx = 0;// dp initialmemset(f, 0x3f, sizeof f);// dist initialmemset(dist, -1, sizeof dist);// orderorder.clear();
}void add(int a, int b)
{e[idx] = b, ne[idx] = h[a], h[a] = idx ++;
}int main()
{int t;  cin >> t;while (t -- ){// inputscanf("%d%d", &n, &m);// initialInitial();for (int i = 1, u, v; i <= m; i ++ ){scanf("%d%d", &u, &v);add(u, v);}// bfs for distqueue<int> que;que.push(1);    dist[1] = 0;order.push_back(1);int u, v;while (que.size()){u = que.front();    que.pop();for (int i = h[u]; ~i; i = ne[i]){v = e[i];if (dist[v] == -1){dist[v] = dist[u] + 1;que.push(v);order.push_back(v);}}}/*for (int i = 1; i <= n; i ++ )printf("i=%d dist=%d\n", i, dist[i]);*/// dfs for dp/*从距离最远的开始，进行枚举dp*/for (int j = n - 1, u, v; j >= 0; j -- ){u = order[j];f[u] = dist[u];for (int i = h[u]; ~i; i = ne[i]){v = e[i];if (dist[u] >= dist[v])  // u -> v 需要耗费一次机会, 只可以 取dist{f[u] = min(f[u], dist[v]);}else    // 不需要消耗机会，直接最优子结构{f[u] = min(f[u], f[v]);}}}// output/*for (int i = 1; i <= n; i ++ )printf("i=%d, f[i][0]=%d, f[i][1]=%d\n", i, f[i][0], f[i][1]);*/printf("%d", 0);for (int i = 2; i <= n; i ++ )printf(" %d", f[i]);puts("");}return 0;
}

---

---

---