文章目录
- 归并排序
- 递归方法实现
- 非递归方法实现
- 求数组的小和
- 求数组的降序对个数
- 快排
- 荷兰国旗问题(Partition过程)
- 快排1.0
- 快排2.0
- 快排3.0
- 堆
- 大根堆
- 堆排序
- 使用堆排序
归并排序
递归方法实现
public class Code01_MergeSort {// 递归方法实现public static void mergeSort1(int[] arr) {if (arr == null || arr.length < 2) {return;}process(arr, 0, arr.length - 1);}// 请把arr[L..R]排有序// l...r N// T(N) = 2 * T(N / 2) + O(N)// O(N * logN)public static void process(int[] arr, int L, int R) {if (L == R) { // base casereturn;}int mid = L + ((R - L) >> 1);process(arr, L, mid);process(arr, mid + 1, R);merge(arr, L, mid, R);}public static void merge(int[] arr, int L, int M, int R) {int[] help = new int[R - L + 1];int i = 0;int p1 = L;int p2 = M + 1;while (p1 <= M && p2 <= R) {help[i++] = arr[p1] <= arr[p2] ? arr[p1++] : arr[p2++];}// 要么p1越界了,要么p2越界了while (p1 <= M) {help[i++] = arr[p1++];}while (p2 <= R) {help[i++] = arr[p2++];}for (i = 0; i < help.length; i++) {arr[L + i] = help[i];}}}
非递归方法实现
public static void mergeSort2(int[] arr) {if (arr == null || arr.length < 2) {return;}int N = arr.length;// 步长int mergeSize = 1;while (mergeSize < N) { // log N// 当前左组的,第一个位置int L = 0;while (L < N) {if (mergeSize >= N - L) {break;}int M = L + mergeSize - 1;int R = M + Math.min(mergeSize, N - M - 1);merge(arr, L, M, R);L = R + 1;}// 防止溢出if (mergeSize > N / 2) {break;}mergeSize <<= 1;}
}
求数组的小和
在一个数组中,一个数数左边比它小的数的总和,叫数的小和,所有数的小和累加起来,叫数组的小和
(一个数右边有多少个数比他 大)
public class Code02_SmallSum {public static int smallSum(int[] arr) {if (arr == null || arr.length < 2) {return 0;}return process(arr, 0, arr.length - 1);}// arr[L..R]既要排好序,也要求小和返回// 所有merge时,产生的小和,累加// 左 排序 merge// 右 排序 merge// mergepublic static int process(int[] arr, int l, int r) {if (l == r) {return 0;}// l < rint mid = l + ((r - l) >> 1);return process(arr, l, mid) + process(arr, mid + 1, r) + merge(arr, l, mid, r);}public static int merge(int[] arr, int L, int m, int r) {int[] help = new int[r - L + 1];int i = 0;int p1 = L;int p2 = m + 1;int res = 0;while (p1 <= m && p2 <= r) {res += arr[p1] < arr[p2] ? (r - p2 + 1) * arr[p1] : 0;help[i++] = arr[p1] < arr[p2] ? arr[p1++] : arr[p2++];}while (p1 <= m) {help[i++] = arr[p1++];}while (p2 <= r) {help[i++] = arr[p2++];}for (i = 0; i < help.length; i++) {arr[L + i] = help[i];}return res;}
}
求数组的降序对个数
(一个数左边有多少个数比他 大)
public class Code03_ReversePair {public static int reverPairNumber(int[] arr) {if (arr == null || arr.length < 2) {return 0;}return process(arr, 0, arr.length - 1);}// arr[L..R]既要排好序,也要求逆序对数量返回// 所有merge时,产生的逆序对数量,累加,返回// 左 排序 merge并产生逆序对数量// 右 排序 merge并产生逆序对数量public static int process(int[] arr, int l, int r) {if (l == r) {return 0;}// l < rint mid = l + ((r - l) >> 1);return process(arr, l, mid) + process(arr, mid + 1, r) + merge(arr, l, mid, r);}public static int merge(int[] arr, int L, int m, int r) {int[] help = new int[r - L + 1];int i = help.length - 1;int p1 = m;int p2 = r;int res = 0;while (p1 >= L && p2 > m) {res += arr[p1] > arr[p2] ? (p2 - m) : 0;help[i--] = arr[p1] > arr[p2] ? arr[p1--] : arr[p2--];}while (p1 >= L) {help[i--] = arr[p1--];}while (p2 > m) {help[i--] = arr[p2--];}for (i = 0; i < help.length; i++) {arr[L + i] = help[i];}return res;}
}
时间复杂度 N*logN
快排
荷兰国旗问题(Partition过程)
public class Code02_PartitionAndQuickSort {public static void swap(int[] arr, int i, int j) {int tmp = arr[i];arr[i] = arr[j];arr[j] = tmp;}// arr[L..R]上,以arr[R]位置的数做划分值// <= X > X// <= X Xpublic static int partition(int[] arr, int L, int R) {if (L > R) {return -1;}if (L == R) {return L;}int lessEqual = L - 1;int index = L;while (index < R) {if (arr[index] <= arr[R]) {swap(arr, index, ++lessEqual);}index++;}swap(arr, ++lessEqual, R);return lessEqual;}// arr[L...R] 玩荷兰国旗问题的划分,以arr[R]做划分值// <arr[R] ==arr[R] > arr[R]public static int[] netherlandsFlag(int[] arr, int L, int R) {if (L > R) { // L...R L>Rreturn new int[] { -1, -1 };}if (L == R) {return new int[] { L, R };}int less = L - 1; // < 区 右边界int more = R; // > 区 左边界int index = L;while (index < more) { // 当前位置,不能和 >区的左边界撞上if (arr[index] == arr[R]) {index++;} else if (arr[index] < arr[R]) {
// swap(arr, less + 1, index);
// less++;
// index++; swap(arr, index++, ++less);} else { // >swap(arr, index, --more);}}swap(arr, more, R); // <[R] =[R] >[R]return new int[] { less + 1, more };}}
快排1.0
O(N^2)
<= 和> 两个区域
public static void quickSort1(int[] arr) {if (arr == null || arr.length < 2) {return;}process1(arr, 0, arr.length - 1);}public static void process1(int[] arr, int L, int R) {if (L >= R) {return;}// L..R partition arr[R] [ <=arr[R] arr[R] >arr[R] ]int M = partition(arr, L, R);process1(arr, L, M - 1);process1(arr, M + 1, R);}
快排2.0
O(N^2)
相比于1.0,< = > 三个区域
public static void quickSort2(int[] arr) {if (arr == null || arr.length < 2) {return;}process2(arr, 0, arr.length - 1);}// arr[L...R] 排有序,快排2.0方式public static void process2(int[] arr, int L, int R) {if (L >= R) {return;}// [ equalArea[0] , equalArea[0]]int[] equalArea = netherlandsFlag(arr, L, R);process2(arr, L, equalArea[0] - 1);process2(arr, equalArea[1] + 1, R);}
快排3.0
O(NlogN)
相比于2.0,在数组中随机选择一个数,和arr[R]交换,开始2.0
public static void quickSort3(int[] arr) {if (arr == null || arr.length < 2) {return;}process3(arr, 0, arr.length - 1);}public static void process3(int[] arr, int L, int R) {if (L >= R) {return;}swap(arr, L + (int) (Math.random() * (R - L + 1)), R);int[] equalArea = netherlandsFlag(arr, L, R);process3(arr, L, equalArea[0] - 1);process3(arr, equalArea[1] + 1, R);}

堆
结构上:完全二叉树
大根堆
public class Code02_Heap {public static class MyMaxHeap {private int[] heap;private final int limit;private int heapSize;public MyMaxHeap(int limit) {heap = new int[limit];this.limit = limit;heapSize = 0;}public boolean isEmpty() {return heapSize == 0;}public boolean isFull() {return heapSize == limit;}public void push(int value) {if (heapSize == limit) {throw new RuntimeException("heap is full");}heap[heapSize] = value;// value heapSizeheapInsert(heap, heapSize++);}// 用户此时,让你返回最大值,并且在大根堆中,把最大值删掉// 剩下的数,依然保持大根堆组织public int pop() {int ans = heap[0];swap(heap, 0, --heapSize);heapify(heap, 0, heapSize);return ans;}// 新加进来的数,现在停在了index位置,请依次往上移动,// 移动到0位置,或者干不掉自己的父亲了,停!private void heapInsert(int[] arr, int index) {// [index] [index-1]/2// index == 0while (arr[index] > arr[(index - 1) / 2]) {swap(arr, index, (index - 1) / 2);index = (index - 1) / 2;}}// 从index位置,往下看,不断的下沉// 停:较大的孩子都不再比index位置的数大;已经没孩子了private void heapify(int[] arr, int index, int heapSize) {int left = index * 2 + 1;while (left < heapSize) { // 如果有左孩子,有没有右孩子,可能有可能没有!// 把较大孩子的下标,给largestint largest = left + 1 < heapSize && arr[left + 1] > arr[left] ? left + 1 : left;largest = arr[largest] > arr[index] ? largest : index;if (largest == index) {break;}// index和较大孩子,要互换swap(arr, largest, index);index = largest;left = index * 2 + 1;}}private void swap(int[] arr, int i, int j) {int tmp = arr[i];arr[i] = arr[j];arr[j] = tmp;}}
}
堆排序
public class Code03_HeapSort {// 堆排序额外空间复杂度O(1)public static void heapSort(int[] arr) {if (arr == null || arr.length < 2) {return;}// O(N*logN)
// for (int i = 0; i < arr.length; i++) { // O(N)
// heapInsert(arr, i); // O(logN)
// }// O(N)for (int i = arr.length - 1; i >= 0; i--) {heapify(arr, i, arr.length);}int heapSize = arr.length;swap(arr, 0, --heapSize);// O(N*logN)while (heapSize > 0) { // O(N)heapify(arr, 0, heapSize); // O(logN)swap(arr, 0, --heapSize); // O(1)}}// arr[index]刚来的数,往上public static void heapInsert(int[] arr, int index) {while (arr[index] > arr[(index - 1) / 2]) {swap(arr, index, (index - 1) / 2);index = (index - 1) / 2;}}// arr[index]位置的数,能否往下移动public static void heapify(int[] arr, int index, int heapSize) {int left = index * 2 + 1; // 左孩子的下标while (left < heapSize) { // 下方还有孩子的时候// 两个孩子中,谁的值大,把下标给largest// 1)只有左孩子,left -> largest// 2) 同时有左孩子和右孩子,右孩子的值<= 左孩子的值,left -> largest// 3) 同时有左孩子和右孩子并且右孩子的值> 左孩子的值, right -> largestint largest = left + 1 < heapSize && arr[left + 1] > arr[left] ? left + 1 : left;// 父和较大的孩子之间,谁的值大,把下标给largestlargest = arr[largest] > arr[index] ? largest : index;if (largest == index) {break;}swap(arr, largest, index);index = largest;left = index * 2 + 1;}}public static void swap(int[] arr, int i, int j) {int tmp = arr[i];arr[i] = arr[j];arr[j] = tmp;}
}
使用堆排序
已知一个几乎有序的数组。几乎有序是指,如果把数组排好顺序的话,每个元素移动的距离一定不超过k,并且k相对于数组长度来说是比较小的(时间复杂度:N*logK)。
// 默认小根堆
PriorityQueue<Integer> heap = new PriorityQueue<>();
public class Code04_SortArrayDistanceLessK {public static void sortedArrDistanceLessK(int[] arr, int k) {if (k == 0) {return;}// 默认小根堆PriorityQueue<Integer> heap = new PriorityQueue<>();int index = 0;// 0...K-1for (; index <= Math.min(arr.length - 1, k - 1); index++) {heap.add(arr[index]);}int i = 0;for (; index < arr.length; i++, index++) {heap.add(arr[index]);arr[i] = heap.poll();}while (!heap.isEmpty()) {arr[i++] = heap.poll();}}
}
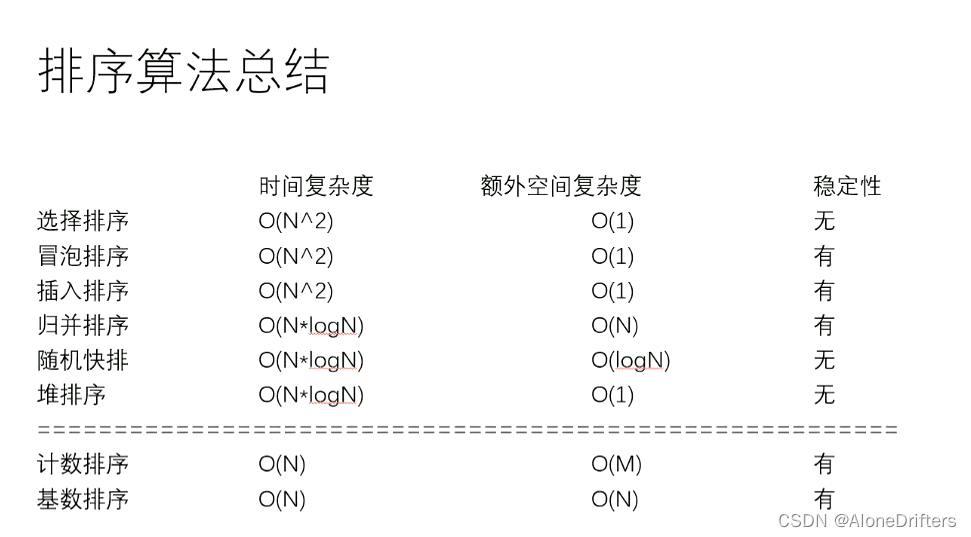

——快乐纪中)



)




)








