二叉查找树转顺序排列双向链表

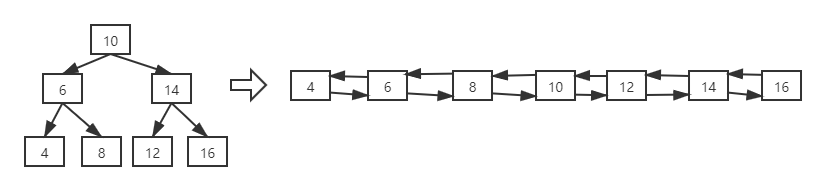
• 题目：输入一颗二叉查找树，将二叉查找树转成一个排序的双向链表，要求不能创建任何新节点，只调整树节点中指针的指向。例如下图所示：
  
• 本次二叉查找树节点定义使用之前文章 数据结构与算法–二叉查找树实现原理中节点定义，下文中相关api实现同样使用该文中的方法，文中对二叉查找树相关的api有详细的说明推演，如有问题可到文中查看。节点实例如下：

/*** 二叉树节点对象定义** @author liaojiamin* @Date:Created in 15:24 2020/12/11*/
public class BinaryNode implements Comparable {private Object element;private BinaryNode left;private BinaryNode right;private int count;
............

• 理论基础：

  • 二叉树中节点与双向链表的节点都存在两个指针，只是指向不同而已。
  • 二叉搜索树中左子节点总是小于主节点，右子节点总是大于主节点，在中序遍历中就是顺序的将每个节点读取
  • 那么我们通过中序遍历的思想将二叉查找树转顺序双向链表，将原先指向左节点的指针调整为链表的指向前一个节点，指向右节点的指针指向后一个节点
  • 如上方式得到我们转换的结果

• 问题分析：

  • 有关树的题型我们必然会想到递归的，有树遍历必有递归，再者递归必然只考虑最简单情况，这样不至于将自己绕进去
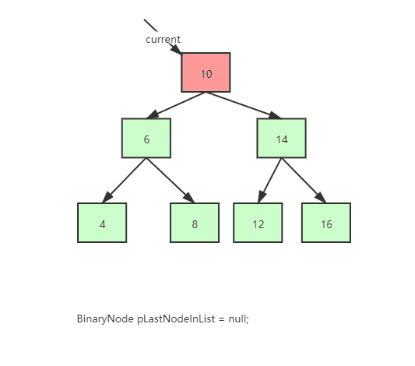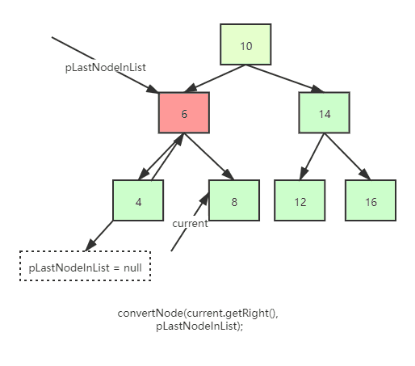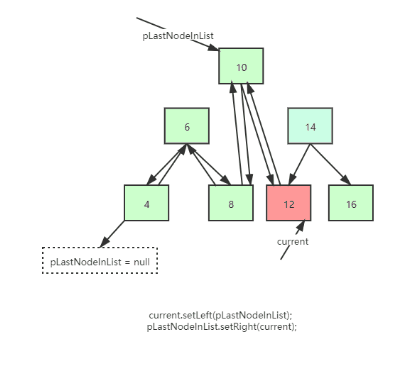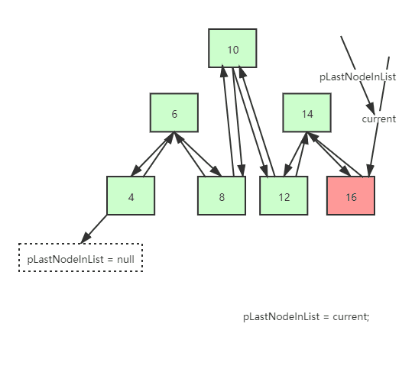
  • 考虑三层节点最简单情况，如上图中，分三部分 10 根节点，6 为根节点的左子树，14 为根节点的右子树
  • 根据链表定义，10 节点将和左子树的8 链接，和右子树的12 链接
  • 也就是中序遍历的顺序 （4，6，8）–> 10 -->（12,14，16）
  • 左子树为例，只需要增加两个指针指向，4–>6, 8–>6 两个指针，最后返回8 节点
  • 记录当前节点为current，pLastNodeInList指向需要改变指向的节点，当递归到current = 6，左子树pLastNodeInList指向最小节点4，只需要增加指针指向current.setleft = pLastNodeInList && pLastNodeInList.right=current，其实第一个是已经存在的，但是仅仅是左节点情况存在，我们左右节点统一处理，这样用一个递归解决。接着回到上一节点pLastNodeInList = current = 6，
  • 继续递归右子树，current=8, current.left = pLastNodeInList = 6 && pLastNodeInList .right = current， 此处第二步骤指针已经存在，同样统一处理。
  • 那么我们将每一个左，右子树用同样规则递归处理得到最终的结果，我们最终返回pLastNodeInList ，指向root节点
  • 在递归left方向得到链表头

• 图解分析：

• 通过如上分析有如下代码：

/*** 二叉查找树转顺序排列的双向链表** @author liaojiamin* @Date:Created in 14:27 2021/5/19*/
public class ConvertBinaryToList {public static void main(String[] args) {BinaryNode node = new BinaryNode(null, null, null);BinarySearchTree searchTree = new BinarySearchTree();Random random = new Random();for (int i = 0; i < 8; i++) {node = searchTree.insert(random.nextInt(100), node);}printMiddle(node);BinaryNode doubleLink = convertBinary(node);BinaryNode headNode = doubleLink;while (headNode.getLeft() != null) {headNode = headNode.getLeft();}while (headNode.getRight() != null) {System.out.print("value: " + headNode.getElement());System.out.print("left: " + (headNode.getLeft() != null ? headNode.getLeft().getElement() : ""));System.out.print("right: " + (headNode.getRight() != null ? headNode.getRight().getElement() : ""));System.out.println();headNode = headNode.getRight();if(headNode.getRight() == null ){System.out.print("value: " + headNode.getElement());System.out.print("left: " + (headNode.getLeft() != null ? headNode.getLeft().getElement() : ""));System.out.print("right: " + (headNode.getRight() != null ? headNode.getRight().getElement() : ""));}}}/*** 二叉查找树转双向链表，顺序* 递归处理后，找出最左节点*/public static BinaryNode convertBinary(BinaryNode root) {BinaryNode pLastNodeInList = null;pLastNodeInList = convertNode(root, pLastNodeInList);BinaryNode pHeadOfLast = pLastNodeInList;while (pHeadOfLast != null && pHeadOfLast.getLeft() != null) {pHeadOfLast = pHeadOfLast.getLeft();}return pHeadOfLast;}public static BinaryNode convertNode(BinaryNode node, BinaryNode pLastNodeInList) {if (node == null) {return pLastNodeInList;}BinaryNode current = node;if (current.getLeft() != null) {//递归左节点pLastNodeInList = convertNode(current.getLeft(), pLastNodeInList);}//此时node节点是根，将左子树的最大节点pLastNodeInList 设置为根的left节点current.setLeft(pLastNodeInList);if (pLastNodeInList != null) {//此时的pLastNodeInList 是左子树的最大节点，他的right应该是root，也就是当前的currentpLastNodeInList.setRight(current);}//此时左节点干完，左节点的最大节点是当前root也就是currentpLastNodeInList = current;//递归右节点if (current.getRight() != null) {pLastNodeInList = convertNode(current.getRight(), pLastNodeInList);}return pLastNodeInList;}/*** 中序遍历*/public static void printMiddle(BinaryNode root) {if (root == null || root.getElement() == null) {return;}printMiddle(root.getLeft());System.out.println(root.getElement());printMiddle(root.getRight());}}

上一篇：数据结构与算法–复杂链表的复制
下一篇：数据结构与算法–字符串的排列组合问题