907. 子数组的最小值之和（单调栈+贡献法）

https://leetcode.cn/problems/sum-of-subarray-minimums/description/?envType=daily-question&envId=2023-11-27

提示：

1 <= arr.length <= 3 * 10^4
1 <= arr[i] <= 3 * 10^4

class Solution {private static final int MOD = (int)1e9 + 7;public int sumSubarrayMins(int[] arr) {long ans = 0;           // 使用long总归是有利于避免溢出int n = arr.length;// 计算每个数字作为最小值的贡献，即找到左右两侧第一个小于它的（一边严格小于，另一边小于等于）Deque<Integer> stk = new ArrayDeque();int[] right = new int[n], left = new int[n];Arrays.fill(left, -1);Arrays.fill(right, n);for (int i = 0; i < n; ++i) {while (!stk.isEmpty() && arr[i] <= arr[stk.peek()]) {right[stk.pop()] = i;}if (!stk.isEmpty()) left[i] = stk.peek();stk.push(i);}for (int i = 0; i < n; ++i) {ans = (ans + (long)arr[i] * (right[i] - i) * (i - left[i])) % MOD;}return (int)ans;}
}

1670. 设计前中后队列⭐（设计数据结构）

https://leetcode.cn/problems/design-front-middle-back-queue/description/?envType=daily-question&envId=2023-11-28

提示：

1 <= val <= 10^9
最多调用 1000 次 pushFront， pushMiddle， pushBack， popFront， popMiddle 和 popBack 。

解法1——双向链表

自己写的代码如下：

class FrontMiddleBackQueue {Node head = new Node(), tail = new Node();int sz = 0;public FrontMiddleBackQueue() {head.next = tail;tail.prev = head;}public void pushFront(int val) {Node node = new Node(val, head, head.next);head.next.prev = node;head.next = node;sz++;}public void pushMiddle(int val) {Node cur = head;for (int i = 0; i < sz / 2; ++i) {cur = cur.next;}Node node = new Node(val, cur, cur.next);cur.next.prev = node;cur.next = node;sz++;}public void pushBack(int val) {Node node = new Node(val, tail.prev, tail);tail.prev.next = node;tail.prev = node;sz++;}public int popFront() {if (sz == 0) return -1;int res = head.next.val;head.next.next.prev = head;head.next = head.next.next;--sz;return res;}public int popMiddle() {if (sz == 0) return -1;Node cur = head;for (int i = 0; i < (sz - 1) / 2; ++i) cur = cur.next;int res = cur.next.val;cur.next.next.prev = cur;cur.next = cur.next.next;--sz;return res;}public int popBack() {if (sz == 0) return -1;int res = tail.prev.val;tail.prev.prev.next = tail;tail.prev = tail.prev.prev;--sz;return res;}
}class Node {int val = -1;Node prev = null, next = null;Node() {};Node(int _val, Node _prev, Node _next) {this.val = _val;this.prev = _prev;this.next = _next;}
}/*** Your FrontMiddleBackQueue object will be instantiated and called as such:* FrontMiddleBackQueue obj = new FrontMiddleBackQueue();* obj.pushFront(val);* obj.pushMiddle(val);* obj.pushBack(val);* int param_4 = obj.popFront();* int param_5 = obj.popMiddle();* int param_6 = obj.popBack();*/

一种优化方法是额外一个指针指向中间节点，参见：https://leetcode.cn/problems/design-front-middle-back-queue/solutions/502014/she-ji-qian-zhong-hou-dui-lie-by-zerotrac2/?envType=daily-question&envId=2023-11-28 的方法二。

解法2——两个双端队列

参考官方题解的思想 和 0x3f的代码写的。

class FrontMiddleBackQueue {// 左边的双端队列和右边的双端队列等长 或恰好大1。可以保证中间节点是左队列的结尾节点Deque<Integer> left = new ArrayDeque<>(), right = new ArrayDeque<>();public FrontMiddleBackQueue() {}public void pushFront(int val) {left.addFirst(val);balance();}public void pushMiddle(int val) {if (left.size() > right.size()) right.offerFirst(left.pollLast());left.offerLast(val);}public void pushBack(int val) {right.offerLast(val);balance();}public int popFront() {if (left.isEmpty()) return -1;int res = left.pollFirst();balance();return res;}public int popMiddle() {if (left.isEmpty()) return -1;int res = left.pollLast();balance();return res;}public int popBack() {if (left.isEmpty()) return -1;int res = right.isEmpty()? left.pollLast(): right.pollLast();balance();return res;}// 保持两个队列的相对大小public void balance() {if (left.size() > right.size() + 1) {right.offerFirst(left.pollLast());} else if (left.size() < right.size()) {left.offerLast(right.pollFirst());}}
}/*** Your FrontMiddleBackQueue object will be instantiated and called as such:* FrontMiddleBackQueue obj = new FrontMiddleBackQueue();* obj.pushFront(val);* obj.pushMiddle(val);* obj.pushBack(val);* int param_4 = obj.popFront();* int param_5 = obj.popMiddle();* int param_6 = obj.popBack();*/

2336. 无限集中的最小数字

https://leetcode.cn/problems/smallest-number-in-infinite-set/description/?envType=daily-question&envId=2023-11-29

解法1——维护最小变量mn 和 哈希表维护已经去掉的数字

维护最小变量mn，哈希表记录已经去除的数字。
当新添加数字时，如果更小，则更新 mn 变量。

class SmallestInfiniteSet {int mn = 1;Set<Integer> mv = new HashSet<>();public SmallestInfiniteSet() {}public int popSmallest() {int res = mn;mv.add(mn);while (mv.contains(mn)) mn++;return res;}public void addBack(int num) {if (mv.contains(num)) {mv.remove(num);if (num < mn) mn = num;}}
}/*** Your SmallestInfiniteSet object will be instantiated and called as such:* SmallestInfiniteSet obj = new SmallestInfiniteSet();* int param_1 = obj.popSmallest();* obj.addBack(num);*/

解法2——维护原本可用的最小值 和 有序集合维护后期添加的数值👌

去除时 是 去除最小的。
添加时 也只能添加 当前没有的，也是小的。

用一个变量维护上界，用一个有序集合维护新加的可用小数据。

class SmallestInfiniteSet {private int thres;private TreeSet<Integer> set;public SmallestInfiniteSet() {thres = 1;set = new TreeSet<Integer>();}public int popSmallest() {if (set.isEmpty()) {int ans = thres;++thres;return ans;}int ans = set.pollFirst();return ans;}public void addBack(int num) {if (num < thres) {set.add(num);}}
}

1657. 确定两个字符串是否接近（理解操作本质）⭐

https://leetcode.cn/problems/determine-if-two-strings-are-close/description/?envType=daily-question&envId=2023-11-30

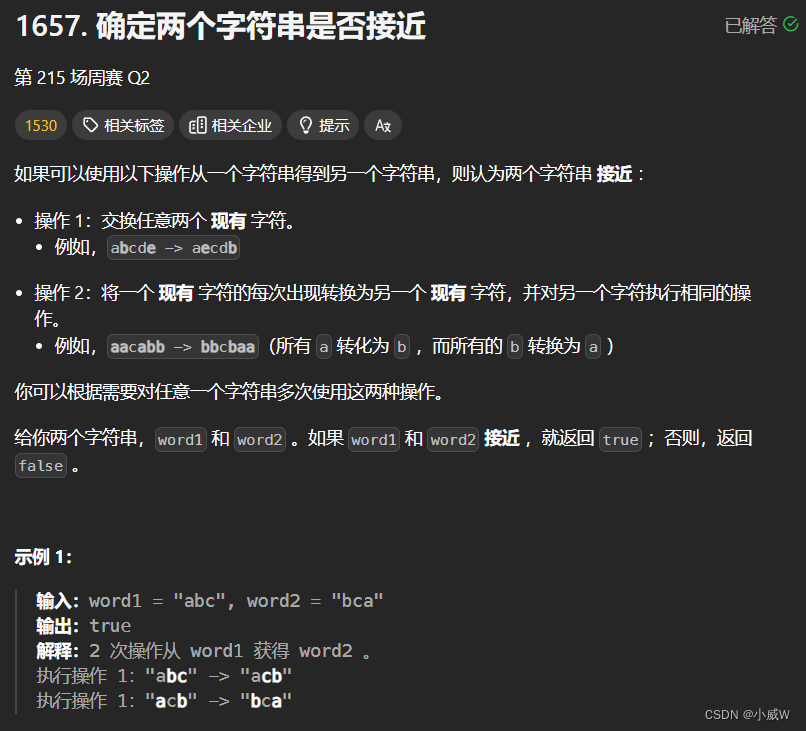
提示：
1 <= word1.length, word2.length <= 10^5
word1 和 word2 仅包含小写英文字母

https://leetcode.cn/problems/determine-if-two-strings-are-close/solutions/2547579/li-jie-cao-zuo-ben-zhi-jian-ji-xie-fa-py-b18i/?envType=daily-question&envId=2023-11-30

class Solution {public boolean closeStrings(String word1, String word2) {if (word1.length() != word2.length()) return false;int sMask = 0, tMask = 0;int[] sCnt = new int[26], tCnt = new int[26];for (char ch: word1.toCharArray()) {sMask |= 1 << (ch - 'a');sCnt[ch - 'a']++;}for (char ch: word2.toCharArray()) {tMask |= 1 << (ch - 'a');tCnt[ch - 'a']++;}Arrays.sort(sCnt);Arrays.sort(tCnt);return sMask == tMask && Arrays.equals(sCnt, tCnt);}
}

2661. 找出叠涂元素（哈希表、计数统计）

https://leetcode.cn/problems/first-completely-painted-row-or-column/description/?envType=daily-question&envId=2023-12-01

提示：

m == mat.length
n = mat[i].length
arr.length == m * n
1 <= m, n <= 10^5
1 <= m * n <= 10^5
1 <= arr[i], mat[r][c] <= m * n
arr 中的所有整数 互不相同
mat 中的所有整数 互不相同

class Solution {public int firstCompleteIndex(int[] arr, int[][] mat) {int m = mat.length, n = mat[0].length;// 记录位置信息int[] c = new int[m * n + 1], r = new int[m * n + 1];for (int i = 0; i < m; ++i) {for (int j = 0; j < n; ++j) {r[mat[i][j]] = i;c[mat[i][j]] = j;}}// 计数统计int[] cc = new int[n], rc = new int[m];for (int i = 0; i < m * n; ++i) {int v = arr[i], row = r[v], col = c[v];cc[col]++;rc[row]++;if (cc[col] == m || rc[row] == n) return i;}return -1;}
}

1094. 拼车（差分）

https://leetcode.cn/problems/car-pooling/description/?envType=daily-question&envId=2023-12-02

提示：

1 <= trips.length <= 1000
trips[i].length == 3
1 <= numPassengersi <= 100
0 <= fromi < toi <= 1000
1 <= capacity <= 10^5

用差分 表示 from 到 to 的范围内增加了多少人，然后再还原。

class Solution {public boolean carPooling(int[][] trips, int capacity) {int[] diff = new int[1001];for (int[] t: trips) {diff[t[1]] += t[0];diff[t[2]] -= t[0];}int s = 0;for (int i = 0; i < 1001; ++i) {s += diff[i];if (s > capacity) return false;}return true;}
}

1423. 可获得的最大点数（滑动窗口）

https://leetcode.cn/problems/maximum-points-you-can-obtain-from-cards/description/?envType=daily-question&envId=2023-12-03

提示：
1 <= cardPoints.length <= 10^5
1 <= cardPoints[i] <= 10^4
1 <= k <= cardPoints.length

就是找到长度为 n - k ，和最小的窗口，答案是 sum - mn。

class Solution {public int maxScore(int[] cardPoints, int k) {int n = cardPoints.length, sum = 0, s = 0, mn = Integer.MAX_VALUE / 2;if (k == n) return Arrays.stream(cardPoints).sum();k = n - k;for (int l = 0, r = 0; r < n; ++r) {s += cardPoints[r];sum += cardPoints[r];if (r - l + 1 == k) {mn = Math.min(mn, s);s -= cardPoints[l++];}}return sum - mn;}
}