LeetCode：1483. 树节点的第 K 个祖先（倍增 Java）

1483. 树节点的第 K 个祖先

题目描述：

实现代码与解析：

倍增

原理思路：

1483. 树节点的第 K 个祖先

题目描述：

给你一棵树，树上有 n 个节点，按从 0 到 n-1 编号。树以父节点数组的形式给出，其中 parent[i] 是节点 i 的父节点。树的根节点是编号为 0 的节点。

树节点的第 k 个祖先节点是从该节点到根节点路径上的第 k 个节点。

实现 TreeAncestor 类：

TreeAncestor（int n， int[] parent） 对树和父数组中的节点数初始化对象。
getKthAncestor(int node, int k) 返回节点 node 的第 k 个祖先节点。如果不存在这样的祖先节点，返回 -1 。

示例 1：

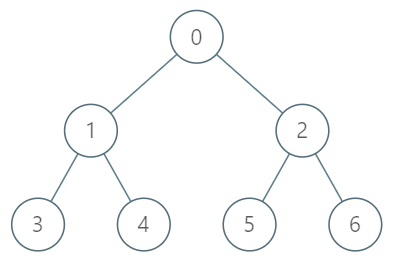
输入：
["TreeAncestor","getKthAncestor","getKthAncestor","getKthAncestor"]
[[7,[-1,0,0,1,1,2,2]],[3,1],[5,2],[6,3]]输出：
[null,1,0,-1]解释：
TreeAncestor treeAncestor = new TreeAncestor(7, [-1, 0, 0, 1, 1, 2, 2]);treeAncestor.getKthAncestor(3, 1);  // 返回 1 ，它是 3 的父节点
treeAncestor.getKthAncestor(5, 2);  // 返回 0 ，它是 5 的祖父节点
treeAncestor.getKthAncestor(6, 3);  // 返回 -1 因为不存在满足要求的祖先节点

提示：

1 <= k <= n <= 5 * 104
parent[0] == -1 表示编号为 0 的节点是根节点。
对于所有的 0 < i < n ，0 <= parent[i] < n 总成立
0 <= node < n
至多查询 5 * 104 次

实现代码与解析：

倍增

class TreeAncestor {int M = 17;int[][] an;public TreeAncestor(int n, int[] parent) {an = new int[n][M];// 初始化for (int i = 0; i < n; i++) {Arrays.fill(an[i], -1);}for (int i = 0; i < n; i++) {an[i][0] = parent[i];}for (int j = 1; j < M; j++) {for (int i = 0; i < n; i++) {if (an[i][j - 1] != -1) {an[i][j] = an[an[i][j - 1]][j - 1];}}}}public int getKthAncestor(int node, int k) {for (int j = 0; j < M; j++) {if (((k >> j) & 1) != 0) {node = an[node][j];if (node == -1) return -1;}}return node;}
}