算法体系-12 第十二二叉树的基本算法下

一实现二叉树的按层遍历

1.1 描述

1）其实就是宽度优先遍历，用队列

2）可以通过设置flag变量的方式，来发现某一层的结束（看题目）看下边的第四题解答

1.2 代码

public class Code01_LevelTraversalBT {public static class Node {public int value;public Node left;public Node right;public Node(int v) {value = v;}}public static void level(Node head) {if (head == null) {return;}Queue<Node> queue = new LinkedList<>();queue.add(head);while (!queue.isEmpty()) {Node cur = queue.poll();System.out.println(cur.value);if (cur.left != null) {queue.add(cur.left);}if (cur.right != null) {queue.add(cur.right);}}}

二实现二叉树的序列化和反序列化

2.1描述

1）先序方式序列化和反序列化

2）按层方式序列化和反序列化

将二叉树序力化为唯一的字符串叫序力化，字符串也能转出唯一的数二叉树叫反序力化

2.2 分析

2.3 前序列化代码

public static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static Queue<String> preSerial(Node head) {Queue<String> ans = new LinkedList<>();pres(head, ans);return ans;}public static void pres(Node head, Queue<String> ans) {if (head == null) {ans.add(null);} else {ans.add(String.valueOf(head.value));pres(head.left, ans);pres(head.right, ans);}}

2.4 前序反序列化

public static Node buildByPreQueue(Queue<String> prelist) {if (prelist == null || prelist.size() == 0) {return null;}return preb(prelist);}public static Node preb(Queue<String> prelist) {String value = prelist.poll();if (value == null) {return null;}Node head = new Node(Integer.valueOf(value));head.left = preb(prelist);head.right = preb(prelist);return head;}

2.5 中序列化代码

由上图可以知道，中序序例化是有歧义的，所以不存在中序的序列化

public static Queue<String> inSerial(Node head) {Queue<String> ans = new LinkedList<>();ins(head, ans);return ans;}public static void ins(Node head, Queue<String> ans) {if (head == null) {ans.add(null);} else {ins(head.left, ans);ans.add(String.valueOf(head.value));ins(head.right, ans);}}

2.7 中序反列化

2.8 后序列化代码

public static Queue<String> posSerial(Node head) {Queue<String> ans = new LinkedList<>();poss(head, ans);return ans;}public static void poss(Node head, Queue<String> ans) {if (head == null) {ans.add(null);} else {poss(head.left, ans);poss(head.right, ans);ans.add(String.valueOf(head.value));}}

2.9后序反列化代码

public static Node buildByPosQueue(Queue<String> poslist) {if (poslist == null || poslist.size() == 0) {return null;}// 左右中  ->  stack(中右左) 默认是左右中，这种情况没法首先没法建立头节点，因此进行转化为头在前面的情况，把它放入stack(中右左)，这是头就先出来，就可以新建headStack<String> stack = new Stack<>();while (!poslist.isEmpty()) {stack.push(poslist.poll());}return posb(stack);}public static Node posb(Stack<String> posstack) {String value = posstack.pop();if (value == null) {return null;}Node head = new Node(Integer.valueOf(value));head.right = posb(posstack);head.left = posb(posstack);return head;}

3.0 按层序列化和反序列化

3.0.1分析

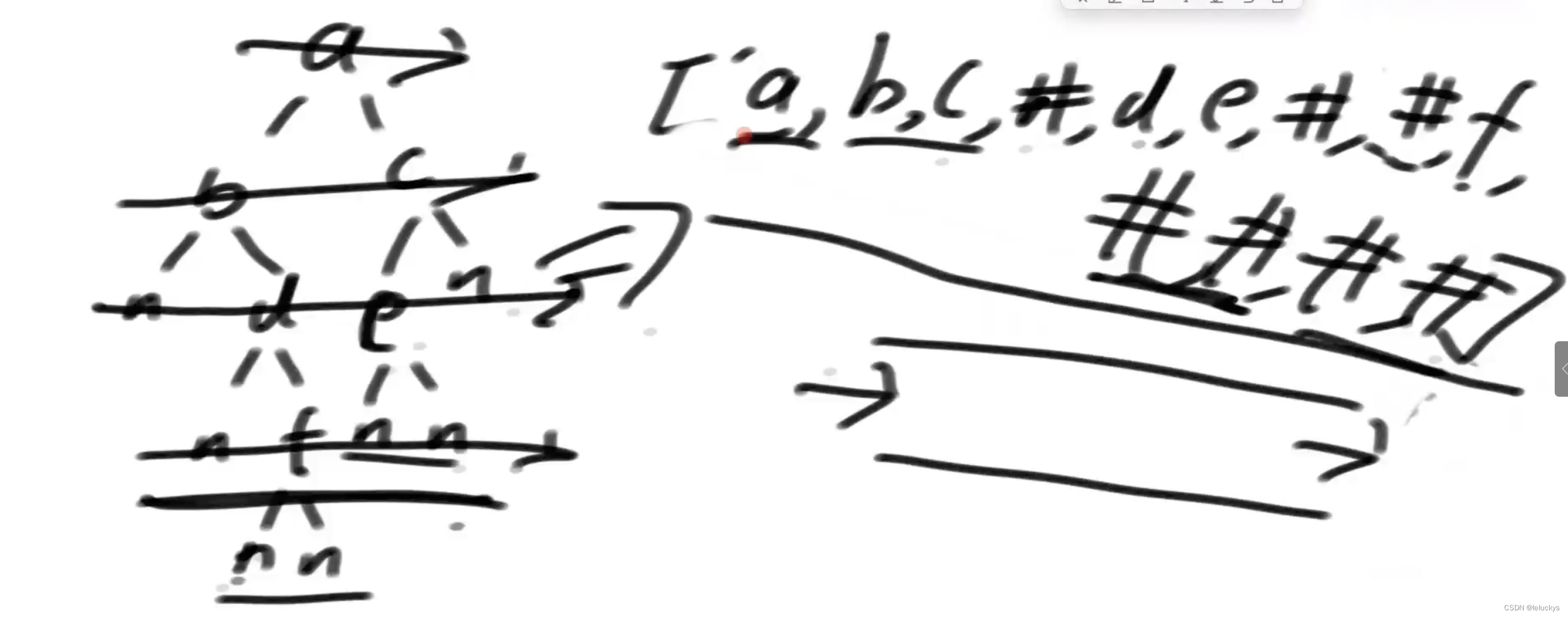3.0.2 按层序列化代码

public static Queue<String> levelSerial(Node head) {Queue<String> ans = new LinkedList<>();if (head == null) {ans.add(null);} else {ans.add(String.valueOf(head.value));Queue<Node> queue = new LinkedList<Node>();queue.add(head);while (!queue.isEmpty()) {head = queue.poll(); // head 父   子if (head.left != null) {ans.add(String.valueOf(head.left.value));queue.add(head.left);} else {ans.add(null);}if (head.right != null) {ans.add(String.valueOf(head.right.value));queue.add(head.right);} else {ans.add(null);}}}return ans;}

3.0.3 按层反序列化代码

public static Node buildByLevelQueue(Queue<String> levelList) {if (levelList == null || levelList.size() == 0) {return null;}Node head = generateNode(levelList.poll());Queue<Node> queue = new LinkedList<Node>();if (head != null) {queue.add(head);}//因为要记录上一次的节点，这里借用队列来完成Node node = null;while (!queue.isEmpty()) {node = queue.poll();node.left = generateNode(levelList.poll());node.right = generateNode(levelList.poll());if (node.left != null) {queue.add(node.left);}if (node.right != null) {queue.add(node.right);}}return head;}public static Node generateNode(String val) {if (val == null) {return null;}return new Node(Integer.valueOf(val));}

三 Encode N-ary Tree to Binary Tree

3.1 描述

一颗多叉树，序历化为为二叉树，二叉树也能转为原来的多叉树；

3.2 分析

第一步先将多叉树的每个节点的孩子放在对应节点左树的右边界上；

左树的节点为该节点的第一个树，反回来看某个节点是否有孩子看该节点左数是否有右边孩子

结构如下

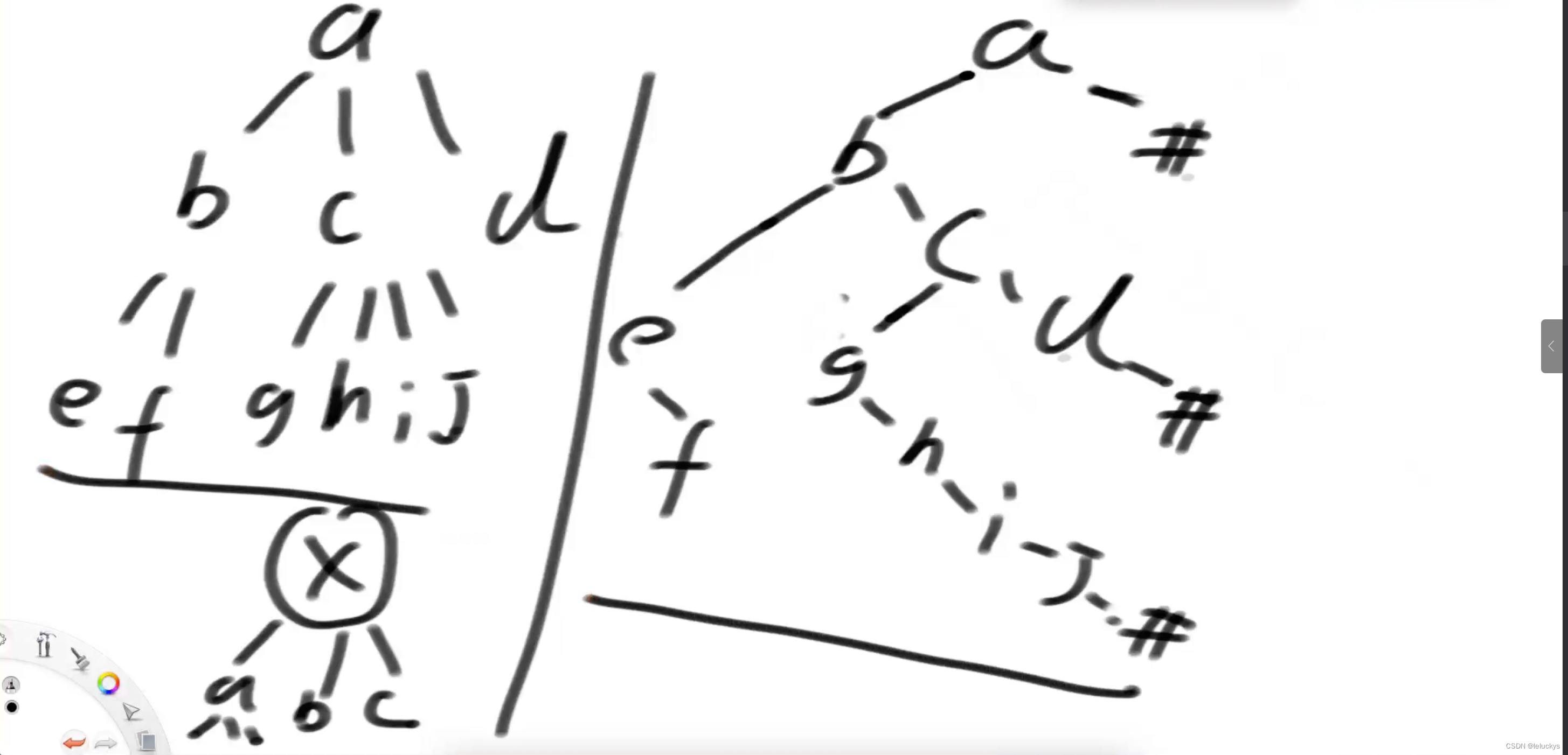
3.3 代码

package class11;import java.util.ArrayList;
import java.util.List;// 本题测试链接：https://leetcode.com/problems/encode-n-ary-tree-to-binary-tree
public class Code03_EncodeNaryTreeToBinaryTree {// 提交时不要提交这个类public static class Node {public int val;public List<Node> children;public Node() {}public Node(int _val) {val = _val;}public Node(int _val, List<Node> _children) {val = _val;children = _children;}};// 提交时不要提交这个类public static class TreeNode {int val;TreeNode left;TreeNode right;TreeNode(int x) {val = x;}}// 只提交这个类即可class Codec {// Encodes an n-ary tree to a binary tree.public TreeNode encode(Node root) {if (root == null) {return null;}TreeNode head = new TreeNode(root.val);head.left = en(root.children);return head;}private TreeNode en(List<Node> children) {TreeNode head = null;TreeNode cur = null;for (Node child : children) {TreeNode tNode = new TreeNode(child.val);if (head == null) {head = tNode;} else {cur.right = tNode;}cur = tNode;cur.left = en(child.children);}return head;}// Decodes your binary tree to an n-ary tree.public Node decode(TreeNode root) {if (root == null) {return null;}return new Node(root.val, de(root.left));}public List<Node> de(TreeNode root) {List<Node> children = new ArrayList<>();while (root != null) {Node cur = new Node(root.val, de(root.left));children.add(cur);root = root.right;}return children;}}}

四求二叉树最宽的层有多少个节点

4.1 描述

打印二叉树每层的的节点树及最多的节点数；

4.2 分析

根据宽度优先遍历的基础上，要是能知道哪一层结束，那么就能算出每一层的节点数；

设计两个数，Node curEnd = head; // 当前层，最右节点是谁Node nextEnd = null; // 下一层，最右节点是谁

每次遍历当前节点时候，判断该节点是否和记录的curEnd节点相等，相等就是当前层结束了，把当前层的节点数更新到max中，

再将当前节点的每一个左右孩子更新到队列中的过程中，每一步都更新nextEnd的值为当前加队列的值，下一层遍历来的时候更新curEnd值为nextEnd

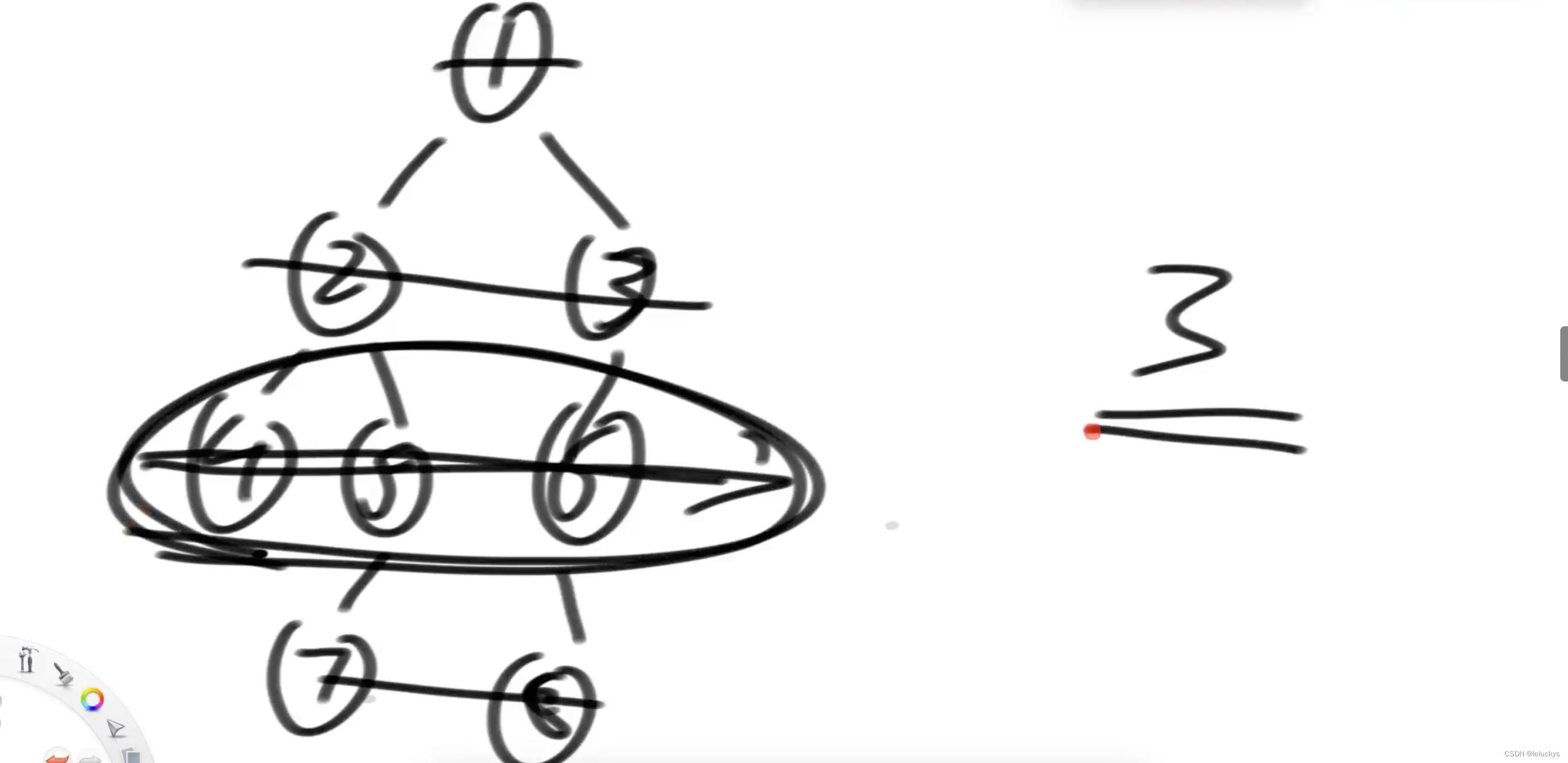
4.3 代码

public static int maxWidthNoMap(Node head) {if (head == null) {return 0;}Queue<Node> queue = new LinkedList<>();queue.add(head);Node curEnd = head; // 当前层，最右节点是谁Node nextEnd = null; // 下一层的最右节点是谁。提前为下一层出来的end节点做准备int max = 0;int curLevelNodes = 0; // 当前层的节点数while (!queue.isEmpty()) {Node cur = queue.poll();if (cur.left != null) {queue.add(cur.left);nextEnd = cur.left;}if (cur.right != null) {queue.add(cur.right);nextEnd = cur.right;}curLevelNodes++;if (cur == curEnd) {max = Math.max(max, curLevelNodes);curLevelNodes = 0;curEnd = nextEnd;}}return max;}//使用额外HashMapdpublic static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static int maxWidthUseMap(Node head) {if (head == null) {return 0;}Queue<Node> queue = new LinkedList<>();queue.add(head);// key 在 哪一层，valueHashMap<Node, Integer> levelMap = new HashMap<>();levelMap.put(head, 1);int curLevel = 1; // 当前你正在统计哪一层的宽度int curLevelNodes = 0; // 当前层curLevel层，宽度目前是多少int max = 0;while (!queue.isEmpty()) {Node cur = queue.poll();int curNodeLevel = levelMap.get(cur);if (cur.left != null) {levelMap.put(cur.left, curNodeLevel + 1);queue.add(cur.left);}if (cur.right != null) {levelMap.put(cur.right, curNodeLevel + 1);queue.add(cur.right);}if (curNodeLevel == curLevel) {curLevelNodes++;} else {max = Math.max(max, curLevelNodes);curLevel++;curLevelNodes = 1;}}max = Math.max(max, curLevelNodes);return max;}

五二叉树中的某个节点，返回该节点的后继节点

5.1 描述

后继节点：比如中序遍历，求该节点的4的后继节点，就是中序遍历遍历到该节点后的所有节点

二叉树结构如下定义：

Class Node {

V value;

Node left;

Node right;

Node parent;

}

给你二叉树中的某个节点，返回该节点的后继节点

5.2 分析中序遍历

5.2.1 方案一先通过parrent 找到的他的跟节点后，然后通root找到他的中序遍历，然后就可以找到该节点的后继节点

方案二

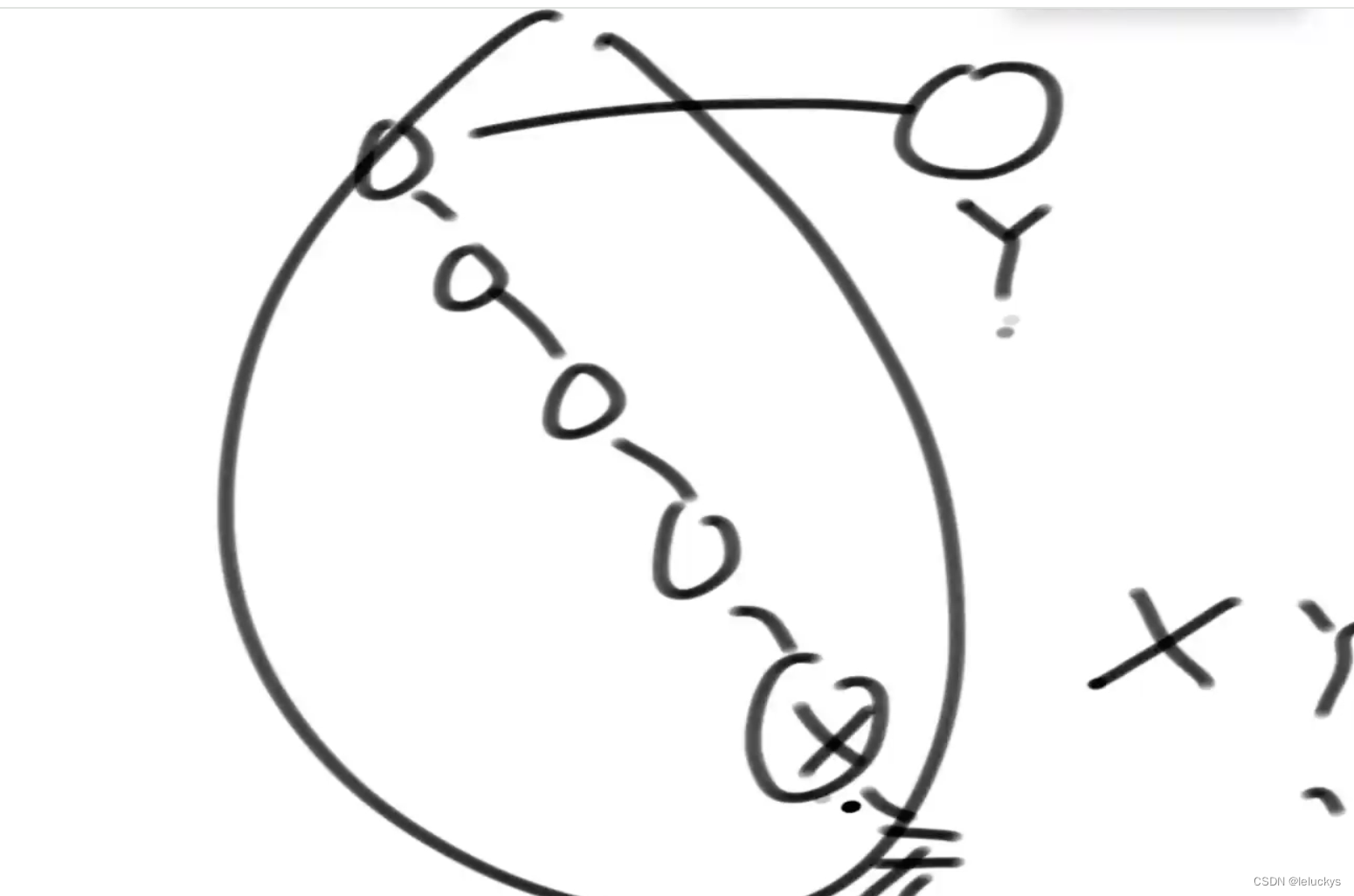
5.2.2 情况1一如果该节点有右数，那么他的后继节点一定是他右树的最左侧节点

情况二当该节点没有右树的时候，去找该节点是谁的节点左树的最右侧节点（中序遍历的本质理解）如果没有右子树，根据中序遍历的特点，下一个就应该是去找该节点是谁的节点左树的最右侧节点

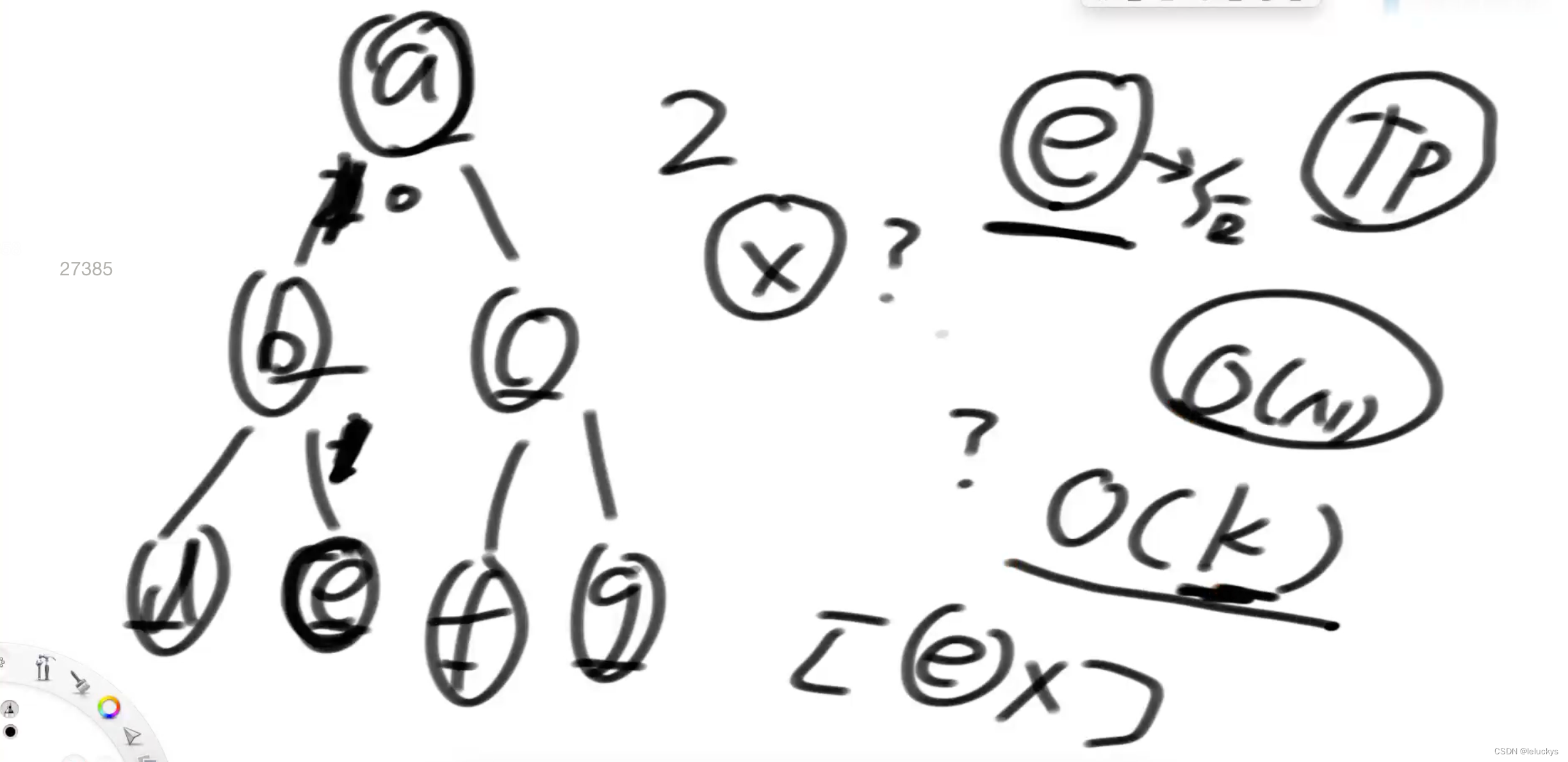
情况二讨论如下找一个数的后继节点，一直往上找，通过找到该节点的最后一个父节点，该节点的右子树就是他的后继节点

如下，x是y左数的最右节点，所以打印完x就该打印y了 == 找的就是那个左树上的最右节点

5.3 代码

public class Code06_SuccessorNode {public static class Node {public int value;public Node left;public Node right;public Node parent;public Node(int data) {this.value = data;}}public static Node getSuccessorNode(Node node) {if (node == null) {return node;}if (node.right != null) {return getLeftMost(node.right);} else { // 无右子树Node parent = node.parent;while (parent != null && parent.right == node) { // 当前节点是其父亲节点右孩子node = parent;parent = node.parent;}return parent;}}public static Node getLeftMost(Node node) {if (node == null) {return node;}while (node.left != null) {node = node.left;}return node;}