关于滑窗

滑窗是通过两个指针（数组下标）维护的。

3. 无重复字符的最长子串

给定一个字符串 s ，请你找出其中不含有重复字符的 最长子串 的长度。
请注意，你的答案必须是 子串 的长度，子序列不是子串。

思路：滑窗范围[pl, pr)，用cnt数组维护滑窗内的每个字符的出现次数（实际上，不是1就是0）,对于即将进入滑窗中的字符s[pr]，如果滑窗中已经出现过了，那么pl右移缩小滑窗范围，使得s[pr]加入滑窗之后，s[pr]的出现次数仍为1，不会超出即可。

class Solution {
public:int lengthOfLongestSubstring(string s) {int cnt[130] = {0};int pl = 0, pr = 0, maxlen = 0, n = s.length();while (pr < n) {if (cnt[s[pr]] == 0) {cnt[s[pr]]++;pr++;} else {do {cnt[s[pl]]--;pl++;} while (s[pr] != s[pl - 1]);}if (maxlen < pr - pl)maxlen = pr - pl;}return maxlen;}
};

438. 找到字符串中所有字母异位词

给定两个字符串 s 和 p，找到 s 中所有 p 的 异位词 的子串，返回这些子串的起始索引。不考虑答案输出的顺序。
异位词 指由相同字母重排列形成的字符串（包括相同的字符串）。

思路：滑窗范围是[i, j)，对于每个即将加入滑窗的字符s[j]，看一下滑窗中该字符的个数（用cnts数组来维护），如果已经满了，那么滑窗左侧就要往右移动（缩小范围）了。

class Solution {int cntp[130], cnts[130];vector<int> ans;public:vector<int> findAnagrams(string s, string p) {int i = 0, j = 0, ns = s.length(), np = p.length();// 计算出p的每个字符的个数for (auto ch : p)cntp[ch]++;while (j <= ns) {if (cnts[s[j]] == cntp[s[j]]) // 满了{if (j - i == np)ans.push_back(i);while (s[i] != s[j]) {cnts[s[i]]--;i++;}cnts[s[i]]--;i++;}cnts[s[j]]++;j++;}return ans;}
};

567. 字符串的排列

这道题和438一模一样。

class Solution {int cnt2[130] = {0}, cnt1[130] = {0};
public:bool checkInclusion(string s2, string s1) {s1 += " ";for (auto ch : s2)cnt2[ch]++;int pl = 0, pr = 0, n1 = s1.length(), n2 = s2.length();while (pr <= n1){cnt1[s1[pr]]++;if (cnt1[s1[pr]] > cnt2[s1[pr]]){if (pr - pl == n2) return true;// pl右移while (cnt1[s1[pr]] > cnt2[s1[pr]]){cnt1[s1[pl]]--;pl++;}}pr++;}return false;}
};

76. 最小覆盖子串

76题跟前面几道题有不同。

class Solution {int cnts[130] = {0}, cntt[130] = {0};int pl = 0, pr = 0;bool hasFindAns = false;bool check(){for (char c = 'A'; c <= 'z'; c++)if (cnts[c] < cntt[c]) return false;return true;}public:string minWindow(string s, string t) {int ns = s.length(), nt = t.length();int lo = 0, hi = 0; //答案for (auto ch : t)cntt[ch]++;//滑窗范围左开右闭while (pr < ns){cnts[s[pr]]++;pr++;while (pl < pr && cnts[s[pl]] > cntt[s[pl]]){cnts[s[pl]]--;pl++;}if (check()){if (!hasFindAns || pr - pl <= hi - lo){lo = pl;hi = pr;hasFindAns = true;}}  }return s.substr(lo, hi-lo);}
};

239. 滑动窗口最大值

虽然题名叫“滑动窗口”，但解法不是上面的滑动窗口。

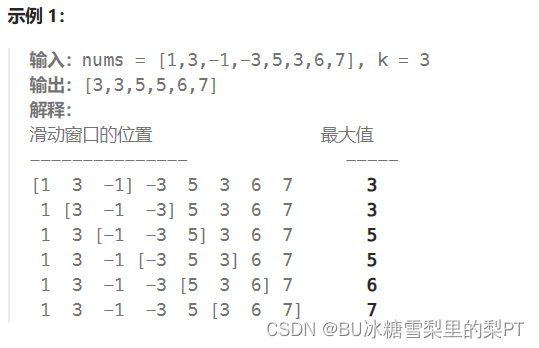
方法一：优先队列+延迟删除 O(nlogn)

struct p{int n;int i;bool operator < (const p & a) const{return n < a.n;}
};class Solution {priority_queue<p> pq;vector<int> ans;
public:vector<int> maxSlidingWindow(vector<int>& nums, int k) {if (nums.size()< k) return ans;for (int i=0; i<k-1; i++)pq.push(p{nums[i], i});for (int i=k-1 ;i<nums.size(); i++){pq.push(p{nums[i], i});while (pq.top().i <= i - k)pq.pop();ans.push_back(pq.top().n);}return ans;}
};

方法二：单调队列 O(n)

因为STL的queue不能从末尾删除元素，所以自己写一个queue

struct p {int n, i;
};template <typename T> struct myQueue {T q[100010];int head = 0, tail = 0;bool empty() { return head == tail; }T back() { return q[tail - 1]; }void pop_back() { tail--; }void pop() { head++; }T front() { return q[head]; }void push(T elem) { q[tail++] = elem; }
};
class Solution {myQueue<p> q;vector<int> ans;public:vector<int> maxSlidingWindow(vector<int>& nums, int k) {if (nums.size() < k)return ans;for (int i = 0; i < nums.size(); i++) {while (!q.empty() && q.back().n <= nums[i])q.pop_back();q.push(p{nums[i], i});if (q.front().i <= i - k)q.pop();if (i > k - 2)ans.push_back(q.front().n);}return ans;}
};