二叉树基础

二叉树种类

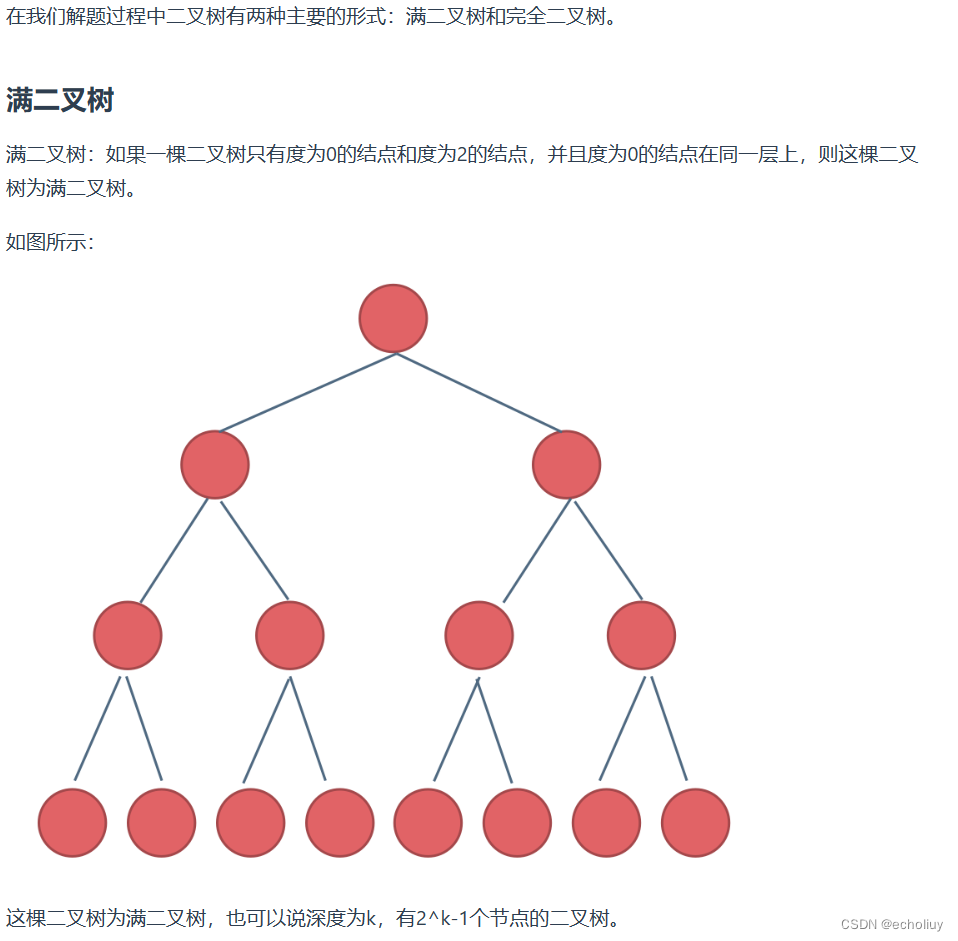
满二叉树

完全二叉树

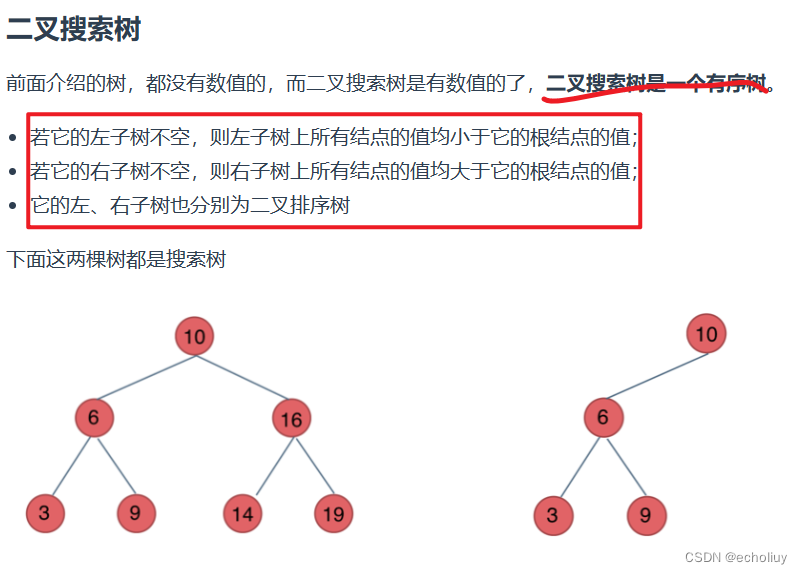
二叉搜索树

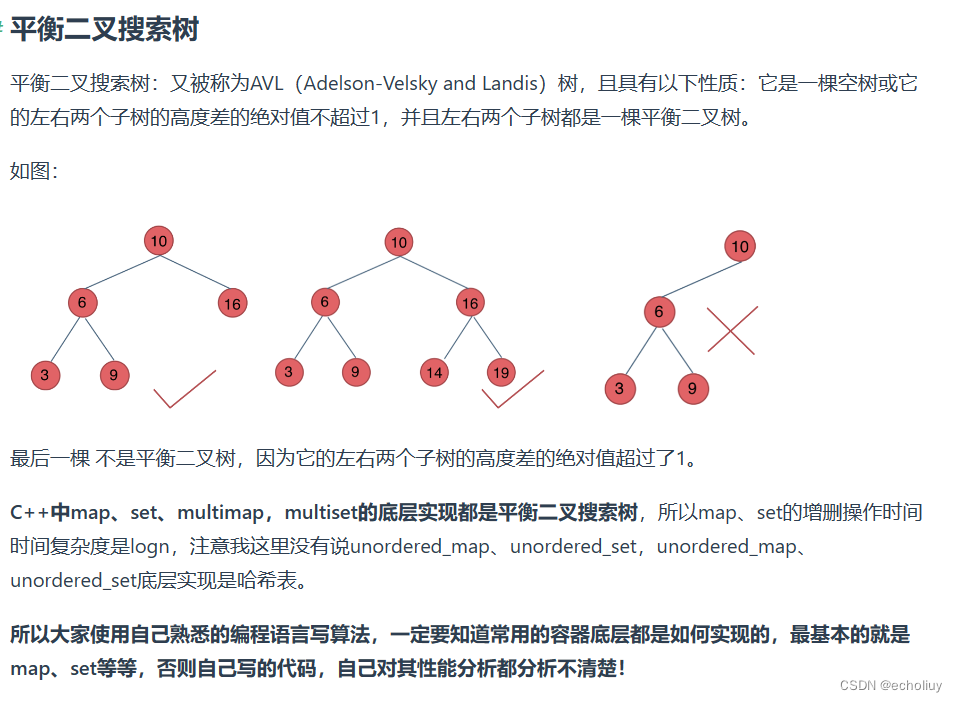
平衡二叉搜索树

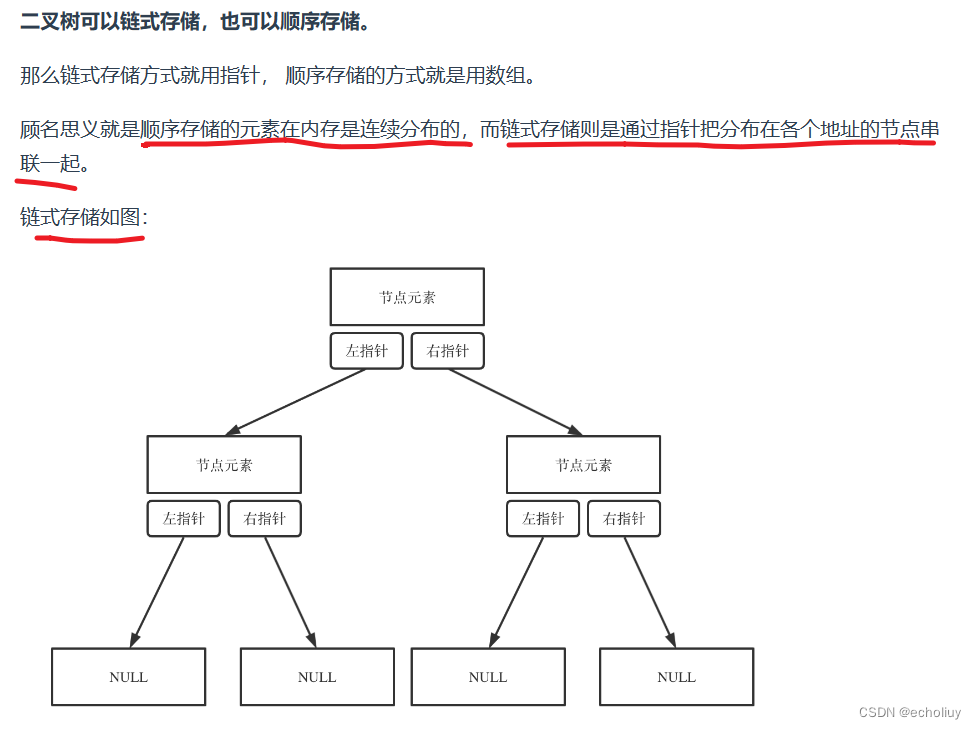
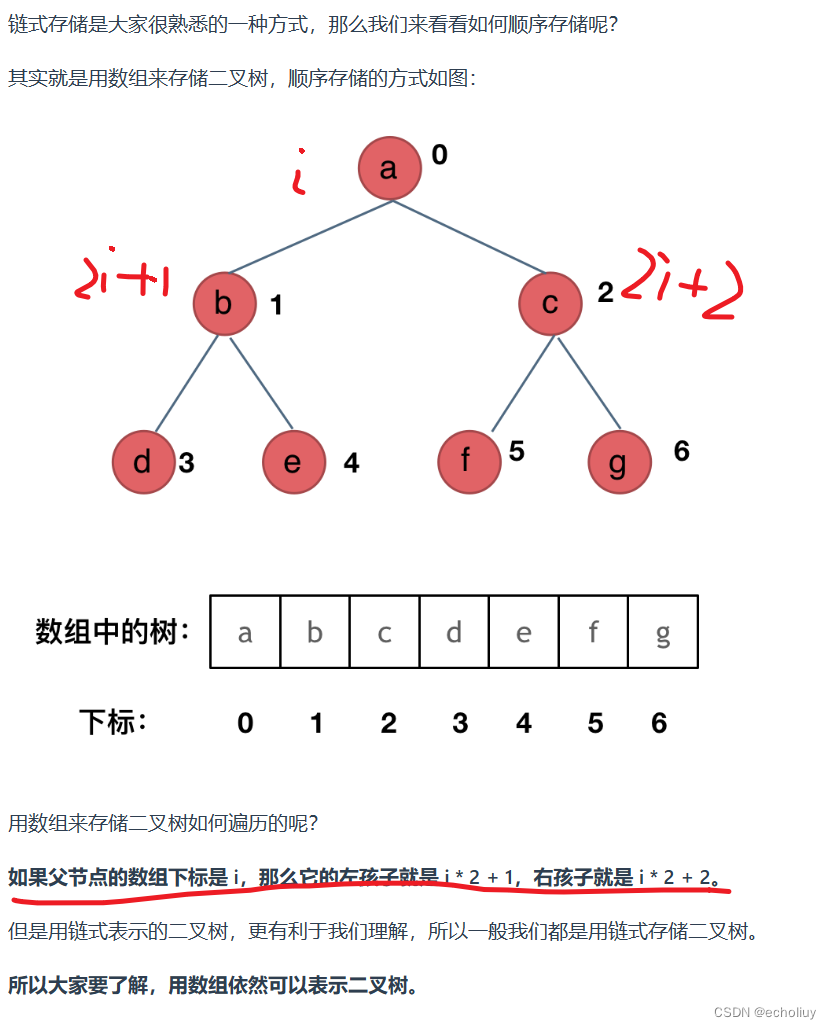
二叉树的存储方式

链式存储

顺序存储

二叉树的遍历方式

二叉树主要有两种遍历方式：

1. 深度优先遍历：先往深走，遇到叶子节点再往回走。
2. 广度优先遍历：一层一层的去遍历。

这两种遍历是图论中最基本的两种遍历方式，后面在介绍图论的时候 还会介绍到。

那么从深度优先遍历和广度优先遍历进一步拓展，才有如下遍历方式：

1. 深度优先遍历
   • 前序遍历（递归法，迭代法）
   • 中序遍历（递归法，迭代法）
   • 后序遍历（递归法，迭代法）
2. 广度优先遍历
   • 层次遍历（迭代法）
     在深度优先遍历中：有三个顺序，前中后序遍历， 有同学总分不清这三个顺序，经常搞混，我这里教大家一个技巧。

这里前中后，其实指的就是中间节点的遍历顺序，只要大家记住 前中后序指的就是中间节点的位置就可以了。

看如下中间节点的顺序，就可以发现，中间节点的顺序就是所谓的遍历方式

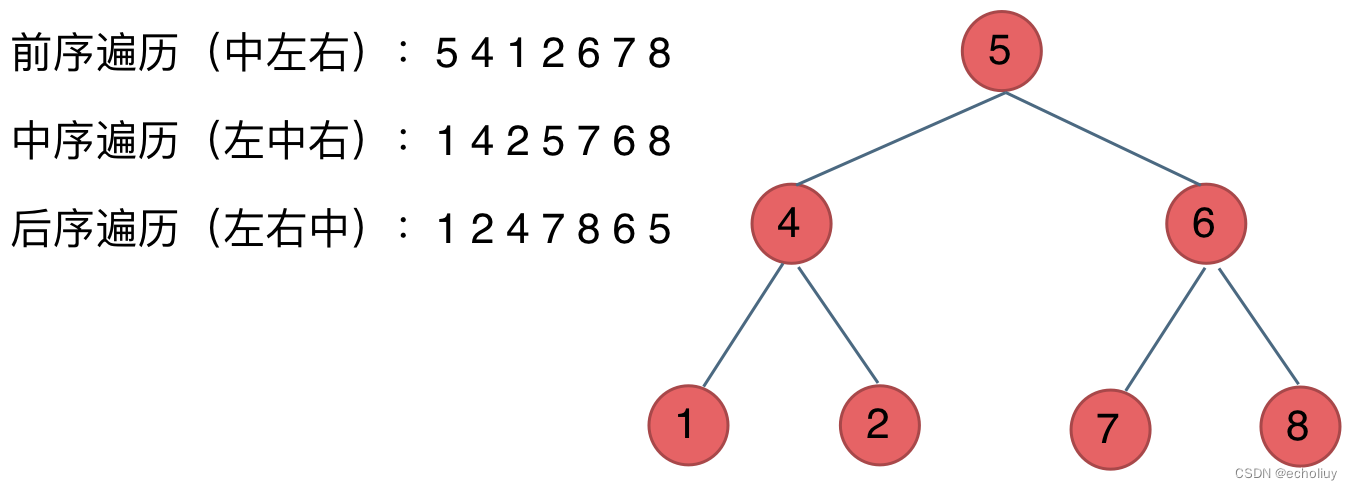
• 前序遍历：中左右
• 中序遍历：左中右
• 后序遍历：左右中
  

二叉树的定义

public class TreeNode {int val;TreeNode left;TreeNode right;TreeNode() {}TreeNode(int val) { this.val = val; }TreeNode(int val, TreeNode left, TreeNode right) {this.val = val;this.left = left;this.right = right;}
}

二叉树的递归遍历

144.二叉树的前序遍历

前序遍历，中左右

代码：

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Integer> preorderTraversal(TreeNode root) {List<Integer> result = new ArrayList<>();//结果数组preorder(root,result);//开始从根节点遍历return result;}public void preorder(TreeNode root,List<Integer> res){if(root==null){return; //直接返回}res.add(root.val);//添加根节点preorder(root.left,res);preorder(root.right,res);}
}

145.二叉树的后序遍历

左右中

代码：

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Integer> postorderTraversal(TreeNode root) {List<Integer> res = new ArrayList<>();postOrder(root,res);return res;}public void postOrder(TreeNode root,List<Integer> res){if(root==null){return;}postOrder(root.left,res);postOrder(root.right,res);res.add(root.val);}
}

94. 二叉树的中序遍历

左中右

代码

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Integer> inorderTraversal(TreeNode root) {List<Integer> res = new ArrayList<>();inOrder(root,res);return res;}public void inOrder(TreeNode root,List<Integer> res){if(root==null){return;}inOrder(root.left,res);res.add(root.val);inOrder(root.right,res);}
}

二叉树的迭代遍历

前序遍历（迭代法）-中左右->中右左（模拟出入栈）

我们先看一下前序遍历。

前序遍历是中左右，每次先处理的是中间节点，
那么先将根节点放入栈中，
然后先将右孩子加入栈，再加入左孩子。

为什么要先加入 右孩子，再加入左孩子呢？ 因为这样出栈的时候才是中左右的顺序。

动画如下：

代码

// 前序遍历顺序：中-左-右，入栈顺序：中-右-左
class Solution {public List<Integer> preorderTraversal(TreeNode root) {List<Integer> res=new ArrayList<>();if(root==null){return res;}Stack<TreeNode> stack=new Stack<>();stack.push(root);//加入根节点while(!stack.isEmpty()){//中TreeNode node=stack.pop();//弹出根节点res.add(node.val);//储存根节点的数值//右if(node.right!=null){stack.push(node.right);}//左if(node.left!=null){stack.push(node.left);}}return res;}
}

后序遍历（迭代法）

思路：

前序遍历的数组储存是中左右，那么把函数翻转，则变成数组储存是中右左，再将数组进行翻转，则变成左右中。

代码：

// 后序遍历顺序 左-右-中 入栈顺序：中-左-右 出栈顺序：中-右-左， 最后翻转结果
class Solution {public List<Integer> postorderTraversal(TreeNode root) {List<Integer> res = new ArrayList<>();Stack<TreeNode> stack=new Stack<>();if(root==null){return res;}stack.push(root);while(!stack.isEmpty()){TreeNode node = stack.pop();res.add(node.val);if(node.left!=null){stack.push(node.left);}if(node.right!=null){stack.push(node.right);}}Collections.reverse(res);//反转数组return res;}
}

中序遍历（迭代法）

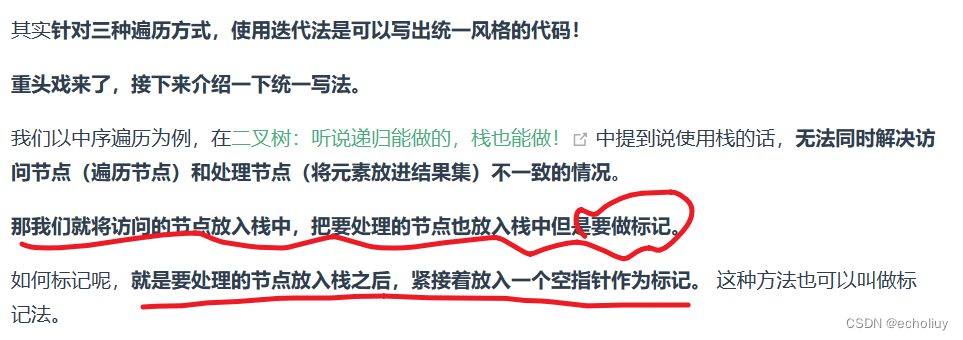
为了解释清楚，我说明一下 刚刚在迭代的过程中，其实我们有两个操作：

1. 处理：将元素放进result数组中
2. 访问：遍历节点
   分析一下为什么刚刚写的前序遍历的代码，不能和中序遍历通用呢，因为前序遍历的顺序是中左右，先访问的元素是中间节点，要处理的元素也是中间节点，所以刚刚才能写出相对简洁的代码，因为要访问的元素和要处理的元素顺序是一致的，都是中间节点。

那么再看看中序遍历，中序遍历是左中右，先访问的是二叉树顶部的节点，然后一层一层向下访问，直到到达树左面的最底部，再开始处理节点（也就是在把节点的数值放进result数组中），这就造成了处理顺序和访问顺序是不一致的。

那么在使用迭代法写中序遍历，就需要借用指针的遍历来帮助访问节点，栈则用来处理节点上的元素。

动画如下：

代码：

// 中序遍历顺序: 左-中-右 入栈顺序： 左-右
class Solution {public List<Integer> inorderTraversal(TreeNode root) {List<Integer> result = new ArrayList<>();if (root == null){return result;}Stack<TreeNode> stack = new Stack<>();TreeNode cur = root;while (cur != null || !stack.isEmpty()){if (cur != null){stack.push(cur);cur = cur.left;}else{cur = stack.pop();result.add(cur.val);cur = cur.right;}}return result;}
}

二叉树的统一迭代法-画图理解增加熟练

前序遍历写法-右左中（反过来）

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Integer> preorderTraversal(TreeNode root) {List<Integer> res = new LinkedList<>();Stack<TreeNode> stack = new Stack<>();if(root!=null){stack.push(root);}while(!stack.isEmpty()){TreeNode node=stack.peek();//栈中的第一个节点if(node!=null){stack.pop();//弹出避免重复，下面再将右左中节点添加到栈中if(node.right!=null){//添加右节点，空节点不入栈stack.push(node.right); }if(node.left!=null){//添加左节点，空节点不入栈stack.push(node.left);}stack.push(node);//添加中节点stack.push(null);//中节点还没有处理，添加空节点null做标记}else{stack.pop();//弹出nullnode=stack.peek();//重新取出栈中元素stack.pop();//取出后再弹出res.add(node.val);}}return res;}
}

中序遍历写法-右中左

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Integer> inorderTraversal(TreeNode root) {List<Integer> res = new ArrayList<>();Stack<TreeNode> stack = new Stack<>();if(root!=null){//先放入根节点stack.push(root);}while(!stack.isEmpty()){TreeNode node = stack.peek();//查找第一个结点if(node!=null){stack.pop();//弹出重复节点，按顺序放入右中左if(node.right!=null){stack.push(node.right);}stack.push(node);stack.push(null);if(node.left!=null){stack.push(node.left);}}else{stack.pop();node = stack.peek();stack.pop();res.add(node.val);}}return res;}
}

后序遍历-中右左

class Solution {public List<Integer> postorderTraversal(TreeNode root) {List<Integer> res = new ArrayList<>();Stack<TreeNode> stack = new Stack<>();if(root!=null){//先放入根节点stack.push(root);}while(!stack.isEmpty()){TreeNode node = stack.peek();//查找第一个结点if(node!=null){stack.pop();//弹出重复节点，按顺序放入中右左stack.push(node);stack.push(null);if(node.right!=null){stack.push(node.right);}if(node.left!=null){stack.push(node.left);}}else{stack.pop();node = stack.peek();stack.pop();res.add(node.val);}}return res;}
}