1.预备知识

1.1 栈

S.empty() //1
S.push(10); //2
S.pop(); //3

1.2 队列

Q.push(5); //1
Q.pop(); //2
Q.push(1); //3

1.3 堆

图：堆
图：堆的函数

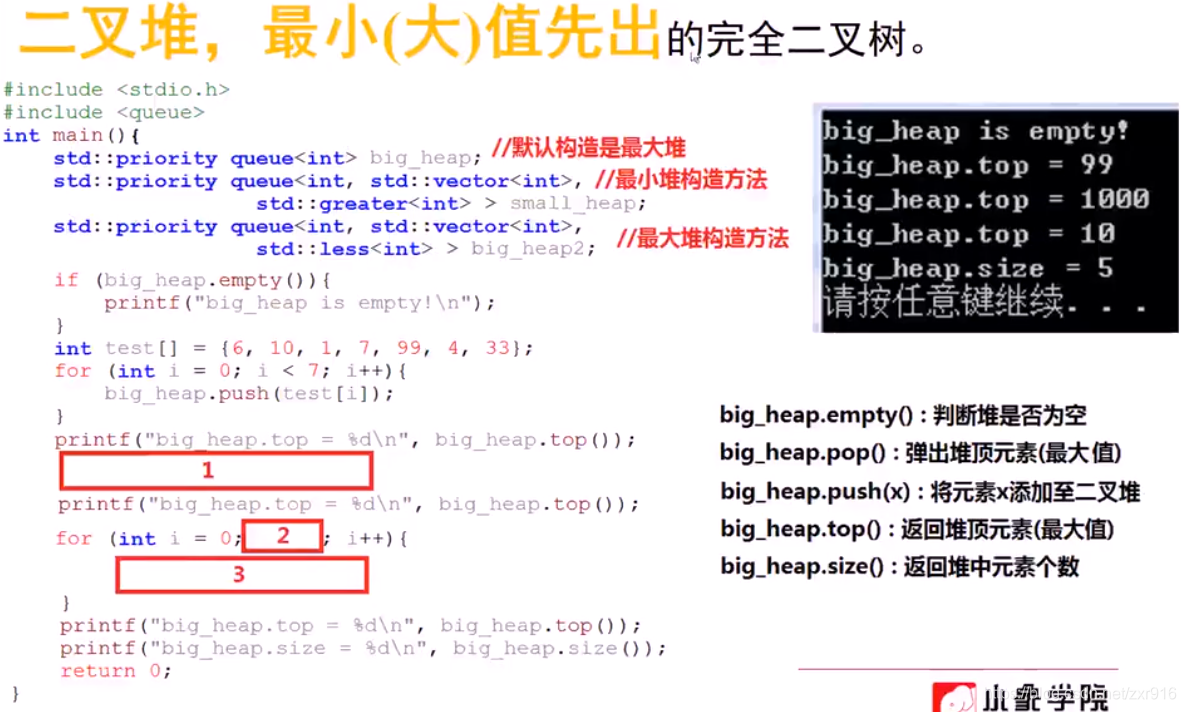
big_heap.push(1000); //1
i<3 // 2
big_heap.pop(); //3

2.用队列实现栈

2.1 题目描述

请你仅使用两个队列实现一个后入先出（LIFO）的栈，并支持普通队列的全部四种操作（push、top、pop 和 empty）

实现 MyStack 类：
void push(int x) 将元素 x 压入栈顶。
int pop() 移除并返回栈顶元素。
int top() 返回栈顶元素。
boolean empty() 如果栈是空的，返回 true ；否则，返回 false 。

你只能使用队列的基本操作 —— 也就是 push to back、peek/pop from front、size 和 is empty 这些操作。

你所使用的语言也许不支持队列。 你可以使用 list （列表）或者 deque（双端队列）来模拟一个队列 , 只要是标准的队列操作即可。

2.2 解题思路

2.3 C++实现

class MyStack {
public:/** Initialize your data structure here. */MyStack() {}/** Push element x onto stack. */void push(int x) {queue<int> temp_queue;temp_queue.push(x);while(!_data.empty()){temp_queue.push(_data.front());_data.pop();}while(!temp_queue.empty()){_data.push(temp_queue.front());temp_queue.pop();}}/** Removes the element on top of the stack and returns that element. */int pop() {int x=_data.front();_data.pop();return x;}/** Get the top element. */int top() {return _data.front();}/** Returns whether the stack is empty. */bool empty() {return _data.empty();}
private:queue<int> _data;
};

3.用栈实现队列

3.1 题目描述

请你仅使用两个栈实现先入先出队列。队列应当支持一般队列支持的所有操作（push、pop、peek、empty）：

实现 MyQueue 类：
void push(int x) 将元素 x 推到队列的末尾
int pop() 从队列的开头移除并返回元素
int peek() 返回队列开头的元素
boolean empty() 如果队列为空，返回 true ；否则，返回 false

说明：
你只能使用标准的栈操作 —— 也就是只有 push to top, peek/pop from top, size, 和 is empty 操作是合法的。
你所使用的语言也许不支持栈。你可以使用 list 或者 deque（双端队列）来模拟一个栈，只要是标准的栈操作即可。

3.2 解题思路

3.3 C++实现

class MyQueue {
public:/** Initialize your data structure here. */MyQueue() {}/** Push element x to the back of queue. */void push(int x) {stack<int> temp_stack;while(!_data.empty()){temp_stack.push(_data.top());_data.pop();}temp_stack.push(x);while(!temp_stack.empty()){_data.push(temp_stack.top());temp_stack.pop();}}/** Removes the element from in front of queue and returns that element. */int pop() {int x=_data.top();_data.pop();return x;}/** Get the front element. */int peek() {return _data.top();}/** Returns whether the queue is empty. */bool empty() {return _data.empty();}
private:stack<int> _data;
};

4.最小栈

4.1 题目描述

设计一个支持 push ，pop ，top 操作，并能在常数时间内检索到最小元素的栈。

push(x) —— 将元素 x 推入栈中。
pop() —— 删除栈顶的元素。
top() —— 获取栈顶元素。
getMin() —— 检索栈中的最小元素。

4.2 解题思路

class MinStack {
public:/** initialize your data structure here. */MinStack() {}void push(int val) {_data.push(val);if(_min.empty()){_min.push(val);}else{if(val>_min.top()){val=_min.top();}_min.push(val);}}void pop() {_data.pop();_min.pop();}int top() {return _data.top();}int getMin() {return _min.top();}
private:stack<int> _data;stack<int> _min;
};

5.合法的出栈序列

5.1 题目描述

5.2 解题思路

图1：模拟入栈出栈
图2：步骤1

图3：步骤2

图4：步骤3
图5：步骤4

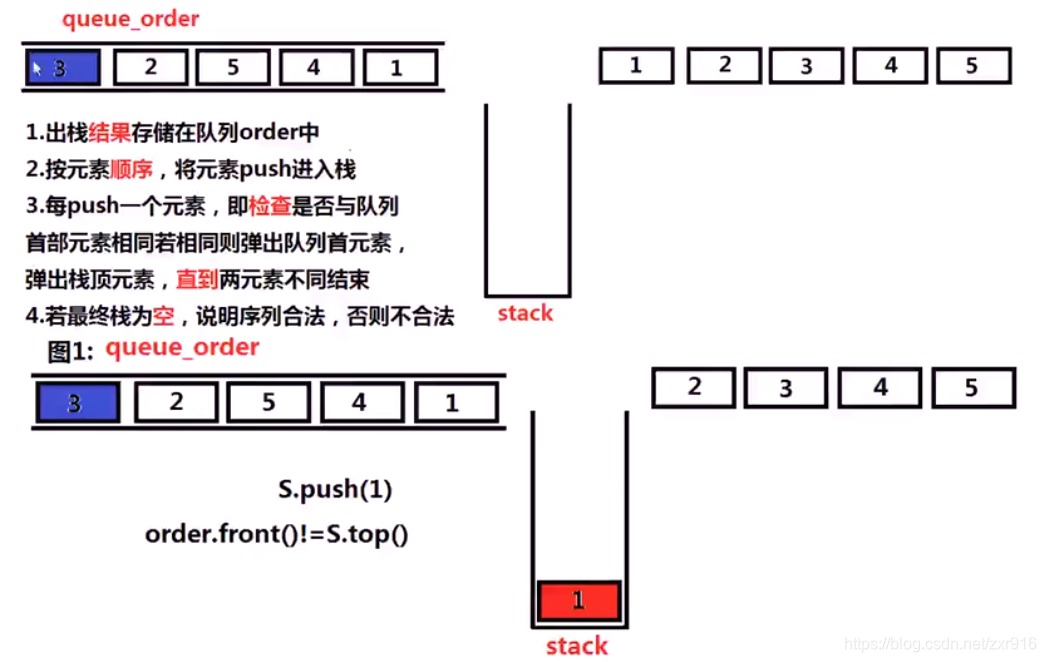
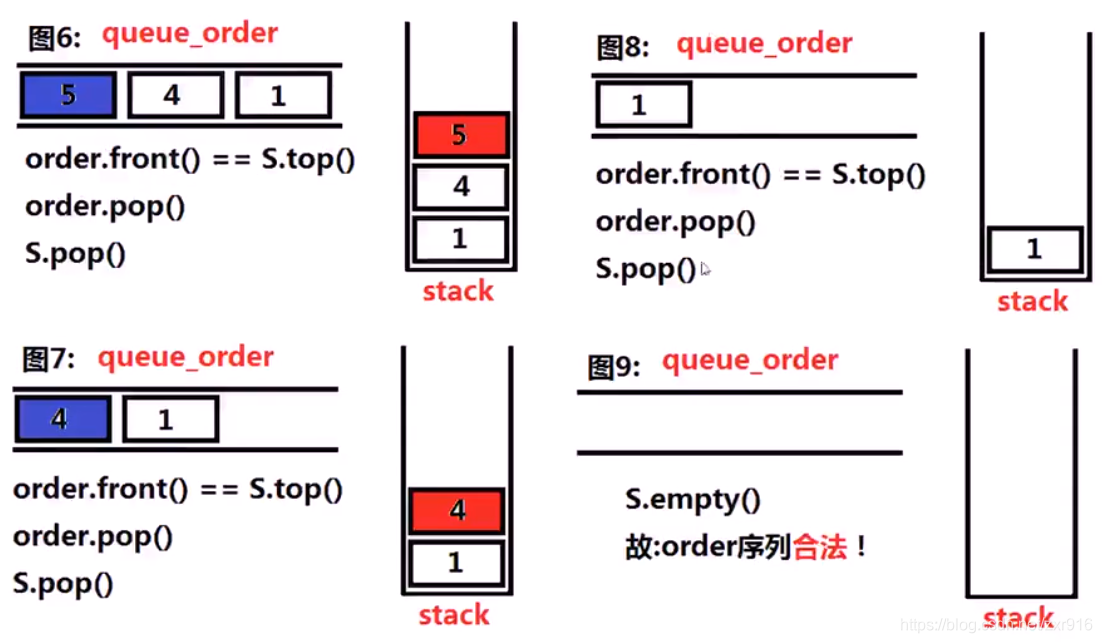
5.3 C++实现

#include <iostream>
#include<queue>
#include<stack>
using namespace std;bool check_is_valid_order(queue<int>& order)
{stack<int> S;int n = order.size();for (int i = 1; i <= n; i++) {S.push(i);while (!S.empty() && S.top() == order.front()) {S.pop();order.pop();}}if (!S.empty()) {return false;}return true;
}int main()
{//合法：32541 ;非法：31245queue<int> order1, order2;bool a1, a2;order1.push(3);order1.push(2);order1.push(5);order1.push(4);order1.push(1);a1 = check_is_valid_order(order1);if (a1 == true) {cout << "32541合法" << endl;}else {cout << "32541非法" << endl;}order2.push(3);order2.push(1);order2.push(2);order2.push(4);order2.push(5);a2 = check_is_valid_order(order2);if (a2 == true) {cout << "31245合法" << endl;}else {cout << "31245非法" << endl;}return 0;
}

6.基本计算器

6.1 题目描述

给你一个字符串表达式 s ，请你实现一个基本计算器来计算并返回它的值。

1 <= s.length <= 3 * 105
s 由数字、'+'、'-'、'('、')'、和 ' ' 组成
s 表示一个有效的表达式

6.2 解题思路

图1：栈内元素计算

图2：使用栈处理优先级

图3：将字符串转化为数字

图4：计算函数

图5：字符串处理思路

7.数组中的第K个最大元素

7.1 题目描述

在未排序的数组中找到第 k 个最大的元素。请注意，你需要找的是数组排序后的第 k 个最大的元素，而不是第 k 个不同的元素。

7.2 解题思路

图1：思路
图2：思路

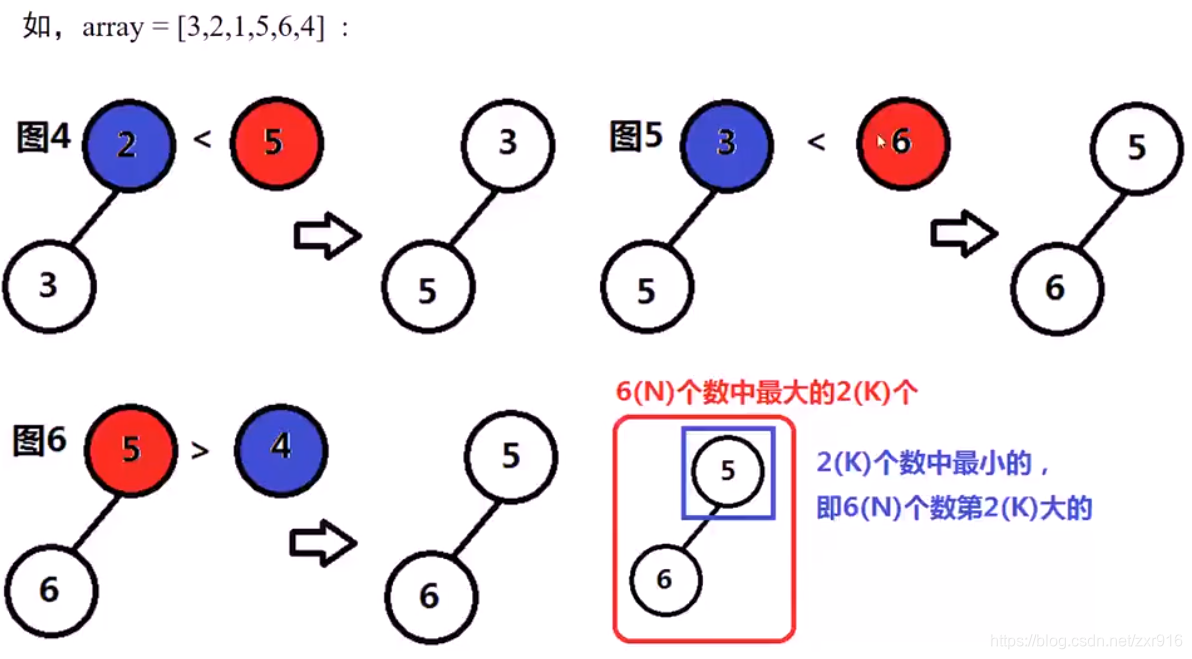
7.3 C++实现

class Solution {
public:int findKthLargest(vector<int>& nums, int k) {priority_queue<int,vector<int>,greater<int>> Q;for(int i=0;i<nums.size();i++){if(Q.size()<k){Q.push(nums[i]);}else if(Q.top()<nums[i]){Q.pop();Q.push(nums[i]);}}return Q.top();}
};

8.数据流的中位数

8.1 题目描述

中位数是有序列表中间的数。如果列表长度是偶数，中位数则是中间两个数的平均值。

例如，
[2,3,4] 的中位数是 3
[2,3] 的中位数是 (2 + 3) / 2 = 2.5

设计一个支持以下两种操作的数据结构：
void addNum(int num) - 从数据流中添加一个整数到数据结构中。
double findMedian() - 返回目前所有元素的中位数。

8.2 解题思路

8.3 C++实现

class MedianFinder {
public:/** initialize your data structure here. */MedianFinder() {}void addNum(int num) {if(big_queue.empty()){big_queue.push(num);return;}if(big_queue.size()==small_queue.size()){if(num<big_queue.top()){big_queue.push(num);}else{small_queue.push(num);}}else if(big_queue.size()>small_queue.size()){if(num>big_queue.top()){small_queue.push(num);}else{small_queue.push(big_queue.top());big_queue.pop();big_queue.push(num);}}else if(small_queue.size()>big_queue.size()){if(num<small_queue.top()){big_queue.push(num);}else{big_queue.push(small_queue.top());small_queue.pop();small_queue.push(num);}}}double findMedian() {if(small_queue.size()==big_queue.size()){return (small_queue.top()+big_queue.top())/2;}else if(small_queue.size()>big_queue.size()){return small_queue.top();}return big_queue.top();}
private:priority_queue<double,vector<double>,greater<double>> small_queue;priority_queue<double,vector<double>,less<double>> big_queue;
};