【问题描述】

给定一个二叉树, 找到该树中两个指定节点的最近公共祖先。百度百科中最近公共祖先的定义为：“对于有根树 T 的两个结点 p、q，最近公共祖先表示为一个结点 x，满足 x 是 p、q 的祖先且 x 的深度尽可能大（一个节点也可以是它自己的祖先）。”例如，给定如下二叉树:  root = [3,5,1,6,2,0,8,null,null,7,4]输入: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 1
输出: 3
解释: 节点 5 和节点 1 的最近公共祖先是节点 3。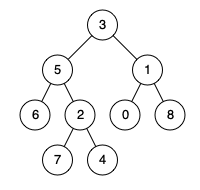

【解答思路】

1. DFS 后序遍历

时间复杂度：O(N^2) 空间复杂度：O(N)

class Solution {public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {if(root == null || root == p || root == q) return root;TreeNode left = lowestCommonAncestor(root.left, p, q);TreeNode right = lowestCommonAncestor(root.right, p, q);if(left == null && right == null) return null; // 1. 可省略if(left == null) return right; // 3.if(right == null) return left; // 4.return root; // 2. if(left != null and right != null)}
}

2. 路径法

public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {Stack<TreeNode> stack = new Stack<>();HashMap<TreeNode, TreeNode> parent = new HashMap<>();//初始化根节点stack.push(root);parent.put(root, null);//将遍历过程中每个节点的父节点保存起来while (!parent.containsKey(p) || !parent.containsKey(q)) {TreeNode cur = stack.pop();if (cur.left != null) {stack.push(cur.left);parent.put(cur.left, cur);}if (cur.right != null) {stack.push(cur.right);parent.put(cur.right, cur);}}HashSet<TreeNode> path = new HashSet<>();// 倒着还原 p 的路径，并将每个节点加入到 set 中while (p != null) {path.add(p);p = parent.get(p);}// 倒着遍历 q 的路径，判断是否在 p 的路径中while (q != null) {if (path.contains(q)) {break;}q = parent.get(q);}return q;
}

【总结】

1.二叉树遍历

• 前序遍历 先输出当前结点的数据，再依次遍历输出左结点和右结点
• 中序遍历 先遍历输出左结点，再输出当前结点的数据，再遍历输出右结点
• 后续遍历 先遍历输出左结点，再遍历输出右结点，最后输出当前结点的数据

2. 树的问题DFS BFS 递归思想 剪枝优化

3.堆栈+HashMap（记录父节点）+HashSet（记录路径）

转载链接:https://leetcode-cn.com/problems/lowest-common-ancestor-of-a-binary-tree/solution/236-er-cha-shu-de-zui-jin-gong-gong-zu-xian-hou-xu/
转载链接：https://leetcode-cn.com/problems/lowest-common-ancestor-of-a-binary-tree/solution/xiang-xi-tong-su-de-si-lu-fen-xi-duo-jie-fa-by-5-2/