对比分析是对两个互相联系的指标进行比较。
绝对数比较(相减):指标在量级上不能差别过大,常用折线图、柱状图
相对数比较(相除):结构分析、比例分析、空间比较分析、动态对比分析
df = pd.DataFrame(np.random.rand(30,2)*1000,columns=['A_sale','B_sale'],index = pd.date_range('2019/6/1','2019/6/30')) df.style.format({'A':'{:.2f}','B':'{:.2f}'})
# df.plot(figsize = (10,5),linestyle ='--',title ='A_sale to B_sale:line',marker = '.') df.plot(kind = 'bar',figsize = (12,5),title = 'A_sale to B_sale:bar',width = 0.8,alpha = 0.8)


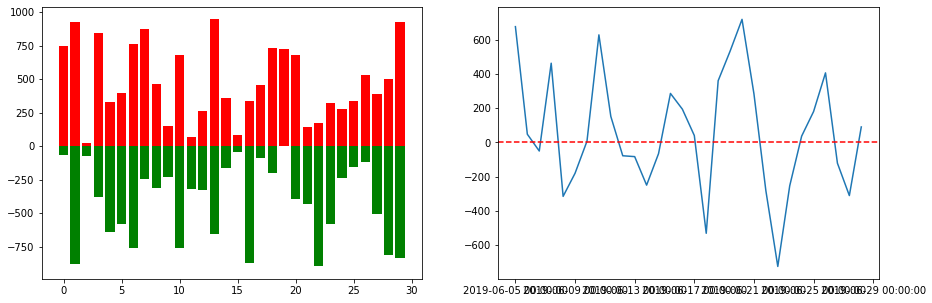
x = range(len(df)) y1 = df['A_sale'] y2 = -df['B_sale']fig,axes = plt.subplots(1,2,figsize = (15,5)) ax1 = axes[0] ax1.bar(x,y1,facecolor = 'r') ax1.bar(x,y2,facecolor = 'g')ax2 = axes[1] y3 = df['A_sale'] - df['B_sale'] ax2.plot(x,y3) plt.axhline(0,linestyle = '--',color = 'r') #在y值为0处生成一条水平线 ax2.set_xticklabels(df.index[::4])
https://www.cnblogs.com/shengyang17/p/9630749.html





)



)







)

