When a single drop of paint is dropped on a surface the amount of space that the drop will cover depends both on time and space. A short amount of time will no be enough for the drop to cover a greater area, and a small surface will bound the surface that the paint can cover. The same rules could be applied to a variety of phenomena, mixing chemicals, bacterial growth, or the spread of ideas or even the spread of infectious diseases. Although there exists a huge amount of research on the modeling of infectious diseases, the application of simple rules can offer some insights and easy interpretability on how a disease could spread.

当将一滴油漆滴在表面上时，该滴将覆盖的空间量取决于时间和空间。 短时间不足以使液滴覆盖更大的区域，而小的表面将束缚油漆可以覆盖的表面。 相同的规则可以应用于多种现象，包括化学物质混合，细菌生长，思想传播甚至传染病传播。 尽管存在大量关于传染病建模的研究，但简单规则的应用可以为疾病的传播提供一些见识并易于解释。

Let’s assume the following, an unknown disease is spreading through time and space in a population. Each square dot represents an individual, a black square represents an individual without the pathogen and a withe square represents an individual with the pathogen. Each frame represents a time step and each time step is equal to the time needed for an individual to spread the disease. As a novel disease, the amount of infected individuals is minimal. Each susceptible individual on the population will be affected by four surrounding neighbors. Those neighbors could be infected or uninfected. And for an individual to be infected there is a minimum of infected neighbors needed to infect the individual.

让我们假设以下情况，一种未知的疾病正在人口中随时间和空间传播。 每个正方形点代表一个个体，黑色正方形代表没有病原体的个体，而带有正方形的方块代表有病原体的个体。 每个帧代表一个时间步长，每个时间步长等于个体传播疾病所需的时间。 作为一种新型疾病，受感染个体的数量很少。 人口中的每个易感个体都将受到周围四个邻居的影响。 那些邻居可能被感染或未被感染。 对于一个要被感染的个体，感染该个体所需的感染邻居最少。

By changing the number of infected neighbors needed to infect an individual a series of scenarios can be evaluated. Under the first couple of scenarios where the individual needs to be surrounded by four, three, or two infected neighbors the infection is unable to spread.

通过更改感染个人所需的被感染邻居的数量，可以评估一系列情况。 在前两个场景中，个人需要被四个，三个或两个受感染的邻居包围，感染无法传播。

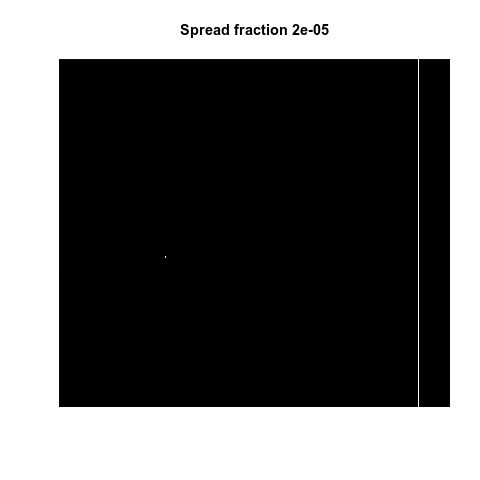
However, when only one infected individual is needed to spread the pathogen a dramatic increase in the infected population can be seen. Different clusters of infection can be observed through time and after some time those clusters start to merge.

但是，当只需要一个被感染的个体来传播病原体时，可以看到感染人口的急剧增加。 随着时间的流逝，可以观察到不同的感染群，一段时间后这些群开始合并。

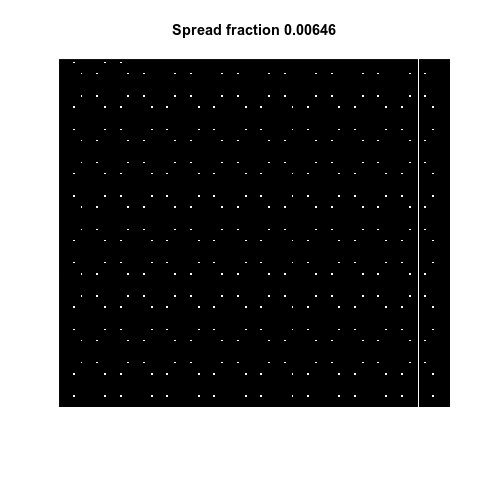
Under the previous scenarios, about 0.01% of the population carries the pathogen in a secluded area. Increasing the number of infected populations to about 1% a similar pattern is observed. When the individual can be infected only when is surrounded by two three or four infected neighbors the infection is unable to spread. However, in some cases, the infection does not disappear but stays in small clusters of infected populations.

在以前的情况下，约有0.01％的人口将病原体携带在一个僻静的地区。 将感染种群的数量增加到大约1％，可以观察到类似的模式。 只有在被两个三个或四个感染邻居包围的情况下，个体才能被感染，这种感染无法传播。 但是，在某些情况下，感染并不会消失，而是停留在感染人群的小群中。

When only one neighbor is needed to infect an individual, the infection spreads dramatically. And almost instantaneously the pathogen can infect the entire population.

当只需要一个邻居来感染一个人时，感染就会Swift蔓延。 而且，病原体几乎可以瞬间感染整个人群。

To this point, it appears that the only scenario where the infection spreads through the population is when the pathogen can infect an individual when only one infected neighbor is needed to propagate the disease. And how rapidly the infection spread is proportional to the initial number of the infected population. Let’s see if that last assumption holds, now an evenly spaced number of infected individuals will be placed over the grid and evaluate the spread of the pathogen.

在这一点上，似乎感染在人群中蔓延的唯一情况是当只需要一个被感染的邻居来传播疾病时，病原体就可以感染一个人。 感染传播的速度与感染人口的初始数量成正比。 让我们看看最后一个假设是否成立，现在将等间隔分布的受感染个体放在网格上并评估病原体的传播。

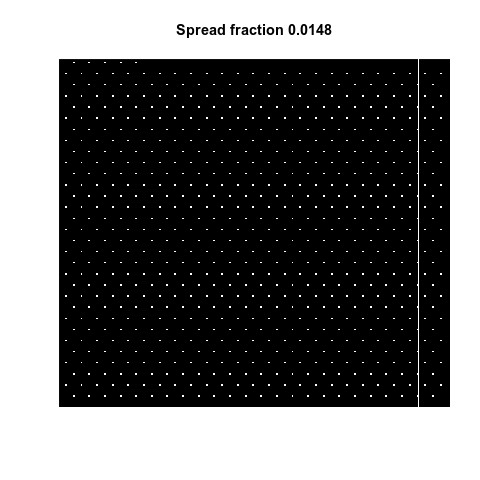
Under those constraints, it appears that an evenly spaced grid is not able to infect the entire population but reach an equilibrium. Looks like the randomness involved in how the individuals carry the pathogen helps its spread.

在这些约束下，似乎间隔均匀的网格无法感染整个种群，但可以达到平衡。 看起来个体携带病原体的方式所涉及的随机性有助于其传播。

Previous simulations exemplify how a pathogen can propagate through space and time. However, with an outbreak of a new disease, a common governmental intervention taken through an outbreak of an unknown disease is the restriction of social mobility, closing economic activities, restricting travel, and putting the population under quarantine. Social distancing is enforced as a measure to return to economic and social activities. Let’s enforce social distancing by adding a grid where there are no individuals available to propagate the disease.

先前的模拟例证了病原体如何在时空中传播。 但是，随着新疾病的爆发，通过未知疾病的爆发而采取的一项政府共同干预措施是限制社会流动性，结束经济活动，限制出行并将人口隔离。 强制执行社会疏离措施，以恢复经济和社会活动。 让我们通过在没有个人可以传播疾病的地方添加一个网格来加强社会疏远。

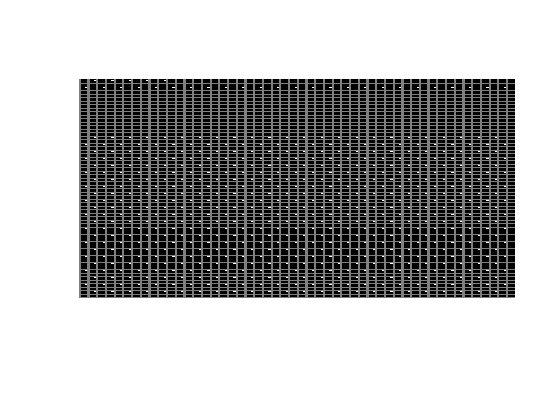
By applying those constrains a series of isolated clusters of infected individuals can be seen under every simulation configuration. Those results can show some resemblance to what can be happening at restaurants, cinemas, or some other establishments with large gatherings of people. If some of the people attending those establishments have the pathogen, the pathogen will be unable to spread trough its neighbors by simply applying social distancing. Diminishing the number of people that can be close together depletes the pathogen capacity to spread.

通过应用这些约束，可以在每种模拟配置下看到一系列隔离的感染个体簇。 这些结果可能与在餐馆，电影院或其他人群聚集的其他场所可能发生的情况相似。 如果在这些机构中的某些人患有病原体，则仅通过社会隔离即可使病原体无法通过邻居传播。 减少可以靠近的人的数量会耗尽病原体的传播能力。

The previous simulations are based on the two-dimensional cellular automata. With four neighbors and one individual in the middle, also known as the five neighbors two-dimensional automata. One of the most famous examples of two-dimensional automata is the one proposed by John Horton Conway also known as the game of life. The complete code to perform the previous simulations can be found in my GitHub by clicking here. See you in the next one.

先前的模拟基于二维细胞自动机。 在中间有四个邻居和一个人，也称为五个邻居二维自动机。 二维自动机最著名的例子之一就是约翰·霍顿·康威(John Horton Conway)提出的例子，也被称为生活游戏。 单击此处，可以在我的GitHub中找到执行先前模拟的完整代码。 下一个见。