Abstract:– Analysis of the visual representations of exponentials.– Proposals to solve current visualization issues.– Call to discussion to come up with a better visual representation convention.

抽象： –分析指数的视觉表示形式。–解决当前可视化问题的建议。–进行讨论以提出更好的视觉表示形式惯例。

“The greatest shortcoming of the human race is our inability to understand the exponential function.” — Albert Allen Bartlett, Physics professor

“人类最大的缺点是我们无法理解指数函数。” —物理教授Albert Allen Bartlett

Our big brains brought us the capability to predict the future, and it worked great when our environment was moving on a (rather slow) linear progression, when our ‘food’ moved around the fields from A to B at a constant pace. But many critical aspects of XXI century life move at an exponential rate: human population, computer power, online

我们的大脑使我们有能力预测未来，当我们的环境以(而不是缓慢的)线性发展，当我们的“食物”以恒定的速度从A移到B时，它发挥了很大的作用。 但是，二十一世纪生活的许多关键方面都以指数级增长：人口，计算机能力，在线

Graphs and data visualization have been helping understand complex numerical information for centuries with one of the most powerful human tool, an image.

数百年来，图形和数据可视化一直通过最强大的人工工具之一图像帮助理解复杂的数字信息。

科学的视觉表示 (Scientific Visual Representations)

Science has two ways to visually represent exponentials: linear & logarithmic.

科学有两种直观地表示指数的方式：线性和对数。

Both are accurate, valid and perfectly understandable by people with a scientific background, but it shows some issues when it comes to the mainstream intuition interpretation.

两者都是准确，有效且具有科学背景的人可以完全理解的，但是当涉及到主流直觉解释时，这会显示一些问题。

一直一样 (All the Same, All The way)

Any section of a linear exponential representation will give you the same trajectory because we tend to escalate the Y axis. The spread of a virus will look similar in a graph of the first 10 days and the first 100 days.

线性指数表示的任何部分都会给您相同的轨迹，因为我们倾向于将Y轴升级。 在前10天和前100天的图表中，病毒的传播看起来类似。

走向无限 (Tendency to Infinite)

An exponential curve seems to tend to infinite, but infinite is a rare thing in the real world; actually, most exponential growth or decays are interrupted by some event. It is the lack of a limit reference that makes it feel an endless progression.

指数曲线似乎趋向于无限，但无限在现实世界中是罕见的。 实际上，大多数指数增长或衰变被某个事件中断。 缺少极限参考使它感到无止境地前进。

缺乏细节 (Lack of details)

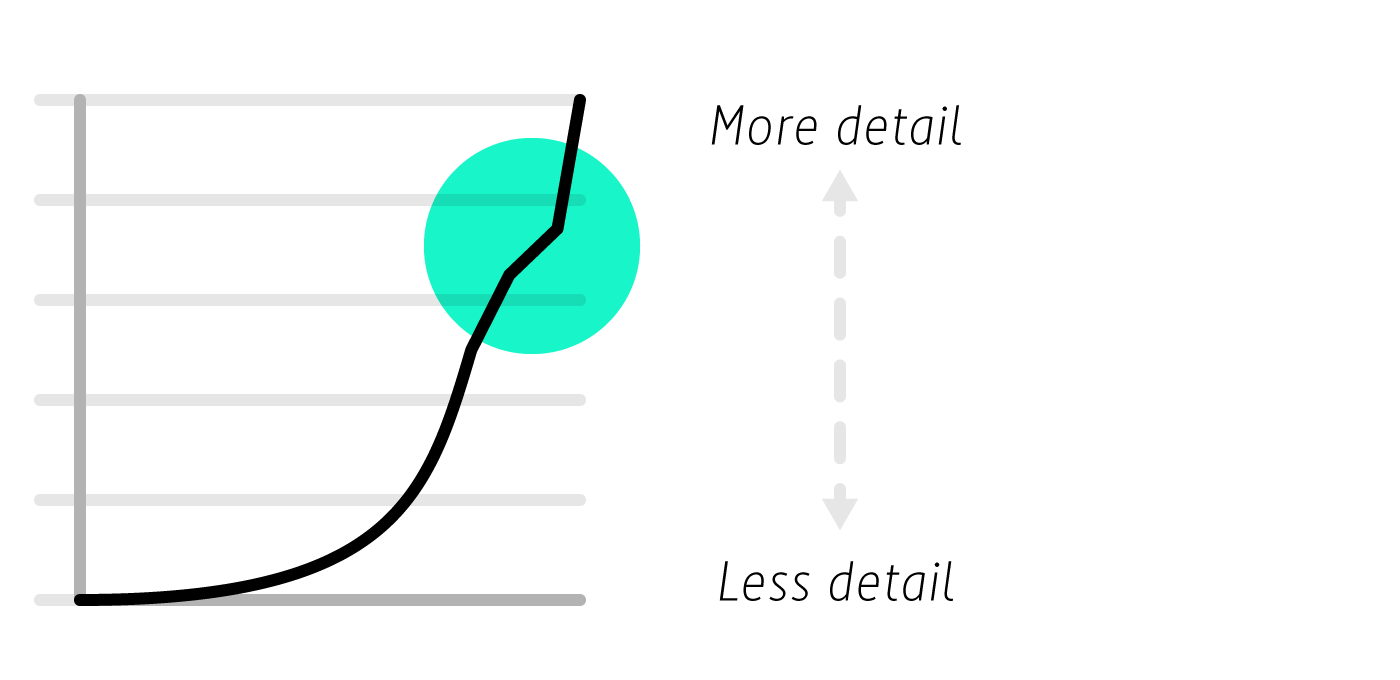
A linear graph will only show more details on the high part of the chart.

线性图只会在图表的较高部分显示更多详细信息。

A logarithmic graph will only show details on the low part of the chart.

对数图将仅在图表的下部显示详细信息。

人民指数 (Exponentials for the People)

Human culture is poor in exponential references. The most relevant ones being:

人类文化的指数引用很差。 最相关的是：

Paal Payasam的传说 (Legend of Paal Payasam)

The Indian Legend of Paal Payasam, in which a reward consisting on doubling the grains of wheat on the squares of a chessboard ends up with an overwhelming amount that surpasses all the amount of wheat grain in the world.

印度传说中的Paal Payasam ，在其中获得的奖励是，在棋盘的正方形上将小麦粒加倍，最终获得的压倒性数量超过了世界上所有小麦粒的数量。

十种力量 (Powers of Ten)

The film Powers of Ten by Charles and Ray Eames, which zooms out from our ‘human scale’ exponentially to the scale of the entire universe and then zooms in to the scale of an atom.

查尔斯和雷·伊姆斯 ( Charles and Ray Eames)制作的电影《十大力量》 ，从我们的“人类规模”以指数方式缩小到整个宇宙的规模，然后放大到原子的规模。

更好的视觉方法 (A Better Visual Approach)

To help the lack of natural human skills to understand exponentials it seems relevant to find new ways to visually represent them in a humanly intuitive way.

为了帮助缺乏自然的人类技能来理解指数，寻找新的方式以人类直观的方式直观地表示它们似乎很重要。

Nowadays, Infographists develop amazingly creative ways to make abstract data understandable to everyone, but they are mostly individual solutions per each case.

如今，Infographists开发了令人惊讶的创造性方法，使每个人都可以理解抽象数据，但是对于每种情况，它们大多是单独的解决方案。

It seems necessary to find a visual system relevant for exponentials, accurate and simple enough to be easily implemented and understood.

似乎有必要找到一种与指数相关的视觉系统，其准确度和简单度应易于实现和理解。

As a Graphic Designer myself, I approached this issue and come up with some early concepts that can help better understand exponentials.

作为一名平面设计师，我亲自解决了这个问题，并提出了一些早期概念，可以帮助更好地理解指数。

While there are out there some examples of exponential visualizations showing its progress as an animation or video –the same way Powers of Ten does or like this example of computing exponential growth– I consider that motion generates more complexity in terms of creation and readability, and we miss the simplicity of a single visual impact. So, while I don’t discard a dynamic solution, I prioritize a static image system.

尽管有一些指数化可视化的示例，以动画或视频的形式展示了它的进展-就像《十方 幂 》所做的一样，还是像这个计算指数级增长的示例一样-我认为运动在创建和可读性方面会产生更多的复杂性，并且我们错过了单一视觉冲击的简单性。 因此，尽管我不放弃动态解决方案，但仍将静态图像系统作为优先级。

分层景观 (Layered Landscape)

Logarithmic graphs visually remind me of a landscape perspective, where closer objects look bigger and further objects look smaller.

对数图在视觉上让我想起了景观角度，附近的物体看起来更大而其他物体看起来更小。

But in logarithmic graphs there’s a disruptively wrong element that kills the perspective, the line. Imagine the line as a path. If we scale the thickness of the line proportionally to the change of scale it matches our ‘perspective language’ and it makes it more comprehensive.

但是在对数图中，存在破坏性的错误元素，这扼杀了透视线。 想象这条线是一条路径。 如果我们根据比例尺的变化成比例地缩放线的粗细，则它与我们的“透视语言”相匹配，并且使其更加全面。

Different visual codes would even make it more equal in terms of data details inside the same chart; for example using escalated circles.

不同的视觉代码甚至可以使同一图表内的数据细节更加平等； 例如使用升级的圈子。

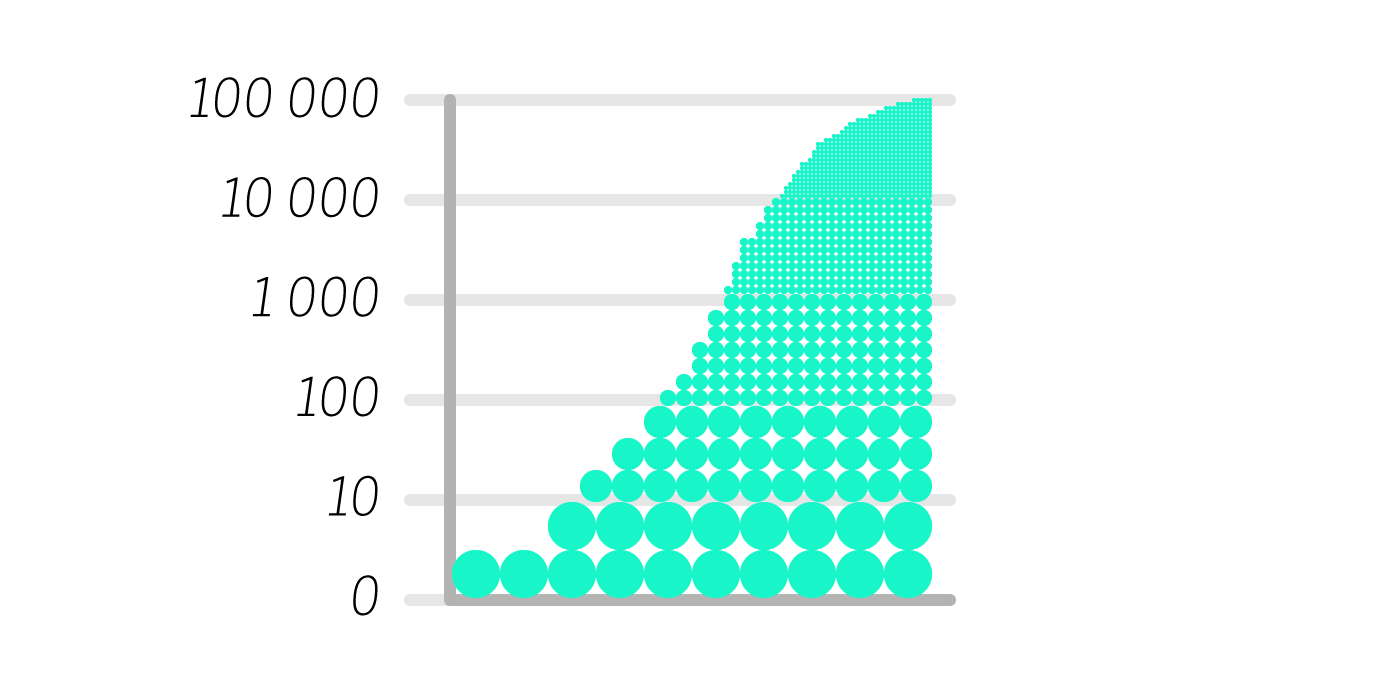
有限参考 (Finite Reference)

As mentioned before, despite the impression of a curve to the infinite, exponentials have limits and representing this reality (as far as possible) would help understand where is this curve going.

如前所述，尽管给人以无限曲线的印象，但指数是有极限的，并且(尽可能)表示这一现实将有助于理解该曲线的去向。

While a context reference is sometimes used in a graph explanation or in the graph itself, the suggestion is to close down the graph itself where the trajectory seems to continue to infinite.

虽然有时在图形说明中或图形本身中使用上下文引用，但建议是在轨迹似乎继续无限的地方关闭图形本身。

工作正在进行中 (Work in Progress)

Those are very simple approximations to solve the issues above mentioned, not except of issues themselves. I’m personally invested in the topic and will try to learn more about it and find better solutions.

这些是解决上述问题的非常简单的近似方法，除了问题本身。 我对这个主题进行了个人投资，并将尝试进一步了解该主题并找到更好的解决方案。

讨论电话 (A Call to Discussion)

I write this article from a designer perspective and with no scientific background, my purpose is to open a debate for science and design to reach a convention that feels natural for everyone to understand. My approaches are just a kick-start and I’d like to hear the thoughts of other creative brains –either scientific or designer.

我是从设计者的角度写这篇文章的，没有科学背景，我的目的是为科学和设计展开一场辩论，以达成让每个人都理解的惯例。 我的方法只是一个开端，我想听听其他有创造力的大脑(无论是科学专家还是设计师)的想法。

I encourage others to explore the topic and I propose the hashtag #VisualizingExponentials (any platform) to follow up on other comments and approaches. I’ll look at putting together a compilation in short time. I’m thrilled to see where this can go.

我鼓励其他人探索该主题，并建议使用#VisualizingExponentials (任何平台)标签，以跟进其他评论和方法。 我将在很短的时间内整理一下汇编。 我很高兴看到这可以去哪里。

Related Links

相关链接

Visual explanation of exponentials: https://setosa.io/ev/exponentiation/ by Victor Powell

：指数的视觉解释 https://setosa.io/ev/exponentiation/ 由 维克多·鲍威尔

An approach to exponential bias applied to SARS-CoV-2 spread case https://towardsdatascience.com/dealing-with-our-systematic-misjudgment-of-covid-19-exponential-growth-3d367c9546d9 by Yannis Kopsinis

Yannis Kopsinis的 SARS-CoV-2传播案例的指数偏倚方法 https://towardsdatascience.com/dealing-with-our-systematic-misjudgment-of-covid-19-exponential-growth-3d367c9546d9