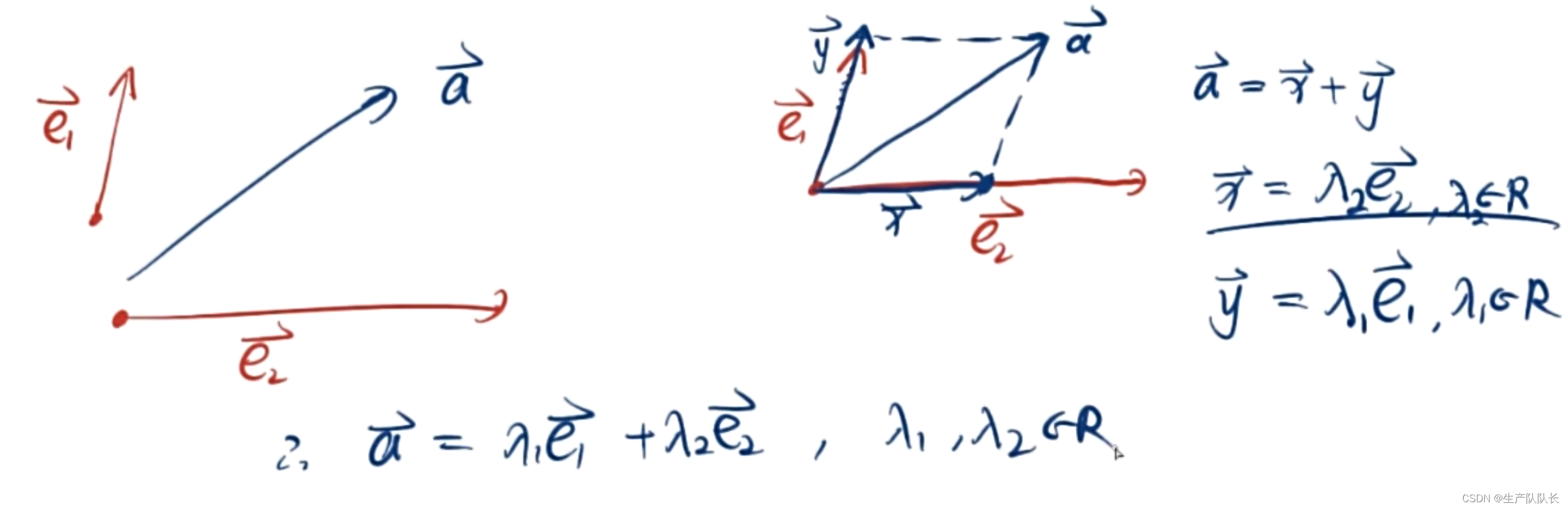
一、基本定理
二、向量夹角
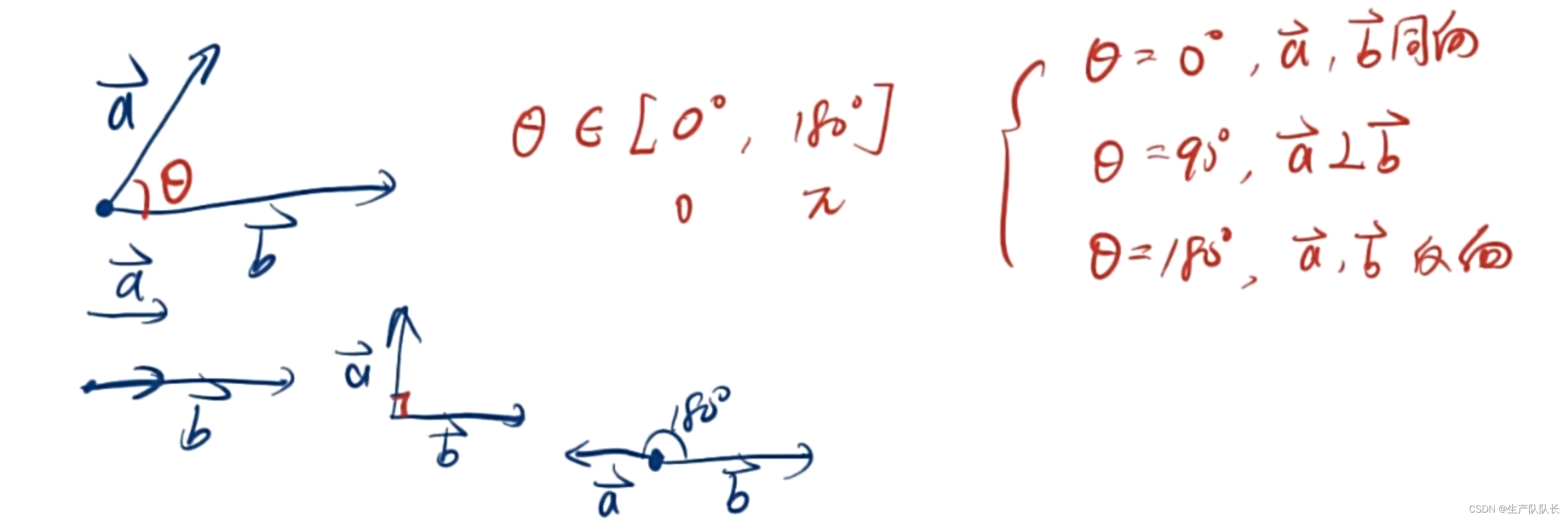
三、常用性质
三角形中线对应的向量与三角形两边的关系
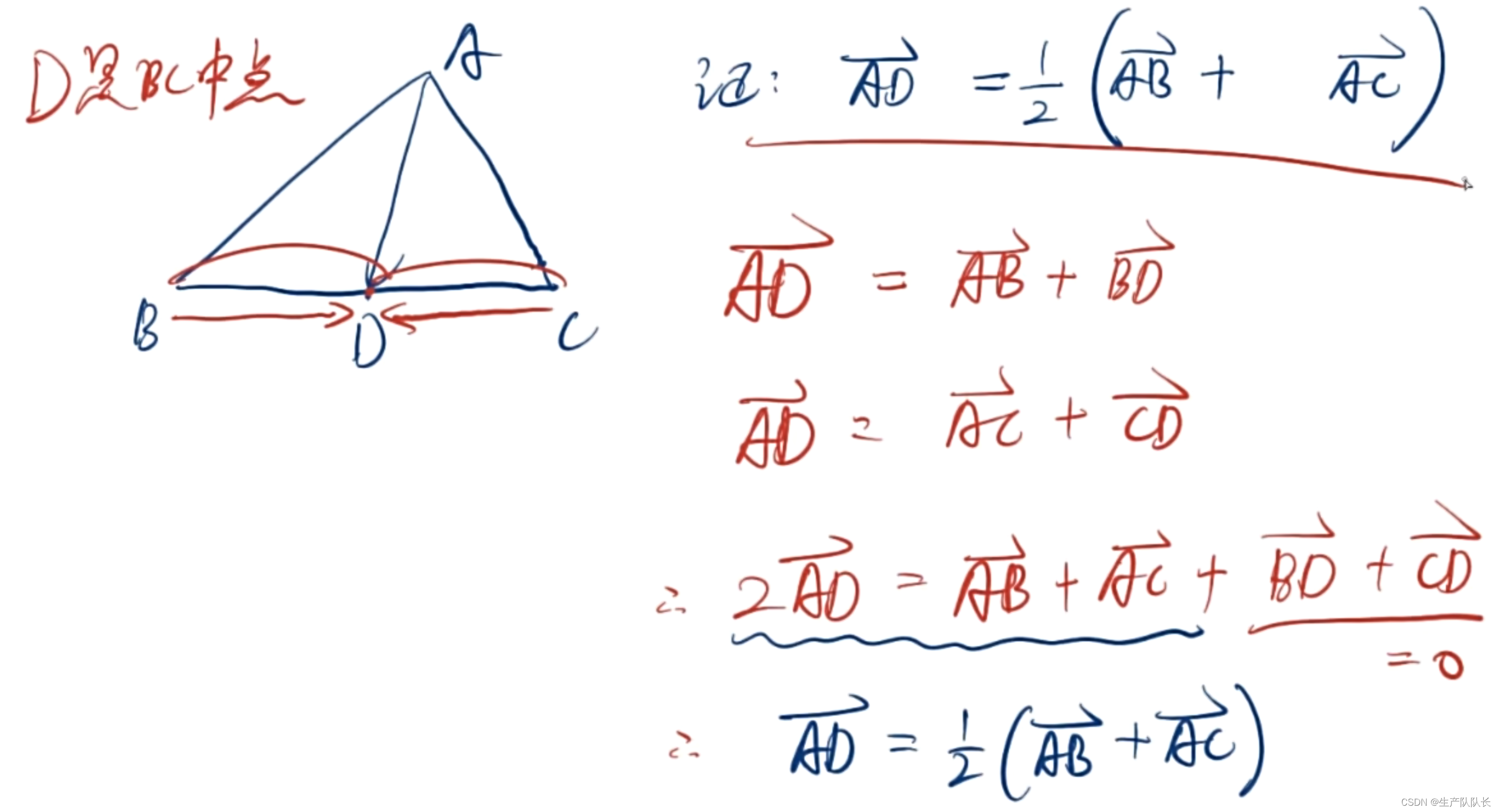
四、练习
例题1
例题2
解
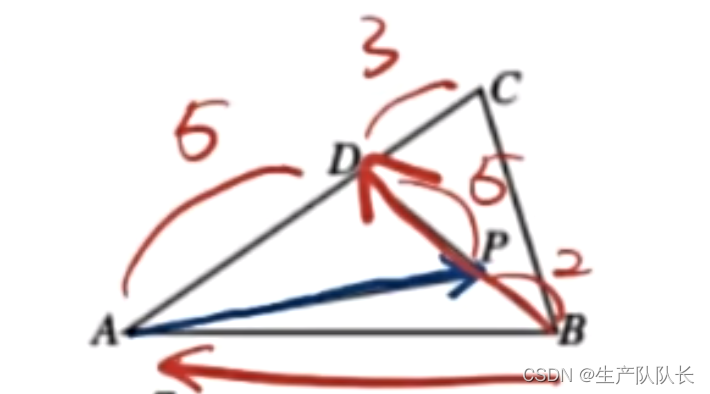
例题3
解
此题，用到了向量的基本定理：若 a → \mathop{a}\limits ^{\rightarrow} a→、 b → \mathop{b}\limits ^{\rightarrow} b→是平面内不共线的两向量，则对于 c → \mathop{c}\limits ^{\rightarrow} c→，有且仅有仅有一组x、y，使得 c → \mathop{c}\limits ^{\rightarrow} c→=x a → \mathop{a}\limits ^{\rightarrow} a→+y b → \mathop{b}\limits ^{\rightarrow} b→。
-架构图)
![[实用技巧]Unity中，Sprite和SpriteRenderer的实用小贴士](http://pic.xiahunao.cn/[实用技巧]Unity中，Sprite和SpriteRenderer的实用小贴士){kind=icon}
