文章目录
- 引言
- 复习
- 树形DP——树的最长路径
- 新作
- 使用dfs非递归计算二叉树的深度
- 多个区间合并删除问题
- 实现思路
- 实现代码
- 参考思路
- 总结
引言
- 这两天可能有点波动,但是算法题还是尽量保证复习和新作一块弄,数量上可能有所差别。
复习
树形DP——树的最长路径
- 这道题是没有完全听完,但是到现在这个阶段,最起码得数组实现邻接链表做完。
无向图的一维数组表示邻接表实现
- 首先说明一下,这里要使用邻接链表实现,这里是使用一维数组实现的邻接链表。
- 同时这里是双向链表,所以,要加两次边,具体是实现如下

下面开始具体的分析
-
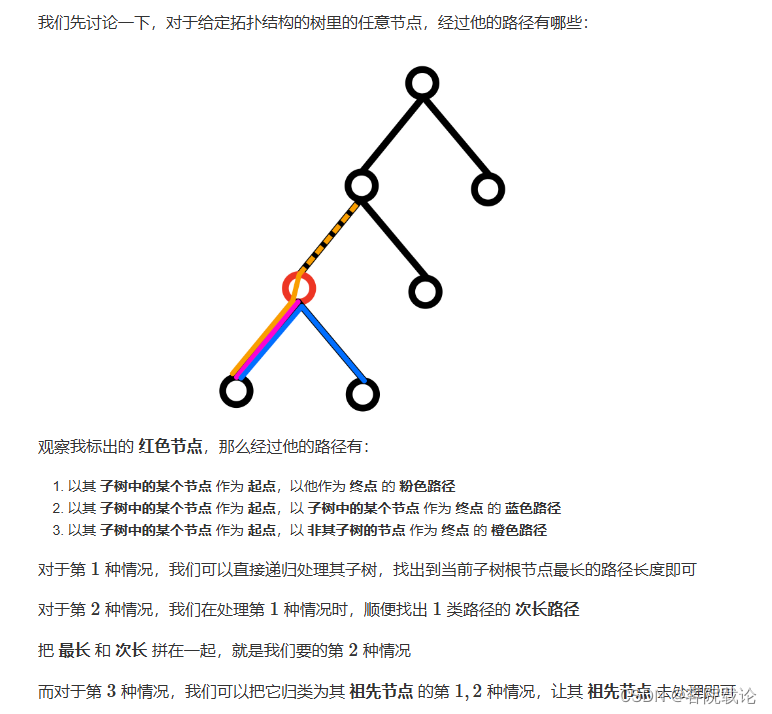
树是一个确定的拓扑结构,每一个节点之间是是存在对应的父子关系,所以列觉路径可以更换为枚举中间节点,具体分析见下图,这是参考别人的,分析的很有道理
-
通过红色节点的有三条路径
- 红色路径,是一红色节点为根节点的最长的路径
- 蓝色路径,是以红色节点为跟节点的最长路径和次长路径的和
- 橙色路径,是红色节点的父节点的相同情况,具体见上图。
-
相当于这道题,就是在遍历的过程中,计算对应的最长路径和子路径,然后在计算两者的和。
图片参考来源
动态规划分析
- 这道题是两个动态规划,分别是动态规划计算最长的路径,次长的路径,而且是一个很明显的动态规划题目,就是子状态影响最终的状态。
- 所以状态转移函数,就是计算的最长路径和次长路径,这里的动态规划,就是两个路径,f1[i]表示以节点i为根节点的最长路径,f2[i]表示以i为根节点的次长路径。具体如下


#include <iostream>
#include <algorithm>
#include <cstring>
using namespace std;const int N = 10010;
int h[N],e[2*N],ne[2*N],w[2*N],idx;
int f1[N],f2[N],res; // f1保存最长的转移路径,f2保存次长的转移路径
int n;void add(int a,int b,int c){e[idx] = b;w[idx] = c;ne[idx] = h[a];h[a] = idx ++;
}void dfs(int r,int father){// 这里r是对应的根节点,father是对应的父节点f1[r] = 0,f2[r] = 0;for (int i = h[r]; ~i; i = ne[i]) {int j = e[i]; // 确定子节点的编号if (j == father) continue;dfs(j,r);if(f1[j] + w[i] >= f1[r]) f2[r] = f1[r],f1[r] = f1[j] + w[i];else if(f1[j] + w[i] > f2[r]) f2[r] = f1[j] + w[i];}res = max(res,f1[r] + f2[r]);
}int main(){cin>>n;// 构建无向图memset(h,-1,sizeof(h));for (int i = 0; i < n -1; ++i) {int a,b,c;cin>>a>>b>>c;add(a,b,c),add(b,a,c);}// 遍历对应的无向图for (int i = h[1]; ~i; i = ne[i]) {cout<<1<<" "<<e [i]<<endl;}cout<<res;return 0;
}
- 这道题拖了那么久,乍一看,其实还是蛮简单的,思路只要清楚了,后续还是很好实现的,然后那个使用一维数组实现的邻接链表的,之前是没有做过,现在知道怎么用了,还是蛮简单的。
新作
使用dfs非递归计算二叉树的深度
-
dfs非递归二叉树高度,一开始写了个经典队列的bfs,意识到不对后开始改,最后没改完,就说了个暴力找到每个叶子的高度的思路。
-
这个忘记的有点多,如果单纯使用栈来实现的话,就需要每一次入栈当前节点的子节点还有对应的深度,然后出栈,如果是叶子节点,就比较一下长度,如果不是,就继续做出栈和入栈的操作。
-
这里实现的基本上和我写的比较类似
#include <iostream>
#include <stack>using namespace std;struct TreeNode{int val;TreeNode* left;TreeNode* right;TreeNode(int x):val(x),left(NULL),right(NULL){};
};// 生成样例
TreeNode* createSampleTree1() {TreeNode* root = new TreeNode(1);root->left = new TreeNode(2);root->right = new TreeNode(3);root->left->left = new TreeNode(4);root->left->right = new TreeNode(5);root->right->right = new TreeNode(6);root->left->left->left = new TreeNode(7);return root;
}int treeHeight(TreeNode* root){// 使用非递归的方式计算的树深度stack<pair<TreeNode *,int>> s;// 根节点入栈并重置深度s.push({root,1});int r = 0;// 出栈并遍历每一个节点的子节点while(!s.empty()){TreeNode* t = s.top().first;int l = s.top().second;s.pop();// 判定左子节点是否为空if (t->left) s.push({t->left,l + 1});if (t->right) s.push({t->right,l + 1});// 比较深度r = max(r,l);}return r;
}int main(){TreeNode* root = createSampleTree1();cout<<treeHeight(root);
}
- 参考方法
- 这里指的学习的是一个auto的使用,通过下述方式可以直接进行遍历使用。
auto [node, depth] = stack.top();
#include <iostream>
#include <stack>
#include <algorithm> // for maxstruct TreeNode {int val;TreeNode *left;TreeNode *right;TreeNode(int x) : val(x), left(NULL), right(NULL) {}
};int treeHeight(TreeNode* root) {if (root == nullptr) return 0;std::stack<std::pair<TreeNode*, int>> stack;stack.push({root, 1});int maxHeight = 0;while (!stack.empty()) {auto [node, depth] = stack.top();stack.pop();if (node != nullptr) {maxHeight = std::max(maxHeight, depth);if (node->left != nullptr) stack.push({node->left, depth + 1});if (node->right != nullptr) stack.push({node->right, depth + 1});}}return maxHeight;
}int main() {// 示例二叉树TreeNode* root = new TreeNode(1);root->left = new TreeNode(2);root->right = new TreeNode(3);root->left->left = new TreeNode(4);root->left->right = new TreeNode(5);std::cout << "树的高度是: " << treeHeight(root) << std::endl;// 清理内存delete root->left->left;delete root->left->right;delete root->left;delete root->right;delete root;return 0;
}
多个区间合并删除问题
- 这个也是在网上搜索的,部分拼多多主管面可能问到的题目,所以这里做一下,具体题目描述如下
给定一个n×2的二维数组,数组的每一行代表一个区间,
如果一个区间被另一个区间包含就删掉该区间,返回剩
下的所有区间。
* 比如: [1 2]被[1 ,3]包含。
实现思路
- 这里是使用自定义排序实现的,如果包含关系,就将之按照包含的关系进行排序,然后在进行从前往后逐步进行遍历,对于相同的数组直接去除。最后剩下的就是对应的元素。
- 自定义排序实现贪婪算法!
- 这个方法确实是有问题的,有一部分样例是没有考虑到,不过背一下这个模版的。
实现代码
#include <iostream>
#include <vector>
#include <algorithm>using namespace std;using Interval = pair<int,int>;vector<Interval> removeContainedIntervals(vector<Interval>& intervals){// 可以对区间进行排序,按照包含关系进行排序vector<Interval> res;sort(intervals.begin(),intervals.end(),[](auto a,auto b){if(a.first <= b.first && a.second >= b.second) return 1;else return 0;});for (int i = 0;i < intervals.size();i ++) {Interval t = intervals[i];res.push_back(t);while((i + 1 < intervals.size())&& t.first <= intervals[i+1].first&& t.second >= intervals[i+1].second)i ++;}return res;
}int main() {vector<vector<Interval>> samples = {{{1, 4}, {2, 3}, {1, 3}, {4, 6}, {5, 7}},
// {{1, 5}, {2, 4}, {6, 8}, {7, 9}, {5, 10}},
// {{1, 2}, {3, 4}, {2, 3}, {1, 5}, {6, 7}},
// {{1, 2}, {2, 3}, {3, 4}, {4, 5}},
// {{1, 3}, {2, 6}, {8, 10}, {15, 18}}};for (size_t i = 0; i < samples.size(); ++i) {vector<Interval> result = removeContainedIntervals(samples[i]);cout << "样例 " << i + 1 << " 剩余的区间为:" << endl;for (const auto& interval : result) {cout << "[" << interval.first << ", " << interval.second << "] ";}cout << endl;}return 0;
}
参考思路
- 这里是一个贪心算法解决区间问题的模板,需要好好练习一下,和我的方法差不多,只不过他是针对单边进行排序的
- 这里是使用标准区间进行比较的,会更加灵活方便一点,比我的方法要好很多。
#include <iostream>
#include <vector>
#include <algorithm>using namespace std;// 定义一个区间类型
using Interval = pair<int, int>;// 比较函数,用于排序区间
bool compareIntervals(const Interval &a, const Interval &b) {if (a.first == b.first) {return a.second < b.second;}return a.first < b.first;
}vector<Interval> removeContainedIntervals(vector<Interval>& intervals) {// 如果区间列表为空,直接返回空列表if (intervals.empty()) {return {};}// 按照起始点排序,起始点相同则按照终止点排序sort(intervals.begin(), intervals.end(), compareIntervals);vector<Interval> result;Interval last = intervals[0]; // 初始化第一个区间作为比较基准for (size_t i = 1; i < intervals.size(); ++i) {// 如果当前区间不被上一个区间包含if (intervals[i].first > last.first && intervals[i].second > last.second) {result.push_back(last); // 保留上一个区间last = intervals[i]; // 更新比较基准} else {// 如果当前区间的终止点更长,更新比较基准if (intervals[i].second > last.second) {last = intervals[i];}}}// 最后一个区间也需要保留result.push_back(last);return result;
}int main() {vector<vector<Interval>> samples = {{{1, 4}, {2, 3}, {1, 3}, {4, 6}, {5, 7}},{{1, 5}, {2, 4}, {6, 8}, {7, 9}, {5, 10}},{{1, 2}, {3, 4}, {2, 3}, {1, 5}, {6, 7}},{{1, 2}, {2, 3}, {3, 4}, {4, 5}},{{1, 3}, {2, 6}, {8, 10}, {15, 18}}};for (size_t i = 0; i < samples.size(); ++i) {vector<Interval> result = removeContainedIntervals(samples[i]);cout << "样例 " << i + 1 << " 剩余的区间为:" << endl;for (const auto& interval : result) {cout << "[" << interval.first << ", " << interval.second << "] ";}cout << endl;}return 0;
}
总结
- 复习的那个最长路径,还是蛮简单的,今天终于看懂了,继续加油吧。明天可以快速写一下。
- 今天算是做了两道新的题目,dfs获取树的深度那道题,是自己做出来的,然后区间合并的那道题,是参考别人的,不过看了题解,加油吧!明天把题目再过一遍!
 的保留与重置)





)



)






)

)