个人主页~

二叉树-堆（上）
栈和队列

---

四、堆的代码实现

Heap.h

#pragma once#include <stdio.h>
#include <stdlib.h>
#include <assert.h>
typedef int HPDataType;typedef struct Heap
{HPDataType* a;int size;int capacity;
}Heap;
// 堆的初始化
void HeapInit(Heap* hp);
// 堆的销毁
void HeapDestory(Heap* hp);
// 堆的插入
void HeapPush(Heap* hp, HPDataType x);
// 堆的删除
void HeapPop(Heap* hp);
// 取堆顶的数据
HPDataType HeapTop(Heap* hp);
// 堆的数据个数
int HeapSize(Heap* hp);
// 堆的判空
int HeapEmpty(Heap* hp);
void AdjustUp(HPDataType* a, int child); 
//向下调整算法
void AdjustDown(HPDataType* a, int n, int parent);

Heap.c

#include "Heap.h"void Swap(HPDataType* n1, HPDataType* n2)
{HPDataType* tmp = *n1;*n1 = *n2;*n2 = tmp;
}void HeapInit(Heap* hp)
{assert(hp);hp->a = NULL;hp->capacity = hp->size = 0;
}void HeapDestory(Heap* hp)
{assert(hp);free(hp->a);hp->a = NULL;hp->capacity = hp->size = 0;
}void HeapPush(Heap* hp, HPDataType x)
{assert(hp);if(hp->capacity == hp->size){int newcapacity = hp->capacity == 0 ? 4 : hp->capacity * 2;HPDataType* tmp = (HPDataType*)realloc(hp->a, newcapacity);if (tmp == NULL){perror("realloc fail");return;}hp->a = tmp;hp->capacity = newcapacity;}hp->a[hp->size] = x;hp->size++;AdjustUp(hp->a, hp->size - 1);
}void HeapPop(Heap* hp)
{assert(hp);assert(!HeapEmpty(hp));Swap(&hp->a[0], &hp->a[hp->size - 1]);hp->size--;AdjustDown(hp->a, hp->size, 0);
}HPDataType HeapTop(Heap* hp)
{assert(hp);assert(!HeapEmpty(hp));return hp->a[0];
}int HeapSize(Heap* hp)
{assert(hp);return hp->size;
}int HeapEmpty(Heap* hp)
{assert(hp);return hp->size == 0;
}void AdjustUp(HPDataType* a, int child)
{int parent = (child - 1) / 2;//while (parent >= 0)while (child > 0){if (a[child] > a[parent]){Swap(&a[child], &a[parent]);child = parent;parent = (child - 1) / 2;}else{break;}}
}void AdjustDown(HPDataType* a, int n, int parent)
{int child = parent * 2 + 1;while (child < n){// 选出左右孩子中小/大的那个if (child + 1 < n && a[child + 1] > a[child]){child++;}if (a[child] > a[parent]){Swap(&a[parent], &a[child]);parent = child;child = parent * 2 + 1;}else{break;}}
}

test.c

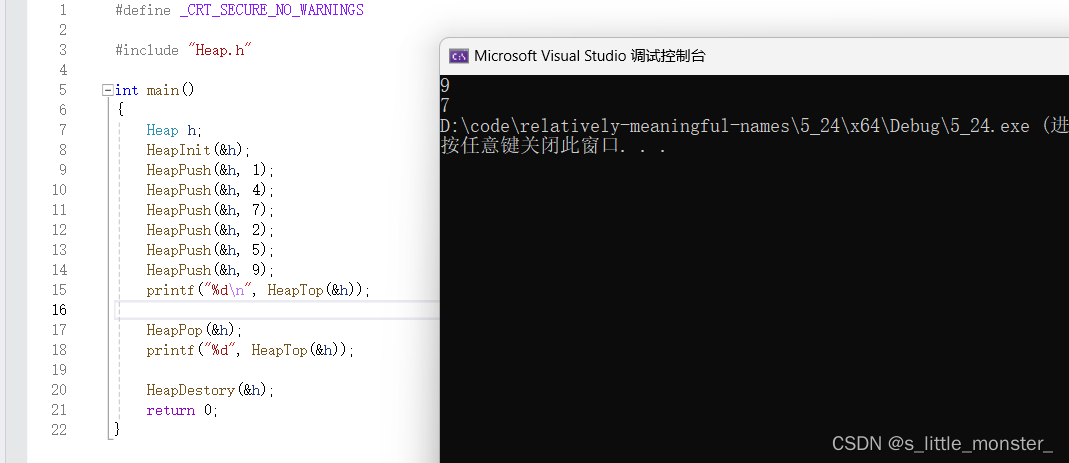
#include "Heap.h"int main()
{Heap h;HeapInit(&h);HeapPush(&h, 1);HeapPush(&h, 4);HeapPush(&h, 7);HeapPush(&h, 2);HeapPush(&h, 5);HeapPush(&h, 9);printf("%d\n", HeapTop(&h));HeapPop(&h);printf("%d", HeapTop(&h));HeapDestory(&h);return 0;
}

五、堆的应用

堆排序思想进行排序

我们在上面实现了堆，如果想要升序数组就建大堆，降序数组就建小堆
但建堆并不意味着建完就可以了，想要升序/降序数组的话，建完大堆/小堆后用向下调整算法将堆调整成小堆/大堆，这样调整出来的堆就是一个升序/降序数组

在排序当中，堆排序是一种时间复杂度较低的排序，要远优于冒泡排序，在使用堆排序时，要使用向下调整算法，这样我们就可以最大限度的减少时间的使用

在堆排序中有一个很经典的问题就是TopK问题，即一堆数据，个数为n（n>>k）,求这堆数据中最大/最小的k个数据
如果是求前k个最大的元素，则用前k个元素建小堆
如果是求前k个最小的元素，则用前k个元素建大堆
然后再用剩下的n-k个元素一次与堆顶元素来比较，不满足则替换堆顶元素
也就是说，我们用求前k个最大数据来举例，我们先将整组数据的前k个元素建一个小堆，小堆的根是整个堆里最小的，用它来和剩余的n-k个元素比较，如果剩余的元素中的某一个比小堆根大，那么就替换掉，再用向下调整算法调整，这样一来，最大的数据都沉底了，堆中最小的数据继续与剩余的数据比较，重复上述步骤，当所有剩余元素都比完了之后，剩下的这个小堆就是前k个最大数

六、二叉树链式结构的实现

BTree.h

#include <stdio.h>
#include <stdlib.h>
#include <assert.h>typedef char BTDataType;typedef struct BinaryTreeNode
{BTDataType data;struct BinaryTreeNode* left;struct BinaryTreeNode* right;
}BTNode;// 通过前序遍历的数组"ABD##E#H##CF##G##"构建二叉树
BTNode* BinaryTreeCreate(BTDataType* a, int* pi);
// 二叉树销毁
void BinaryTreeDestory(BTNode* root);
// 二叉树节点个数
int BinaryTreeSize(BTNode* root);
// 二叉树叶子节点个数
int BinaryTreeLeafSize(BTNode* root);
// 二叉树第k层节点个数
int BinaryTreeLevelKSize(BTNode* root, int k);
// 二叉树查找值为x的节点
BTNode* BinaryTreeFind(BTNode* root, BTDataType x);
// 二叉树前序遍历 
void BinaryTreePrevOrder(BTNode* root);
// 二叉树中序遍历
void BinaryTreeInOrder(BTNode* root);
// 二叉树后序遍历
void BinaryTreePostOrder(BTNode* root);

BTree.c

#define _CRT_SECURE_NO_WARNINGS#include "BTree.h"BTNode* BuyNode(BTDataType x)
{BTNode* new = (BTNode*)malloc(sizeof(BTNode));if (new == NULL){perror("malloc fail");return NULL;}new->data = x;new->left =  NULL;new->right = NULL;return new;
}BTNode* BinaryTreeCreate(BTDataType* a,int n, int* pi) 
{if (*pi >= n || a[*pi] == '#'){ // 如果到达数组末尾或遇到#，则返回NULL  (*pi)++;return NULL;}BTNode* node = BuyNode(a[*pi]);(*pi)++; // 移动到下一个节点  node->left = BinaryTreeCreate(a, n, pi); // 递归创建左子树  node->right = BinaryTreeCreate(a, n, pi); // 递归创建右子树  return node;
}void BinaryTreeDestory(BTNode* root)
{if (root == NULL)return;BinaryTreeDestory(root->left);BinaryTreeDestory(root->right);free(root);
}int BinaryTreeSize(BTNode* root)
{//return root == NULL ? 0 : BinaryTreeSize(root->left) + BinaryTreeSize(root->right) + 1;if (root == NULL)return 0;return BinaryTreeSize(root->left) + BinaryTreeSize(root->right) + 1;
}int BinaryTreeLeafSize(BTNode* root)
{if (root == NULL)return 0;if (root->left == NULL && root->right == NULL)return 1;return BinaryTreeLeafSize(root->left) + BinaryTreeLeafSize(root->right);
}int BinaryTreeLevelKSize(BTNode* root, int k)
{assert(k > 0);if (root == NULL)return 0;if (k == 1)return 1;return BinaryTreeLevelKSize(root->left, k - 1) + BinaryTreeLevelKSize(root->right, k - 1);
}BTNode* BinaryTreeFind(BTNode* root, BTDataType x)
{if (root == NULL)return NULL;if (root->data = x)return root;BTNode* ret1 = BinaryTreeFind(root->left, x);if (ret1)return ret1;BTNode* ret2 = BinaryTreeFind(root->right, x);if (ret2)return ret2;return NULL;
}void BinaryTreePrevOrder(BTNode* root)
{if (root == NULL){printf("N ");return;}printf("%c ", root->data);BinaryTreePrevOrder(root->left);BinaryTreePrevOrder(root->right);}void BinaryTreeInOrder(BTNode* root)
{if (root == NULL){printf("N ");return;}BinaryTreeInOrder(root->left);printf("%c ", root->data);BinaryTreeInOrder(root->right);}void BinaryTreePostOrder(BTNode* root)
{if (root == NULL){printf("N ");return;}BinaryTreeInOrder(root->left);BinaryTreeInOrder(root->right);printf("%c ", root->data);}

test.c

#define _CRT_SECURE_NO_WARNINGS#include "BTree.h"int main()
{int i = 0;BTDataType val[] = { "ABD##E#H##CF##G##" };BTNode* tree = BinaryTreeCreate(val, 17, &i);BinaryTreePrevOrder(tree);printf("\n");BinaryTreeInOrder(tree);printf("\n");BinaryTreePostOrder(tree);printf("\n");printf("%d\n", BinaryTreeSize(tree));printf("%d\n", BinaryTreeLeafSize(tree));printf("%d\n", BinaryTreeLevelKSize(tree,3));BinaryTreeDestory(tree);return 0;
}

---

下一篇我们来详细剖析链式二叉树的实现~