数据结构、算法总述:数据结构/算法 C/C++-CSDN博客
AVL树
定义
- 空二叉树是一个 AVL 树
- 如果 T 是一棵 AVL 树,那么其左右子树也是 AVL 树,并且
,h 是其左右子树的高度
- 树高为
平衡因子:右子树高度 - 左子树高度
创建节点
由于 AVL 树的相关操作需要获取节点高度,因此我们需要为节点类添加 height 变量:
/* AVL 树节点类 */
struct TreeNode {int val{}; // 节点值int height = 0; // 节点高度TreeNode *left{}; // 左子节点TreeNode *right{}; // 右子节点TreeNode() = default;explicit TreeNode(int x) : val(x){}
};“节点高度”是指从该节点到它的最远叶节点的距离,即所经过的“边”的数量。需要特别注意的是,叶节点的高度为 0 ,而空节点的高度为 −1 。我们将创建两个工具函数,分别用于获取和更新节点的高度:
/* 获取节点高度 */
int height(TreeNode *node) {// 空节点高度为 -1 ,叶节点高度为 0return node == nullptr ? -1 : node->height;
}/* 更新节点高度 */
void updateHeight(TreeNode *node) {// 节点高度等于最高子树高度 + 1node->height = max(height(node->left), height(node->right)) + 1;
}节点的平衡因子(balance factor)定义为节点左子树的高度减去右子树的高度,同时规定空节点的平衡因子为 0 。我们同样将获取节点平衡因子的功能封装成函数,方便后续使用:
/* 获取平衡因子 */
int balanceFactor(TreeNode *node) {// 空节点平衡因子为 0if (node == nullptr)return 0;// 节点平衡因子 = 左子树高度 - 右子树高度return height(node->left) - height(node->right);
}旋转
我们将平衡因子绝对值 >1 的节点称为“失衡节点”。根据节点失衡情况的不同,旋转操作分为四种:右旋、左旋、先右旋后左旋、先左旋后右旋。
右旋

/* 右旋操作 */
TreeNode *rightRotate(TreeNode *node) {TreeNode *child = node->left;TreeNode *grandChild = child->right;// 以 child 为原点,将 node 向右旋转child->right = node;node->left = grandChild;// 更新节点高度updateHeight(node);updateHeight(child);// 返回旋转后子树的根节点return child;
}左旋
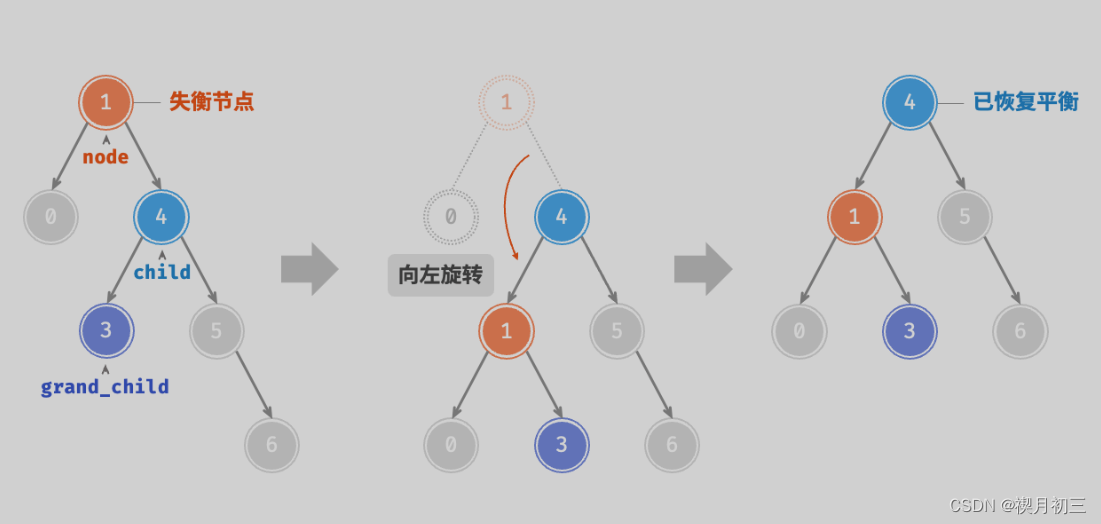
/* 左旋操作 */
TreeNode *leftRotate(TreeNode *node) {TreeNode *child = node->right;TreeNode *grandChild = child->left;// 以 child 为原点,将 node 向左旋转child->left = node;node->right = grandChild;// 更新节点高度updateHeight(node);updateHeight(child);// 返回旋转后子树的根节点return child;
}先左旋后右旋
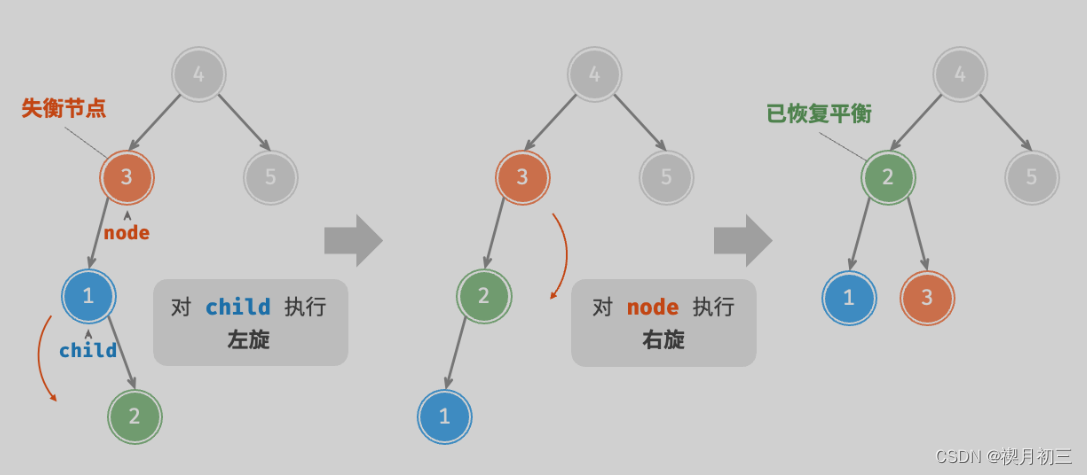
仅使用左旋或右旋都无法使子树恢复平衡。此时需要先对 child 执行“左旋”,再对 node 执行“右旋”。
先右旋后左旋
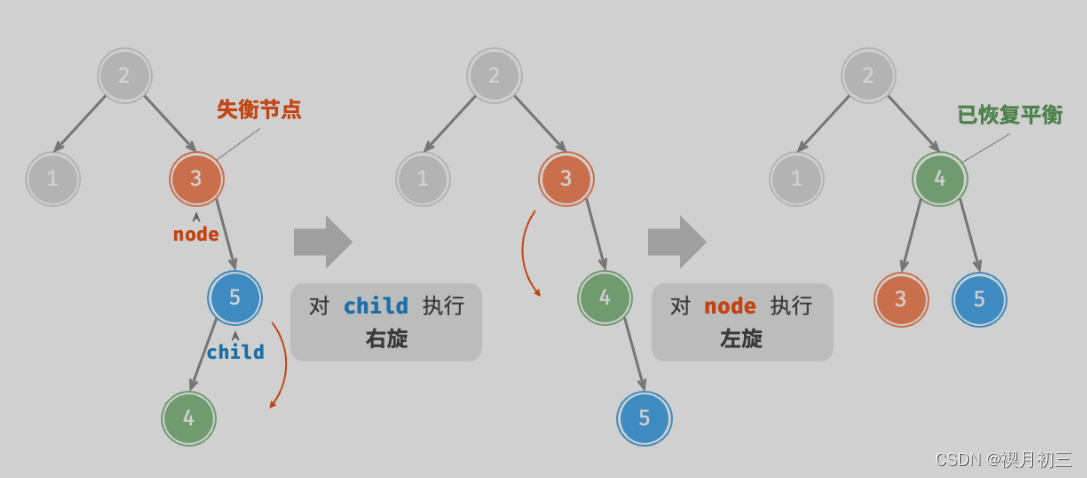
旋转的选择
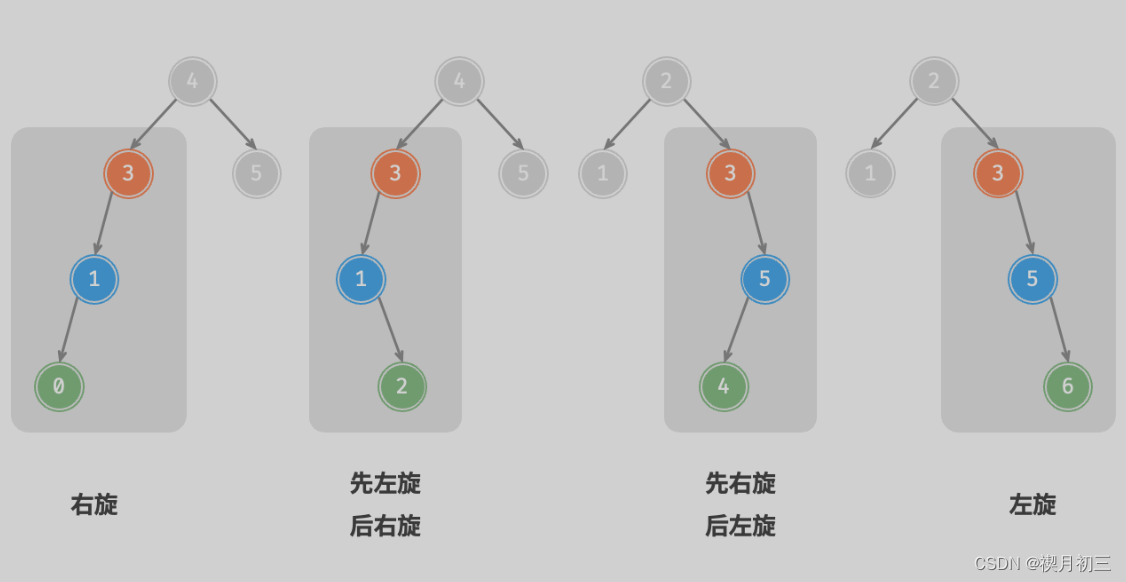
判断失衡节点的平衡因子以及较高一侧子节点的平衡因子的正负号,来确定旋转方式:
| 失衡节点的平衡因子 | 子节点的平衡因子 | 旋转方式 |
|---|---|---|
| >1 | ≥0 | 右旋 |
| >1 | <0 | 先左旋后右旋 |
| <-1 | ≤0 | 左旋 |
| <-1 | >0 | 先右旋后左旋 |
/* 执行旋转操作,使该子树重新恢复平衡 */
TreeNode *rotate(TreeNode *node) {// 获取节点 node 的平衡因子int _balanceFactor = balanceFactor(node);// 左偏树if (_balanceFactor > 1) {if (balanceFactor(node->left) >= 0) {// 右旋return rightRotate(node);} else {// 先左旋后右旋node->left = leftRotate(node->left);return rightRotate(node);}}// 右偏树if (_balanceFactor < -1) {if (balanceFactor(node->right) <= 0) {// 左旋return leftRotate(node);} else {// 先右旋后左旋node->right = rightRotate(node->right);return leftRotate(node);}}// 平衡树,无须旋转,直接返回return node;
}基础操作
插入
在 AVL 树中插入节点后,从该节点到根节点的路径上可能会出现一系列失衡节点。因此,我们需要从这个节点开始,自底向上执行旋转操作,使所有失衡节点恢复平衡。
/* 插入节点 */
void insert(int val) {root = insertHelper(root, val);
}/* 递归插入节点(辅助方法) */
TreeNode *insertHelper(TreeNode *node, int val) {if (node == nullptr)return new TreeNode(val);/* 1. 查找插入位置并插入节点 */if (val < node->val)node->left = insertHelper(node->left, val);else if (val > node->val)node->right = insertHelper(node->right, val);elsereturn node; // 重复节点不插入,直接返回updateHeight(node); // 更新节点高度/* 2. 执行旋转操作,使该子树重新恢复平衡 */node = rotate(node);// 返回子树的根节点return node;
}删除
在二叉搜索树的删除节点方法的基础上,需要从底至顶执行旋转操作,使所有失衡节点恢复平衡。
/* 删除节点 */
void remove(int val) {root = removeHelper(root, val);
}/* 递归删除节点(辅助方法) */
TreeNode *removeHelper(TreeNode *node, int val) {if (node == nullptr)return nullptr;/* 1. 查找节点并删除 */if (val < node->val)node->left = removeHelper(node->left, val);else if (val > node->val)node->right = removeHelper(node->right, val);else {if (node->left == nullptr || node->right == nullptr) {TreeNode *child = node->left != nullptr ? node->left : node->right;// 子节点数量 = 0 ,直接删除 node 并返回if (child == nullptr) {delete node;return nullptr;}// 子节点数量 = 1 ,直接删除 nodeelse {delete node;node = child;}} else {// 子节点数量 = 2 ,则将中序遍历的下个节点删除,并用该节点替换当前节点TreeNode *temp = node->right;while (temp->left != nullptr) {temp = temp->left;}int tempVal = temp->val;node->right = removeHelper(node->right, temp->val);node->val = tempVal;}}updateHeight(node); // 更新节点高度/* 2. 执行旋转操作,使该子树重新恢复平衡 */node = rotate(node);// 返回子树的根节点return node;
}查找
与二叉搜索树一致
AVL树总代码
AVLTree.h
#include <iostream>
#include <assert.h>
using namespace std;template<class K, class V>
struct AVLTreeNode
{AVLTreeNode* _left;AVLTreeNode* _right;AVLTreeNode* _parent;int _bf; // balance factorpair<K, V> _kv;AVLTreeNode(const pair<K, V>& kv): _left(nullptr), _right(nullptr), _parent(nullptr), _bf(0), _kv(kv){}
};template<class K, class V>
class AVLTree
{typedef AVLTreeNode<K, V> Node;
public:bool Insert(const pair<K, V>& kv){if (_root == nullptr){_root = new Node(kv);}Node* cur = _root;Node* parent = nullptr;while (cur){if (cur->_kv.first < kv.first){parent = cur;cur = cur->_right;}else if (cur->_kv.first > kv.first){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(kv);if (parent->_kv.first > kv.first)parent->_left = cur;elseparent->_right = cur;cur->_parent = parent;while (parent)//最坏情况一直更新到root,此时parent为nullptr{if (cur == parent->_left)//更新平衡因子parent->_bf--;elseparent->_bf++;if (parent->_bf == 0){break;}else if (parent->_bf == 1 || parent->_bf == -1)//向上查找,检测是否出现不平衡{cur = cur->_parent;parent = parent->_parent;}else if (parent->_bf == 2 || parent->_bf == -2){//旋转if (parent->_bf == 2 && cur->_bf == 1)//2,1说明右边子树深度阶梯式增加,所以往左旋转RotateL(parent);//左单旋else if (parent->_bf == -2 && cur->_bf == -1)//-2,-1说明左边子树深度阶梯式增加,所以往右旋转RotateR(parent);//右单旋else if (parent->_bf == -2 && cur->_bf == 1)//-2, 1说明左边子树中间深,先左单旋提高中间节点,再右单旋提高中间节点RotateLR(parent);else if (parent->_bf == 2 && cur->_bf == -1)//2, -1说明右边子树中间深,先右单旋提高中间节点,再左单旋提高中间节点RotateRL(parent);elseassert(false);break;}else //说明插入前AVL出现了问题,直接报错{assert(false);}}return true;}//左单旋void RotateL(Node* parent){Node* subR = parent->_right;Node* subRL = subR->_left;parent->_right = subRL;if (subRL)subRL->_parent = parent;subR->_left = parent;Node* ppNode = parent->_parent;parent->_parent = subR;if (parent == _root){_root = subR;subR->_parent = nullptr;}else{if (ppNode->_left == parent)ppNode->_left = subR;elseppNode->_right = subR;subR->_parent = ppNode;}parent->_bf = 0;subR->_bf = 0;}//右单旋void RotateR(Node* parent){Node* subL = parent->_left;Node* subLR = subL->_right;parent->_left = subLR;if (subLR)subLR->_parent = parent;subL->_right = parent;Node* ppNode = parent->_parent;parent->_parent = subL;if (parent == _root){_root = subL;subL->_parent = nullptr;}else{if (ppNode->_left == parent)ppNode->_left = subL;elseppNode->_right = subL;subL->_parent = ppNode;}parent->_bf = 0;subL->_bf = 0;}//左右双旋void RotateLR(Node* parent){Node* subL = parent->_left;Node* subLR = subL->_right;int bf = subLR->_bf;RotateL(parent->_left);RotateR(parent);if (bf == -1){subLR->_bf = 0;subL->_bf = 0;parent->_bf = 1;}else if (bf == 1){subLR->_bf = 0;subL->_bf = -1;parent->_bf = 0;}else if (bf == 0){subLR->_bf = 0;subL->_bf = 0;parent->_bf = 0;}else{assert(false);}}//右左双旋void RotateRL(Node* parent){Node* subR = parent->_right;Node* subRL = subR->_left;int bf = subRL->_bf;RotateR(parent->_right);RotateL(parent);if (bf == -1){subR->_bf = 1;subRL->_bf = 0;parent->_bf = 0;}else if (bf == 1){subR->_bf = 0;subRL->_bf = 0;parent->_bf = -1;}else if (bf == 0){subR->_bf = 0;subRL->_bf = 0;parent->_bf = 0;}else{assert(false);}}//中序void InOrder(){_InOrder(_root);cout << "end" << endl;}private://中序void _InOrder(Node* root){if (root == nullptr)return;_InOrder(root->_left);cout << root->_kv.first << " - ";_InOrder(root->_right);}Node* _root = nullptr;
};
应用
- 组织和存储大型数据,适用于高频查找、低频增删的场景。
红黑树
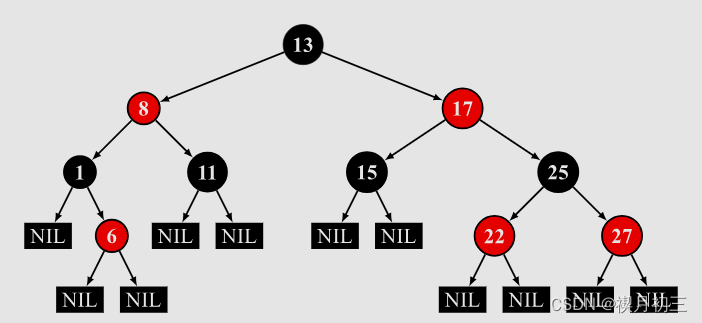
红黑树是一种自平衡的二叉搜索树。每个节点额外存储了一个 color 字段 ("RED" or "BLACK"),用于确保树在插入和删除时保持平衡
性质
- 节点为红色或黑色
- 根节点必须为黑色
- NIL 节点(空叶子节点)为黑色
- 红色节点的子节点为黑色
- 从根节点到 NIL 节点的每条路径上的黑色节点数量相同
创建节点
enum Colour
{RED,BLACK
};template<class K, class V>
struct RBTreeNode
{RBTreeNode* _left;RBTreeNode* _right;RBTreeNode* _parent;pair<K, V> _kv;Colour _col;
};
_left:左子树_right:右子树_parent:父节点_kv:节点存储的值_col:该节点的颜色
构造函数
RBTreeNode(const pair<K, V>& kv): _left(nullptr), _right(nullptr), _parent(nullptr), _kv(kv), _col(RED)//初始化为红节点
{}
红黑树本体,类中只存储根节点_root
template<class K, class V>
class RBTree
{typedef RBTreeNode<K, V> Node;
private:Node* _root = nullptr;
}
插入
以二叉搜索树插入逻辑为基础:
bool Insert(const pair<K, V>& kv)
{if (_root == nullptr){_root = new Node(kv);_root->_col = BLACK;//保持根为黑节点}Node* cur = _root;Node* parent = nullptr;while (cur){if (cur->_kv.first < kv.first){parent = cur;cur = cur->_right;}else if (cur->_kv.first > kv.first){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(kv);if (parent->_kv.first > kv.first)parent->_left = cur;elseparent->_right = cur;cur->_parent = parent;//调整红黑树//......//......//......return true;
}
代码分析:
if (_root == nullptr) {_root = new Node(kv);_root->_col = BLACK;//保持根为黑节点 }插入节点时,根节点
_root为空,说明当前整棵树都为空,那么直接插入值作为根节点即可,但是根节点必须是黑色节点,而我们新插入的节点是红色,所以要将其调整为黑色节点。
while (cur) {if (cur->_kv.first < kv.first){parent = cur;cur = cur->_right;}else if (cur->_kv.first > kv.first){parent = cur;cur = cur->_left;}else{return false;} }找到合适的插入位置,当
key大于当前节点cur->_kv.first < kv.first,那么cur就向左寻找,反之向右寻找。如果当前节点值等于key,那么说明该节点已经存在,返回false代表插入失败。当我们的cur为空指针,说明已经找到了插入的节点,此时跳出循环进行插入。
cur = new Node(kv);if (parent->_kv.first > kv.first)parent->_left = cur; elseparent->_right = cur;cur->_parent = parent;到达此处,说明前面已经找到插入的位置了,而
parent节点就是插入位置的父亲节点。根据key的大小,来判断插入到左边还是右边,插入完成后,再让新节点的_parent指向parent。
分情况调整红黑树
对于红黑树的插入,我们需要关注新节点的父亲parent,祖父grandfather,叔叔uncle三个节点:

1.根据parent节点的颜色,来判断是否需要调整
parent节点为黑色
新插入的节点默认为红色,所以新插入节点不会影响路径上黑色节点的数目,而
parent是黑节点,我们也没有出现连续的红色节点,所以这种情况无需任何调整,直接插入就可以。
parent节点为红色
如果父亲节点为红色,我们就会出现连续的红色节点,这时我们就需要进行调整了
当parent为红色,我们就需要再根据uncle的颜色,将插入分类两类:uncle为红色以及uncle为黑色

注意:由于parent是红色节点,此时的grandfather一定是黑色节点
2.根据uncle节点的颜色,来判断如何调整
uncle节点为红色
当uncle节点为红色,此时需要进行变色

由于新插入了红色的cur节点,此时parent与cur出现了连续的红色节点,于是我们将parent改为黑色。但是此时以parent为根的所有路径就会多出一个黑节点,于是把grandfather变为红色,来抵消这个新增的黑节点。但是此时以uncle为根的路径又会少一个黑节点,于是把uncle变黑
但是我们将grandfather变为了红色,这有可能会影响到上一层节点
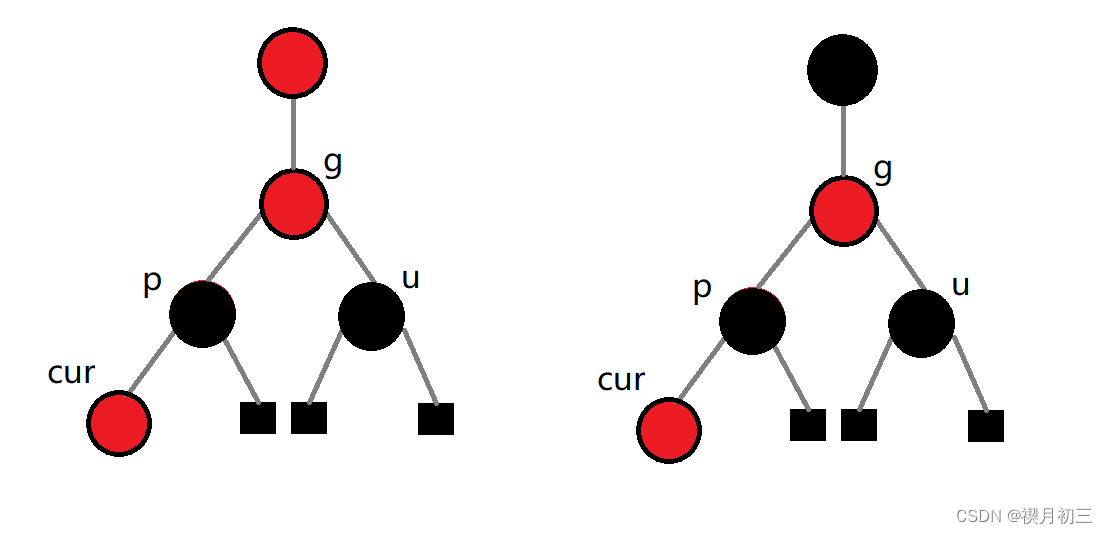
grandfather变红之后,可能出现两个红色节点相连的情况,所以我们要写一个while循环,来反复向上检查。
while (parent && parent->_col == RED)//只有parent为红,才更新 (parent可能不存在)
{Node* grandfather = parent->_parent;if (parent == grandfather->_left){Node* uncle = grandfather->_right;//uncle存在且为红节点if (uncle && uncle->_col == RED){parent->_col = uncle->_col = BLACK;grandfather->_col = RED;cur = grandfather;parent = cur->_parent;}else//uncle为黑节点 {//其它处理}}else{Node* uncle = grandfather->_left;//uncle存在且为红节点if (uncle && uncle->_col == RED){parent->_col = uncle->_col = BLACK;grandfather->_col = RED;cur = grandfather;parent = cur->_parent;}else//uncle为黑节点 {//其它处理}}
}_root->_col = BLACK;//在循环内部不判断root情况,统一处理
代码分析:
while (parent && parent->_col == RED)用于检测
cur的parent的颜色,通过我们前面的推导,如果parent为红色才需要调整,因此进入循环的条件之一是parent为红色。另外的parent有可能为NIL,此时我们要避免访问空指针,所以空指针也不能进循环
if (parent == grandfather->_left) { } else { }检测parent 节点是grandfather的左子树还是右子树,这将涉及到如何找uncle以及下一种情况的调整,此时我们要分类讨论:
当parent == grandfather->_left成立,那么uncle就是grandfather的右子树:Node* uncle = grandfather->_right;,反之就是左子树
if (uncle && uncle->_col == RED) {parent->_col = uncle->_col = BLACK;grandfather->_col = RED;cur = grandfather;parent = cur->_parent; }找到
uncle后,如果uncle是红色,那么直接进行变色操作,把parent和uncle的颜色变为黑色,grandfather变为红色。
随后由于我们的变色操作可能会影响上一层,此时调整节点,进入下一次while循环
_root->_col = BLACK;在先前的while循环中,有可能出现对
_root节点的操作,导致_root的颜色改变,而_root需要保持黑色。如果我们在循环内部,每一次都检测_root有点麻烦了,于是我们直接在每一次调整完节点后,把_root强行矫正为黑色
uncle节点为黑色
又因为红黑树中,NIL也算作黑色节点,所以uncle为黑色分为以下两种情况:
uncle为空指针uncle不为空指针

如果
uncle为空指针,那么cur一定是新插入的节点。
因为如果cur不是新插入的节点,那么cur和parent一定有一个原先是黑色节点,不然会出现连续的红色节点。但是如果cur和parent有一个是黑色节点,那么grandfather的左子树就比右子树多出一个黑节点,这就违背了红黑树规则。无论怎样,原先的树都不可能符合规则,所以cur一定是新插入的节点,破坏了规则。
如果
uncle不为空指针,那么cur一定是从黑色节点变成的红色节点(不是新插入的)。
因为如果uncle存在,那么grandfather的右子树就存在一个黑节点,而parent是红节点,所以cur和parent的右子树中都至少有一个黑节点,才能保证每一条路径黑节点数目相同。因此cur原先一定是黑节点,是因为cur下层插入了新节点,然后通过while循环向上走,影响到了当前层。
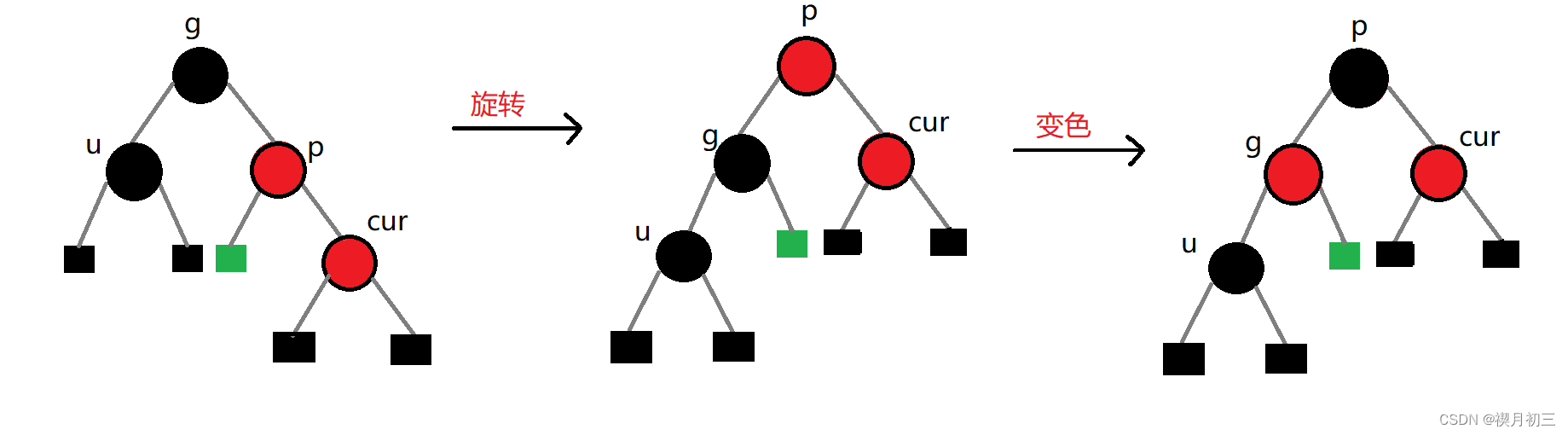
对于这种uncle为黑色的情况,我们需要通过旋转+变色来维持红黑树。
旋转又分单旋和双旋(同AVL树)
当
cur与parent的关系和parent与grandfather的关系一致时,需要进行单旋

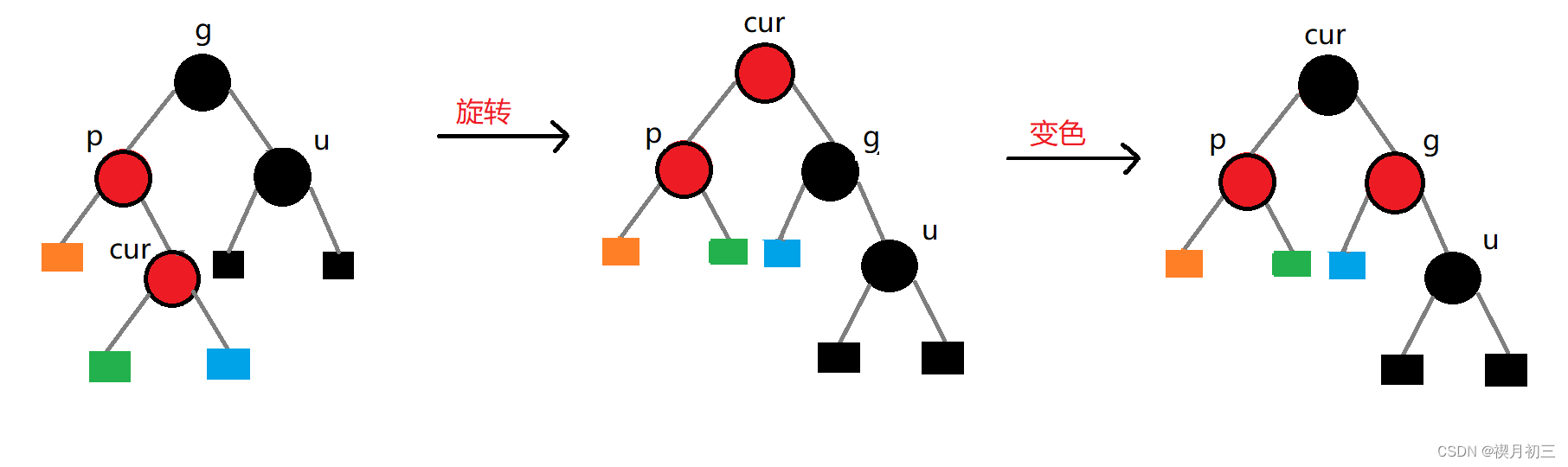
当
cur与parent的关系和parent与grandfather的关系不一致时,需要进行双旋
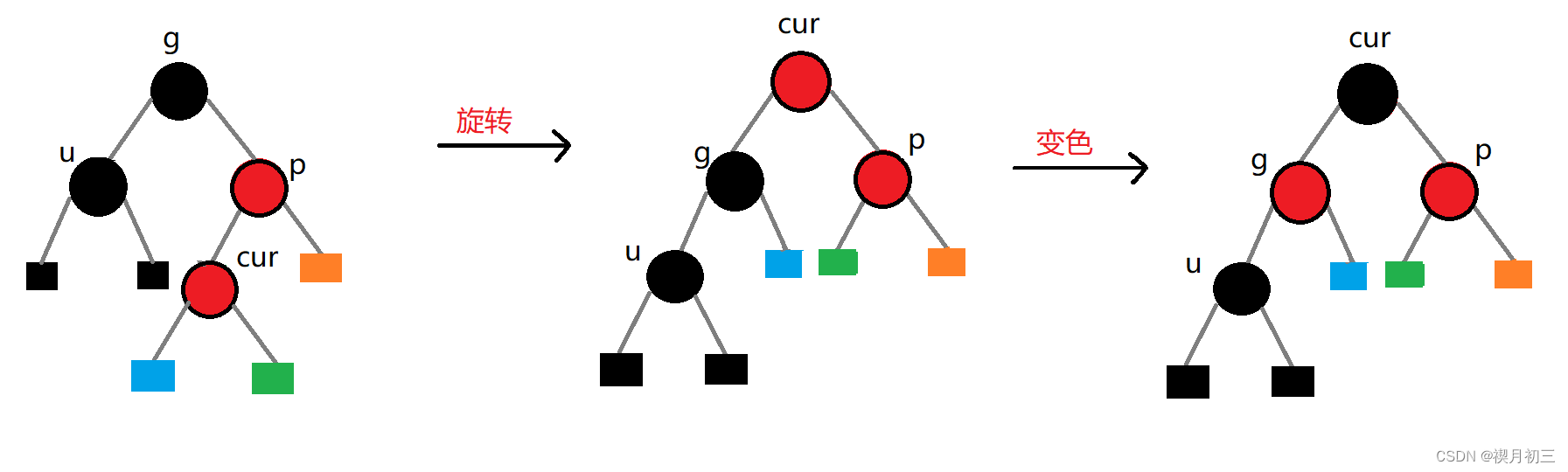
当parent == grandfather->_left
else//uncle为黑节点 (旋转)
{if (cur == parent->_left){RotateR(grandfather);//右单旋parent->_col = BLACK;//变色grandfather->_col = RED;//变色}else{RotateL(parent);//左右双旋 - 左单旋RotateR(grandfather);//左右双旋 - 右单旋cur->_col = BLACK;//变色grandfather->_col = RED;//变色}break;//旋转后一定平衡
}
当parent == grandfather->_right
else//uncle为黑节点 (旋转)
{if (cur == parent->_right){RotateL(grandfather);//左单旋parent->_col = BLACK;//变色grandfather->_col = RED;//变色}else{RotateR(parent);//右左双旋 - 右单旋RotateL(grandfather);//右左双旋 - 左单旋cur->_col = BLACK;//变色grandfather->_col = RED;//变色}break;//旋转后一定平衡
}
insert总代码
bool Insert(const pair<K, V>& kv)
{if (_root == nullptr){_root = new Node(kv);_root->_col = BLACK;//保持根为黑节点}Node* cur = _root;Node* parent = nullptr;while (cur){if (cur->_kv.first < kv.first){parent = cur;cur = cur->_right;}else if (cur->_kv.first > kv.first){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(kv);if (parent->_kv.first > kv.first)parent->_left = cur;elseparent->_right = cur;cur->_parent = parent;while (parent && parent->_col == RED)//只有parent为红,才更新 (parent可能不存在){Node* grandfather = parent->_parent;if (parent == grandfather->_left){Node* uncle = grandfather->_right;//uncle存在且为红节点if (uncle && uncle->_col == RED){parent->_col = uncle->_col = BLACK;grandfather->_col = RED;cur = grandfather;parent = cur->_parent;}else//uncle不存在或为黑节点 (旋转){if (cur == parent->_left){RotateR(grandfather);parent->_col = BLACK;grandfather->_col = RED;}else{RotateL(parent);RotateR(grandfather);cur->_col = BLACK;grandfather->_col = RED;}break;//旋转后一定平衡}}else{Node* uncle = grandfather->_left;//uncle存在且为红节点if (uncle && uncle->_col == RED){parent->_col = uncle->_col = BLACK;grandfather->_col = RED;cur = grandfather;parent = cur->_parent;}else//uncle不存在或为黑节点 (旋转){if (cur == parent->_right){RotateL(grandfather);parent->_col = BLACK;grandfather->_col = RED;}else{RotateR(parent);RotateL(grandfather);cur->_col = BLACK;grandfather->_col = RED;}break;//旋转后一定平衡}}}_root->_col = BLACK;//在循环内部不判断root情况,统一处理return true;
}
红黑树总代码
RBTree.h
#include <iostream>
#include <assert.h>
using namespace std;enum Colour
{RED,BLACK
};template<class K, class V>
struct RBTreeNode
{RBTreeNode* _left;RBTreeNode* _right;RBTreeNode* _parent;pair<K, V> _kv;Colour _col;RBTreeNode(const pair<K, V>& kv): _left(nullptr), _right(nullptr), _parent(nullptr), _kv(kv), _col(RED){}
};template<class K, class V>
class RBTree
{typedef RBTreeNode<K, V> Node;
public:bool Insert(const pair<K, V>& kv){if (_root == nullptr){_root = new Node(kv);_root->_col = BLACK;//保持根为黑节点}Node* cur = _root;Node* parent = nullptr;while (cur){if (cur->_kv.first < kv.first){parent = cur;cur = cur->_right;}else if (cur->_kv.first > kv.first){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(kv);if (parent->_kv.first > kv.first)parent->_left = cur;elseparent->_right = cur;cur->_parent = parent;while (parent && parent->_col == RED)//只有parent为红,才更新 (parent可能不存在){Node* grandfather = parent->_parent;if (parent == grandfather->_left){Node* uncle = grandfather->_right;//uncle存在且为红节点if (uncle && uncle->_col == RED){parent->_col = uncle->_col = BLACK;grandfather->_col = RED;cur = grandfather;parent = cur->_parent;}else//uncle不存在或为黑节点 (旋转){if (cur == parent->_left){RotateR(grandfather);parent->_col = BLACK;grandfather->_col = RED;}else{RotateL(parent);RotateR(grandfather);cur->_col = BLACK;grandfather->_col = RED;}break;//旋转后一定平衡}}else{Node* uncle = grandfather->_left;//uncle存在且为红节点if (uncle && uncle->_col == RED){parent->_col = uncle->_col = BLACK;grandfather->_col = RED;cur = grandfather;parent = cur->_parent;}else//uncle不存在或为黑节点 (旋转){if (cur == parent->_right){RotateL(grandfather);parent->_col = BLACK;grandfather->_col = RED;}else{RotateR(parent);RotateL(grandfather);cur->_col = BLACK;grandfather->_col = RED;}break;//旋转后一定平衡}}}_root->_col = BLACK;//在循环内部不判断root情况,统一处理return true;}//左单旋void RotateL(Node* parent){Node* subR = parent->_right;Node* subRL = subR->_left;parent->_right = subRL;if (subRL)subRL->_parent = parent;subR->_left = parent;Node* ppNode = parent->_parent;parent->_parent = subR;if (parent == _root){_root = subR;subR->_parent = nullptr;}else{if (ppNode->_left == parent)ppNode->_left = subR;elseppNode->_right = subR;subR->_parent = ppNode;}}//右单旋void RotateR(Node* parent){Node* subL = parent->_left;Node* subLR = subL->_right;parent->_left = subLR;if (subLR)subLR->_parent = parent;subL->_right = parent;Node* ppNode = parent->_parent;parent->_parent = subL;if (parent == _root){_root = subL;subL->_parent = nullptr;}else{if (ppNode->_left == parent)ppNode->_left = subL;elseppNode->_right = subL;subL->_parent = ppNode;}}size_t Size(){return _Size(_root);}size_t _Size(Node* root){if (root == nullptr)return 0;;return _Size(root->_left) + _Size(root->_right) + 1;}Node* Find(const K& key){Node* cur = _root;while (cur){if (cur->_kv.first < key){cur = cur->_right;}else if (cur->_kv.first > key){cur = cur->_left;}else{return cur;}}return nullptr;}//中序void InOrder(){_InOrder(_root);cout << "end" << endl;}int Height(){return _Height(_root);}private://中序void _InOrder(Node* root){if (root == nullptr)return;_InOrder(root->_left);cout << root->_kv.first << " - ";_InOrder(root->_right);}//求高度int _Height(Node* root){if (root == nullptr)return 0;return max(Height(root->_left), Height(root->_right)) + 1;}Node* _root = nullptr;
};
应用
- 作为map&set的底层






)



)


协议层(包格式))





文件映射 (File Mapping))