1129. 热浪 - AcWing题库

这道题可以有三种方法来做，朴素版的dijkstra、堆优化版的dijkstra和spfa算法

（1）spfa算法 

 这里的队列用循环队列，而不是像模板那样用普通队列是因为它的队列长度不确定

import java.util.*;public class Main{static int N = 2510, M = 2 * 6200 + 10;static int n, m, S, T, idx;static int[] dist = new int[N];static int[] q = new int[N];static int[] h = new int[N], ne = new int[M], e = new int[M], w = new int[M];//邻接表static boolean[] st = new boolean[N];//邻接表存储public static void add(int a, int b, int c){e[idx] = b;w[idx] = c;ne[idx] = h[a];h[a] = idx ++;}public static void spfa(){Arrays.fill(dist, 0x3f3f3f3f);dist[S] = 0;int hh = 0, tt = 1;//循环队列q[0] = S;st[S] = true;//在队列里面while(hh != tt){//循环队列判断为空的条件int t = q[hh ++];if(hh == N) hh = 0;st[t] = false;//取出了这个元素for(int i = h[t]; i != -1; i = ne[i]){int j = e[i];if(dist[j] > dist[t] + w[i]){dist[j] = dist[t] + w[i];if(!st[j]){q[tt ++] = j;//加入循环队列if(tt == N) tt = 0;st[j] = true;}}}}}public static void main(String[] args){Scanner sc = new Scanner(System.in);n = sc.nextInt();//点m = sc.nextInt();//边S = sc.nextInt();//起点T = sc.nextInt();//终点//建图Arrays.fill(h, -1);//这个一定要记得for(int i = 0; i < m; i ++){int a = sc.nextInt();int b = sc.nextInt();int c = sc.nextInt();add(a, b, c);//无向图add(b, a, c);}spfa();System.out.print(dist[T]);}
}

（2） dijkstra堆优化算法

import java.util.*;class PII implements Comparable<PII>{int distance, num;public PII(int distance, int num){this.distance = distance;this.num = num;}public int compareTo(PII o){return distance - o.distance;}
}public class Main{static int N = 2510, M = 2 * 6200 + 10;static int n, m, S, T, idx;static int[] dist = new int[N];static int[] h = new int[N], ne = new int[M], e = new int[M], w = new int[M];//邻接表static boolean[] st = new boolean[N];//邻接表存储public static void add(int a, int b, int c){e[idx] = b;w[idx] = c;ne[idx] = h[a];h[a] = idx ++;}public static void dijkstra(){PriorityQueue<PII> q = new PriorityQueue<>();//优先队列Arrays.fill(dist, 0x3f3f3f3f);dist[S] = 0;q.offer(new PII(0, S));while(!q.isEmpty()){PII t = q.poll();int distance = t.distance;//到起点的距离int num = t.num;//点的编号if(st[num]) continue;//遍历过了st[num] = true;//标记为遍历过for(int i = h[num]; i != -1; i = ne[i]){int j = e[i];if(dist[j] > distance + w[i]){dist[j] = distance + w[i];if(!st[j]) q.offer(new PII(dist[j], j));}}}}public static void main(String[] args){Scanner sc = new Scanner(System.in);n = sc.nextInt();//点m = sc.nextInt();//边S = sc.nextInt();//起点T = sc.nextInt();//终点//建图Arrays.fill(h, -1);//这个一定要记得for(int i = 0; i < m; i ++){int a = sc.nextInt();int b = sc.nextInt();int c = sc.nextInt();add(a, b, c);//无向图add(b, a, c);}dijkstra();System.out.print(dist[T]);}
}

1128. 信使 - AcWing题库

        这道题是让我们求出指挥部到所有点的最短距离，取一个最大值，如果存在一个点距离指挥部的距离是正无穷的话，就输出-1。 

        这道题虽然是让我们求单源最短路，但是我们也可以用多源最短路的Floyd算法来求。

Floyd算法 

import java.util.*;public class Main{static int N = 110;static int[][] dist = new int[N][N];static int n, m;public static void main(String[] args){Scanner sc = new Scanner(System.in);n = sc.nextInt();m = sc.nextInt();for(int i = 1; i <= n; i ++){for(int j = 1; j <= n; j ++){if(i == j) dist[i][j] = 0;else dist[i][j] = 0x3f3f3f3f;}}for(int i = 1; i <= m; i ++){int a = sc.nextInt();int b = sc.nextInt();int c = sc.nextInt();dist[a][b] = dist[b][a] = Math.min(dist[a][b], c);//防止出现重边}//开始Floy算法for(int k = 1; k <= n; k ++){for(int i = 1; i <= n; i ++){for(int j = 1; j <= n; j ++){dist[i][j] = Math.min(dist[i][j], dist[i][k] + dist[k][j]);}}}//找到最大的那条最短路径int res = 0;for(int i = 1; i <= n; i ++){if(dist[1][i] == 0x3f3f3f3f){res = -1;break;}res = Math.max(res, dist[1][i]);}//输出最大值System.out.print(res);}
}

 

 1127. 香甜的黄油 - AcWing题库

        这道题是本质上是一个多源最短路问题，让我们算出每个点到其他点的距离之和的最小值，但是这道题的数据很大，如果用Floyd算法会超时，所以这里我们可以用spfa算法算出n个点的情况，去一个最小值。

spfa算法 

import java.util.*;public class Main{static int N = 810, M = 3000, INF = 0x3f3f3f3f;static int n, m, p, idx;static int[] id = new int[N];//奶牛所在牧场编号static int[] q = new int[N];//循环队列static int[] h = new int[N], e = new int[M], ne = new int[M], w = new int[M];static boolean[] st = new boolean[N];static int[] dist = new int[N];public static void add(int a, int b, int c){e[idx] = b;w[idx] = c;ne[idx] = h[a];h[a] = idx ++;}public static int spfa(int u){Arrays.fill(dist, INF);dist[u] = 0;int hh = 0, tt = 1;q[0] = u;st[u] = true;while(hh != tt){int t = q[hh ++];if(hh == N) hh = 0;st[t] = false;for(int i = h[t]; i != -1; i = ne[i]){int j = e[i];if(dist[j] > dist[t] + w[i]){dist[j] = dist[t] + w[i];if(!st[j]){q[tt ++] = j;if(tt == N) tt = 0;st[j] = true;}}}}int res = 0;for(int i = 0; i < n; i ++){int j = id[i];//奶牛所在牧场编号if(dist[j] == INF){return INF;} res += dist[j];}return res;}public static void main(String[] args){Scanner sc = new Scanner(System.in);n = sc.nextInt();p = sc.nextInt();m = sc.nextInt();Arrays.fill(h, -1);for(int i = 0; i < n; i ++){id[i] = sc.nextInt();}for(int i = 1; i <= m; i ++){int a = sc.nextInt();int b = sc.nextInt();int c = sc.nextInt();add(a, b, c);//无向图add(b, a, c);}int res = INF;for(int i = 1; i <= p; i ++){//所有奶牛到第i个牧场的距离之和res = Math.min(res, spfa(i));}System.out.print(res);}
}

 

 1126. 最小花费 - AcWing题库

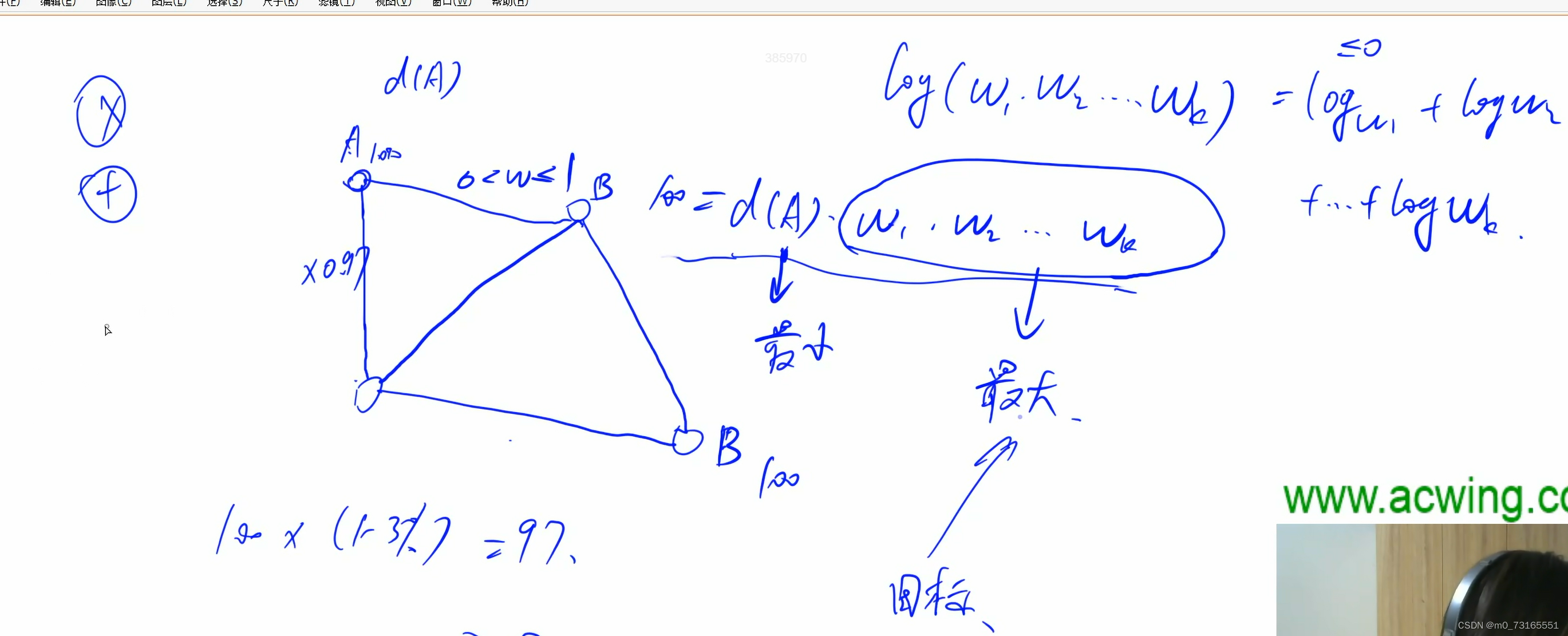
这道题要我们求a的最少钱数，因为有100=da*w1*w2*...(其中这里的w是指1-手续费%，也就是汇率），要先da最小，那么就是说要w的乘积最大 

朴素版dijkstra算法 

import java.util.*;public class Main{static int N = 2010;static int n, m, start, end;static double[][] g = new double[N][N];//邻接矩阵存储边static double[] dist = new double[N];//起点到其他点的距离static boolean[] st = new boolean[N];public static void dijkstra(){//Arrays.fill(dist, 0x3f3f3f3f);我们要求的是dist的最大值，所以这里不需要这句话dist[start] = 1;for(int i = 1; i <= n; i ++){//遍历n次，每次找到一个int t = -1;for(int j = 1; j <= n; j ++){if(!st[j] && (t == -1 || dist[j] > dist[t])){t = j;}}st[t] = true;//标记为遍历过for(int j = 1; j <= n; j ++){dist[j] = Math.max(dist[j], dist[t] * g[t][j]);}}}public static void main(String[] args){Scanner sc = new Scanner(System.in);n = sc.nextInt();m = sc.nextInt();for(int i = 0; i < m; i ++){int a = sc.nextInt();int b = sc.nextInt();int c = sc.nextInt();double z = (100.0 - c) / 100.0;//这里为什么取最大值？因为原本是要求c最小的，而现在的z与c的关系是相反的，所以取最大g[a][b] = g[b][a] = Math.max(g[a][b], z);//防止重边}start = sc.nextInt();end = sc.nextInt();dijkstra();//我们求的dist是w乘积的最大值，如果想要da的最大值，就要用100来除distSystem.out.printf("%.8f", 100.0 / dist[end]);}
}

 

920. 最优乘车 - AcWing题库

换车的最小次数=坐车的最小次数 - 1 

由于这道题所有边的权重是1，所以可以直接用bfs，不需要用dijkstra、spfa什么的

import java.util.*;
import java.io.*;public class Main{static int N = 510;static int n, m, res, INF = 0x3f3f3f3f;static int[] q = new int[N];//用数组来模拟队列static boolean[][] g = new boolean[N][N];//true表示连通，false表示不连通static int[] dist = new int[N];//最小坐车次数static int[] cnt = new int[N];//每条线路经过的巴士站public static void bfs(){Arrays.fill(dist, INF);dist[1] = 0;int hh = 0, tt = -1;q[++ tt] = 1;while(hh <= tt){int t = q[hh ++];//取出队头for(int i = 1; i <= n; i ++){if(g[t][i] && dist[i] > dist[t] + 1){dist[i] = dist[t] + 1;q[++ tt] = i;}}}}public static void main(String[] args)throws IOException{BufferedReader br = new BufferedReader(new InputStreamReader(System.in));String[] s = br.readLine().split(" ");m = Integer.parseInt(s[0]);n = Integer.parseInt(s[1]);for(int i = 0; i < m; i ++){String[] arr = br.readLine().split(" ");int len = arr.length;//这条线路经过多少个车站for(int j = 0; j < len; j ++) cnt[j] = Integer.parseInt(arr[j]);for(int j = 0; j < len; j ++){//这个车站和它后面的车站之间都连通（单程）for(int k = j + 1; k < len; k ++){g[cnt[j]][cnt[k]] = true;//连通置为true}}}bfs();if(dist[n] == INF) System.out.print("NO");else System.out.print(dist[n] - 1);}
}

 

903. 昂贵的聘礼 - AcWing题库

用一个虚拟远点将这些点联系起来，建图 

 

 

import java.util.*;public class Main{static int N = 110;static int n, m;static int[][] g = new int[N][N];//用于建图static int[] dist = new int[N];static boolean[] st = new boolean[N];static int[] level = new int[N];//每个点的等级public static int dijkstra(int down, int up){//因为要枚举多个区间，所以每次开始之前都要进行初始化Arrays.fill(st, false);Arrays.fill(dist, 0x3f3f3f3f);dist[0] = 0;//虚拟远点的距离置为0//朴素版的dijkstra，因为建立了一个虚拟远点，所以循环的时候要从0开始for(int i = 0; i <= n; i ++){int t = -1;for(int j = 0; j <= n; j ++){if(!st[j] && (t == -1 || dist[t] > dist[j])){t = j;}}st[t] = true;//更新其他点的值for(int j = 0; j <= n; j ++){if(level[j] >= down && level[j] <= up){dist[j] = Math.min(dist[j], dist[t] + g[t][j]);}}}return dist[1];}public static void main(String[] args){Scanner sc = new Scanner(System.in);m = sc.nextInt();n = sc.nextInt();//初始化for(int i = 0; i < N; i ++){Arrays.fill(g[i], 0x3f3f3f3f);}for(int i = 0; i < N; i ++){g[i][i] = 0;}//建图for(int i = 1; i <= n; i ++){int price = sc.nextInt();level[i] = sc.nextInt();int cnt = sc.nextInt();//有几种兑换方式g[0][i] = price;//虚拟远点到当前点的距离（不兑换，直接购买）for(int j = 1; j <= cnt; j ++){int id = sc.nextInt();int cost = sc.nextInt();g[id][i] = cost;//兑换物品到当前点的距离}}int res = 0x3f3f3f3f;//枚举等级的左端点，找到最小的等级，因为我们一定要将第一个点包含在内for(int i = level[1] - m; i <= level[1]; i ++) res = Math.min(res, dijkstra(i, i + m));System.out.print(res);}
}