matlab动画_弹簧振子振动的matlab动画演示

用Matlab阐述物理中的胡克定律，为弹簧振子震动的matlab动画示意图，另有一个为不同质量不同弹簧系数的比较。spring.m,compare.m

rectangle('position',[12,8.5,2,0.3],'FaceColor',[0.5,0.3,0.4]);
axis([0,15,-1,10]); 
hold on  
plot([13,13],[7,8.5],'r','linewidth',2); y=2:.2:7; 
M=length(y);
x=12+mod(1:M,2)*2;
x(1)=13; 
x(end-3:end)=13;
D=plot(x,y);   C=0:.1:2*pi;
r=0.35; 
t1=r*sin(C);
F1=fill(13+r*cos(C),2+t1,'r'); set(gca,'ytick',[0:2:9]); 
set(gca,'yticklabels',num2str([-1:3]')); 
plot([0,15],[3.3,3.3],'black');
H1=plot([0,13],[3.3,3.3],'y');
Q=plot(0,3.8,'color','r'); td=[];
yd=[]; 
T=0; 
text(2,9,'F=-kx','fontsize',16);
set(gcf,'doublebuffer','on'); 
while T<12; pause(0.2); Dy=(3/2-1/2*sin(pi*T))*1/2;Y=-(y-2)*Dy+7; Yf=Y(end)+t1; td=[td,T];yd=[yd,Y(end)];set(D,'ydata',Y);set(F1,'ydata',Yf,'facecolor',rand(1,3));set(H1,'xdata',[T,13],'ydata',[Y(end),Y(end)]); set(Q,'xdata',td,'ydata',yd) ; T=T+0.1;drawnow;
end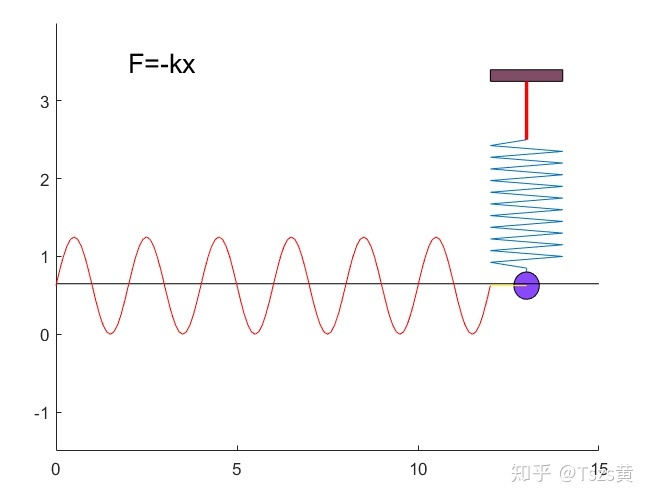

rectangle('position',[9,8.5,2,0.3],'FaceColor',[0.1,0.3,0.4]);
axis([0,15,-1,10]); 
hold on  
plot([10,10],[7,8.5],'r','linewidth',2); y=4:.13:9; 
M=length(y);
x=9+mod(1:M,2)*2;
x(1)=10; 
x(end-6:end)=10;
D=plot(x,y);   C=0:.1:2*pi;
r=0.5; 
t1=r*sin(C);
F1=fill(10+r*cos(C),2+t1,'r'); set(gca,'ytick',[0:2:9]); 
set(gca,'yticklabels',num2str([-1:3]')); 
plot([0,12],[3.3,3.3],'black');
H1=plot([0,10],[3.3,3.3],'b');
w=plot(0,3.8,'color','g'); td=[];
yd=[]; 
T=0; 
text(2,9,'F=-kx','fontsize',16);
set(gcf,'doublebuffer','on'); 
while T<12; pause(0.2); Dy=(1/2-1/3*sin(pi*T))*3/2;Y=-(y-4)*Dy+7; Yf=Y(end)+t1; td=[td,T];yd=[yd,Y(end)];set(D,'ydata',Y);set(F1,'ydata',Yf,'Facecolor',rand(1,3));set(H1,'xdata',[T,10],'ydata',[Y(end),Y(end)]); set(w,'xdata',td,'ydata',yd) ; T=T+0.1;drawnow;
end