如果信号x(t)是周期信号,那么对于所有t,存在一个最小正数T,使得x(t)=x(t+T)其中T为这个周期信号的最小正周期。根据周期函数的周期性:x(t)=x(t+N*T)(N为整数)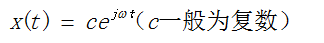 则
则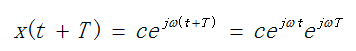 因为x(t)是周期信号,那么
因为x(t)是周期信号,那么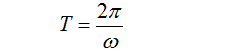 那么周期信号T必须满足倍数关系,也就是N*2π倍数关系(N为整数),而不能是非2π倍数关系。对于复指数信号的最小正周期为T,同时对应的基波频率w0,然而这个复指数信号可能含有其他谐波频率的复指数,而这些复指数是以T为基准周期的,实际上比最小正周期T小。而谐波复指数信号的周期必须是最小正周期的T\K,(其中K为非零整数),复指数信号才可能含有谐波复指数信号。例如:
那么周期信号T必须满足倍数关系,也就是N*2π倍数关系(N为整数),而不能是非2π倍数关系。对于复指数信号的最小正周期为T,同时对应的基波频率w0,然而这个复指数信号可能含有其他谐波频率的复指数,而这些复指数是以T为基准周期的,实际上比最小正周期T小。而谐波复指数信号的周期必须是最小正周期的T\K,(其中K为非零整数),复指数信号才可能含有谐波复指数信号。例如: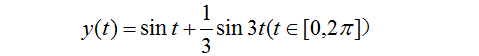 这个周期函数最小正周期2π,但含有3次谐波的函数,3次谐波周期为基波周期的2π\3,因此这个信号中不仅有基波信号,还有三次谐波信号。
这个周期函数最小正周期2π,但含有3次谐波的函数,3次谐波周期为基波周期的2π\3,因此这个信号中不仅有基波信号,还有三次谐波信号。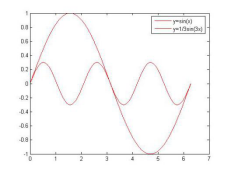 在一个最小正周期内,只有最小正周期是谐波周期的整数倍时,两者波形的起点和终点才能重合。周期信号x(t)是以T为最小正周期的,与x(t)有关的周期谐波复指数信号集合可以表示如下:
在一个最小正周期内,只有最小正周期是谐波周期的整数倍时,两者波形的起点和终点才能重合。周期信号x(t)是以T为最小正周期的,与x(t)有关的周期谐波复指数信号集合可以表示如下: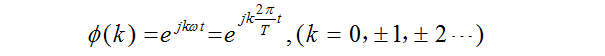 那么对于一个周期为T0的信号就可用周期复指数谐波信号叠加而成。
那么对于一个周期为T0的信号就可用周期复指数谐波信号叠加而成。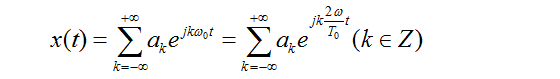 上式的表达式就是傅里叶级数,其中ak代表周期复指数谐波信号的振幅分量,又称傅里叶系数或者频谱系数,w0代表信号的基波频率,kw0代表谐波频率,当K=0时为常数,当K=1时为基波频率,当K=2时为2次谐波。以周期为T0的信号可以用复指数谐波信号叠加而成,那么应该如何求解傅里叶系数呢?
上式的表达式就是傅里叶级数,其中ak代表周期复指数谐波信号的振幅分量,又称傅里叶系数或者频谱系数,w0代表信号的基波频率,kw0代表谐波频率,当K=0时为常数,当K=1时为基波频率,当K=2时为2次谐波。以周期为T0的信号可以用复指数谐波信号叠加而成,那么应该如何求解傅里叶系数呢?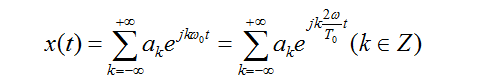 方程两边同时乘以-jnwt,两边同时积分。
方程两边同时乘以-jnwt,两边同时积分。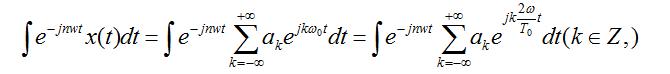
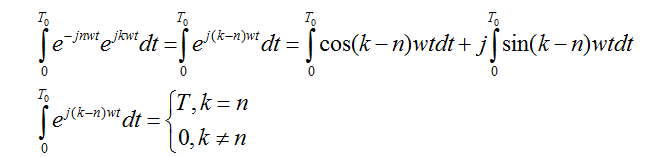
 如果周期信号为实信号,傅里叶系数存在以下关系ak*=a-k(ak的共轭复数等于a-k)证明:如果x(t)是实周期函数,那么有x(t)*=x(t)(共轭关系)
如果周期信号为实信号,傅里叶系数存在以下关系ak*=a-k(ak的共轭复数等于a-k)证明:如果x(t)是实周期函数,那么有x(t)*=x(t)(共轭关系)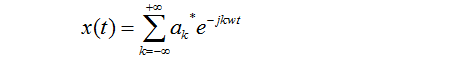 令k=-k,目的是调换位置,使得复指数项从负到正。
令k=-k,目的是调换位置,使得复指数项从负到正。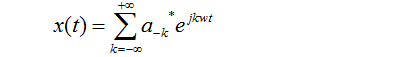 因为
因为 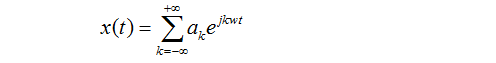 比较两式得:
比较两式得: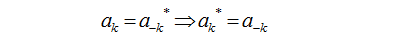 从而ak的共轭等于a-k。例题1:x(t)是以基波频率为2π的周期信号,x(t)写成以下形式。
从而ak的共轭等于a-k。例题1:x(t)是以基波频率为2π的周期信号,x(t)写成以下形式。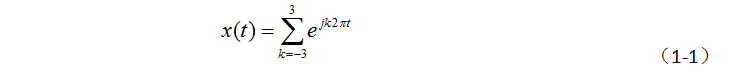 其中,a0=1,,a1=a-1=1/4,a2=a-2=1/2,a3=a-3=1/3,求其傅里叶展开式解:将(1-1)式中具有同一谐波频率的谐波分量合在一起,重新组合得
其中,a0=1,,a1=a-1=1/4,a2=a-2=1/2,a3=a-3=1/3,求其傅里叶展开式解:将(1-1)式中具有同一谐波频率的谐波分量合在一起,重新组合得 例题2:x(t)是sint,基波频率为2π的实信号,求其傅里叶展开式。由欧拉公式得:
例题2:x(t)是sint,基波频率为2π的实信号,求其傅里叶展开式。由欧拉公式得: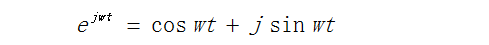 则
则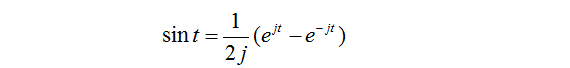 则k1=1/2j,k-1=-1/2j,ak=0(k≠±1)例题3,在电工学中,函数信号发生器可以产生方波,方波的幅值和相角可以调整,为了方便,设定原点为方波的对称点,幅值为1,最小正周期为2,波形以下展示。
则k1=1/2j,k-1=-1/2j,ak=0(k≠±1)例题3,在电工学中,函数信号发生器可以产生方波,方波的幅值和相角可以调整,为了方便,设定原点为方波的对称点,幅值为1,最小正周期为2,波形以下展示。
 f(t)的周期为2,频率为π,f(t)用傅里叶级数展开
f(t)的周期为2,频率为π,f(t)用傅里叶级数展开 通过推导
通过推导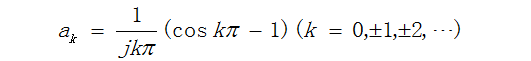 联立两个方程:用三角级数表示
联立两个方程:用三角级数表示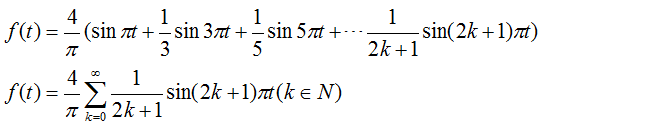 取k=17,自变量t为2π,Matlab代码如下:>> clear all>> x=0:0.001:2*pi;>> y=4/pi*(sin(pi*x)+1/3*sin(3*pi*x)+1/5*sin(5*pi*x)+1/7*sin(7*pi*x)+1/9*sin(9*pi*x)+1/11*sin(11*pi*x)+1/13*sin(13*pi*x)+1/15*sin(15*pi*x)+1/17*sin(17*pi*x));>> plot(x,y,'b')输出二维图形如下:
取k=17,自变量t为2π,Matlab代码如下:>> clear all>> x=0:0.001:2*pi;>> y=4/pi*(sin(pi*x)+1/3*sin(3*pi*x)+1/5*sin(5*pi*x)+1/7*sin(7*pi*x)+1/9*sin(9*pi*x)+1/11*sin(11*pi*x)+1/13*sin(13*pi*x)+1/15*sin(15*pi*x)+1/17*sin(17*pi*x));>> plot(x,y,'b')输出二维图形如下:
 f(t)的周期为2,基波频率为π,f(t)用傅里叶级数展开
f(t)的周期为2,基波频率为π,f(t)用傅里叶级数展开 通过推导
通过推导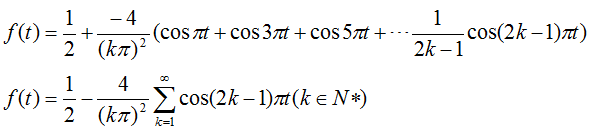 也可以用Matlab进行傅里叶展开验证,取变量t为2π,k=11时,如下图所示。代码如下:>> clear all>> x=0:0.001:2*pi;>> y=0.5-4*(1/(pi*pi)*cos(pi*x)+1/(9*pi*pi)*cos(3*pi*x)+1/(25*pi*pi)*cos(5*pi*x)+1/(49*pi*pi)*cos(7*pi*x)+1/(81*pi*pi)*cos(9*pi*x)+1/(121*pi*pi)*cos(11*pi*x)+1/(169*pi*pi)*cos(13*pi*x));>> plot(x,y,'r')输出二维图形如下:
也可以用Matlab进行傅里叶展开验证,取变量t为2π,k=11时,如下图所示。代码如下:>> clear all>> x=0:0.001:2*pi;>> y=0.5-4*(1/(pi*pi)*cos(pi*x)+1/(9*pi*pi)*cos(3*pi*x)+1/(25*pi*pi)*cos(5*pi*x)+1/(49*pi*pi)*cos(7*pi*x)+1/(81*pi*pi)*cos(9*pi*x)+1/(121*pi*pi)*cos(11*pi*x)+1/(169*pi*pi)*cos(13*pi*x));>> plot(x,y,'r')输出二维图形如下:
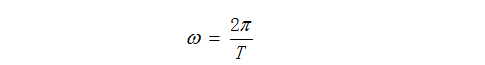
称为这个信号的基波频率
周期信号x(t)也可以用周期复指数信号表示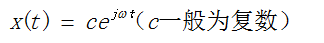 则
则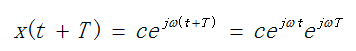 因为x(t)是周期信号,那么
因为x(t)是周期信号,那么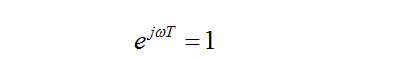
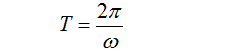 那么周期信号T必须满足倍数关系,也就是N*2π倍数关系(N为整数),而不能是非2π倍数关系。对于复指数信号的最小正周期为T,同时对应的基波频率w0,然而这个复指数信号可能含有其他谐波频率的复指数,而这些复指数是以T为基准周期的,实际上比最小正周期T小。而谐波复指数信号的周期必须是最小正周期的T\K,(其中K为非零整数),复指数信号才可能含有谐波复指数信号。例如:
那么周期信号T必须满足倍数关系,也就是N*2π倍数关系(N为整数),而不能是非2π倍数关系。对于复指数信号的最小正周期为T,同时对应的基波频率w0,然而这个复指数信号可能含有其他谐波频率的复指数,而这些复指数是以T为基准周期的,实际上比最小正周期T小。而谐波复指数信号的周期必须是最小正周期的T\K,(其中K为非零整数),复指数信号才可能含有谐波复指数信号。例如: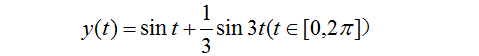 这个周期函数最小正周期2π,但含有3次谐波的函数,3次谐波周期为基波周期的2π\3,因此这个信号中不仅有基波信号,还有三次谐波信号。
这个周期函数最小正周期2π,但含有3次谐波的函数,3次谐波周期为基波周期的2π\3,因此这个信号中不仅有基波信号,还有三次谐波信号。 在一个最小正周期内,只有最小正周期是谐波周期的整数倍时,两者波形的起点和终点才能重合。周期信号x(t)是以T为最小正周期的,与x(t)有关的周期谐波复指数信号集合可以表示如下:
在一个最小正周期内,只有最小正周期是谐波周期的整数倍时,两者波形的起点和终点才能重合。周期信号x(t)是以T为最小正周期的,与x(t)有关的周期谐波复指数信号集合可以表示如下: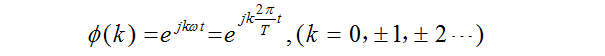 那么对于一个周期为T0的信号就可用周期复指数谐波信号叠加而成。
那么对于一个周期为T0的信号就可用周期复指数谐波信号叠加而成。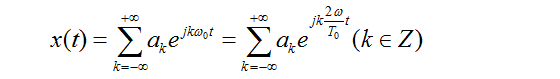 上式的表达式就是傅里叶级数,其中ak代表周期复指数谐波信号的振幅分量,又称傅里叶系数或者频谱系数,w0代表信号的基波频率,kw0代表谐波频率,当K=0时为常数,当K=1时为基波频率,当K=2时为2次谐波。以周期为T0的信号可以用复指数谐波信号叠加而成,那么应该如何求解傅里叶系数呢?
上式的表达式就是傅里叶级数,其中ak代表周期复指数谐波信号的振幅分量,又称傅里叶系数或者频谱系数,w0代表信号的基波频率,kw0代表谐波频率,当K=0时为常数,当K=1时为基波频率,当K=2时为2次谐波。以周期为T0的信号可以用复指数谐波信号叠加而成,那么应该如何求解傅里叶系数呢?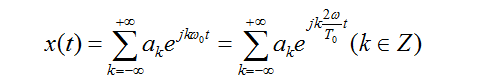 方程两边同时乘以-jnwt,两边同时积分。
方程两边同时乘以-jnwt,两边同时积分。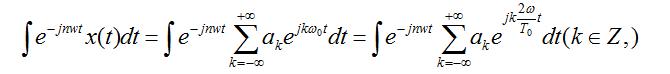
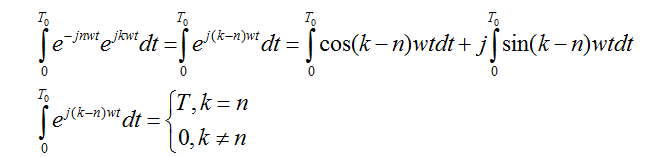
 如果周期信号为实信号,傅里叶系数存在以下关系ak*=a-k(ak的共轭复数等于a-k)证明:如果x(t)是实周期函数,那么有x(t)*=x(t)(共轭关系)
如果周期信号为实信号,傅里叶系数存在以下关系ak*=a-k(ak的共轭复数等于a-k)证明:如果x(t)是实周期函数,那么有x(t)*=x(t)(共轭关系)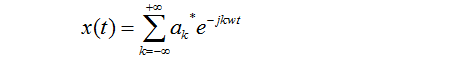 令k=-k,目的是调换位置,使得复指数项从负到正。
令k=-k,目的是调换位置,使得复指数项从负到正。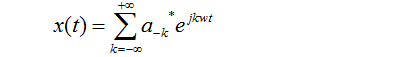 因为
因为 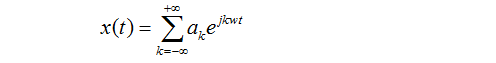 比较两式得:
比较两式得: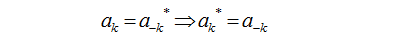 从而ak的共轭等于a-k。例题1:x(t)是以基波频率为2π的周期信号,x(t)写成以下形式。
从而ak的共轭等于a-k。例题1:x(t)是以基波频率为2π的周期信号,x(t)写成以下形式。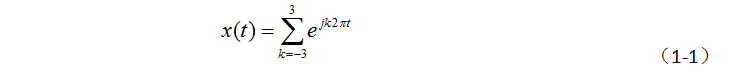 其中,a0=1,,a1=a-1=1/4,a2=a-2=1/2,a3=a-3=1/3,求其傅里叶展开式解:将(1-1)式中具有同一谐波频率的谐波分量合在一起,重新组合得
其中,a0=1,,a1=a-1=1/4,a2=a-2=1/2,a3=a-3=1/3,求其傅里叶展开式解:将(1-1)式中具有同一谐波频率的谐波分量合在一起,重新组合得 例题2:x(t)是sint,基波频率为2π的实信号,求其傅里叶展开式。由欧拉公式得:
例题2:x(t)是sint,基波频率为2π的实信号,求其傅里叶展开式。由欧拉公式得: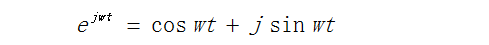 则
则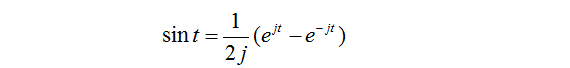 则k1=1/2j,k-1=-1/2j,ak=0(k≠±1)例题3,在电工学中,函数信号发生器可以产生方波,方波的幅值和相角可以调整,为了方便,设定原点为方波的对称点,幅值为1,最小正周期为2,波形以下展示。
则k1=1/2j,k-1=-1/2j,ak=0(k≠±1)例题3,在电工学中,函数信号发生器可以产生方波,方波的幅值和相角可以调整,为了方便,设定原点为方波的对称点,幅值为1,最小正周期为2,波形以下展示。
 f(t)的周期为2,频率为π,f(t)用傅里叶级数展开
f(t)的周期为2,频率为π,f(t)用傅里叶级数展开 通过推导
通过推导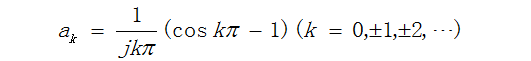 联立两个方程:用三角级数表示
联立两个方程:用三角级数表示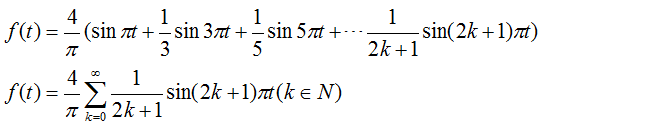 取k=17,自变量t为2π,Matlab代码如下:>> clear all>> x=0:0.001:2*pi;>> y=4/pi*(sin(pi*x)+1/3*sin(3*pi*x)+1/5*sin(5*pi*x)+1/7*sin(7*pi*x)+1/9*sin(9*pi*x)+1/11*sin(11*pi*x)+1/13*sin(13*pi*x)+1/15*sin(15*pi*x)+1/17*sin(17*pi*x));>> plot(x,y,'b')输出二维图形如下:
取k=17,自变量t为2π,Matlab代码如下:>> clear all>> x=0:0.001:2*pi;>> y=4/pi*(sin(pi*x)+1/3*sin(3*pi*x)+1/5*sin(5*pi*x)+1/7*sin(7*pi*x)+1/9*sin(9*pi*x)+1/11*sin(11*pi*x)+1/13*sin(13*pi*x)+1/15*sin(15*pi*x)+1/17*sin(17*pi*x));>> plot(x,y,'b')输出二维图形如下:

 f(t)的周期为2,基波频率为π,f(t)用傅里叶级数展开
f(t)的周期为2,基波频率为π,f(t)用傅里叶级数展开 通过推导
通过推导
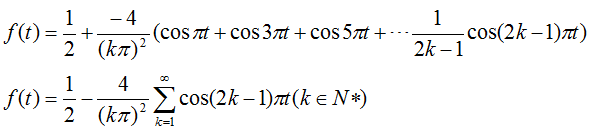 也可以用Matlab进行傅里叶展开验证,取变量t为2π,k=11时,如下图所示。代码如下:>> clear all>> x=0:0.001:2*pi;>> y=0.5-4*(1/(pi*pi)*cos(pi*x)+1/(9*pi*pi)*cos(3*pi*x)+1/(25*pi*pi)*cos(5*pi*x)+1/(49*pi*pi)*cos(7*pi*x)+1/(81*pi*pi)*cos(9*pi*x)+1/(121*pi*pi)*cos(11*pi*x)+1/(169*pi*pi)*cos(13*pi*x));>> plot(x,y,'r')输出二维图形如下:
也可以用Matlab进行傅里叶展开验证,取变量t为2π,k=11时,如下图所示。代码如下:>> clear all>> x=0:0.001:2*pi;>> y=0.5-4*(1/(pi*pi)*cos(pi*x)+1/(9*pi*pi)*cos(3*pi*x)+1/(25*pi*pi)*cos(5*pi*x)+1/(49*pi*pi)*cos(7*pi*x)+1/(81*pi*pi)*cos(9*pi*x)+1/(121*pi*pi)*cos(11*pi*x)+1/(169*pi*pi)*cos(13*pi*x));>> plot(x,y,'r')输出二维图形如下:
参考内容
[1]奥本海姆.信号与系统[M],第二版,北京:电子工业出版社,2013.
[2]王晶,翁国庆.张有兵.电力系统的MATLAB/SIMMULINK仿真与应用[M],西安:西安电子科技大学出版社,2008.








)



搭建及使用)









