Floyd Warshall算法

Description:

描述：

This is a very popular interview problem to find all pair shortest paths in any graph. This problem has been featured in interview rounds of Samsung.

这是一个非常流行的面试问题，用于在任何图中找到所有对最短路径。该问题已在三星的采访回合中提到。

Problem statement:

问题陈述：

Given a weighted directed graph, the problem is to find the shortest distances between every pair of vertices. The Graph is represented by an adjacency matrix, and any cell arr[i][j] denotes the weight of the edge (path cost) from node i to node j (if it exists) else INF.

给定一个加权有向图，问题在于找到每对顶点之间的最短距离。该图由邻接矩阵表示，任何单元格arr [i] [j]表示从节点i到节点j (如果存在)或其他INF的边的权重(路径成本)。

Input: N=5

输入： N = 5

Adjacency matrix:

邻接矩阵：

Example:

例：

So the graph for the above input is,

因此，上述输入的图形为

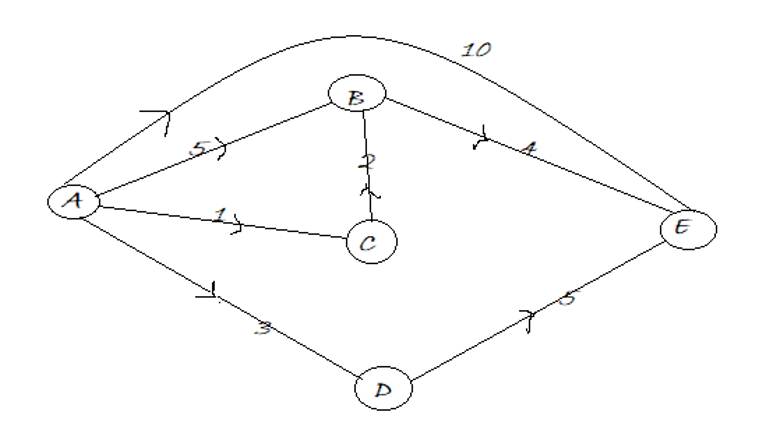
Figure 1: Directed Graph for which all pair shortest distance path needed to be found

图1：有向图，需要找到所有对的最短距离路径

    A → B: 5
A → C: 1
A → D: 3
A → E: 10
B → A: INF
B → C: INF
B → D: INF
B → E: 4
C → A: INF
C → B: 2
C → D: INF
C → E: INF
D → A: INF
D → B: INF
D → C: INF
D → E: 5
E → A: INF
E → B: INF
E → C: INF
E → D: INF

Problem solution:

问题方案：

The Floyd Warshall algorithm computes the all pair shortest path in any weighted graph from the adjacency matrix. It also works for negative weight edges.

Floyd Warshall算法根据邻接矩阵计算任何加权图中的所有对最短路径。它也适用于负重量边缘。

The algorithm is very simple to compute. Basically to compute the shortest path between i^th node to j^th node we check whether there is an intermediate node that reduces the distance, i.e., the path cost.

该算法非常容易计算。基本上，为了计算第i ^个节点到^第 j ^个节点之间的最短路径，我们检查是否存在缩短距离的中间节点，即路径成本。

Let,

让，

    D(i,j) = Distance from i^th node to i^th node

We check for whether there is any intermediate node, say v such that,

我们检查是否存在中间节点，例如v，

    D(i,u) + D(u,j) < D(i,j), for any intermidiate node u,uЄ[1,n]and u≠i,u≠j

Initially we consider the adjacency matrix to be the shortest distance table.

最初，我们认为邻接矩阵是最短距离表。

Based on this concept, the Floyd-Warshall algorithm is designed.

基于此概念，设计了Floyd-Warshall算法。

So, initially the shortest distance table is,

因此，最短距离表最初是

After updating the shortest distance from A to other nodes,

更新了从A到其他节点的最短距离后，

So on.

等等。

C++ implementation:

C ++实现：

#include <bits/stdc++.h>
using namespace std;
void FloydWarshal(long long int** arr, int n)
{
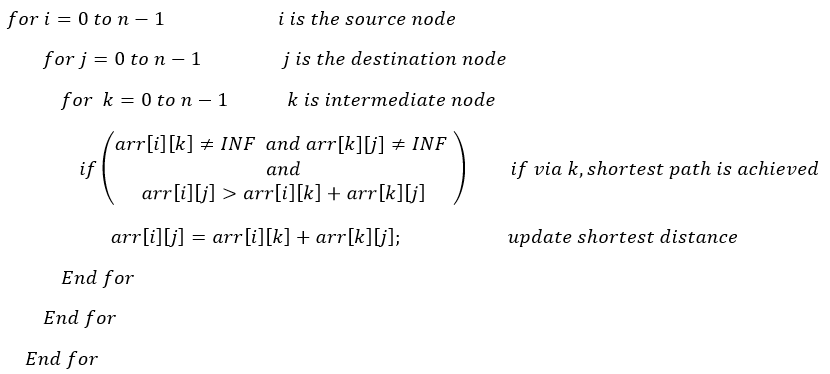
for (int i = 0; i < n; i++) { //source node
for (int j = 0; j < n; j++) { //destination node
for (int k = 0; k < n; k++) { //intermediate node
// if shortest path via the intermediate node exists
if (arr[i][k] != INT_MAX && arr[k][j] != INT_MAX && arr[i][j] > arr[i][k] + arr[k][j])
arr[i][j] = arr[i][k] + arr[k][j]; //update shortest distance
}
}
}
cout << "Printing all pair shortest path distance\n";
for (int i = 0; i < n; i++) {
for (int j = 0; j < n; j++) {
cout << char(i + 'A') << "->" << char(j + 'A') << ":";
if (arr[i][j] == INT_MAX)
cout << "INF"
<< "\n";
else
cout << arr[i][j] << "\n";
}
}
}
int main()
{
int n;
string item;
cout << "Number of node in the graph is:\n";
cin >> n;
long long int** arr = (long long int**)(malloc(sizeof(long long int*) * n));
cout << "Enter the weights\n";
//build the adjacency matrix
for (int j = 0; j < n; j++) {
arr[j] = (long long int*)(malloc(sizeof(long long int) * n));
for (int k = 0; k < n; k++) {
cout << "Enter weight of " << char(j + 'A') << " -> " << char(k + 'A') << endl;
cin >> item;
if (item == "INF")
arr[j][k] = INT_MAX;
else
arr[j][k] = stoi(item);
}
}
// function to compute all pair shortest distance
FloydWarshal(arr, n);
return 0;
}

Output

输出量

Number of node in the graph is:
5
Enter the weights
Enter weight of A -> A
0
Enter weight of A -> B
5
Enter weight of A -> C
1
Enter weight of A -> D
3
Enter weight of A -> E
10
Enter weight of B -> A
INF
Enter weight of B -> B
0
Enter weight of B -> C
INF
Enter weight of B -> D
INF
Enter weight of B -> E
4
Enter weight of C -> A
INF
Enter weight of C -> B
2
Enter weight of C -> C
0
Enter weight of C -> D
INF
Enter weight of C -> E
INF
Enter weight of D -> A
INF
Enter weight of D -> B
INF
Enter weight of D -> C
INF
Enter weight of D -> D
0
Enter weight of D -> E
5
Enter weight of E -> A
INF
Enter weight of E -> B
INF
Enter weight of E -> C
INF
Enter weight of E -> D
INF
Enter weight of E -> E
0
Printing all pair shortest path distance
A->A:0
A->B:3
A->C:1
A->D:3
A->E:7
B->A:INF
B->B:0
B->C:INF
B->D:INF
B->E:4
C->A:INF
C->B:2
C->C:0
C->D:INF
C->E:6
D->A:INF
D->B:INF
D->C:INF
D->D:0
D->E:5
E->A:INF
E->B:INF
E->C:INF
E->D:INF
E->E:0