LeetCode 2280. 表示一个折线图的最少线段数（几何）

文章目录

- 1. 题目
- 2. 解题

1. 题目

给你一个二维整数数组 stockPrices ，其中 stockPrices[i] = [dayi, pricei] 表示股票在 dayi 的价格为 pricei 。
折线图 是一个二维平面上的若干个点组成的图，横坐标表示日期，纵坐标表示价格，折线图由相邻的点连接而成。
比方说下图是一个例子：
在这里插入图片描述

请你返回要表示一个折线图所需要的 最少线段数 。

示例 1：

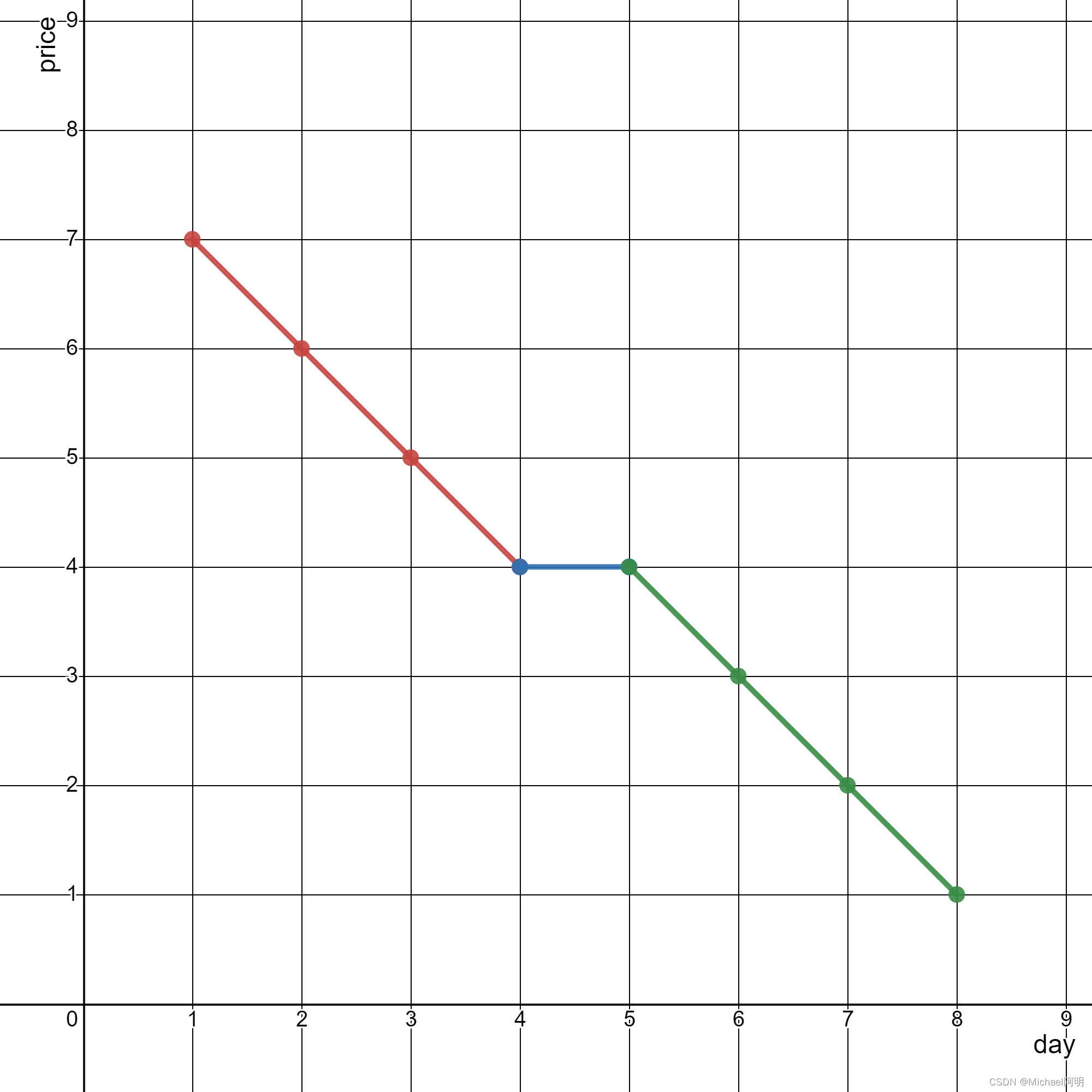
在这里插入图片描述

输入：stockPrices = [[1,7],[2,6],[3,5],[4,4],
[5,4],[6,3],[7,2],[8,1]]
输出：3
解释：
上图为输入对应的图，横坐标表示日期，纵坐标表示价格。
以下 3 个线段可以表示折线图：
- 线段 1 （红色）从 (1,7) 到 (4,4) ，经过 (1,7) ，(2,6) ，(3,5) 和 (4,4) 。
- 线段 2 （蓝色）从 (4,4) 到 (5,4) 。
- 线段 3 （绿色）从 (5,4) 到 (8,1) ，经过 (5,4) ，(6,3) ，(7,2) 和 (8,1) 。
可以证明，无法用少于 3 条线段表示这个折线图。

示例 2：
在这里插入图片描述

输入：stockPrices = [[3,4],[1,2],[7,8],[2,3]]
输出：1
解释：
如上图所示，折线图可以用一条线段表示。提示：
1 <= stockPrices.length <= 10^5
stockPrices[i].length == 2
1 <= dayi, pricei <= 10^9
所有 dayi 互不相同 。

来源：力扣（LeetCode）
链接：https://leetcode.cn/problems/minimum-lines-to-represent-a-line-chart
著作权归领扣网络所有。商业转载请联系官方授权，非商业转载请注明出处。

2. 解题

排序，按横坐标排序
计算每相邻的3个点之间的斜率是否一样
$y2−y1x2−x1=y1−y0x1−x0⇒dy2∗dx1=dy1∗dx2\frac{y_2-y_1}{x_2-x_1} = \frac{y_1-y_0}{x_1-x_0} \Rightarrow dy_2*dx_1 = dy_1*dx_2$

class Solution:def minimumLines(self, stockPrices: List[List[int]]) -> int:n = len(stockPrices)if n <= 2:return n-1ans = 1stockPrices.sort()for i in range(2, n):dy0, dx0 = stockPrices[i][1]-stockPrices[i-1][1], stockPrices[i][0]-stockPrices[i-1][0]dy1, dx1 = stockPrices[i-1][1]-stockPrices[i-2][1], stockPrices[i-1][0]-stockPrices[i-2][0]if dy0*dx1 != dy1*dx0:ans += 1return ans