二叉树节点结构体
class Node {
public:int val;Node* left;Node* right;Node(int a) : val(a), left(nullptr), right(nullptr) {}Node(int a, Node* l, Node* r): val(a), left(l), right(r) {}}
1、递归遍历
递归遍历二叉树,每个节点的遍历顺序叫递归序(视频讲解链接)
递归过程中,树的每个节点都会被访问3次。聚焦到某一个节点。第一次访问该结点后,执行一段代码,再递归执行左子树。左子树递归完毕,会再一次回到该节点,继续往下执行一段代码后,跳到右子树递归执行。右子树递归完毕,又再次回到该节点,执行剩下的代码。
void f(Node* head) {if (!head) return;// ......第一次到该节点后,执行的代码(节点访问操作,比如打印值)f(head->left);// ......第二次到该节点后,执行的代码f(head->right);// ......第三次到该节点后,执行的代码
}
利用递归序,可以很容易得到3种遍历顺序
- 先序遍历(根左右):第1次到一个节点才打印
- 中序遍历(左根右):第2次到一个节点才打印
- 后序遍历(左右根):第3次到一个节点才打印
void preOrderRecur(Node* head) {if (!head) return;std::cout << head->val << " ";preOrderRecur(head->left);preOrderRecur(head->right);
}
void inOrderRecur(Node* head) {if (!head) return;inOrderRecur(head->left);std::cout << head->val << " ";inOrderRecur(head->right);
}
void posOrderRecur(Node* head) {if (!head) return;posOrderRecur(head->left); posOrderRecur(head->right);std::cout << head->val << " ";
}

2、非递归遍历
非递归遍历二叉树是通过使用辅助数据结构(通常是栈)来模拟遍历过程,从而避免了递归带来的函数调用开销。
2.1 先序遍历(深度优先遍历)(单栈)
leetcode 144 简单
先将根节点压入栈中,然后循环执行以下步骤:
- 弹出并访问
- 先右后左,把两个孩子入栈(如果有)
先右后左压栈,出栈就是先左后右了
#include <stack>
#include <iostream>
void preOrderUnRecur(Node* head) {std::cout << "pre-order: ";if (head) {std::stack<Node*> myStack;// 先压入头结点myStack.push(head);// 循环执行while (!myStack.empty()) {// 弹出并访问head = myStack.top();std::cout << head->val << " ";myStack.pop();// 先右后左if (head->right)myStack.push(head->left);if (head->left)myStack.push(head->right);}}std::cout << endl;
}
2.2 后序遍历(双栈)
leetcode 145 简单
后序遍历与前序遍历很像,相当于逆序一下(需要额外一个栈)。
先序是根左右 ,如果弹出时不访问,而是放入另一个收集栈,则每个子树在收集栈中从上到下就会是右左根的顺序,而我们需要左右根,因此在压入第一个栈时先左后右即可。
先将根节点压入第一个栈,然后循环执行以下步骤:
- 弹出并压入第二个栈(收集栈)
- 其子节点入栈,先左后右(如果有)
最终,第二个栈中的元素弹出顺序即为后序遍历的结果。
#include <stack>
#include <iostream>
void posOrderUnRecur(Node* head) {std::cout << "in-order: ";if (!head) return;std::stack<Node*> s1;std::stack<Node*> s2;// 压入根节点s1.push(head);// 循环while (!s1.empty()) {// 弹出并压入收集栈head = s1.top();s1.pop();s2.push(head);// 先左后右if (head->left)s1.push(head->left);if (head->left)s1.push(head->right);}// 单独打印收集栈while (!s2.empty()) {std::cout << s2.top() << " ";s2.pop();}std::cout << endl;
}
2.3 中序遍历(单栈)
leetcode 94 简单
循环执行以下步骤:
- 对每棵子树的左边界节点按从上到下的顺序压入栈中
- 弹出栈顶节点并访问(打印)
- 对其右子树重复以上操作
稍微麻烦一些,死记咯。其详细过程看视频链接
#include <stack>
#include <iostream>
void inOrderUnRecur(Node* head) {std::cout << "in-order :";if (head) {std::stack<Node*> s;while (!s.empty() || head) {if (head) { // head复用了,最左边节点全部入栈stack.push(head);head = head->left;} else { // 直到head为空,左边节点已全部入栈// 出栈并访问栈顶节点head = s.top(); std::cout << head->val << " ";s.pop()// 下一步对右子树重复以上循环head = head->right }}}std::cout << std::endl;
}
看完代码后,看这个视频链接非常有助于理解记忆这个过程。
这个过程,其实就是把整棵树,用左边界进行分解,每个左边界入栈顺序是从根节点到叶子结点,那么弹出顺序必然是叶子结点到根节点,即符合左根,然后每次一个根节点出栈后,会立马对它右子树重复以上过程,从而实现对该树而言的根右。综上所述每一课子树局部都是左根右的顺序,任何一个整体树叶依旧是左根右。满足中序遍历要求
2.3 宽度优先遍历(层序遍历)(单队列)
leetcode 102 中等
思路简单,借用一个队列
先把根节点入队,然后循环执行
- 出队并访问
- 其子节点入队,先左后右(如果有)
#include <queue>
#include <iostream>void levelOrder(Node* head) {if (!head) return;std::queue<Node*> q;q.push(head);while (!queue.empty()) {head = q.front();std::cout << head ->val << " ";q.pop();if (head->left)q.push(head->left);if (head->right)q.push(head->right);}
}
2.3.1 例题:求二叉树最大宽度
leetcode 662 中等
不光是层序遍历,还要统计当前在第几层以及该层的节点数
代码讲解视频链接
#include <limits>
#include <queue>
#include <unordered_map>int treeWidth(Node* head) {if (!head) return;std::queue<Node*> queue;queue.push(head);std::unordered_map<Node*, int> levelMap; // 统计每个节点以及该节点所在层数levelMap.emplace(head, 1); // or levelMap.push(std::pair(head,1)) <utility>;int curLevel = 1; // 当前统计层int curLevelNodes = 0; // 当前层的节点数int max = INT_MIN;while (!s.empty()) {// 出队,获取该节点所在层Node* cur = queue.front();queue.pop();int curNodeLevel = levelMap.at(cur); // 该节点所在层与当前正在统计层一致if (curNodeLevel == curLevel) {curLevelNodes++;} else { // 不一致,清算,开始统计下一层max = std::max(max, curLevelNodes);curLevel++;curLevelNodes = 1;}// 把出队节点左右孩子入队,其所在层数为cur所在层+1if (cur->left) {levelMap.emplace(cur->left, curNodeLevel+1);queue.push(cur->left);}if (cur->right) {levelMap.emplace(cur->right, curNodeLevel+1);queue.push(cur->right);}}}
3、概念
3.1 二叉搜索树(BST)
对于每个节点,它的值大于其左子树中的所有节点的值,同时小于其右子树中的所有节点的值。 且不包含重复的节点值。
BST的特性:
- 查找: 可以快速查找特定值,通过比较节点值并根据大小关系逐步向下遍历树。
- 插入: 插入新节点,保持树的结构仍然满足BST的性质。
- 删除: 可以删除搜索二叉树中的节点,需要考虑不同情况下的处理方式,以保持树的性质。
- 中序遍历: 得到一个升序的节点值序列。
3.1.1 判断一棵树是否是BST(递归中序遍历)
leetcode 98 中等
可以采用递归中序遍历,判断节点是否为升序。如果忘了递归遍历法请回看第1节中的内容
int pre = INT_MIN;
bool isBST1(Node* head) {if (!head) {return true; // 空树也是BST}if (!isBST(head->left)) {return false;}// 中序遍历需要在这里做节点访问操作if (head->val <= pre) {// BST左树严格小于根节点,所以判断别忘了=号return false;}pre = head->val;return isBST(head->right);
}
也可以采用先递归中序遍历拿到所有节点值,后判断是否为升序排列也可以,不过要额外空间O(N)。看起来更复杂,实则逻辑更简单
void inOrderRecur(Node* head, std::vector<int>& inOrderArr) {if (!head ) return true;inOrderRecur(head->left, inOrderArr));inOrderArr.emplace_back(head->val);inOrderRecur(head->right, inOrderArr); } bool isBST2(Node* head) {std::vector<int> inOrderArr;inOrderRecur(head, inOrderArr);for (int i = 0; i < inOrderArr.size() - 1; i++) {if (inOrderArr[i] >= inOrderArr[i+1])return false;}return true; }
3.1.2 判断一棵树是否是BST(非递归中序遍历)
- 对当前的左边界节点按从上到下的顺序压入栈中
- 弹出栈顶节点并访问(打印)
- 对其右子树重复以上操作
#include <stack>
#include <limits>
bool isBST3(Node* head) {int pre = INT_MIN; // long pre = LONG_MIN;if (head) {std::stack<Node*> stack;while (!stack.siEmpty() || head) {if (head) { // 左边界节点循环入栈stack.emplace(head);head = head->left;} else {// 出栈head = stack.top();// 访问if (head->val <= pre) {return false;} else {pre = head->val;}// 队右子树重复以上步骤head = head->right;}}}// 左边界入栈}
需要注意的是,如果插入的节点顺序不合理,搜索二叉树可能会变得不平衡,导致查找、插入和删除操作的性能下降。为了避免这种情况,有时候需要采取平衡二叉搜索树(例如 AVL 树、红黑树)来保持树的平衡性。
3.2 完全二叉树(CBT)
一种尽可能填满层级的二叉树,左侧的节点优先填充。
1/ \2 3/ \ / 4 5 6
3.2.1 判别一棵树是否是CBT(层序遍历)
LeetCode 958 中等
使用层序遍历(广度优先遍历),按层级顺序访问每个节点。
- 如果遇到任何一个节点有右无左,false
- 遇到第一个孩子不双全的节点后,后面的所有节点都必须是叶子节点(无孩),否则false。
#include <queue>
bool isCBT(Node* head) {std::queue<Node*> queue;queue.emplace(head);bool firstNotFull = false; // 遇到第一个不双全节点while (!queue.empty()) {head = queue.front();queue.pop();Node* l = head->left;Node* r = head->right;// 有右无左 or 第一次遇到不双全节点后,后续任意节点有孩(非叶子节点)if ( (!l && r) || (firstNotFull && (l || r)) ) {return false;}if (l) queue.emplace(l);if (r) queue.emplace(r);if (!l || !r) {// 孩子不全有firstNotFull = true;}}return true;
}
3.3 平衡二叉树(BBT)(介绍树形DP递归套路)
平衡二叉树(BBT):任何一棵子树,左右树的高度差不超过1。
树形DP问题,递归套路:
- 在遇到二叉树问题的时候,先思考如何向左右树拿信息,两棵树的操作必须相同
- 然后根据已有信息罗列并判断本树的可能性
一般题型都能做出来,无法用这个套路求解的题,一般都很难了,不太容易考到
代码框架也很简单参考下面的例子
以判断平衡二叉树为例,看如何解二叉树题目。
例题:判断一棵树是否平衡
(1) 左树:是否平衡,计算高度
(2) 右树:是否平衡,计算高度
(3) |左树高度 - 右树高度| <= 1
满足以上三个条件,则是平衡二叉树
#include <tuple>
#include <algorithm> // std::max()
#include <cmath>
bool isBalance(Node* head) {return std::get<0>(process(head));
}
// 返回值是:是否平衡,树深度
std::tuple<bool, int> process(Node* x) {if (!x) { // base,x为空return {true, 0};}// 拿左右树信息auto [leftIsBBT, leftH] = process(x.left);auto [rightIsBBT, rightH] = process(x.right);// 根x所代表的树是否平衡bool isBBT = leftIsBBT && rightIsBBT && std::abs(leftH - rightH) < 2;// 根x所代表的树的高度int h = std::max(leftH, rightH) + 1;return {isBBT, h};
};
C++中多返回值传统来讲都用返回一个结构体来实现,C++11提供了
元组std::tuple可以实现返回多个值。不过他最不方便的是需要用std::get<>()来获取指定的第几个成员,代码看上去很臃肿
C++17特性:结构化绑定,可以实现这样的取值:(同样适用于结构体、数组)std::tuple<int, string, float> myTuple; auto [v1, v2, v3] = myTuple; // auto [_, v2, _] = myTuple;
再来一道: 判断BST
(1) 左树:是否为BST,计算最大值 leftMax
(2) 右树:是否为BST,计算最小值 rightMin
(3) eftMax < head.val && rightMin > head.val
满足以上三个条件,则是二叉搜索树。但是左右树操作不同,递归不了怎么办?—— 强行让他们相同,把左树右树的信息改成三个(求他俩全集),即都提供:是否为BST、最大值、最小值
#include <tuple>
#include <algorithm>
#include <cmath>
bool isBST(Node* head) {return std::get<1>(process(head));
}
// 模板中参数分别表示:是否有效、是否为BST、最小值、最大值
std::tuple<bool, bool, int, int> process(Node* x) {if (!x) { // 因为空树情况min max的值不好指定,因此引入一个标志变量,便于后续判断return {false, false, 0, 0}; // 仅当第一个成员为true,后三个成员才有效}auto [leftIsValid, leftIsBST, leftMax, leftMin] = process(x.left);auto [rightIsValid, rightIsBST, rightMax, rightMin] = process(x.right);// 更新用来向上返回的min max, 初始化为根节点的值int min = x.value;int max = x.value;if (leftIsValid) { min = std::min(min, leftMin);max = std::max(max, leftMax);}if (rightIsValid) {min = std::min(min, rightMin);max = std::max(max, rightMax);}bool isBST;// 如果左子树不为空 且 它不是BST 或 它的max >= x.val 则本树不是BSTif (leftIsValid && (!leftIsBST || leftMax >= x.val)) {isBST = false;} // 同理判断右树是否满足条件if (rightIsValid && (!rightIsBST || rightMin <= x.val)) {isBST = false;}return {true, isBST, min, max};
}
3.4 满二叉树(FBT)
深度 h h h 和节点个数 n n n 满足 n = 2 n − 1 n = 2^{n}-1 n=2n−1
一样,用树形DP套路来解决
(1) 左右树信息:子树深度、节点数
(2) 判断本节点所代表的树是否是满二叉树
#include <algorithm>
#include <tuple>
bool isFBT(Node* head) {auto [h, n] = process(head);return n == 1 << h - 1; // 1左移h位就是乘以h个2,再-1 2^n - 1
}std::tuple<int, int> process(Node* x) {if (!x) {return {0, 0};}auto [leftH, leftNodes] = process(x.left);auto [rightH, rightNodes] = process(x.right);int height = std::max(leftH, rightH) + 1;int nodes = leftNodes + rightNodes + 1;return {h, nodes};
}
4 刷题
4.1 题:给定一棵树上的两个节点,找到他们的最低公共祖先节点
递归解法:
- 遍历整棵树,记录每个节点的父节点到map中,使得可以实现从树下往上走
- 从第一个目标节点开始从下往上记录所有父节点到哈希set中
- 挨个检查第二个目标节点的祖先父、爷等节点是否在set中,第一个重复节点就是公共祖先
#incldue <unordered_map>
#include <unordered_set>
Node* lca(Node* head, Node* o1, Node* o2) {std::unordered_map<Node*, Node*> fatherMap;// 记录所有结点的父节点fatherMap.emplace(head, head);process(head, fatherMap);// 记录o1结点的祖先们std::unordered_set<Node*> set1;Node* cur = o1;while (cur != fatherMap[cur]) {// 终止条件为二叉树根节点,cur! = head也行set1.emplace(cur);cur = fatherMap[cur];}set1.emplace(head); // 上面循环到head就停了,还需单独放入head// 检查o2的祖先节点们,在set1中首次重复的节点,就是公共节点cur = o2;while (cur != head) {if (set1.find(fatherMap[cur]) != fatherMap.end())return fatherMap[cur];cur = fatherMap[cur];}
}void process(Node* head, std::unordered_map<Node*, Node*>& fatherMap) {if (!head) return;fatherMap.emplace(head.left, head);fatherMap.emplace(head.right, head);process(head->left, fatherMap);process(head->right,fatherMap);
}
另一种优化后的写法,考虑o1 o2有哪些分布情况
- o1是o2的LCA 或 o2是o1的LCA
- 两者不互为公共祖先,往上追的话在某个节点汇聚
Node* lca(Node* head, Node* o1, Node* o2) {if (!head || head == o1 || head == o2) { // base casereturn head;}Node* left = lca(head->left, o1, o2);Node* right = lac(head->right, o1, o2);// 左右都有返回值(服务于情况2,对情况1永远不成立)if (left != nullptr && right != nullptr) {return head; // 当左右都有返回值,则返回自己,自己就是公共祖先}// 都没返回值或有一个有返回值(返回不为空的那个,都没有则返回null)return left != null ? left : right;
}
4.2 题:找中序遍历下的某个节点的后继节点
二叉树结构如下:
class Node {int val;Node* left;Node* right;Node* parent;Node(int x) : val(x), left(nullptr), right(nullptr) {}
};
假设现在有一棵树,每个节点的parent都指向正确的父节点,头结点的parent指向为null。中序遍历中,某个节点的下一个节点称为后继结点,请设计一个函数,返回二叉树中某个节点的后继结点
方法1:最简单的中序遍历一次树,得到一个中序结果,然后遍历该列表找到后继节点,这样的代价是O(N),但这样好像连父指针都没用到,肯定有更快的方法。
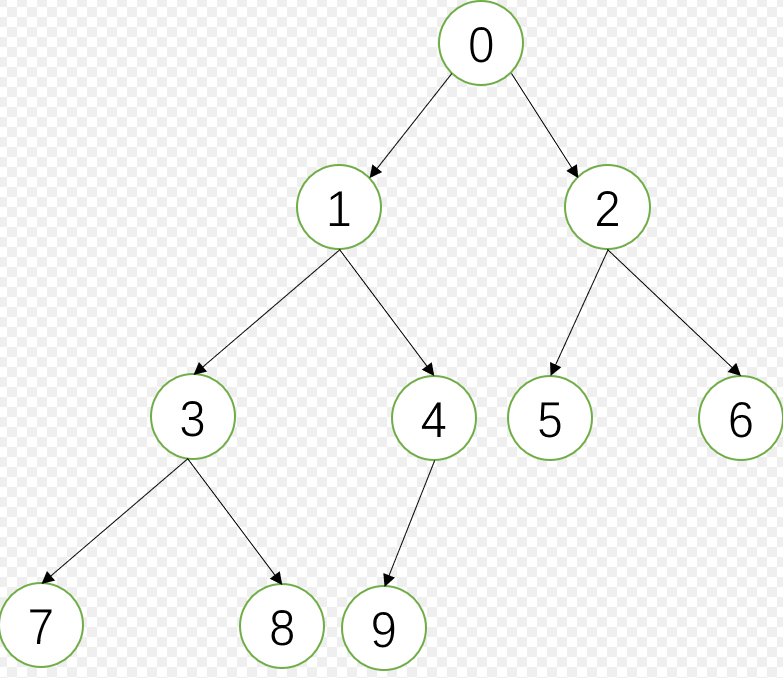
实际上也不难,对于下面这棵树而言,中序遍历结果为:7381940526,我们观察可以知道以就一个规律:靠左侧的节点优先
所以对于任何一个节点的中序后继,就很简单了:
- 有右子树,则后继为右子树最左侧节点
- 无右树,看看自己是不是父节点的左孩子,如果是,则后继为父节点,如果不是则继续找。。。直到找到某个节点是它父节点的左孩子为止
- 如果到最后都没找到(父节点为null了,到头节点了),那就没有后继节点。即他是整棵树最右侧节点
代码:
Node* getLeftMost(Node* node) {if (!node) return node;while (node->left) {node = node->left;}return node;
}
Node* getSuccessorNode(Node* node) {if (!node) return node;// 有右子树if (node->right) {return getLeftMost(node->right);} else {// 没右子树,往上窜Node* parent = node->parent;while (parent && node != parent->left) {node = parent;parent = node->parent;}// while执行完有两种情况:// 1 parent为空,目前节点是最右侧节点// 2 parent不为空,它就是后继return parent; }
}
4.3 二叉树的序列化与反序列化
二叉树的序列化与反序列化是指将一个二叉树转换为字符串表示,以便存储或传输,并且能够将该字符串重新转换回原始的二叉树结构。这种操作在很多应用中都有用途,例如在网络传输二叉树数据、保存到文件中,或者在数据结构中进行持久化存储。
- 序列化:树->字符串
- 反序列化:字符串->树
序列化 (Serialize):
用先序遍历将二叉树转换为字符串。在遍历过程中,将每个节点的值添加到字符串中,同时用特殊字符来表示空节点。(中序后续层序都是一样的)
std::string serializeByPre(Node* head) {if (!head) return "#,";std::string res = head->val + ",";res += serializeByPre(head->left);res += serializeByPre(head->right);return res;
}
反序列化 (Serialize):
// 根据分隔符提取字符串各个段
Node* reconByPreString(std::string preStr) {std::istringstream iss(preStr);std::queue<std::string> queue;std::string val;while (std::getline(iss, val, '_')) {queue.push(val);}return reconPreOrder(queue);
}
Node* reconPreOrder(std::queue<std::string>& queue) {std::string value = queue.front();queue.pop();if (value == "#") return nullptr;Node* head = new Node(std::stoi(value));head->left = reconPreOrder(queue);head->right = reconPreOrder(queue); return head;
}
4.4 打印凹凸折痕问题
一张纸条,对折N次,请设计一个函数从上到下打印所有折痕的凹凸性 视频地址
第一次对折,一个凸痕
第二次对折,在第一次的凸痕的上下方各出现一个凹痕和凸痕
后面每一次对折的所有痕迹的上下出现一个凹痕一个凸痕
根据规律,这其实会形成一个二叉树,想从纸条的上到下,则中序遍历即可

代码
// down为真表示凹,i是节点层数,N为总层数
void printProcess(int i, int N, bool down) {if (i > n) {return;}printProcess(i + 1, N, true); // 所有节点的左孩子都是凹if (down) {std::cout << "凹 ";} else {std::cout << "凸 ";}printProcess(i + 1, N, false);
}
)




![P1328 [NOIP2014 提高组] 生活大爆炸版石头剪刀布](http://pic.xiahunao.cn/P1328 [NOIP2014 提高组] 生活大爆炸版石头剪刀布)

(甲骨文))





真题解析#中国电子学会#全国青少年软件编程等级考试)





)