1. 计算器问题

思路：此题不考虑括号和负数情况，单纯使用栈即可解决。注意的是数字可能是多位数需要保留完整的num， 保留数字的前缀符号，当碰到加号，存进去；当碰到减号，存相反数进去；当碰到乘除就弹出栈顶元素运算后把结果存进去；最后相加就是结果

class Solution {public int calculate(String s) {Deque<Integer> stack = new ArrayDeque<Integer>();char preSign = '+';int num = 0;int n = s.length();for (int i = 0; i < n; ++i) {char c = s.charAt(i);if(c >= '0' && c <= '9'){num = num*10 + c - '0';} if((!(c >= '0' && c <= '9')&&c != ' ') || i==n-1 ){switch(preSign){case '+' :stack.push(num);break;case '-' :stack.push(-num);break;case '/':stack.push(stack.pop()/num);break;case '*':stack.push(stack.pop()*num);break;}preSign = c;num=0;}}num = 0;while(!stack.isEmpty()){num += stack.pop();}return num;}
}

 2.逆波兰表达式

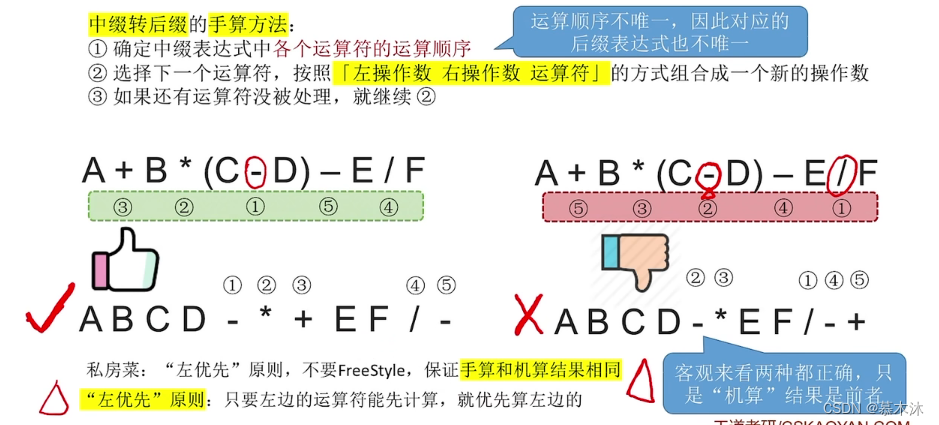
• 中缀表达式
  • 运算符在两个操作数中间
• 后缀表达式
  • 运算符在两个操作数后面
• 前缀表达式
  • 运算符在两个操作数前面

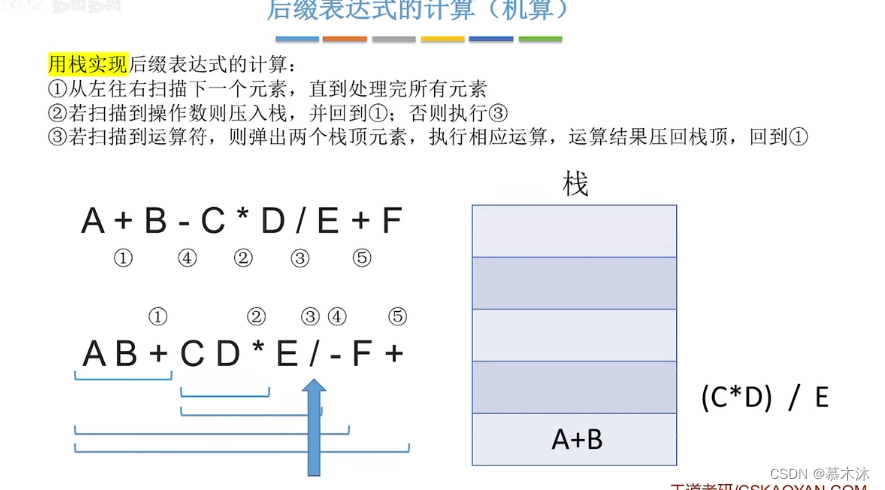
class Solution {public int evalRPN(String[] tokens) {Deque<Integer> stack = new LinkedList<>();for(int i = 0;i<tokens.length;i++){if(!Character.isDigit(tokens[i].charAt(0)) && tokens[i].length() == 1){int num2 = stack.pop();int num1 = stack.pop();switch (tokens[i]) {case "+":stack.push(num1 + num2);break;case "-":stack.push(num1 - num2);break;case "*":stack.push(num1 * num2);break;case "/":stack.push(num1 / num2);break;default:}}else{stack.push(Integer.parseInt(tokens[i]));}}return stack.pop();}
}