算法入门篇六 二叉树

牛客网 算法入门篇 左程云老师 个人复习，如果侵全，设为私密

二叉树遍历（递归）

  • 先序遍历（中，左，右）

  • 中序遍历（左，中，右）

  • 后序遍历（左，右，中）

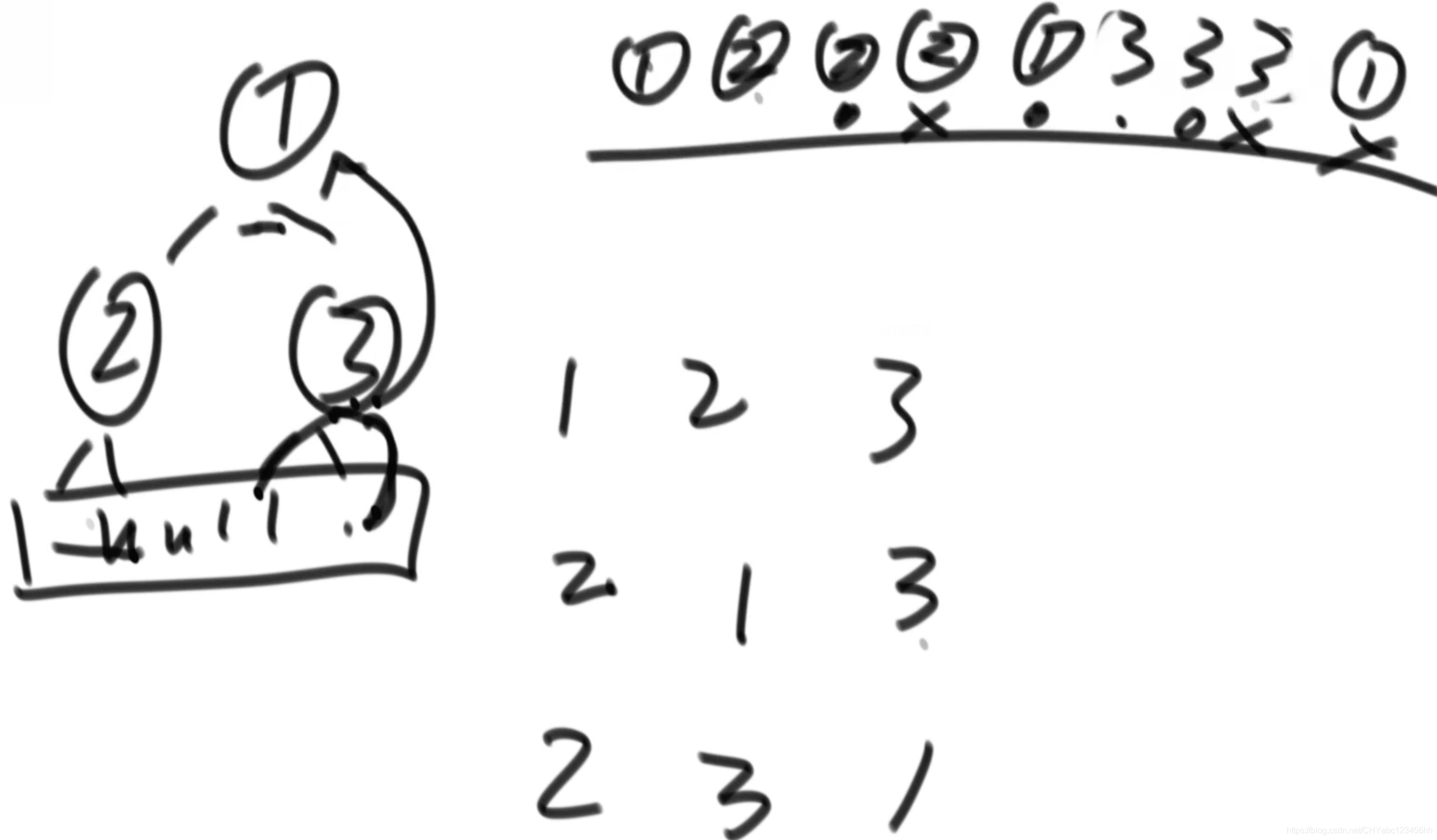
  • 如上图所示结构，二叉树的遍历本质上都是递归序，1、2和3节点每个都会出现三次，比如从节点1出发，来到节点2，节点2的左边为空，返回，打印2，右边为空，返回打印2，再返回到节点1，节点3类似。所以最后输出的序列为1，2，2，2，1，3，3，3，1。
  • 如果打印递归序出现的第1次的元素，就是先序遍历
  • 如果打印递归序出现的第2次的元素，就是中序遍历
  • 如果打印递归序出现的第3次的元素，就是后序遍历

二叉树遍历（非递归）

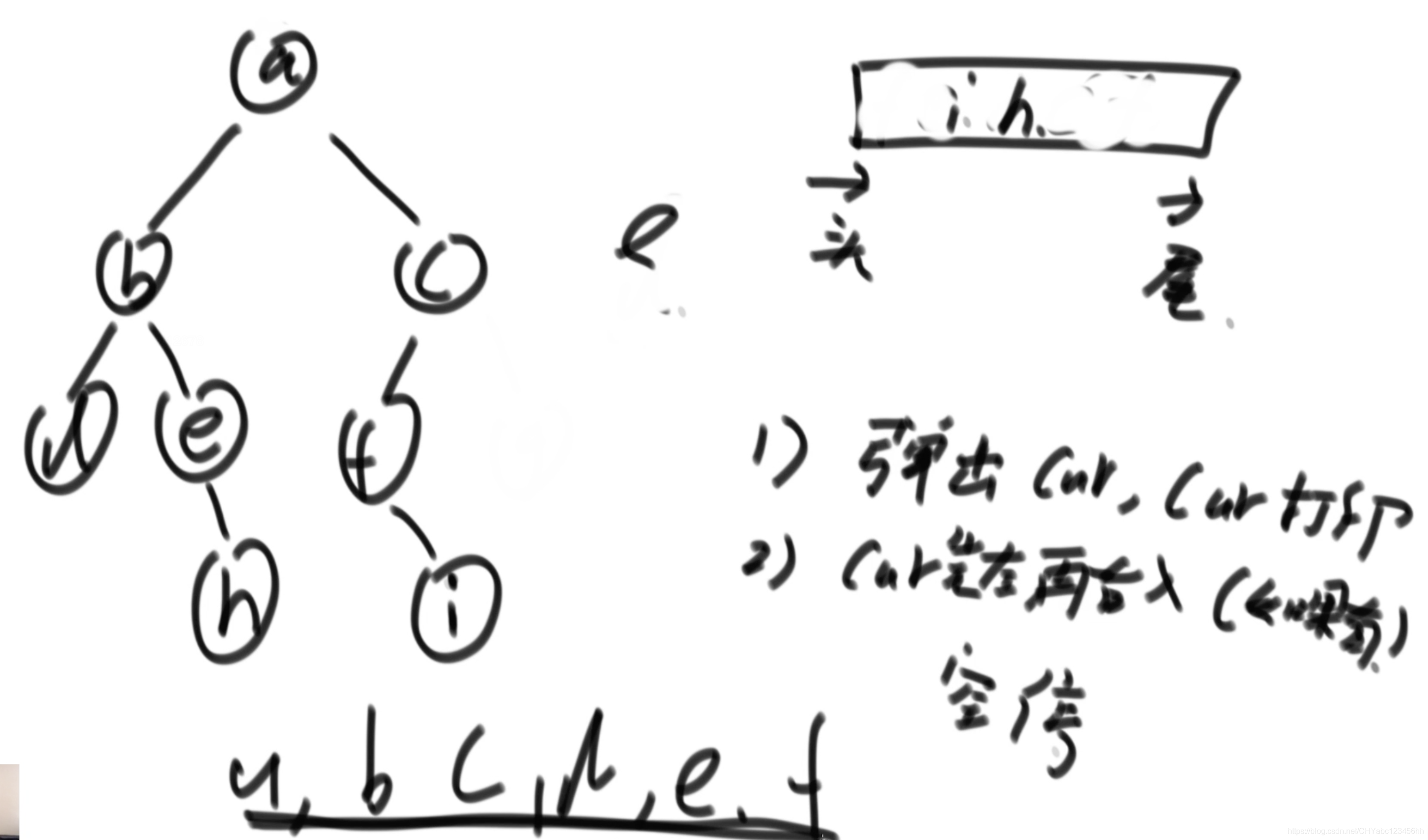
先序遍历

  • 二叉树的结构如图所示，准备一个栈用于接收数据
  • 原则只有两点：1，栈中弹出节点叫做cur（当前节点），弹出就打印；2，先打印cur的右节点，仔打印左节点，没有就无需操作。栈空就停止。
  • 1进栈，弹出1，打印1；将3和2压入栈中，弹出2，打印2，将2的孩子节点4和5押入栈中；因为先押入右，再压左，因此先将5押入，再押入4；弹出4，打印4；如上所述，先序遍历为1，2，4，5，3，6，7

代码

package class05;import java.util.Stack;public class Code01_PreInPosTraversal {public static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static void f(Node head) {// 1if (head == null) {return;}// 1f(head.left); //2//2f(head.right);// 3// 3}public static void preOrderRecur(Node head) {if (head == null) {return;}System.out.print(head.value + " ");preOrderRecur(head.left); preOrderRecur(head.right);}public static void inOrderRecur(Node head) {if (head == null) {return;}inOrderRecur(head.left);System.out.print(head.value + " ");inOrderRecur(head.right);}public static void posOrderRecur(Node head) {if (head == null) {return;}posOrderRecur(head.left);posOrderRecur(head.right);System.out.print(head.value + " ");}public static void preOrderUnRecur(Node head) {System.out.print("pre-order: ");if (head != null) {Stack<Node> stack = new Stack<Node>();stack.add(head);while (!stack.isEmpty()) {head = stack.pop();System.out.print(head.value + " ");if (head.right != null) {stack.push(head.right);}if (head.left != null) {stack.push(head.left);}}}System.out.println();}public static void inOrderUnRecur(Node head) {System.out.print("in-order: ");if (head != null) {Stack<Node> stack = new Stack<Node>();while (!stack.isEmpty() || head != null) {if (head != null) {stack.push(head);head = head.left;} else {head = stack.pop();System.out.print(head.value + " ");head = head.right;}}}System.out.println();}public static void posOrderUnRecur1(Node head) {System.out.print("pos-order: ");if (head != null) {Stack<Node> s1 = new Stack<Node>();Stack<Node> s2 = new Stack<Node>();s1.push(head);while (!s1.isEmpty()) {head = s1.pop();s2.push(head);if (head.left != null) {s1.push(head.left);}if (head.right != null) {s1.push(head.right);}}while (!s2.isEmpty()) {System.out.print(s2.pop().value + " ");}}System.out.println();}public static void posOrderUnRecur2(Node h) {System.out.print("pos-order: ");if (h != null) {Stack<Node> stack = new Stack<Node>();stack.push(h);Node c = null;while (!stack.isEmpty()) {c = stack.peek();if (c.left != null && h != c.left && h != c.right) {stack.push(c.left);} else if (c.right != null && h != c.right) {stack.push(c.right);} else {System.out.print(stack.pop().value + " ");h = c;}}}System.out.println();}public static void main(String[] args) {Node head = new Node(5);head.left = new Node(3);head.right = new Node(8);head.left.left = new Node(2);head.left.right = new Node(4);head.left.left.left = new Node(1);head.right.left = new Node(7);head.right.left.left = new Node(6);head.right.right = new Node(10);head.right.right.left = new Node(9);head.right.right.right = new Node(11);// recursiveSystem.out.println("==============recursive==============");System.out.print("pre-order: ");preOrderRecur(head);System.out.println();System.out.print("in-order: ");inOrderRecur(head);System.out.println();System.out.print("pos-order: ");posOrderRecur(head);System.out.println();// unrecursiveSystem.out.println("============unrecursive=============");preOrderUnRecur(head);inOrderUnRecur(head);posOrderUnRecur1(head);posOrderUnRecur2(head);}}

中序遍历（非递归）

  • 原则只有两点：1，栈中弹出节点叫做cur（当前节点），弹出就打印；2，先打印cur的左节点，仔打印右节点，没有就无需操作。栈空就停止。
  • 不断将右节点分成左和中节点

后序遍历（非递归）

  • 原则只有两点：1，栈中弹出节点叫做cur（当前节点），弹出不打印，放到一个新的栈中；2，最后将第二个栈中的元素打印，相当于是（左，右，中），即后序遍历

直观打印二叉树

package class05;public class Code02_PrintBinaryTree {public static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static void printTree(Node head) {System.out.println("Binary Tree:");printInOrder(head, 0, "H", 17);System.out.println();}public static void printInOrder(Node head, int height, String to, int len) {if (head == null) {return;}printInOrder(head.right, height + 1, "v", len);String val = to + head.value + to;int lenM = val.length();int lenL = (len - lenM) / 2;int lenR = len - lenM - lenL;val = getSpace(lenL) + val + getSpace(lenR);System.out.println(getSpace(height * len) + val);printInOrder(head.left, height + 1, "^", len);}public static String getSpace(int num) {String space = " ";StringBuffer buf = new StringBuffer("");for (int i = 0; i < num; i++) {buf.append(space);}return buf.toString();}public static void main(String[] args) {Node head = new Node(1);head.left = new Node(-222222222);head.right = new Node(3);head.left.left = new Node(Integer.MIN_VALUE);head.right.left = new Node(55555555);head.right.right = new Node(66);head.left.left.right = new Node(777);printTree(head);head = new Node(1);head.left = new Node(2);head.right = new Node(3);head.left.left = new Node(4);head.right.left = new Node(5);head.right.right = new Node(6);head.left.left.right = new Node(7);printTree(head);head = new Node(1);head.left = new Node(1);head.right = new Node(1);head.left.left = new Node(1);head.right.left = new Node(1);head.right.right = new Node(1);head.left.left.right = new Node(1);printTree(head);}}

求二叉树的最大宽度

使用队列

  • 使用一个队列，从头部进入，从尾巴出来；
  • 原则：弹出当前节点cur，弹出并打印；当前节点的话存在左右节点的话，先放入左节点，再放入右节点。如果不存在孩子节点，等队列为null的话，就停止输出。但是，存在一个问题，我们不知道哪些节点是类属于一层的，因此需要进行指定。需要引入哈希表来统计相关的层数、以及最大的跨度

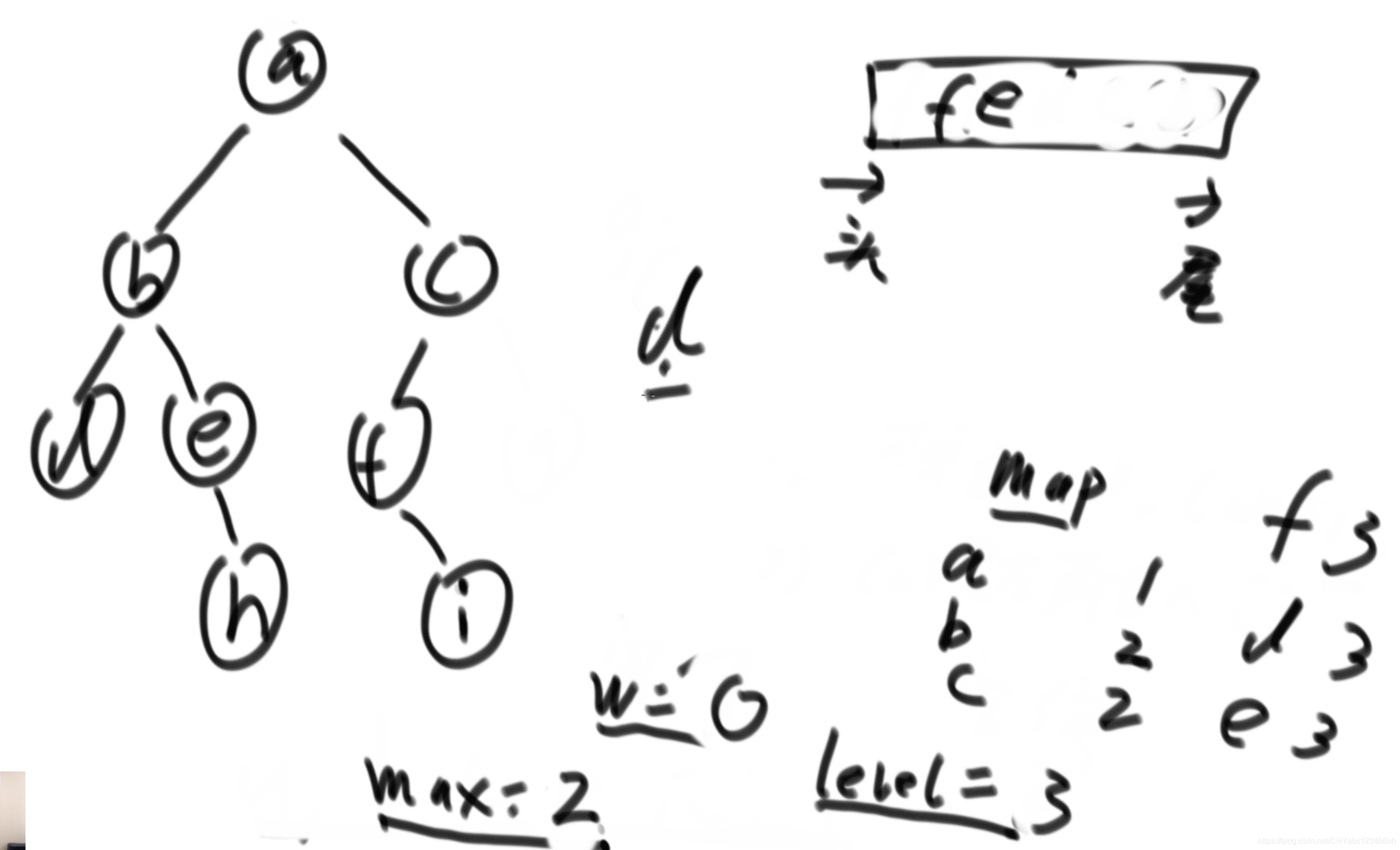
使用哈希表

  • 引入哈希表，设置三个变量，max为全局最大宽度，w为统计当前层级的宽度值，level记录统计层级
  • 初始设置max=-1，w=0，level=1；当a输入队列，w变为1，level显示当前层级为1，当a出队列，将其孩子节点b和c放入队列，当b出队列，level查询发现b是2层的，因此将w和max比较大小，将大的数值赋值给max，然后将w清除数据，重新统计第二层级的数的个数。以此类推。

代码

package class05;import java.util.HashMap;
import java.util.LinkedList;
import java.util.Queue;public class Code03_TreeMaxWidth {public static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static int w(Node head) {if(head == null) {return 0;}Queue<Node> queue = new LinkedList<>();queue.add(head);HashMap<Node, Integer> levelMap = new HashMap<>();levelMap.put(head, 1);int curLevel = 1;int curLevelNodes = 0;int max = Integer.MIN_VALUE;while(!queue.isEmpty()) {Node cur = queue.poll();int curNodeLevel = levelMap.get(cur);if(curNodeLevel == curLevel) {curLevelNodes++;} else {max = Math.max(max, curLevelNodes);curLevel++;curLevelNodes = 1;}if(cur.left !=null) {levelMap.put(cur.left, curNodeLevel+1);queue.add(cur.left);}if(cur.right !=null) {levelMap.put(cur.right, curNodeLevel+1);queue.add(cur.right);}}return max;}public static int getMaxWidth(Node head) {if (head == null) {return 0;}int maxWidth = 0;int curWidth = 0;// 目前的层数int curLevel = 0;// node 所在的层数HashMap<Node, Integer> levelMap = new HashMap<>();levelMap.put(head, 1);LinkedList<Node> queue = new LinkedList<>();queue.add(head);Node node = null;Node left = null;Node right = null;while (!queue.isEmpty()) {node = queue.poll();left = node.left;right = node.right;if (left != null) {levelMap.put(left, levelMap.get(node) + 1);queue.add(left);}if (right != null) {levelMap.put(right, levelMap.get(node) + 1);queue.add(right);}if (levelMap.get(node) > curLevel) {curWidth = 1;curLevel = levelMap.get(node);} else {curWidth++;}maxWidth = Math.max(maxWidth, curWidth);//更新最后一层，因为最后一层没有触发逻辑}return maxWidth;}public static void main(String[] args) {// TODO Auto-generated method stub}}

 二叉树的递归套路

如何判断一棵树是满二叉树

  • 性质 节点数 = 2^树的高度 - 1
  • 思路 假设以x为头节点，只有满足性质才是一个满二叉树。在容许向左右两个孩子要信息的前提下，应该要什么信息，才可以解决问题。
public class IsFull{public static class Node{public int value;public Node left;public Node right;public Node(int data){this.value = data;}}public static boolean isFull(Node head){Info info = processInfo(head);int size = info.size;int height = info.height;return size == (1<<height) - 1;}public static class Info{public int size;public int height;public Info(int s,int h){size = s;height = h;}}public static Info processInfo(Node x){if(x == 0){return new Info(0,0);}Info leftInfo = processInfo(x.left);Info rightInfo = processInfo(x.right);int size = leftInfo.size + rightInfo.size + 1;int height = Math.max(leftInfo.height,rightInfo.height) + 1;return new Info(size, height);}public static void main(String[] args) {}
}

方法归纳

  • 假设要求以x为头的答案
  • 向左右两个孩子要信息，去分析构成答案的主要元素
  • 确定向左右孩子要的信息，有可能左右要的信息不一样
  • 组织收集到的信息

判断以x为头的二叉树是否是平衡二叉树

  • 判断左右孩子的高度差是否相差小于等于1
  • 如果左右孩子不满足平衡二叉树，那么此平衡二叉树不成立

代码

import jdk.vm.ci.code.site.Infopoint;public class IsFull{public static class Node{public int value;public Node left;public Node right;public Node(int data){this.value = data;}}public static class Info{public boolean isBalanced;public int height;public Info(boolean is,int h){isBalanced = is;height = h;}}public static Info process(Node x){if(x == nll){return new Info(true,0);//return null;}Info leftInfo = process(x.left);Info rightInfo = process(x.right);int subTreeMaxHeight = 0;if(leftInfo != null){subTreeMaxHeight = leftInfo.height;}if(rightInfo!=null){subTreeMaxHeight = Math.max(subTreeMaxHeight,rightInfo.height);}int height = 1 + subTreeMaxHeight;boolean isBalanced = true;if(leftInfo!=null && !leftInfo.isBalanced){isBalanced=false;}if(rightInfo!= null && !rightInfo.isBalanced){isBalanced = false;}int leftH = leftInfo != null ? leftInfo.height : 0;int rightH = leftInfo != null ? rightInfo.height : 0; if(Math.abs(leftH - rightH)>1){isBalanced = false;}return new Infopoint(isBalanced, height);}public static void main(String[] args) {}
}

求树中两个节点的最大距离

情况分类

和头节点x无关

  • 左树上的最大距离
  • 右树上的最大距离

和头节点x相关

  • 左边距离x最远和x到右边最远距离（树的高度）

代码

import org.graalvm.compiler.nodes.calc.LeftShiftNode;
import org.graalvm.compiler.nodes.calc.RightShiftNode;import jdk.vm.ci.code.site.Infopoint;public class IsFull{public static int maxDistance(Node head){Info info = process(head);return info.maxDistance;}public static class Info{public int maxDistance;public int height;public Info(boolean is,int h){maxDistance = d;height = h;}}public static Info process(Node x){if(x == null){return new Info(0,0);}Info leftInfo = process(x.left);Info rightInfo = process(x.right);int height = Math.max(leftInfo.height,rightInfo.height) + 1;int maxDistance = Math.max(leftInfo.height + rightInfo.height + 1,Math.max(leftInfo.height,rightInfo.height));return new Info(maxDistance,height);}   public static void main(String[] args) {}
}

判断一个树是否是搜索二叉树

套路

判定条件

  • 左树是否是搜索二叉树
  • 右树是否是搜索二叉树
  • 左边最大的是否小于 x节点
  • 右边最小的是否大于 x节点

代码

package class05;import java.util.LinkedList;
import java.util.Stack;import class05.Code01_PreInPosTraversal.Node;public class Code04_IsBST {public static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static class ReturnData {public boolean isBST;public int min;public int max;public ReturnData(boolean is, int mi, int ma) {isBST = is;min = mi;max = ma;}}public static ReturnData process(Node x) {if(x == null) {return null;}ReturnData leftData = process(x.left);ReturnData rightData = process(x.right);int min = x.value;int max = x.value;if(leftData!=null) {min = Math.min(min, leftData.min);max = Math.max(max, leftData.max);}if(rightData!=null) {min = Math.min(min, rightData.min);max = Math.max(max, rightData.max);}
//      boolean isBST = true;
//      if(leftData!=null && (!leftData.isBST   ||  leftData.max >= x.value  )) {
//          isBST= false;
//      }
//      if(rightData!=null && ( !rightData.isBST || x.value >= rightData.min   )) {
//          isBST= false;
//      }boolean isBST = false;if((leftData != null ? (leftData.isBST  &&  leftData.max < x.value) : true)&&(rightData !=null ? (rightData.isBST  && rightData.min > x.value) : true)        ) {isBST = true;}return new ReturnData(isBST, min, max);}public static boolean isF(Node head) {if(head == null) {return true;}Info data = f(head);return data.nodes == (1 << data.height - 1);}public static class Info{public int height;public int nodes;public Info(int h, int n) {height = h;nodes = n;}}public static Info f(Node x) {if(x == null) {return new Info(0,0);}Info leftData = f(x.left);Info rightData = f(x.right);int height  = Math.max(leftData.height,rightData.height)+1;int nodes = leftData.nodes + rightData.nodes + 1;return new Info(height, nodes);}public static boolean inOrderUnRecur(Node head) {if (head == null) {return true;}int pre = Integer.MIN_VALUE;Stack<Node> stack = new Stack<Node>();while (!stack.isEmpty() || head != null) {if (head != null) {stack.push(head);head = head.left;} else {head = stack.pop();if (head.value <= pre) {return false;}pre = head.value;head = head.right;}}return true;}public static boolean isBST(Node head) {if (head == null) {return true;}LinkedList<Node> inOrderList = new LinkedList<>();process(head, inOrderList);int pre = Integer.MIN_VALUE;for (Node cur : inOrderList) {if (pre >= cur.value) {return false;}pre = cur.value;}return true;}public static void process(Node node, LinkedList<Node> inOrderList) {if (node == null) {return;}process(node.left, inOrderList);inOrderList.add(node);process(node.right, inOrderList);}}

也可以中序遍历

  • 只要递增，就是搜索二叉树

  • 基于非递归中序遍历改进，由先前的打印，变为和前一个节点比较

代码

public static boolean inOrderUnRecur(Node head){if(head == null){return true;}int pre = Integer.MIN_VALUE;Stack<Node> stack = new Stack<Node>();while(!stack.isEmpty() || head != null){if(head != null){stack.push(head);head = head.left;}else{head = stack.pop();if(head.value <= pre){return false;}pre = head.value;head = head.right;}}return true;}

不可以使用套路来做

判断一棵树是否是完全二叉树

  • 如果使用条件，左子树是否是完全二叉树，右子树是否是完全二叉树来判定根节点是否是完全二叉树

  • 即使左子树和右子树都是完全二叉树，但是左子树比右子树少整整一层的情形下，判定失败

思路

  • 宽度优先遍历，任何一个节点不能有右节点，没有左节点。
  • 当第一次发现某节点左右不双全，后续节点都是右节点
package class05;import java.util.LinkedList;public class Code05_IsCBT {public static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static boolean isCBT(Node head) {if (head == null) {return true;}LinkedList<Node> queue = new LinkedList<>();// 是否遇到过左右两个孩子不双全的节点boolean leaf = false;Node l = null;Node r = null;queue.add(head);while (!queue.isEmpty()) {head = queue.poll();l = head.left;r = head.right;if (// 如果遇到了不双全的节点之后，又发现当前节点不是叶节点(leaf && !(l == null && r == null)) || (l == null && r != null)) {return false;}if (l != null) {queue.add(l);}if (r != null) {queue.add(r);}if (l == null || r == null) {leaf = true;}}return true;}}

求n1和n2的最低公共主先

划分情况（x为头节点）

  • x无n1和n2
  • x只有n1
  • x只有n2
  • x有n1和n2：左n1n2；右n1n2；左n1右n2；左n2右n1

代码

package class05;import java.util.HashMap;
import java.util.HashSet;public class Code07_LowestCommonAncestor {public static class Node {public int value;public Node left;public Node right;public Node(int data) {this.value = data;}}public static Node lowestAncestor(Node head, Node o1, Node o2) {if (head == null || head == o1 || head == o2) { // base casereturn head;}Node left = lowestAncestor(head.left, o1, o2);Node right = lowestAncestor(head.right, o1, o2);if (left != null && right != null) {return head;}// 左右两棵树，并不都有返回值return left != null ? left : right;}public static class Record1 {private HashMap<Node, Node> map;public Record1(Node head) {map = new HashMap<Node, Node>();if (head != null) {map.put(head, null);}setMap(head);}private void setMap(Node head) {if (head == null) {return;}if (head.left != null) {map.put(head.left, head);}if (head.right != null) {map.put(head.right, head);}setMap(head.left);setMap(head.right);}public Node query(Node o1, Node o2) {HashSet<Node> path = new HashSet<Node>();while (map.containsKey(o1)) {path.add(o1);o1 = map.get(o1);}while (!path.contains(o2)) {o2 = map.get(o2);}return o2;}}public static class Record2 {private HashMap<Node, HashMap<Node, Node>> map;public Record2(Node head) {map = new HashMap<Node, HashMap<Node, Node>>();initMap(head);setMap(head);}private void initMap(Node head) {if (head == null) {return;}map.put(head, new HashMap<Node, Node>());initMap(head.left);initMap(head.right);}private void setMap(Node head) {if (head == null) {return;}headRecord(head.left, head);headRecord(head.right, head);subRecord(head);setMap(head.left);setMap(head.right);}private void headRecord(Node n, Node h) {if (n == null) {return;}map.get(n).put(h, h);headRecord(n.left, h);headRecord(n.right, h);}private void subRecord(Node head) {if (head == null) {return;}preLeft(head.left, head.right, head);subRecord(head.left);subRecord(head.right);}private void preLeft(Node l, Node r, Node h) {if (l == null) {return;}preRight(l, r, h);preLeft(l.left, r, h);preLeft(l.right, r, h);}private void preRight(Node l, Node r, Node h) {if (r == null) {return;}map.get(l).put(r, h);preRight(l, r.left, h);preRight(l, r.right, h);}public Node query(Node o1, Node o2) {if (o1 == o2) {return o1;}if (map.containsKey(o1)) {return map.get(o1).get(o2);}if (map.containsKey(o2)) {return map.get(o2).get(o1);}return null;}}// for test -- print treepublic static void printTree(Node head) {System.out.println("Binary Tree:");printInOrder(head, 0, "H", 17);System.out.println();}public static void printInOrder(Node head, int height, String to, int len) {if (head == null) {return;}printInOrder(head.right, height + 1, "v", len);String val = to + head.value + to;int lenM = val.length();int lenL = (len - lenM) / 2;int lenR = len - lenM - lenL;val = getSpace(lenL) + val + getSpace(lenR);System.out.println(getSpace(height * len) + val);printInOrder(head.left, height + 1, "^", len);}public static String getSpace(int num) {String space = " ";StringBuffer buf = new StringBuffer("");for (int i = 0; i < num; i++) {buf.append(space);}return buf.toString();}public static void main(String[] args) {Node head = new Node(1);head.left = new Node(2);head.right = new Node(3);head.left.left = new Node(4);head.left.right = new Node(5);head.right.left = new Node(6);head.right.right = new Node(7);head.right.right.left = new Node(8);printTree(head);System.out.println("===============");Node o1 = head.left.right;Node o2 = head.right.left;System.out.println("o1 : " + o1.value);System.out.println("o2 : " + o2.value);System.out.println("ancestor : " + lowestAncestor(head, o1, o2).value);System.out.println("===============");}}

 

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.mzph.cn/news/447213.shtml

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈email:809451989@qq.com，一经查实，立即删除！

相关文章

算法入门篇七 前缀树

算法入门篇七 前缀树

牛客网 左程云老师的算法入门课 找二叉树的节点的后继节点 原则 如果节点有右子树&#xff0c;那么后继节点就是右子树的最左边的第一个节点如果节点没有右子树&#xff0c;如果节点是父节点的右孩子&#xff0c;就继续往上找&#xff0c;直到找到一个父节点是沿途节点的父节…
阅读更多...
算法入门篇八 贪心算法

算法入门篇八 贪心算法

牛客网 左程云老师的算法入门课 贪心算法 贪心算法的解题步骤 例子 题目要求 解题策略 按照结束时间早的会议先安排&#xff0c;比如先安排【2&#xff0c;4】&#xff0c;当4结束了&#xff0c;所有开始时间小于4的全部淘汰&#xff0c;【1&#xff0c;7】、【3&#xff…
阅读更多...
算法入门篇九 暴力递归

算法入门篇九 暴力递归

牛客网 左程云老师的算法入门课 暴力递归 原则 汉诺塔问题 问题 打印n层汉诺塔从左边移动到最右边的过程 思想 一共六个过程&#xff0c;左到右、左到中&#xff0c;中到左&#xff0c;中到右&#xff0c;右到左&#xff0c;右到中&#xff0c;互相嵌套使用 左到右 将1…
阅读更多...
rtsp和sdp

rtsp和sdp

RTSP 是由Realnetwork 和Netscape共同提出的如何有效地在IP网络上传输流媒体数据的应用层协议 。 实时流协议&#xff08;RTSP&#xff09;建立并控制一个或几个时间同步的连续流媒体&#xff0c;如音频和视频。尽管连续媒体流与控制流交叉是可能的&#xff0c;RTSP本身并不发…
阅读更多...
使用javascript实现对于chineseocr的API调用

使用javascript实现对于chineseocr的API调用

ChineseOCR在线API 网页地址 界面 提供多种接口调用方式&#xff0c;比如在线调用、Javascript api调用、curl api调用和python api调用四种方式&#xff0c;本次使用javascript api调用的方式进行OCR识别在线Javascript工具 在线工具网页链接在线Base64 转化工具 在线工具…
阅读更多...
移动流媒体业务的技术与标准

移动流媒体业务的技术与标准

1 引言 　　流媒体业务是从Internet上发展起来的一种多媒体应用&#xff0c;指使用流&#xff08;Streaming&#xff09;方式在网络上传输的多媒体文件&#xff0c;包括音频、视频和动画等。 　　流媒体传输技术的主要特点是以流&#xff08;streaming&#xff09;的形式进行多…
阅读更多...
使用python实现对于chineseocr的API调用

使用python实现对于chineseocr的API调用

ChineseOCR在线API 网页链接 界面 提供多种接口调用方式&#xff0c;比如在线调用、Javascript api调用、curl api调用和python api调用四种方式&#xff0c;本次使用javascript api调用的方式进行OCR识别在线Base64 转化工具 Base64在线小工具代码修改 新增一个变量fill_w…
阅读更多...
算法入门篇十 图

算法入门篇十 图

图的存储方式 临接表临接矩阵 表达 点集/边集有向图/无向图 Graph&#xff08;大结构就是图&#xff09;&#xff08;包含点集合和边集合&#xff09; import java.util.HashMap; import java.util.HashSet;public class Graph {public HashMap<Integer, Node> nodes;…
阅读更多...
超文本传输协议

超文本传输协议

超文本传输协议 超文本传输协议超文件传输协定(HTTP&#xff0c;HyperTextTransfer Protocol)是因特网上应用最为广泛的一种网络传输协定。所有的WWW文件都必须遵守这个标准。设计HTTP最初的目的是为了提供一种发布和接收HTML页面的方法。 目录 介绍请求信息请求方法安全方法超…
阅读更多...
利用MFC调用libvlc.dll作一个简单的播放器

利用MFC调用libvlc.dll作一个简单的播放器

简单介绍MFC调用libvlc.dll作一个简单的播放器&#xff0c;抛砖引玉&#xff0c;各位VC达人继续深入研究&#xff0c;Jeremiah对VC确实不太感兴趣&#xff0c;所以就不做太深入的研究了。2009.10.29修改&#xff1a;加入clip_children属性设置。参开第1步。环境&#xff1a; …
阅读更多...
使用Remix编写Solidity语言的小例子

使用Remix编写Solidity语言的小例子

设置数值/取数值/加法运算 讲解 uint默认使用256位数的整型view表示这个函数仅仅对于数据仅仅是读取&#xff0c;没有修改操作returns(uint )&#xff0c;如果单纯指定uint&#xff0c;返回的是函数体内的return值&#xff0c;如果包含uint sum,uint SAD_a&#xff0c;那么返…
阅读更多...
RTP协议栈简介

RTP协议栈简介

流媒体指的是在网络中使用流技术传输的连续时基媒体&#xff0c;其特点是在播放前不需要下载整个文件&#xff0c;而是采用边下载边播放的方式&#xff0c;它是视频会议、IP电话等应用场合的技术基础。RTP是进行实时流媒体传输的标准协议和关键技术&#xff0c;本文介绍如何在L…
阅读更多...
使用多线程的方式调用chineseocr_API

使用多线程的方式调用chineseocr_API

ChineseOCR在线API 网页链接 界面 提供多种接口调用方式&#xff0c;比如在线调用、Javascript api调用、curl api调用和python api调用四种方式&#xff0c;本次使用javascript api调用的方式进行OCR识别代码 import glob import base64 import os import requests import …
阅读更多...
开源好代码 音视频

开源好代码 音视频

VirtualDub 一、简介 图1VirtualDub主界面 VirtualDub是一款开源的音视频捕获、处理软件。VirtualDub也可称为一款多媒体编辑软件&#xff0c;因为它包含了多媒体输入、编辑、处理、输出等各个环节&#xff0c;但是作者并未将它定位为一款多媒体编辑软件&#xff08;参见官网&a…
阅读更多...
深入理解Solidity 二

深入理解Solidity 二

Solidity数据位置 所有复杂的数据类型&#xff0c;即数组、结构和映射类型&#xff0c;都会有一个额外属性“数据位置”&#xff0c;用来指定数据的存储位置&#xff0c;即数据是存储在memory还是存储在storage里面根据上下文环境&#xff0c;IDE会自动指定数据的默认存储位置…
阅读更多...
VOIP简介

VOIP简介

一、什么是VOIP VOIP全称为&#xff08;VoiceOver Internet Protocol&#xff09;&#xff0c;是一种利用Internet网络进行语音通信的技术&#xff0c;更通俗一点说&#xff0c;就是IP电话。就是以IP分组交换网为传输平台&#xff0c;对模拟的语音信号进行编码压缩&#xff0c…
阅读更多...
深入理解Solidity 三

深入理解Solidity 三

Solidity函数声明和类型 函数的值类型有两类&#xff1a;内部&#xff08;internal&#xff09;类型和外部&#xff08;external&#xff09;类型内部函数只可以在当前合约内部被调用&#xff08;即在当前代码块内&#xff0c;包括内部库函数和继承函数&#xff09;&#xff0c…
阅读更多...
安装solc模块4.25版本

安装solc模块4.25版本

使用国产阿里云的cnpm 如果不知道cnpm 参考链接 安装solc模块4.25版本 npm i solc0.4.25 --save -g查看安装是否成功 可以配置软连接使用solc&#xff0c;我的没有配置 solcjs --version
阅读更多...
在pycharm中使用conda虚拟环境（conda虚拟环境是已经创建好的),解决python安装包文件很费劲的问题

在pycharm中使用conda虚拟环境（conda虚拟环境是已经创建好的),解决python安装包文件很费劲的问题

查看conda的虚拟环境 使用PyCharm连接conda创建的虚拟环境&#xff0c;需要一个前提就是虚拟环境必须存在&#xff0c;使用conda env list命令查看虚拟环境列表打开PyCharm软件 打开pycharm&#xff0c;选择File->setting->Project:****->Project Interperter&#…
阅读更多...
完成一个H.265/HEVC码流分析工具

完成一个H.265/HEVC码流分析工具

经过大约一个月左右的业余时间&#xff0c;终于初步完成一个H.265/HEVC码流分析工具。时间包括平时的周末、晚上&#xff0c;以及调休的集中时间。当然&#xff0c;中秋回家过节不写代码。截至今天&#xff0c;经过多种H.265序列测试&#xff0c;也有各种工具对比&#xff0c;基…
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023