akka-stream的Graph是一种运算方案,它可能代表某种简单的线性数据流图如:Source/Flow/Sink,也可能是由更基础的流图组合而成相对复杂点的某种复合流图,而这个复合流图本身又可以被当作组件来组合更大的Graph。因为Graph只是对数据流运算的描述,所以它是可以被重复利用的。所以我们应该尽量地按照业务流程需要来设计构建Graph。在更高的功能层面上实现Graph的模块化(modular)。按上回讨论,Graph又可以被描述成一种黑盒子,它的入口和出口就是Shape,而内部的作用即处理步骤Stage则是用GraphStage来形容的。下面是akka-stream预设的一些基础数据流图:
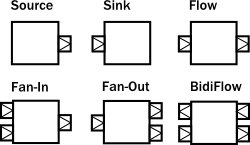
上面Source,Sink,Flow代表具备线性步骤linear-stage的流图,属于最基础的组件,可以用来构建数据处理链条。而Fan-In合并型,Fan-Out扩散型则具备多个输入或输出端口,可以用来构建更复杂的数据流图。我们可以用以上这些基础Graph来构建更复杂的复合流图,而这些复合流图又可以被重复利用去构建更复杂的复合流图。下面就是一些常见的复合流图:
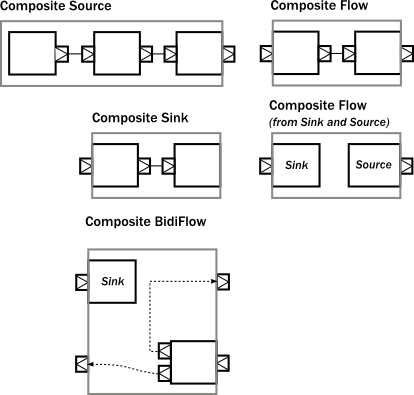
注意上面的Composite Flow(from Sink and Source)可以用Flow.fromSinkAndSource函数构建:
def fromSinkAndSource[I, O](sink: Graph[SinkShape[I], _], source: Graph[SourceShape[O], _]): Flow[I, O, NotUsed] =fromSinkAndSourceMat(sink, source)(Keep.none) 这个Flow从流向来说先Sink再Source是反的,形成的Flow上下游间无法协调,即Source端终结信号无法到达Sink端,因为这两端是相互独立的。我们必须用CoupledTermination对象中的fromSinkAndSource函数构建的Flow来解决这个问题:
/*** Allows coupling termination (cancellation, completion, erroring) of Sinks and Sources while creating a Flow them them.* Similar to `Flow.fromSinkAndSource` however that API does not connect the completion signals of the wrapped stages.*/
object CoupledTerminationFlow {@deprecated("Use `Flow.fromSinkAndSourceCoupledMat(..., ...)(Keep.both)` instead", "2.5.2")def fromSinkAndSource[I, O, M1, M2](in: Sink[I, M1], out: Source[O, M2]): Flow[I, O, (M1, M2)] =Flow.fromSinkAndSourceCoupledMat(in, out)(Keep.both)
从上面图列里的Composite BidiFlow可以看出:一个复合Graph的内部可以是很复杂的,但从外面看到的只是简单的几个输入输出端口。不过Graph内部构件之间的端口必须按照功能逻辑进行正确的连接,剩下的就变成直接向外公开的界面端口了。这种机制支持了层级式的模块化组合方式,如下面的图示:
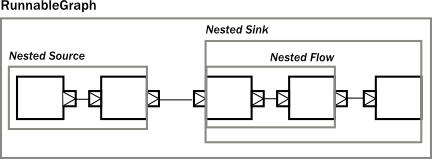
最后变成:
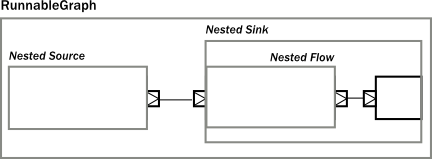
在DSL里我们可以用name("???")来分割模块:
val nestedFlow =Flow[Int].filter(_ != 0) // an atomic processing stage.map(_ - 2) // another atomic processing stage.named("nestedFlow") // wraps up the Flow, and gives it a name val nestedSink =nestedFlow.to(Sink.fold(0)(_ + _)) // wire an atomic sink to the nestedFlow.named("nestedSink") // wrap it up// Create a RunnableGraph val runnableGraph = nestedSource.to(nestedSink)
在下面这个示范里我们自定义一个某种功能的流图模块:它有2个输入和3个输出。然后我们再使用这个自定义流图模块组建一个完整的闭合流图:
import akka.actor._ import akka.stream._ import akka.stream.scaladsl._import scala.collection.immutableobject GraphModules {def someProcess[I, O]: I => O = i => i.asInstanceOf[O]case class TwoThreeShape[I, I2, O, O2, O3](in1: Inlet[I],in2: Inlet[I2],out1: Outlet[O],out2: Outlet[O2],out3: Outlet[O3]) extends Shape {override def inlets: immutable.Seq[Inlet[_]] = in1 :: in2 :: Niloverride def outlets: immutable.Seq[Outlet[_]] = out1 :: out2 :: out3 :: Niloverride def deepCopy(): Shape = TwoThreeShape(in1.carbonCopy(),in2.carbonCopy(),out1.carbonCopy(),out2.carbonCopy(),out3.carbonCopy())} //a functional module with 2 input 3 outputdef TwoThreeGraph[I, I2, O, O2, O3] = GraphDSL.create() { implicit builder =>val balancer = builder.add(Balance[I](2))val flow = builder.add(Flow[I2].map(someProcess[I2, O2]))TwoThreeShape(balancer.in, flow.in, balancer.out(0), balancer.out(1), flow.out)}val closedGraph = GraphDSL.create() {implicit builder =>import GraphDSL.Implicits._val inp1 = builder.add(Source(List(1,2,3))).outval inp2 = builder.add(Source(List(10,20,30))).outval merge = builder.add(Merge[Int](2))val mod23 = builder.add(TwoThreeGraph[Int,Int,Int,Int,Int])inp1 ~> mod23.in1inp2 ~> mod23.in2mod23.out1 ~> merge.in(0)mod23.out2 ~> merge.in(1)mod23.out3 ~> Sink.foreach(println)merge ~> Sink.foreach(println)ClosedShape} }object TailorGraph extends App {import GraphModules._implicit val sys = ActorSystem("streamSys")implicit val ec = sys.dispatcherimplicit val mat = ActorMaterializer()RunnableGraph.fromGraph(closedGraph).run()scala.io.StdIn.readLine()sys.terminate()}
这个自定义的TwoThreeGraph是一个复合的流图模块,是可以重复使用的。注意这个~>符合的使用:akka-stream只提供了对预设定Shape作为连接对象的支持如:
def ~>[Out](junction: UniformFanInShape[T, Out])(implicit b: Builder[_]): PortOps[Out] = {...}def ~>[Out](junction: UniformFanOutShape[T, Out])(implicit b: Builder[_]): PortOps[Out] = {...}def ~>[Out](flow: FlowShape[T, Out])(implicit b: Builder[_]): PortOps[Out] = {...}def ~>(to: Graph[SinkShape[T], _])(implicit b: Builder[_]): Unit =b.addEdge(importAndGetPort(b), b.add(to).in)def ~>(to: SinkShape[T])(implicit b: Builder[_]): Unit =b.addEdge(importAndGetPort(b), to.in)
... 所以对于我们自定义的TwoThreeShape就只能使用直接的端口连接了:
def ~>[U >: T](to: Inlet[U])(implicit b: Builder[_]): Unit =b.addEdge(importAndGetPort(b), to) 以上的过程显示:通过akka的GraphDSL,对复合型Graph的构建可以实现形象化,大部分工作都在如何对组件之间的端口进行连接。我们再来看个较复杂复合流图的构建过程,下面是这个流图的图示:
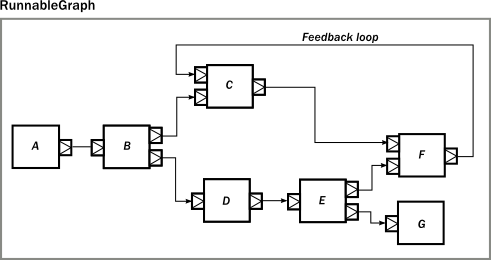
可以说这是一个相对复杂的数据处理方案,里面甚至包括了数据流回路(feedback)。无法想象如果用纯函数数据流如scalaz-stream应该怎样去实现这么复杂的流程,也可能根本是没有解决方案的。但用akka GraphDSL可以很形象的组合这个数据流图;
import GraphDSL.Implicits._RunnableGraph.fromGraph(GraphDSL.create() { implicit builder =>val A: Outlet[Int] = builder.add(Source.single(0)).outval B: UniformFanOutShape[Int, Int] = builder.add(Broadcast[Int](2))val C: UniformFanInShape[Int, Int] = builder.add(Merge[Int](2))val D: FlowShape[Int, Int] = builder.add(Flow[Int].map(_ + 1))val E: UniformFanOutShape[Int, Int] = builder.add(Balance[Int](2))val F: UniformFanInShape[Int, Int] = builder.add(Merge[Int](2))val G: Inlet[Any] = builder.add(Sink.foreach(println)).inC <~ FA ~> B ~> C ~> FB ~> D ~> E ~> FE ~> GClosedShape}) 另一个端口连接方式的版本如下:
RunnableGraph.fromGraph(GraphDSL.create() { implicit builder =>val B = builder.add(Broadcast[Int](2))val C = builder.add(Merge[Int](2))val E = builder.add(Balance[Int](2))val F = builder.add(Merge[Int](2))Source.single(0) ~> B.in; B.out(0) ~> C.in(1); C.out ~> F.in(0)C.in(0) <~ F.outB.out(1).map(_ + 1) ~> E.in; E.out(0) ~> F.in(1)E.out(1) ~> Sink.foreach(println)ClosedShape
}) 如果把上面这个复杂的Graph切分成模块的话,其中一部分是这样的:
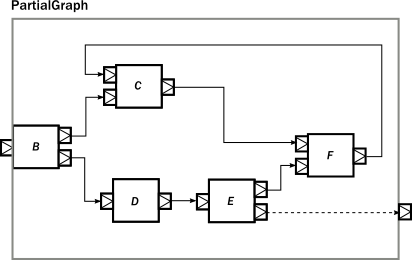
val partial = GraphDSL.create() { implicit builder =>val B = builder.add(Broadcast[Int](2))val C = builder.add(Merge[Int](2))val E = builder.add(Balance[Int](2))val F = builder.add(Merge[Int](2))C <~ FB ~> C ~> FB ~> Flow[Int].map(_ + 1) ~> E ~> FFlowShape(B.in, E.out(1))}.named("partial") 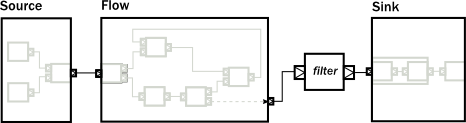
// Convert the partial graph of FlowShape to a Flow to get
// access to the fluid DSL (for example to be able to call .filter())
val flow = Flow.fromGraph(partial)// Simple way to create a graph backed Source
val source = Source.fromGraph( GraphDSL.create() { implicit builder =>val merge = builder.add(Merge[Int](2))Source.single(0) ~> mergeSource(List(2, 3, 4)) ~> merge// Exposing exactly one output portSourceShape(merge.out)
})// Building a Sink with a nested Flow, using the fluid DSL
val sink = {val nestedFlow = Flow[Int].map(_ * 2).drop(10).named("nestedFlow")nestedFlow.to(Sink.head)
}// Putting all together
val closed = source.via(flow.filter(_ > 1)).to(sink) 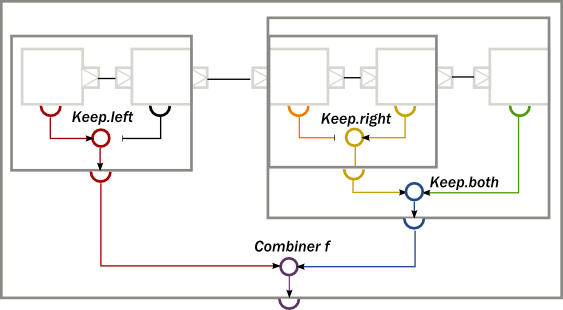
返回运算结果是通过viaMat, toMat来实现的。简写的via,to默认选择流图左边运算产生的结果。
(C语言实现))


(C语言实现))

)








(C语言实现))
之算法基础)

)

