[Leetcode][第98 450 700 701题][JAVA][二叉搜索树的合法性、增、删、查][递归][深度遍历]

【二叉搜索树定义】（BST）

二叉搜索树（Binary Search Tree，简称 BST）是一种很常用的的二叉树。它的定义是：一个二叉树中，任意节点的值要大于等于左子树所有节点的值，且要小于等于右边子树的所有节点的值。
在这里插入图片描述

【二叉树算法框架】

void traverse(TreeNode root) {// root 需要做什么？在这做。// 其他的不用 root 操心，抛给框架traverse(root.left);traverse(root.right);
}

【二叉搜索树算法框架】

void BST(TreeNode root, int target) {if (root.val == target)// 找到目标，做点什么if (root.val < target) BST(root.right, target);if (root.val > target)BST(root.left, target);
}

【问题描述】

实现 BST 的基础操作：判断 BST 的合法性、增、删、查。

【解答思路】

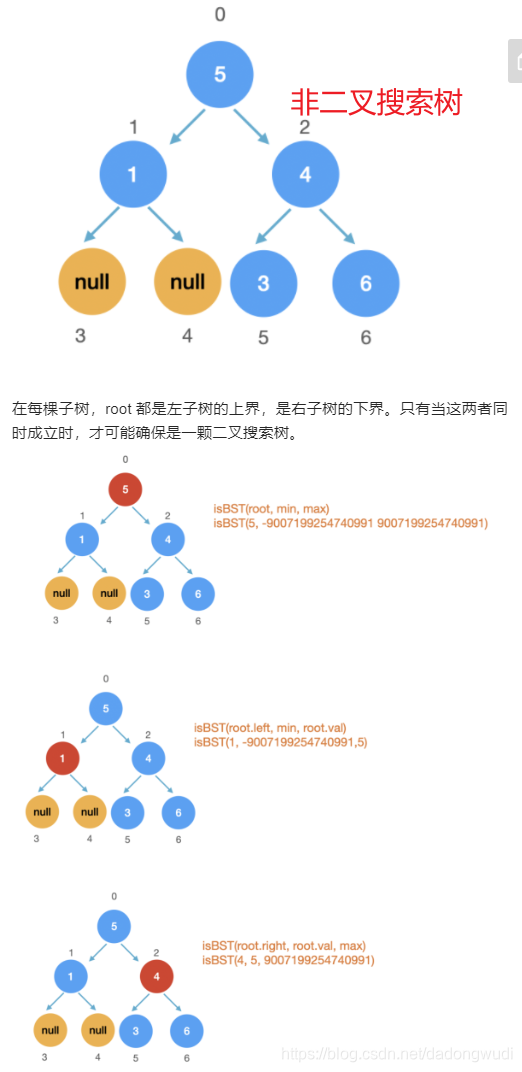
在这里插入图片描述

1. 判断 BST 的合法性

root 需要做的不只是和左右子节点比较，而是要整个左子树和右子树所有节点比较。

boolean isValidBST(TreeNode root) {return isValidBST(root, null, null);
}boolean isValidBST(TreeNode root, TreeNode min, TreeNode max) {if (root == null) return true;if (min != null && root.val <= min.val) return false;if (max != null && root.val >= max.val) return false;return isValidBST(root.left, min, root) && isValidBST(root.right, root, max);
}

2. 在 BST 中查找一个数是否存在

框架

boolean isInBST(TreeNode root, int target) {if (root == null) return false;if (root.val == target) return true;return isInBST(root.left, target)|| isInBST(root.right, target);
}

利用特性

boolean isInBST(TreeNode root, int target) {if (root == null) return false;if (root.val == target)return true;if (root.val < target) return isInBST(root.right, target);if (root.val > target)return isInBST(root.left, target);// root 该做的事做完了，顺带把框架也完成了，妙
}

3. 在 BST 中插入一个数

对数据结构的操作无非遍历 + 访问，遍历就是“找”，访问就是“改”。具体到这个问题，插入一个数，就是先找到插入位置，然后进行插入操作。
直接套BST 中的遍历框架，加上“改”的操作即可。一旦涉及“改”，函数就要返回 TreeNode 类型，并且对递归调用的返回值进行接收。

void BST(TreeNode root, int target) {if (root.val == target)// 找到目标，做点什么if (root.val < target) BST(root.right, target);if (root.val > target)BST(root.left, target);
}

4. 在 BST 中删除一个数

在这里插入图片描述

TreeNode deleteNode(TreeNode root, int key) {if (root == null) return null;if (root.val == key) {// 这两个 if 把情况 1 和 2 都正确处理了if (root.left == null) return root.right;if (root.right == null) return root.left;// 处理情况 3TreeNode minNode = getMin(root.right);root.val = minNode.val;root.right = deleteNode(root.right, minNode.val);} else if (root.val > key) {root.left = deleteNode(root.left, key);} else if (root.val < key) {root.right = deleteNode(root.right, key);}return root;
}TreeNode getMin(TreeNode node) {// BST 最左边的就是最小的while (node.left != null) node = node.left;return node;
}

注意一下，这个删除操作并不完美，因为我们一般不会通过 root.val = minNode.val 修改节点内部的值来交换节点，而是通过一系列略微复杂的链表操作交换 root 和 minNode 两个节点。因为具体应用中，val 域可能会很大，修改起来很耗时，而链表操作无非改一改指针，而不会去碰内部数据。

【总结】

1. 二叉树算法设计的总路线：把当前节点要做的事做好，其他的交给递归框架，不用当前节点操心。

2.如果当前节点会对下面的子节点有整体影响，可以通过辅助函数增长参数列表，借助参数传递信息。

3.在二叉树框架之上，扩展出一套 BST 遍历框架

void BST(TreeNode root, int target) {if (root.val == target)// 找到目标，做点什么if (root.val < target) BST(root.right, target);if (root.val > target)BST(root.left, target);
}