T1 [JZOJ2642] 游戏
题目描述
Alice和Bob在玩一个游戏,游戏是在一个N*N的矩阵上进行的,每个格子上都有一个正整数。当轮到Alice/Bob时,他/她可以选择最后一列或最后一行,并将其删除,但必须保证选择的这一行或这一列所有数的和为偶数。如果他/她不能删除最后一行或最后一列,那么他/她就输了。两人都用最优策略来玩游戏,Alice先手,问Alice是否可以必胜?
分析
这个说辞...一看就知道是博弈论
众所周知,博弈论有两个重要结论:
1.一个状态是必败状态当且仅当它任意后继都是必胜状态
2.一个状态是必胜状态当且仅当它存在后继是必败状态
于是设 $f[i][j]$ 为矩阵为 $i$ 行 $j$ 列时该回合操作方的状态($1$ 为必胜,$0$ 为必败),显然 $f[1][1]=1$
同时需要将 $f[1][i]$ 和 $f[i][1]$ 初始化,还要记录所有横轴和纵轴的前缀和
然后分别讨论删除最后一行和最后一列时的后继状态,若该行或该列无法被删除,则该后继视为必胜
考场上写这题的时候已经不早了,感觉有点慌,幸好最后过了


#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <cmath>
#include <queue>
using namespace std;
#define N 1005int T, n;
int g[N][N], f[N][N], p1[N][N], p2[N][N];int main() {scanf("%d", &T);while (T--) {scanf("%d", &n);for (int i = 1; i <= n; i++)for (int j = 1; j <= n; j++) {scanf("%d", &g[i][j]);p1[i][j] = p1[i][j - 1] + g[i][j];p2[i][j] = p2[i - 1][j] + g[i][j];}f[1][1] = 1;for (int i = 2; i <= n; i++) {int t1, t2;if (p1[1][i] % 2) t1 = 1;else t1 = 0;if (p2[1][i] % 2) t2 = 1;else if (f[1][i - 1]) t2 = 1;else t2 = 0;if (t1 && t2) f[1][i] = 0;else f[1][i] = 1;}for (int i = 2; i <= n; i++) {int t1, t2;if (p2[i][1] % 2) t2 = 1;else t2 = 0;if (p2[i][1] % 2) t1 = 1;else if (f[i - 1][1]) t1 = 1;else t1 = 0;if (t1 && t2) f[1][i] = 0;else f[i][1] = 1;}for (int i = 2; i <= n; i++)for (int j = 2; j <= n; j++) {int t1, t2;if (p1[i][j] % 2) t1 = 1;else if (f[i - 1][j]) t1 = 1;else t1 = 0;if (p2[i][j] % 2) t2 = 1;else if (f[i][j - 1]) t2 = 1;else t2 = 0;if (t1 && t2) f[i][j] = 0;else f[i][j] = 1;}if (f[n][n]) printf("W\n");else printf("L\n");}return 0;
} T2 [JZOJ2643] 六边形
题目描述
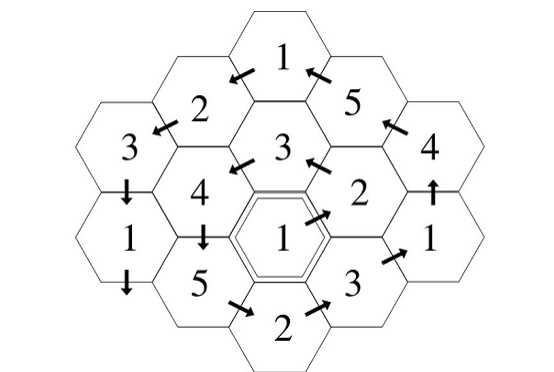
棋盘是由许多个六边形构成的,共有5种不同的六边形编号为1到5,棋盘的生成规则如下:
1.从中心的一个六边形开始,逆时针向外生成一个个六边形。
2.对于刚生成的一个六边形,我们要确定它的种类,它的种类必须满足与已生成的相邻的六边形不同。
3.如果有多个种类可以选,我们选择出现次数最少的种类。
4.情况3下还有多个种类可以选,我们选择数字编号最小的。
现在要你求第N个生成的六边形的编号?
前14个六边形生成图如下:
分析
这是个纯模拟,感觉没有什么要分析的
主要就是要多注意细节,考场上少写了一句代码,直接掉到了 $45.5$ 分
而且每次一写模拟就写得贼慢


//考场上写得有点繁琐 #include <iostream> #include <cstdio> #include <cstring> #include <algorithm> #include <cmath> #include <queue> using namespace std; #define ll long long #define inf 0x3f3f3f3f #define N 10005int T, n, c = 2, now = 8, s, e, ok, g1, g2; int q[25], g[N], book[6], sum[6];int main() {scanf("%d", &T);for (int i = 1; i <= T; i++) {scanf("%d", q + i);n = max(n, q[i]);}g[1] = 1; g[2] = 2; g[3] = 3;g[4] = 4; g[5] = 5; g[6] = 2; g[7] = 3;sum[1] = sum[4] = sum[5] = 1;sum[2] = sum[3] = 2; sum[0] = inf;s = 2; e = 7;while (++c) {for (int i = 1; i <= 6; i++) {for (int j = 1; j < c; j++) {memset(book, 0, sizeof book);int minsum = inf;if (j != c - 1) {if (now == e + 1) {book[g[s]] = book[g[e]] = 1;for (int k = 1; k <= 5; k++)if (!book[k])minsum = min(minsum, sum[k]);for (int k = 1; k <= 5; k++)if (!book[k] && sum[k] == minsum) {g[now++] = k; sum[k]++; break;}g1 = s; g2 = s + 1; s = e + 1;}else {book[g[g1]] = book[g[g2]] = book[g[now - 1]] = 1;for (int k = 1; k <= 5; k++)if (!book[k])minsum = min(minsum, sum[k]);for (int k = 1; k <= 5; k++)if (!book[k] && sum[k] == minsum) {g[now++] = k; sum[k]++; break;}g1++; g2++;}}else {if (i == 6) e = now, book[g[s]] = 1;book[g[g1]] = book[g[now - 1]] = 1;for (int k = 1; k <= 5; k++)if (!book[k])minsum = min(minsum, sum[k]);for (int k = 1; k <= 5; k++)if (!book[k] && sum[k] == minsum) {g[now++] = k; sum[k]++; break;}}if (now > n) {ok = 1; break;}}if (ok) break;}if (ok) break;}for (int i = 1; i <= T; i++)printf("%d\n", g[q[i]]);return 0; }
T3 [JZOJ2644] 数列
题目描述
给你一个长度为N的正整数序列,如果一个连续的子序列,子序列的和能够被K整除,那么就视此子序列合法,求原序列包括多少个合法的连续子序列?
对于一个长度为8的序列,K=4的情况:2, 1, 2, 1, 1, 2, 1, 2 。它的答案为6,子序列是位置1->位置8,2->4,2->7,3->5,4->6,5->7。
分析
看到题目就先写了前缀和枚举区间 $O(n^2)$ 暴力 $30 \, pts$
当时看了半天觉得这是最可做的一题,结果看了数据范围还是没想出来 $O(n \, log \, n)$ 做法
结果考完试下午看了下大家的讨论,发现正解是 $O(k)$
具体就是把每个前缀和按 $k$ 取模,记录每个余数出现的次数 $sum$
显然,前缀和所得余数相同的的两项之间的区间和,一定能被 $k$ 整除
所以在余数相同的项中,我们可以任意挑选两项组成一个合法区间
因此答案为 $\sum\limits_{i=0}^{k-1} \binom{sum[i]}{2}$
要注意,第 $0$ 项的前缀和余数视为 $0$


#include <iostream> #include <cstdio> #include <cstring> #include <algorithm> #include <cmath> #include <queue> using namespace std; #define ll long long #define N 50005 #define K 1000005int T, n, k, x; int pre[N], sum[K]; ll ans, c[K];int main() {c[2] = 1;for (int i = 3; i <= N; i++)c[i] = c[i - 1] + i - 1;scanf("%d", &T);while (T--) {ans = 0;scanf("%d%d", &k, &n);for (int i = 1; i <= k; i++) sum[i] = 0;sum[0] = 1;for (int i = 1; i <= n; i++) {scanf("%d", &x);pre[i] = (pre[i - 1] + x) % k;sum[pre[i]]++;}for (int i = 0; i < k; i++)ans += c[sum[i]];printf("%lld\n", ans);}return 0; }






和splice())和js引用的三种方法总结—2019年1月16日...)
)

)








)
