最短公共子序列_最短公共超序列

最短公共子序列

Problem statement:

问题陈述：

Given two strings, you have to find the shortest common super sequence between them and print the length of the super sequence.

给定两个字符串，您必须找到它们之间最短的公共超级序列，并打印超级序列的长度。

    Input:
T Test case
T no of input string will be given to you.
E.g.
3
abcd abxy
sghk rfgh
svd vjhfd
Constrain 
1≤ length (string1) ≤100
1≤ length (string2) ≤100
Output:
Print the length of the shortest super sequence formed from these two strings.

Example

例

    T=3
Input:
abcd abxy
Output:
6 (abcdxy)
Input:
sghk rfgh
Output:
6 (srfghk)
Input:
svd vjhfd
Output:
6 (svjhfd)

Explanation with example:

举例说明：

Let there are two strings str1 and str2.

假设有两个字符串str1和str2 。

    str1 = "abcd" 
str2 = "abxy"

To find out the length of the super sequence from these two string is a bit difficult. To solve this problem we have to follow these steps:

从这两个字符串中找出超级序列的长度有些困难。要解决此问题，我们必须遵循以下步骤：

We have to find out the longest common subsequence between the two strings. From "abcd" and "abxy" the longest common subsequence is "ab".
我们必须找出两个字符串之间最长的公共子序列。从“ abcd”和“ abxy”开始，最长的公共子序列是“ ab”。
After getting the longest common subsequence we will add the non-common elements with the longest common subsequence. Non-common characters from "abcd" is "cd" and from "abxy" is "xy".
得到最长的公共子序列后，我们将添加具有最长的公共子序列的非公共元素。来自“ abcd”的非常见字符为“ cd”，来自“ abxy”的非常见字符为“ xy”。

Therefore the length of the shortest common super sequence is:

因此，最短公共超序列的长度为：

    length of the two strings-length of the longest common subsequencs

The length of the shortest common super sequence is

6 (abcdxy) 6(abcdxy)

Using a dynamic programming algorithm to find out the longest common subsequence between two given string is very efficient and fast as compared to the recursion approach.

与递归方法相比，使用动态编程算法找出两个给定字符串之间最长的公共子序列非常有效且快速。

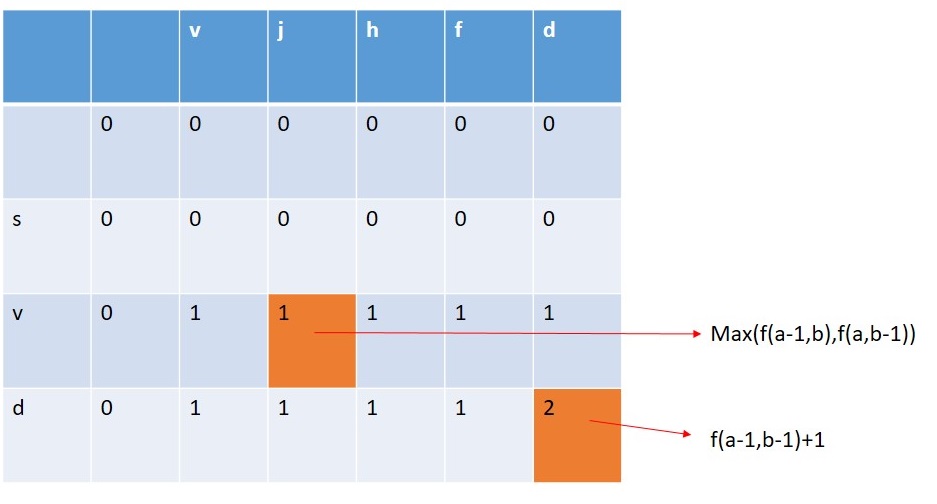
Let f(a,b) = count the number of common subsequences from the two string starting from 0 to position a and starting from 0 to position b.

令f(a，b) =计算两个字符串中从0开始到位置a以及从0开始到位置b的公共子序列数。

Considering the two facts:

考虑到两个事实：

If the character of string1 at index a and character of string1 at index b are the same then we have to count how many characters are the same between the two strings before these indexes? Therefore,
如果索引a的字符串 1的字符和索引b的字符串 1的字符相同，那么我们必须计算在这些索引之前两个字符串之间有多少个相同的字符？因此，
```
    f(a,b)=f(a-1,b-1)+1
```
If the character of string1 at index a and character of string1 at index b are not same then we have to calculate the maximum common character count between (0 to a-1 of string1 and 0 to b of string2) and (0 to a of string1 and 0 to b-1 of string2).
如果字符串 2中的索引和字符串1的字符索引b中的符不相同，则我们来计算之间的最大公共字符计数(0到字符串1的A-1和0至b 字符串2)和(0至a的string1和0到string2的 b-1 )。
```
    f(a,b)=max⁡(⁡(f(a-1,b),f(a,b-1))
```

For the two strings:

对于两个字符串：

    str1 = "abcd"
str2 = "abxy"

Problem solution:

问题方案：

Recursive algorithm:

递归算法：

    int Function(str1,pos1,str2,pos2):
if pos1>=str1.length() || pos2>=str2.length()
return 0
for a=pos1 to 0 
for b=pos1 to 0
if str[a]==str[a+l-1]
return Function(str1,a-1,str2,b-1)+1
else
return max(Function(str1,a,str2,b-1),Function(str1,a-1,str2,b));

DP conversion:

DP转换：

    int Function(str1,str2):
arr[len1+1][len2+1]
for len=1 to str1.length()
for a=1 to str2.length()
if str[a]==str[b]
arr[a][b]=arr[a-1][b-1]+1
else
arr[a][b]=max(arr[a][b-1],arr[a-1][b])
return arr[len1-1][len2-1]

C++ Implementation:

C ++实现：

#include <bits/stdc++.h>
using namespace std;
int count_common_subsequence(string str1, string str2)
{
int len1 = str1.length();
int len2 = str2.length();
int arr[len1 + 1][len2 + 1];
memset(arr, 0, sizeof(arr));
for (int i = 1; i <= len1; i++) {
for (int j = 1; j <= len2; j++) {
if (str1[i - 1] == str2[j - 1]) {
arr[i][j] = arr[i - 1][j - 1] + 1;
}
else {
arr[i][j] = max(arr[i - 1][j], arr[i][j - 1]);
}
}
}
return arr[len1][len2];
}
int main()
{
int t;
cout << "Test Case : ";
cin >> t;
while (t--) {
string str1, str2;
cout << "Enter the strings : ";
cin >> str1 >> str2;
cout << "Length of the longest common subsequence: " << count_common_subsequence(str1, str2) << endl;
}
return 0;
}

Output

输出量

Test Case : 3
Enter the strings : svd vjhfd
Length of the longest common subsequence: 2
Enter the strings : sghk rfgh
Length of the longest common subsequence: 2
Enter the strings : abcd abxy
Length of the longest common subsequence: 2