1.why:

数组&链表&树

 

 

2. 大纲

 

2.1前中后序

public class HeroNode {private int no;private String name;private  HeroNode left;//默认为nullprivate HeroNode right;//默认为nullpublic HeroNode(int no, String name) {this.no = no;this.name = name;}public int getNo() {return no;}public void setNo(int no) {this.no = no;}public String getName() {return name;}public void setName(String name) {this.name = name;}public HeroNode getLeft() {return left;}public void setLeft(HeroNode left) {this.left = left;}public HeroNode getRight() {return right;}public void setRight(HeroNode right) {this.right = right;}@Overridepublic String toString() {return "HeroNode{" +"no=" + no +", name='" + name + '\'' +'}';}//遍历//编写前序遍历方法,被谁调用this就是谁public void preOrder(){System.out.println(this);//先输出父节点//递归先左子树前遍历if(this.left!=null){this.left.preOrder();}//递归向右子树前序遍历if (this.right!=null){this.right.preOrder();}};//编写中序遍历方法public void infixOrder(){//递归先左子树前遍历if(this.left!=null){this.left.infixOrder();}//再输出父节点System.out.println(this);//递归向右子树前序遍历if (this.right!=null){this.right.infixOrder();}};//编写后序遍历方法public void postOrder(){//递归先左子树前遍历if(this.left!=null){this.left.postOrder();}//递归向右子树前序遍历if (this.right!=null){this.right.postOrder();}//最后输出父节点System.out.println(this);};}

2.2查找节点

 //查找//前序查找public HeroNode preSearch(int HeroNo){//判断当前节点是不是if (this.getNo()==HeroNo){return this;};//左子树前序查找//如果左递归前序查找节点，找到结点，则返回HeroNode resNode = null;//辅助节点if (this.getLeft()!=null) {resNode =this.getLeft().preSearch(HeroNo);}//resNode不为空才返回，因为右子树仍未查找if (resNode!=null){return resNode;}if (this.getRight()!=null){resNode = this.getRight().preSearch(HeroNo);}return resNode;}//中序查找public HeroNode infixSearch(int HeroNo){//左子树前序查找//如果左递归前序查找节点，找到结点，则返回HeroNode resNode = null;//辅助节点if (this.getLeft()!=null) {resNode =this.getLeft().preSearch(HeroNo);}//resNode不为空才返回，因为右子树仍未查找if (resNode!=null){return resNode;};//判断当前节点是不是if (this.getNo()==HeroNo){return this;}//查找右子树if (this.getRight()!=null){resNode = this.getRight().preSearch(HeroNo);}return resNode;}//后序查找public HeroNode postSearch(int HeroNo){//左子树前序查找//如果左递归前序查找节点，找到结点，则返回HeroNode resNode = null;//辅助节点if (this.getLeft()!=null) {resNode =this.getLeft().preSearch(HeroNo);}//resNode不为空才返回，因为右子树仍未查找if (resNode!=null){return resNode;};if (this.getRight()!=null){resNode = this.getRight().preSearch(HeroNo);}if (resNode!=null){return resNode;};//最后判断当前节点是不是if (this.getNo()==HeroNo){return this;};return resNode;}

2.3删除节点（基本） 

//删除public void deleteNode(int HeroNo){//判断左子节点if (this.left!=null && this.left.getNo()==HeroNo){this.left=null;return;}//判断右子节点if (this.right!=null&&this.right.getNo()==HeroNo){this.right=null;return;}//遍历左子树if (this.left!=null){this.left.deleteNode(HeroNo);}if(this.right!=null){this.right.deleteNode(HeroNo);}}

2.4二叉树 （root节点）

public class BinaryTree {//rootprivate HeroNode root;public void setRoot(HeroNode root) {this.root = root;};//遍历二叉树//前序遍历public void preOrder(){if (this.root!=null){this.root.preOrder();}else {System.out.println("二叉树为空");}}//中序遍历public void infixOrder(){if (this.root!=null){this.root.infixOrder();}else {System.out.println("二叉树为空");}}//后续遍历public void postOrder(){if (this.root!=null){this.root.postOrder();}else {System.out.println("二叉树为空");}}//查找二叉树指定节点//前序查找public HeroNode preSearch(int HeroNo){if (this.root!=null){return this.root.preSearch(HeroNo);}else {return null;}}//中序查找public HeroNode infixSearch(int HeroNo){if (this.root!=null){return this.root.infixSearch(HeroNo);}else {return null;}}//后序查找public HeroNode postSearch(int HeroNo){if (this.root!=null){return this.root.postSearch(HeroNo);}else {return null;}}public void delNode(int HeroNo){if(root!=null){if (root.getNo()==HeroNo){root=null;}else {root.deleteNode(HeroNo);}}else {System.out.println("空树，无法删除");}}
}

2.5顺序存储二叉树  （为完全二叉树，公式）

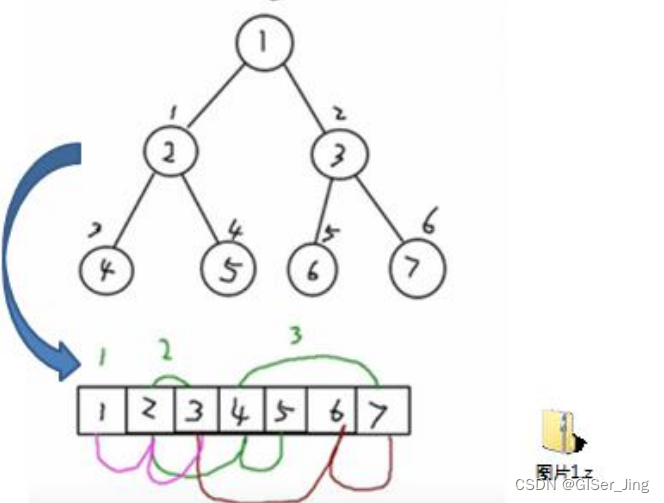
public class ArrBinaryTree {private int [] arr;//存储结点的数组public ArrBinaryTree(int[] arr) {this.arr = arr;}//重载public void preOrder(){preOrder(0);}public void infixOrder(){infixOrder(0);}/**** @param index  arr[index]=i*///前序public void preOrder(int index){if(arr == null ||arr.length==0){System.out.println("数组为空");}System.out.println(arr[index]);//向左递归遍历if ((index*2+1)<arr.length){preOrder(2*index+1);}//向右递归遍历if ((index*2+2)<arr.length){preOrder(2* index+2);}}//中序public void infixOrder(int index){if(arr == null ||arr.length==0){System.out.println("数组为空");}//向左递归遍历if ((index*2+1)<arr.length){infixOrder(index*2+1);}System.out.println(arr[index]);//向右递归遍历if ((index*2+2)<arr.length){infixOrder(2* index+2);}}
}

 2.6线索化二叉树（节点左右节点类型、前驱指针）

 

 

public class ThreadBinaryTree {private HeroNode root;//为了实现线索化，需要创建要给指向当前结点的前驱结点的指针// 在递归进行线索化时，pre总是保留前一个结点//pre指针private HeroNode pre =null;public HeroNode getRoot() {return root;}public HeroNode getPre() {return pre;}public void setPre(HeroNode pre) {this.pre = pre;}public void setRoot(HeroNode root) {this.root = root;};//重载threadNodes()public void threadedNodes(){this.threadedNodes(root);}/*多回顾*/
//    //中序线索化二叉树public void threadedNodes(HeroNode node){//递归找到最左节点，然后返回if (node == null) {return;}//先线索化左子树threadedNodes(node.getLeft());//线索化当前节点if (node.getLeft()==null){node.setLeft(pre);node.setLeftType(1);}//key！！！if (pre!=null&&pre.getRight()==null){pre.setRight(node);pre.setRightType(1);}pre=node;//线索化右子树threadedNodes(node.getRight());};//***提高：中序、后序***//遍历线索化二叉树public void threadedList(){HeroNode node = root;while (root!=null){while(node!=null){//循环的找到leftType ==1的结点，第一个找到就是8结点// 后面随着遍历而变化,因为当leftType==1时，说明该结点是按照线索化// 处理后的有效结点while (node.getLeftType()==0){node=node.getLeft();}System.out.println(node);while (node.getRightType()==1){node=node.getRight();System.out.println(node);}//替换这个遍历点（对于原本就有右结点的）!!!node=node.getRight();}}}//遍历二叉树//前序遍历public void preOrder(){if (this.root!=null){this.root.preOrder();}else {System.out.println("二叉树为空");}}//中序遍历public void infixOrder(){if (this.root!=null){this.root.infixOrder();}else {System.out.println("二叉树为空");}}//后续遍历public void postOrder(){if (this.root!=null){this.root.postOrder();}else {System.out.println("二叉树为空");}}//查找二叉树指定节点//前序查找public HeroNode preSearch(int HeroNo){if (this.root!=null){return this.root.preSearch(HeroNo);}else {return null;}}//中序查找public HeroNode infixSearch(int HeroNo){if (this.root!=null){return this.root.infixSearch(HeroNo);}else {return null;}}//后序查找public HeroNode postSearch(int HeroNo){if (this.root!=null){return this.root.postSearch(HeroNo);}else {return null;}}public void delNode(int HeroNo){if(root!=null){if (root.getNo()==HeroNo){root=null;}else {root.deleteNode(HeroNo);}}else {System.out.println("空树，无法删除");}}
}