一、DFS与BFS

1.1深度优先搜索(DFS)

DFS不具有最短性

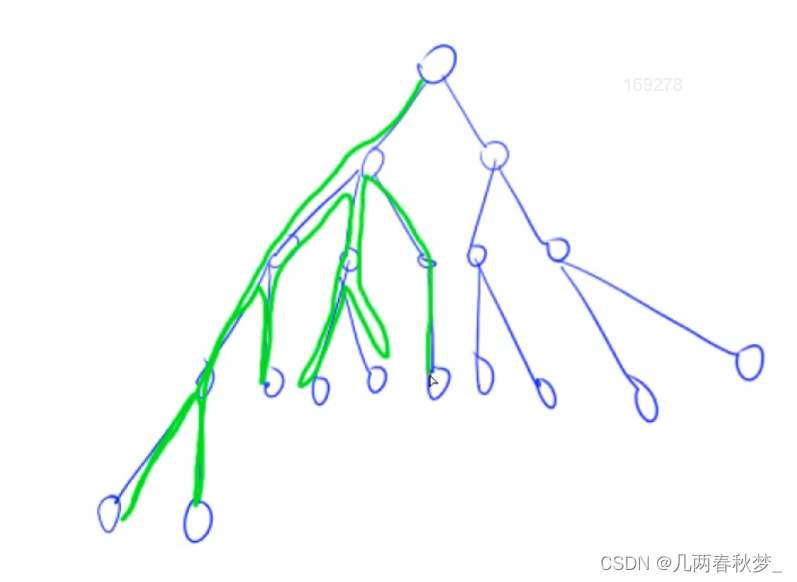
//排列数字问题
#include<iostream>
using namespace std;const int N = 10;
int n;
int path[N];
bool st[N];void dfs(int u)
{if(u == n){for(int i = 0;i < n;i++) printf("%d",path[i]);puts("");return;}for(int i =1;i <= n;i++){if(!st[i]){path[u] = i;st[i] = true;dfs(u + 1);st[i] = false;}}
}
int main()
{cin>>n;dfs(0);return 0;
}

1.2宽度优先搜索(BFS)

一层一层搜索，可以搜到最短路。

 

 

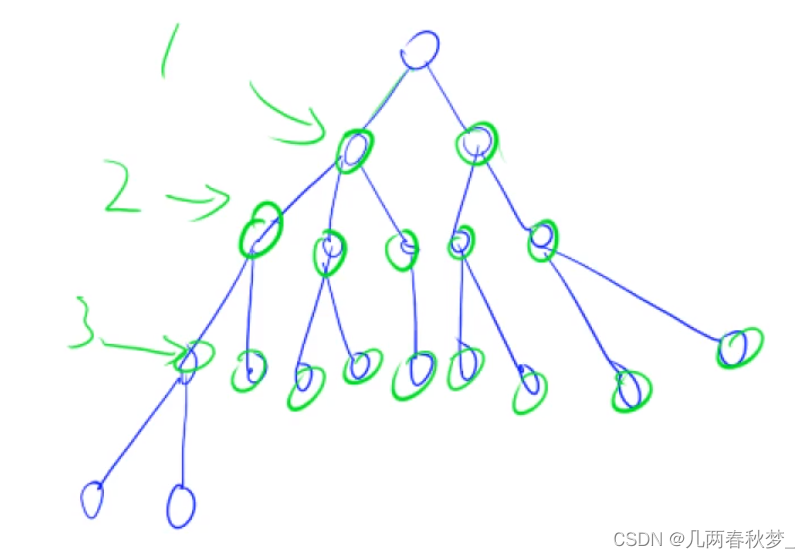
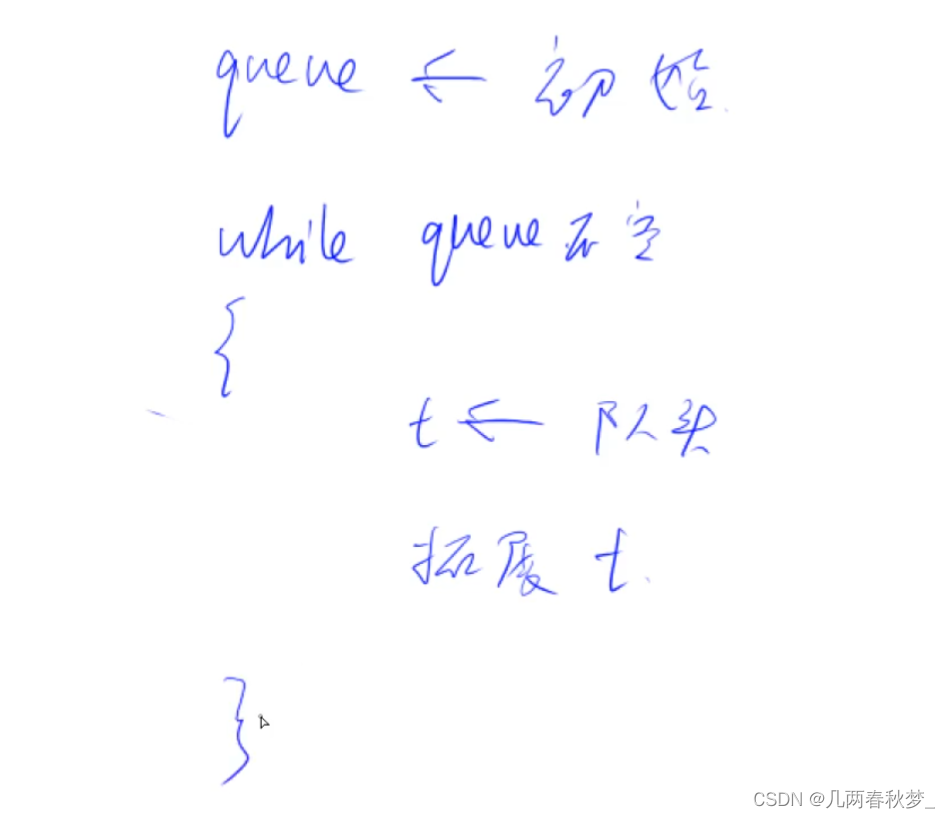
//走迷宫问题
#include<iostream>
#include<algorithm>
#include<queue>
#include<cstring>
using namespace std;typedef pair<int,int> PII;const int N = 110;int n,m;
int g[N][N];
int d[N][N];
PII q[N * N];int bfs()
{int hh = 0,tt = 0;q[0] = {0,0};//初始化为-1memset(d,-1,sizeof d);d[0][0] = 0;//定义头的向量int dx[4] = {-1,0,1,0},dy[4] = {0,1,0,-1};while(hh <= tt){auto t = q[hh ++];for(int  i = 0; i< 4;i++){int x = t.first + dx[i],y = t.second + dy[i];if(x >=0 && x < n && y >= 0 && y < m && g[x][y] ==0 && d[x][y] == -1){d[x][y] = d[t.first][t.second] + 1;q[++ tt] = {x,y};}}}return d[n - 1][m - 1];
}int main()
{cin>>n>>m;for(int  i = 0;i < n;i++)for(int j = 0;j < m;j++)cin>>g[i][j];cout<<bfs()<<endl;return 0;
}

二、树与图的遍历

2.1树与图的深度优先遍历

#include<iostream>
using namespace std;int n,m;
//h存的是n个链表的链表头
//e存的是所有的结点值
//ne存的是每个节点的next指针
int h[N],e[M],ne[M],idx;
bool st[N];void dfs(int u)
{stu[u] = true; //标记一下，已经被搜过了for(int i = h[u];i != -1;i = ne[i]){int j = e[i];if(!st[j]) dfs(j);}
}

2.2树与图的广度优先遍历

 

int n,m;
int h[N],e[N],ne[N],idx;
//d是距离,q是队列
int d[N],q[N];//插入函数
void add(int a,int b)
{e[idx] = b,ne[idx],ne[idx] = h[a],h[a] = idx++;
}
int bfs()
{//定义队头队尾int hh = 0,tt = 0;q[0] = 1;memset(d,-1,sizeof d);d[1] = 0;while(hh <= tt){int t = q[hh ++];for(int i = h[t];i != -1;i = ne[i]){int j = e[i];if(d[j] == -1){d[j] = d[t] + 1;q[++ tt] = j;}}}return d[n];
}

三、拓扑排序

适用于有向图

 

#include<cstring>
#include<iostream>
#include<algorithm>using namespace std;const int N = 100010;int n,m;
int h[N],e[N],ne[N],idx;
//q为队列,d为存储的入度
int q[N],d[N];void add(int a,int b)
{e[idx] = b,ne[idx] = h[a],h[a] = idx++;
}bool topsort()
{int hh = 0,tt = -1;for(int i =1;i <= n;i++){if(!d[i])q[++tt] = i;}while(hh <= tt){int t = q[hh++];for(int i = h[t];i!=-1;i = ne[i]){int j = e[i];d[j]--;if(d[j] == 0) q[++tt] = j;}}return tt == n-1;
}
int main()
{cin>>n>>m;memset(h,-1,sizeof h);for(int  i = 0;i < m;i++){int a,b;cin>>a>>b;add(a,b);}if(topsort()){for(int i =0;i < n;i++) printf("%d",q[i]);puts("");}else{puts("-1");}return 0;
}