1. 引言
前序博客有:
- SNARK原理示例
- SNARK性能及安全——Prover篇
- SNARK性能及安全——Verifier篇

上图摘自STARKs and STARK VM: Proofs of Computational Integrity。

上图选自:Dan Boneh 斯坦福大学 CS251 Fall 2023 Building a SNARK 课件。
SNARK方案由 Polynomial IOP(信息理论对象) ➕ 多项式承诺方案(密码学对象) 组成。
当前的Polynomial IOP主要分为三大类:
- 1)基于interactive proofs(IPs)的Polynomial IOP:如Hyrax、vSQL、Libra、Virgo等。【 P P P无需做FFT运算】
- 2)基于multi-prover interactive proofs(MIPs)的Polynomial IOP:如Spartan、Brakedown、Xiphos等。【 P P P无需做FFT运算】
- 3)基于constant-round的Polynomial IOP:如Marlin、PlonK、StarkWare的SNARKs等。【 P P P需要做FFT运算】
以上方案都是通过增加 P P P开销,来减少proof长度以及降低 V V V开销。
以上1)2)类,只要其结合的多项式承诺方案也不需要FFT,则 P P P无需做FFT运算。
当前的多项式承诺方案主要分为四大类:
- 1)基于pairing的多项式承诺方案(既不transparent,也不post-quantum)
- 如KZG10、PST13、ZGKPP18等。
- 独特属性有:具有constant sized evaluation proofs。
- 2)基于discrete logarithm的多项式承诺方案(transparent,但不post-quantum)
- 如BCCGP16、Bulletproofs、Hyrax、Dory等。【其中Dory即需要discret-log hardness,还需要pairing。】
- 3)基于IOPs+hashing(transparent 且 post-quantum)
- 如Ligero、FRI、Brakedown等。
- 4)基于Groups of unknown order的多项式承诺方案(若使用class groups具有transparent属性,但不是post-quantum的)
- 如DARK、Dew等。
- 由于使用class groups, P P P非常慢。
现有的多项式承诺方案有:
- KZG多项式承诺:PlonK和Marlin采用KZG多项式承诺。其既不是transparent的,也不是post-quantum secure的。2020年。
- Bulletproofs多项式承诺:为transparent的,但不是post-quantum secure的。2017年。
- Hyrax多项式承诺:为transparent的,但不是post-quantum secure的。2017年。
- Dory多项式承诺:为transparent的,但不是post-quantum secure的。2020年。
- FRI多项式承诺:为post-quantum secure的。2017年。基于纠错码。
- Ligero多项式承诺:为post-quantum secure的。2017年。基于纠错码。
- Ligero++多项式承诺:为post-quantum secure的。2020年。基于纠错码。
- Brakedown多项式承诺:为post-quantum secure的。2021年。基于纠错码。
- Orion多项式承诺:为post-quantum secure的。2022年。基于纠错码。
上面的5种post-quantum secure多项式承诺方案均是基于纠错码,使用的唯一密码学原语为哈希。同时具有如下属性:
- 验证开销随着bits of security的位数增加而增加。(所谓验证开销,是由 哈希evaluation数量以及field operation数量 来衡量的。)
粗略来说,这些post-quantum多项式承诺方案的单次“迭代”仅可实现小数量的bits of security(如2-4)。因此,需重复该协议多次来“放大”安全等级,而随着每次重复,验证开销也会随之增加。因此,控制PQ-SNARKs的验证开销(对应为区块链应用中的gas开销),协议设计者通常有动力将security level设置为较低值。
除极少数例外情况(见2018年论文 Batching Techniques for Accumulators with Applications to IOPs and Stateless Blockchains)外,Non-PQ-SNARK(透明和不透明)中不会出现具体安全和验证成本之间的紧张关系。Non-PQ-SNARK使用椭圆曲线群,其中离散对数被认为很难计算,其安全级别由所使用的群确定。椭圆曲线群的合适大小,以及每个群操作的成本,随着所需的安全级别而增长。然而,proof 中群元素的数量与群的选择无关。
而在PQ-SNARKs中,不仅hash evaluation的size会随着所需的安全等级增加而增加,proof中所需的evaluation数以及Verifier计算的 evaluation数也将随着所需安全等级增加而增加。
本文重点关注Transparent 且 Post-quantum zkSNARKs,在:
- libiop: a C++ library for IOP-based zkSNARKs(C++)【2021年5月后未更新】
中实现了几种仅依赖于lightweight symmetric cryptography(任意Cryptographic hash function)的 Transparent 且 Post-quantum zkSNARKs 方案:
- 1)Ligero协议:argument size为 O ( N ) O(\sqrt{N}) O(N),见2017年论文Ligero: Lightweight Sublinear Arguments Without a Trusted Setup。
- 2)Aurora协议:argument size为 O ( log 2 N ) O(\log ^2 N) O(log2N),见2018年论文Aurora: Transparent Succinct Arguments for R1CS。
- 3)Fractal协议:argument size为 O ( log 2 N ) O(\log ^2 N) O(log2N),见2019年论文FRACTAL: Post-Quantum and Transparent Recursive Proofs from Holography。
以上这3种Transparent 且 Post-quantum zkSNARKs 方案,均支持基于smooth prime fields和binary extension fields的R1CS。所谓R1CS,为一种NP-complete relation that generalizes arithmetic circuit satisfiability。
不同于Ligero,其中Aurora和Fractal中有额外有趣的点在于:
- FRI low-degree test:详情见Fast Reed-Solomon Interactive Oracle Proofs of Proximity。
2. 由IOPs 到 zkSNARKs
Interactive Oracle Proofs(IOPs):
- 为Probabilistically checkable proof 的multi-round generalization
- IOPs的性能,优于,PCPs
- libiop: a C++ library for IOP-based zkSNARKs库,通过Interactive Oracle Proofs(IOPs)(本文接下来按作者名,称为BCS transformation),实现了由IOPs构建的zkSNARKs。
BCS transformation:
- 使用cryptographic hash function(模型化为random oracle)
- 来将,任意public-coin IOP
- 编译为某SNARG
该SNARG具有如下属性:
- transparent:生成/验证proof strings所需的全局参数,仅为,该哈希函数
- post-quantum:在quantum random oracle模型内,其是安全的
- lightweight:除该哈希函数之外,无需其它密码学依赖
BCS transformation的描述见:
- Eli Ben-Sasson、Alessandro Chiesa 和 Nicholas Spooner 2016年论文 Interactive Oracle Proofs
BCS transformation的post-quantum安全性论证见:
- Alessandro Chiesa、Peter Manohar 和 Nicholas Spooner 2020年论文 Succinct Arguments in the Quantum Random Oracle Model
BCS transformation保持了proof of knowledge:
- 若底层的IOP是proof of knowledge,则所生成的SNARG为an argument of knowledge(即 a SNARK)
同理,BCS transformation保持了zero knowledge:
- 若底层IOP是(honest-verifier)zero knowledge,则所生成的SNARK是zero knowledge的(即 a zkSNARG)
同时,BCS transformation可扩展为:
- 向holographic IOPs,编译为,preprocessing SNARGs
- 详情见:Alessandro Chiesa、Dev Ojha 和 Nicholas Spooner 2019年论文 FRACTAL: Post-Quantum and Transparent Recursive Proofs from Holography
- 该特性,使得SNARGs可为,任意计算(而不仅仅是结构化计算),提供fast verification。
3. IOP协议
- https://github.com/scipr-lab/libiop/tree/master/libiop/iop:该目录下包含了编写IOP协议的基础设施。
- https://github.com/scipr-lab/libiop/tree/master/libiop/protocols:该目录下包含了几个使用该基础设施实现的协议,具体为:
| language | round complexity | oracle length (field elts) | query complexity | indexer time (field ops) | prover time (field ops) | verifier time (field ops) | |
|---|---|---|---|---|---|---|---|
| Ligero-IOP | R1CS | 2 | O(N) | O(N0.5) | N/A | O(N logN) | O(N) |
| Aurora-IOP | R1CS | O(log N) | O(N) | O(log N) | N/A | O(N logN) | O(N) |
| Fractal-IOP | R1CS | O(log N) | O(N) | O(log N) | O(N logN) | O(N logN) | O(log N) |
其中:
- 1)Ligero-IOP:见2017年论文Ligero: Lightweight Sublinear Arguments Without a Trusted Setup。
- 更准确来说,Ligero论文中仅描述了arithmetic circuits构建。而Aurora论文附录中解释了如何将,该arithmetic circuits构建,扩展为,支持R1CS。因此,实际在libiop: a C++ library for IOP-based zkSNARKs代码库中所实现的Ligero协议,借助了Aurora论文中的该扩展技术。
- 2)Aurora-IOP:见2018年论文Aurora: Transparent Succinct Arguments for R1CS。
- 3)Fractal-IOP:见2019年论文FRACTAL: Post-Quantum and Transparent Recursive Proofs from Holography。
其中比Ligero-IOP更高效,的,Aurora-IOP和Fractal-IOP,结合了2大元素:【详情见 2018年论文Aurora: Transparent Succinct Arguments for R1CS 】
- 1)RS-encoded IOP,即基于纠错码
- https://github.com/scipr-lab/libiop/tree/master/libiop/protocols/encoded:该目录下包含了RS-encoded IOPs,覆盖了Ligero、Aurora和Fractal协议核心的RS-encoded IOPs。
- 2)对RS code的proximity test
- https://github.com/scipr-lab/libiop/tree/master/libiop/protocols/ldt:该目录下包含了对RS code的proximity tests。其包含了:
- direct test:用于Ligero
- FRI protocol:用于Aurora和Fractal。
- https://github.com/scipr-lab/libiop/tree/master/libiop/protocols/ldt:该目录下包含了对RS code的proximity tests。其包含了:
4. BCS transformation
- https://github.com/scipr-lab/libiop/tree/master/libiop/bcs:该目录下将BCS transformation作为独立组件。
- https://github.com/scipr-lab/libiop/tree/master/libiop/snark:该目录下,包含,将BCS transformation用于以上IOP协议,所获得的zkSNARKs。具体有:
| language | indexer time | prover time | argument size | verifier time | |
|---|---|---|---|---|---|
| Ligero-SNARK | R1CS | N/A | Oκ(N logN) | Oκ(N0.5) | Oκ(N) |
| Aurora-SNARK | R1CS | N/A | Oκ(N logN) | Oκ(log2 N) | Oκ(N) |
| Fractal-SNARK | R1CS | Oκ(N logN) | Oκ(N logN) | Oκ(log2 N) | Oκ(log2 N) |
其中:
- κ 用于表示上表中的asymptotics还依赖于该安全参数。
make_zk:设置该标签,表示该BCS transformation应保留zero knowledge属性;而不设置,则表示该所转换的IOP是非zero knowledge的,因此也无需保留zero knowledge属性。
5. libiop库安装及测试
编译运行前,需安装:
- 依赖项Installation instructions
测试文件见:
- https://github.com/scipr-lab/libiop/blob/master/libiop/tests
若运行所有测试用例,则运行:
make check
若仅运行Aurora协议所有测试用例,则运行:
$ ./test_aurora_snark$ ./test_aurora_protocol
6. libiop库性能分析
- https://github.com/scipr-lab/libiop/tree/master/libiop/profiling:该目录下包含生成,具有timing和argument size信息的,协议执行traces。
- 这些traces均对应为单线程环境
如,可基于181 bit prime field(其中RS-extra-dimensions=3),采用如下命令,为Ligero、Aurora和Fractal创建traces:
$ ./instrument_aurora_snark --make_zk 1 --is_multiplicative 1 --field_size=181 --optimize_localization=1$ ./instrument_fractal_snark --make_zk 1 --is_multiplicative 1 --field_size=181 --optimize_localization=1$ ./instrument_ligero_snark --make_zk 1 --is_multiplicative 1 --field_size=181 --RS_extra_dimensions=3
根据以上命令所生成的traces,绘制了如下性能分析图表:
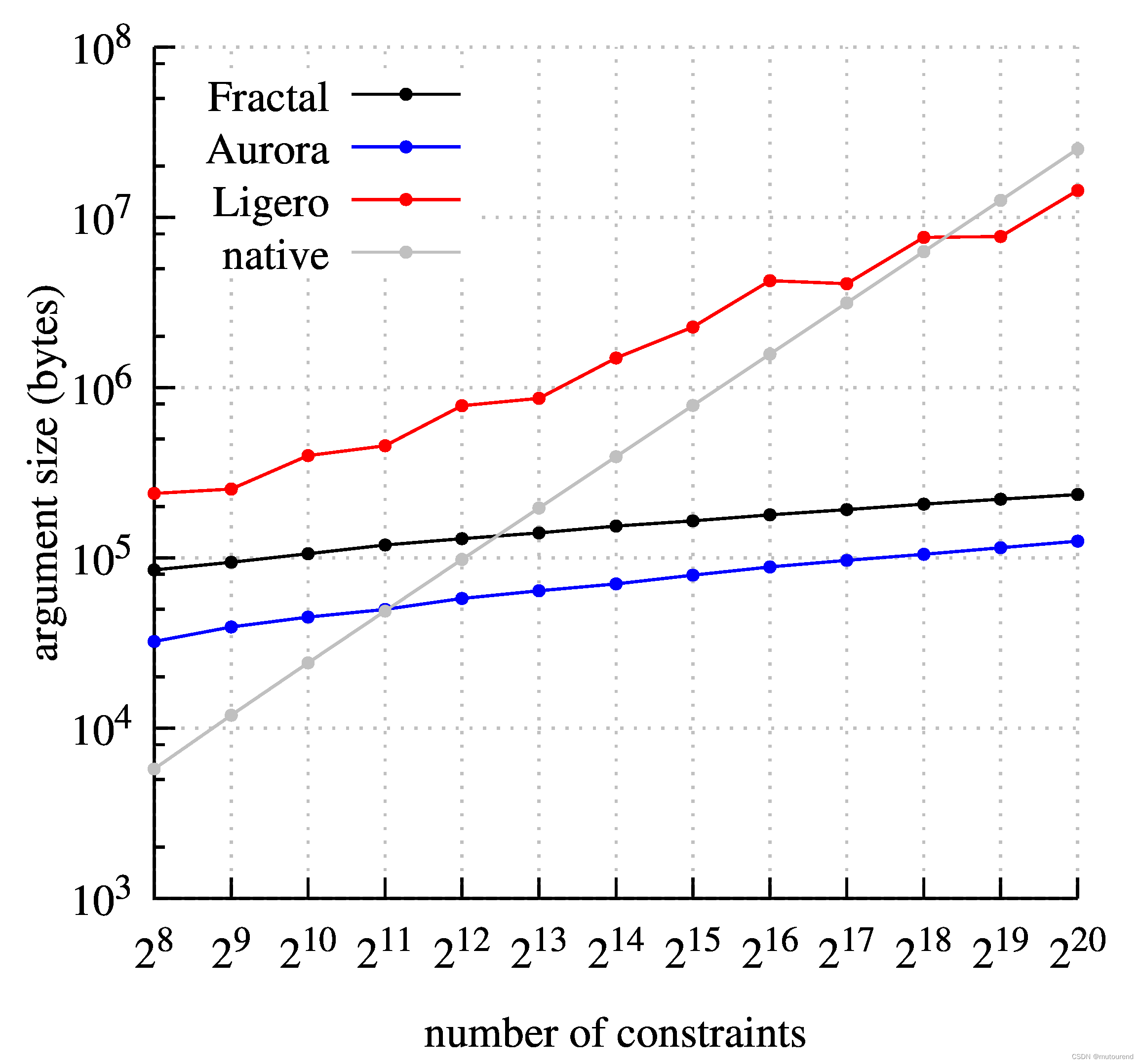
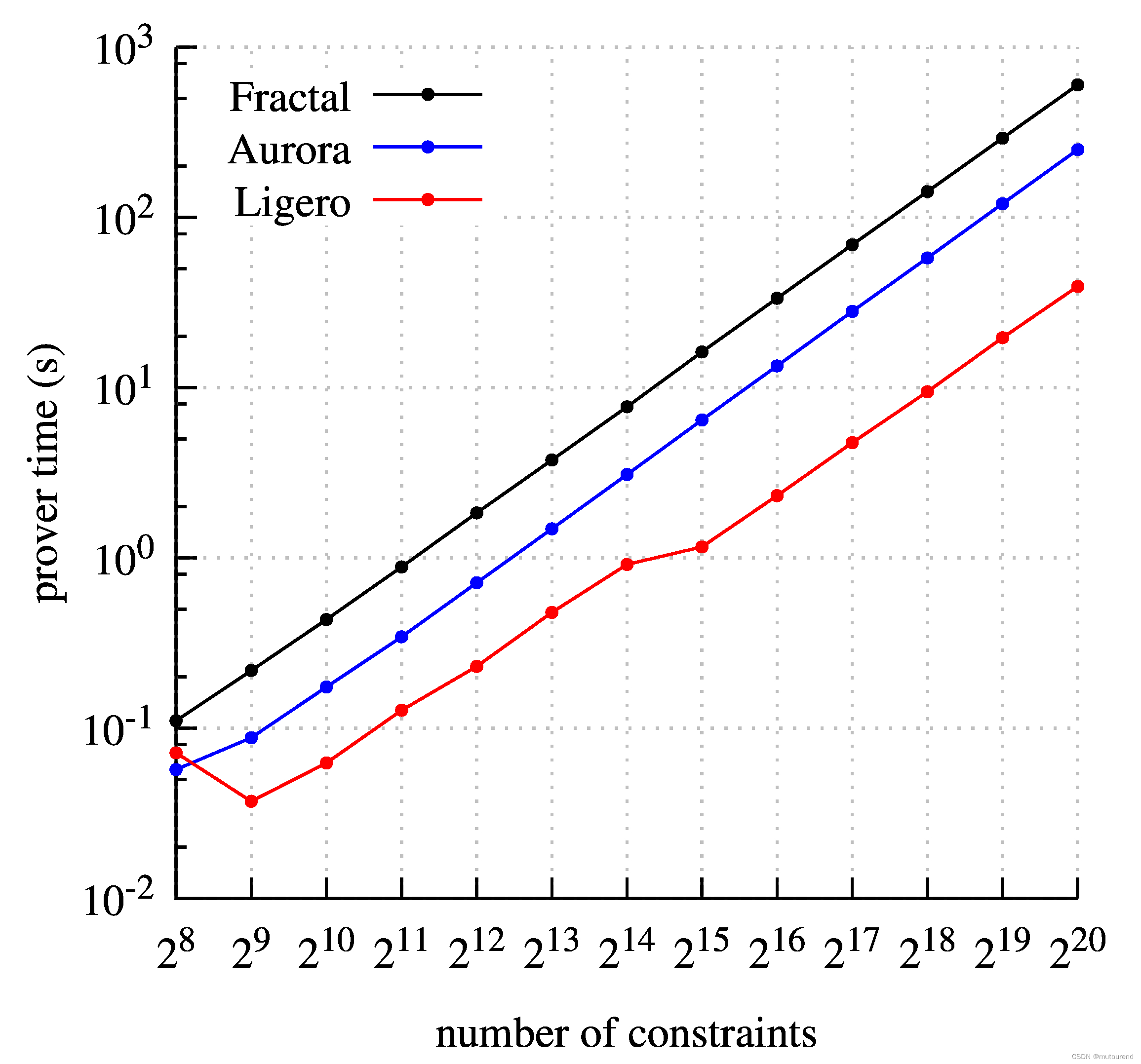
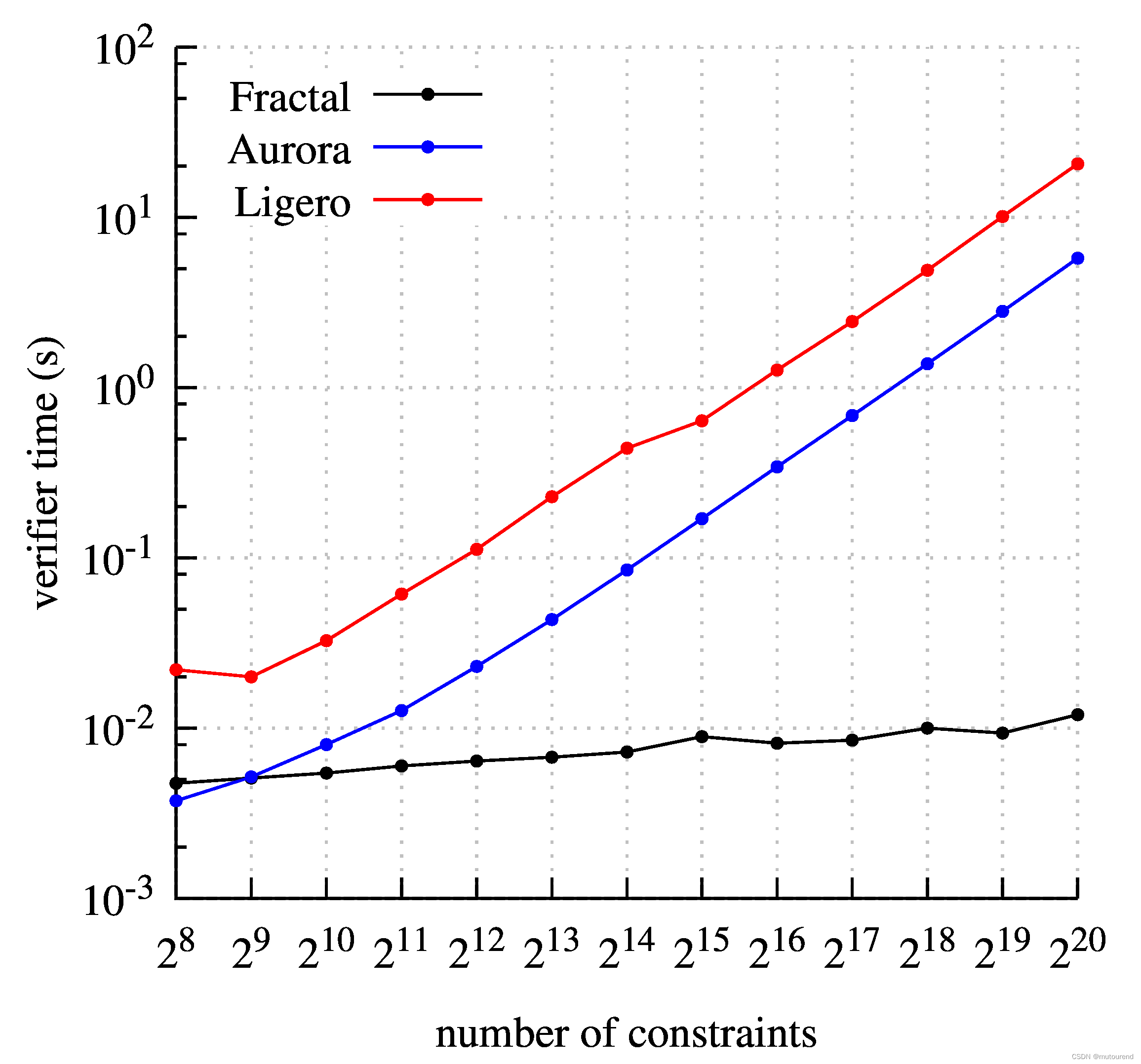
参考资料
[1] libiop: a C++ library for IOP-based zkSNARKs


)

和侦听器(watch))







)


)
:无侵入式的宽松模式泳道)


