高斯消元

用来求解方程组
$$\begin{gathered} a_{11}{x}_1+a_{12}{x}_2+\cdots +a_{1n}{x}_n=b_1 \\ {a}_{21}{x}_1+a_{22}{x}_2+\cdots +a_{2n}{x}_n=b_2 \\ \dots \\ {a}_{n1}{x}_1+a_{n2}{x}_2+\cdots +a_{nn}{x}_n=b_n \end{gathered}$$
输入是$n\times (n-1)$的矩阵。

对方程组进行以下三种初等行列变换后，方程的解不变：

1. 把某一行乘以一个非零的数
2. 交换某2行
3. 把某行的若干倍加到另一行上去

因此，对任意一个方程组，可以把它变成倒三角形式：
$$\begin{gathered} a_{11}{x}_1+a_{12}{x}_2+\cdots +a_{1n}{x}_n=b_1 \\ {a}_{22}{x}_2+\cdots +a_{2n}{x}_n=b_2 \\ \dots \\ {a}_{(n-1)(n-1)}{x}_{n-1}+a_{(n-1)n}{x}_n=b_{n-1} \\ {a}_{nn}{x}_n=b_n \end{gathered}$$
有三种情况：

1. 完美阶梯型——唯一解
2. 0 = 非零 ———无解
3. 0 = 0 ——无穷多组解

高斯消元步骤：

枚举每一列c：

1. 找到绝对值最大的一行
2. 将该行换到最上面
3. 将该行第一个数变成1
4. 将下面所有行的第c列消成0

题目

详见：https://www.acwing.com/problem/content/description/885/

输入一个包含 n 个方程 n 个未知数的线性方程组。

方程组中的系数为实数。

求解这个方程组。

下图为一个包含 m 个方程 n 个未知数的线性方程组示例：

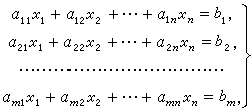
输入格式

第一行包含整数 n。

接下来 n 行，每行包含 n+1 个实数，表示一个方程的 n 个系数以及等号右侧的常数。

输出格式

如果给定线性方程组存在唯一解，则输出共 n 行，其中第 i 行输出第 i 个未知数的解，结果保留两位小数。

注意：本题有 SPJ，当输出结果为 0.00 时，输出 -0.00 也会判对。在数学中，一般没有正零或负零的概念，所以严格来说应当输出 0.00，但是考虑到本题作为一道模板题，考察点并不在于此，在此处卡住大多同学的代码没有太大意义，故增加 SPJ，对输出 -0.00 的代码也予以判对。

如果给定线性方程组存在无数解，则输出 Infinite group solutions。

如果给定线性方程组无解，则输出 No solution。

数据范围

1≤n≤100,
所有输入系数以及常数均保留两位小数，绝对值均不超过 100。

输入样例：

3
1.00 2.00 -1.00 -6.00
2.00 1.00 -3.00 -9.00
-1.00 -1.00 2.00 7.00

输出样例：

1.00
-2.00
3.00

代码

#include<iostream>
#include<algorithm>
#include<cmath>using namespace std;const int N = 110;
const double eps = 1e-6;int n;
double a[N][N];int gauss() {int c, r;for(c = 0, r = 0; c < n; c ++ ) {// 找到绝对值最大的一行 t int t = r;for(int i = r; i < n; i ++ ) {if(fabs(a[i][c]) > fabs(a[t][c])) {t = i;}}if(fabs(a[t][c]) < eps) continue; //如果第t行为0，结束//将该行换到最上面 for(int i = c; i <= n; i ++ ) swap(a[t][i], a[r][i]);  //将该行的第c位设为1（前面都为0） for(int i = n; i >= c; i --) a[r][i] /= a[r][c];//将下面所有行的第c列消成0//也就是从r + 1行开始，对于第i行，第i行第c个位置a[i][c]如果不为0的话，就要消成0// a[i][c]消成0 ： a[i][c] -= a[r][c] * a[i][c]     a[r][c]为1// 那么其他所有列： a[i][j] -= a[r][j] * a[i][c]for(int i = r + 1; i < n; i ++ ) {if(fabs(a[i][c]) > eps) {for(int j = n; j >= c; j -- ) {a[i][j] -= a[r][j] * a[i][c];}}}r ++; }if(r < n) {for(int i = r; i < n; i ++ ) {if(fabs(a[i][n]) > eps)return 2; //无解 }return 1; //有无穷多组解 }//求解唯一解 //从第n - 1 行开始往上，遍历每一行//对于第i行，它的解是a[i][n]的值 for(int i = n - 1; i >= 0; i -- ) {for(int j = i + 1; j < n; j ++ ) {a[i][n] -= a[i][j] * a[j][n];}}return 0; //有唯一解 } int main() {cin >> n;for(int i = 0; i < n; i ++ ) {for(int j = 0; j <= n; j ++ ) {cin >> a[i][j];}}int t = gauss();if(t == 0) {for(int i = 0; i < n; i ++ ) printf("%.2lf\n", a[i][n]);}else if (t == 1) puts("Infinite group solutions");else puts("No solution");return 0;
}