一、 实验目的
(1)掌握图的邻接矩阵和邻接表存储结构。
(2)熟练图的邻接表的基本运算。
(3)加深图的深度优先遍历算法和广度优先遍历算法的理解
二、 实验要求
有下图所示的带权有向图及其对应的邻接矩阵,编写一个程序exp7-1.c,实现图的各种基本运算和下面main函数中的每一步功能。


(1)依据所给的邻接矩阵,创建图的邻接表存储,并输出邻接表结构;
(2)输出从顶点0出发的一个深度优先遍历序列
(3)输出从顶点0出发的一个广度优先遍历序列
(4)销毁图的邻接表。
三、实验环境
Windows+DEV C++( 或者其他编译工具)
四、实验步骤及结果
1.类型声明
typedef char VertexType[10];//顶点的数据类型//声明邻接矩阵类型typedef struct vertex//顶点{int adjvex;//邻接点域VertexType data;//顶点信息} VType;//顶点的类型typedef struct graph//图表{int n,e;//顶点数、边数VType vexs[MAXV];//存放顶点信息int edges[MAXV][MAXV];//邻接矩阵数组} MatGraph;//完整的图邻接矩阵类型//声明邻接表类型typedef struct ANode//边结点{int adjvex;//该边的邻接点编号int weight;//该边的相关信息,例如权值(这里用整型表示)struct ANode *nextarc;//指向下一条边的指针} ArcNode;//边结点的类型typedef struct Vnode //头结点{VertexType data;//顶点信息ArcNode *firstarc;//指向第一个边结点} VNode;// 邻接表的头结点类型typedef struct{int n,e;//图中顶点数n和边数eVNode adjlist[MAXV];//邻接表的头结点数组} AdjGraph;2.图的基本运算在链式存储结构上的实现
void DestroyGraph(AdjGraph *&g)//销毁邻接表运算算法 {int i;ArcNode *pre,*p;for (i=0;i<g->n;i++)// 遍历所有的单链表{pre=g->adjlist[i].firstarc;//p指向第i个单链表的头结点if (pre!=NULL){p=pre->nextarc;while (p!=NULL) //释放第i个单链表的所有边结点{free(pre);pre=p;p=p->nextarc;}free(pre);//释放头结点数组}}free(g);}void DispGraph(AdjGraph *g)//输出邻接表g运算算法{ArcNode *p;int i;for (i=0;i<g->n;i++){printf(" [%2d]",i);p=g->adjlist[i].firstarc;if (p!=NULL)printf("→");while (p!=NULL){printf("%d(%d)",p->adjvex,p->weight);p=p->nextarc;}printf("\n");}}3. 程序exp7-1.c的设计,及完成实验要求中的功能
#include <iostream>using namespace std;#include <stdlib.h>#define MAXV 100//定义最大顶点数#define INF 32767//定义无穷大#include <stdio.h>int visited[MAXV];//访问标志数组int visited1[MAXV];typedef char VertexType[10];//顶点的数据类型//声明邻接矩阵类型typedef struct vertex//顶点{int adjvex;//邻接点域VertexType data;//顶点信息} VType;//顶点的类型typedef struct graph//图表{int n,e;//顶点数、边数VType vexs[MAXV];//存放顶点信息int edges[MAXV][MAXV];//邻接矩阵数组} MatGraph;//完整的图邻接矩阵类型//声明邻接表类型typedef struct ANode//边结点{int adjvex;//该边的邻接点编号int weight;//该边的相关信息,例如权值(这里用整型表示)struct ANode *nextarc;//指向下一条边的指针} ArcNode;//边结点的类型typedef struct Vnode //头结点{VertexType data;//顶点信息ArcNode *firstarc;//指向第一个边结点} VNode;// 邻接表的头结点类型typedef struct{int n,e;//图中顶点数n和边数eVNode adjlist[MAXV];//邻接表的头结点数组} AdjGraph;void CreateGraph1(MatGraph &G,int A[][MAXV],int n,int e) //建立邻接矩阵运算算法{int i,j;G.n=n;G.e=e;for (i=0;i<n;i++)for (j=0;j<n;j++)G.edges[i][j]=A[i][j];}void DestroyGraph1(MatGraph G)//销毁邻接矩阵运算算法{}void DispGraph1(MatGraph G)//输出邻接矩阵运算算法{int i,j;for (i=0;i<G.n;i++){for (j=0;j<G.n;j++)if (G.edges[i][j]<INF)printf("%4d",G.edges[i][j]);elseprintf("%4s","∞");printf("\n");}}int Degree1(MatGraph G,int v)//有向图邻接矩阵顶点度运算算法{int i,d1=0,d2=0,d;if (v<0 || v>=G.n)return -1;for (i=0;i<G.n;i++)if (G.edges[v][i]>0&&G.edges[v][i]<INF)d1++;for (i=0;i<G.n;i++)if (G.edges[i][v]>0&&G.edges[i][v]<INF)d2++;d=d1+d2;return d;}void DFS1(MatGraph G,int v)//邻接矩阵存储的深度优先遍历序列{int w;printf("%d",v);visited1[v]=1;for (w=0;w<G.n;w++)if (G.edges[v][w]!=0&&G.edges[v][w]!=INF&&visited1[w]==0)DFS1(G,w);}void BFS1(MatGraph G,int v)//邻接矩阵存储的广度优先遍历序列{int i,w;int Qu[MAXV],front=0,rear=0;for (i=0;i<G.n;i++) visited1[i]=0;printf("%d",v);visited1[v]=1;rear=(rear+1)%MAXV;Qu[rear]=v;while (front!=rear){front=(front+1)%MAXV;w=Qu[front];for (i=0;i<G.n;i++)if (G.edges[w][i]!=0&&G.edges[w][i]!=INF&&visited1[i]==0){printf("%d",i);visited1[i]=1;rear=(rear+1)%MAXV;Qu[rear]=i;}}}void CreateGraph(AdjGraph *&g,int A[][MAXV],int n,int e)//建立邻接表运算算法{int i,j;ArcNode *p;g=(AdjGraph *)malloc(sizeof(AdjGraph));//动态分配空间g->n=n;g->e=e;for (i=0;i<g->n;i++)//所有的头结点指针置为空g->adjlist[i].firstarc=NULL;for (i=0;i<g->n;i++)//遍历邻接矩阵for (j=g->n-1;j>=0;j--)if (A[i][j]>0&&A[i][j]<INF)//存在一条边<i,j>{p=(ArcNode *)malloc(sizeof(ArcNode));//创建一个结点pp->adjvex=j;//存放邻接点p->weight=A[i][j];//存放权p->nextarc=g->adjlist[i].firstarc;//采用头插法插入结点pg->adjlist[i].firstarc=p;}}void DestroyGraph(AdjGraph *&g)//销毁邻接表运算算法 {int i;ArcNode *pre,*p;for (i=0;i<g->n;i++)// 遍历所有的单链表{pre=g->adjlist[i].firstarc;//p指向第i个单链表的头结点if (pre!=NULL){p=pre->nextarc;while (p!=NULL) //释放第i个单链表的所有边结点{free(pre);pre=p;p=p->nextarc;}free(pre);//释放头结点数组}}4.实验结果截图
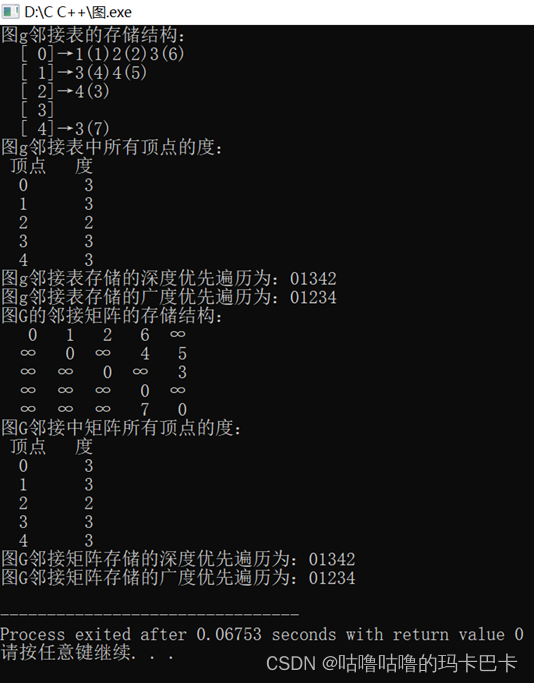
如需源文件,请私信作者,无偿
)



)





)



 讲述循环概念演示while语句)

)


