我们都知道，矩阵的乘法可以表示旋转。那么，这一理论的数学机理是什么呢？以及，这个旋转角度该怎么用矩阵表示呢？

本文用二维向量旋转来推导旋转矩阵的公式。假设，我们有一个向量P(x, y)，准备通过一个旋转矩阵将其旋转到Q(x’, y’)，假设旋转角度为$\alpha$。

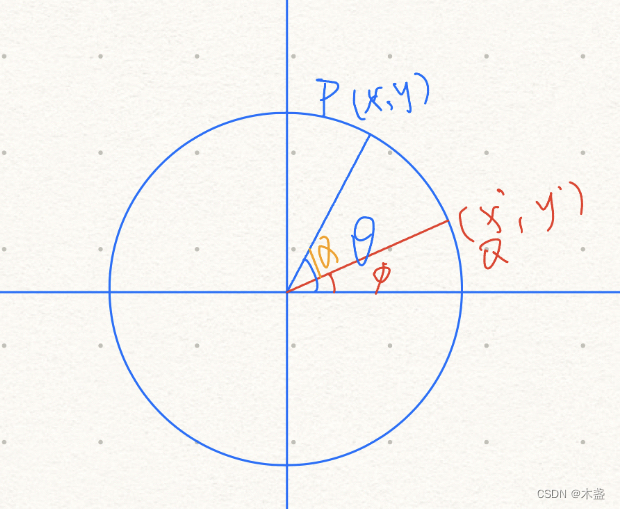
我们用极坐标表示向量P和向量Q，默认原点是向量的起点，$\theta$和$\varphi$分别表示P和Q与x轴正向的夹角。那么有，
$$x=r\ast cos(\theta )\text{\hspace{1em}(1)}$$
$$y=r\ast sin(\theta )\text{\hspace{1em}(2)}$$
$$x^{\prime }=r\ast cos(\varphi )=r\ast cos(\alpha -\theta )\text{\hspace{1em}(3)}$$
$$y^{\prime }=r\ast sin(\varphi )=r\ast sin(\alpha -\theta )\text{\hspace{1em}(4)}$$
咱们用一张图可以清晰解释上面5个公式：

我们直接把式(3)、(4)展开：
$$x^{\prime }=r\ast cos(\theta )cos(\alpha )+r\ast sin(\theta )sin(\alpha )=xcos(\alpha )+ysin(\alpha )\text{\hspace{1em}(5)}$$
$$y^{\prime }=r\ast sin(\alpha )cos(\theta )-r\ast sin(\theta )cos(\alpha )=xsin(\alpha )-ycos(\alpha )\text{\hspace{1em}(6)}$$
整理(5)(6)得知：
$$R=\left[\begin{array}{cc}cos(\alpha )& -sin(\alpha )\\ sin(\alpha )& cos(\alpha )\end{array}\right]$$