目录
一、题目描述
二、初次解答
三、官方解法
四、总结
一、题目描述
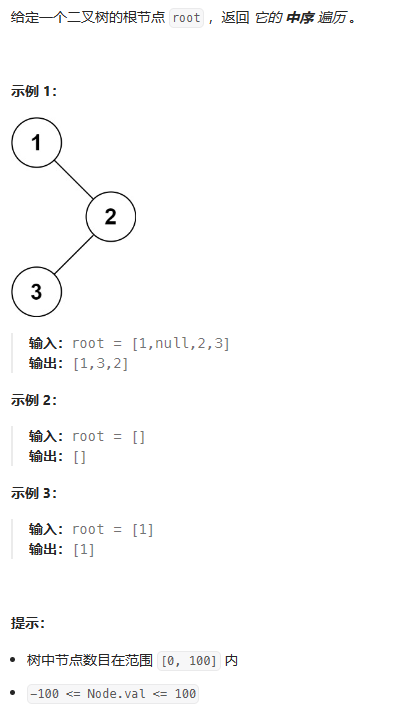
二、初次解答
1. 思路:二叉树的中序遍历。访问二叉树的左子树,再访问二叉树的根节点,最后访问二叉树的右叉树。
2. 代码:
void order(struct TreeNode* root, int* ret, int* p){if(!root)return;order(root->left, ret, p);ret[(*p)++]=root->val;order(root->right, ret, p); }int* inorderTraversal(struct TreeNode* root, int* returnSize) {int* ret=(int*)malloc(sizeof(int)*100);*returnSize=0;order(root, ret, returnSize);return ret; }3. 优点:实现简单,容易想到,且仅需遍历一遍,时间复杂度为O(n)。
4. 缺点:需要递归栈空间,空间复杂度为O(n)。
三、官方解法
1. 思路:迭代遍历二叉树,手动维护栈。每次迭代访问子节点前,将当前节点地址保存到栈内,访问完左节点后,再访问当前节点,最后访问右节点。
2. 代码:
int* inorderTraversal(struct TreeNode* root, int* returnSize) {int* ret=malloc(sizeof(int)*100);struct TreeNode** tmp=malloc(sizeof(struct TreeNode*)*100);int top=0;*returnSize=0;while(root || top>0){while(root){tmp[top++]=root;root=root->left;}root=tmp[--top];ret[(*returnSize)++]=root->val;root=root->right;}return ret; }3. 优点:符合不采用迭代的要求,且时间复杂度为O(n)。
4. 缺点:手动维护栈,空间复杂度依旧为O(n)。
四、总结
当遇到二叉树中序遍历时,使用递归实现最简单,也可以采用迭代手动维护栈空间来实现。







)






 使用教程)




