前言

本人是算法小白，甚至也没有做过Leetcode。所以，我相信【同为菜鸡的我更能理解作为菜鸡的你们的痛点】。

题干

1. 题目描述

张兵和王武是五子棋迷，工作之余经常切磋棋艺。走了一会儿，轮到张兵了，他对着一条线思考起来了，这条线上的棋子分布如下：
用数组表示：-1 0 1 1 1 0 1 0 1 -1
棋子分布说明：

1. -1代表白子，0代表空位，1代表黑子：
2. 数组长度L,满足1<L<40，且L为奇数：

请帮他写一个程序，算出最有利的出子位置。最有利定义如下：

1. 找到一个空位(0)，用棋子(1/-1)填充该位置，可以使得当前子的最大连续长度变大；
2. 如果存在多个位置，返回最靠近中间的较小的那个坐标；
3. 如果不存在可行位置，直接返回-1；
4. 连续长度不能超过5个（五子棋约束）；

2. 输入描述

第一行：当前出子颜色
第二行：当前的棋局状态

3. 输出描述

一个整数，表示出子位置的数组下标

4. 示例

示例1：
输入：

1
-1 0 1 1 1 0 1 0 1 -1 1

输出：

5

说明：当前为黑子（1），放置在下表为5的位置，黑子的最大连续长度由3->5，所以满足条件

示例2：
输入：

-1
-1 0 1 1 1 0 1 0 1 -1 1

输出：

1

说明：当前为白子（-1），唯一可以防止的位置下标为1，婊子的最大长度由1->2，所以满足条件

示例3：
输入：

1
0 0 0 0 1 0 0 0 0 1 0

输出：

5

说明：可行的位置很多，但是5是最接近中间的位置坐标

解答

遇到的问题

这题整体难度不是很大，但还是遇到了一些问题。

1. 如何从控制台输入一个变长的数组（哈，确实不会，没怎么用过Scanner）
2. 在实现过程中，其实我还发现，输入多行、不定长数据还存在无法停止输入的情况（只能通过输入非目标类型数据来停止，但我希望的是【回车】停止）
3. 虽然脑海里大概能想到一个暴力解题办法，但总觉得怪怪的，应该有更好的方式才对

解题思路

暴力解题版：
根据题目给出的条件，做出如下思考：

1. 新增一个方法，用来寻找【下一个空位】。由于是下一个，所以需要一个检索的起始点
2. 新增一个方法，用来获取当前数组的中间位置的下标（其实可以不新增一个方法，单纯是个人喜欢这么做，我比较追求语义清晰跟代码可读性）
3. 新增一个方法，用来计算给定线上棋子的【最大连续长度】
4. 整体思路是，循环遍历每一个可以下子的点，然后记录当前点的位置，以及最大连续长度，还有距离中心点的位置。接着判断最大连续长度是否变大且<5。最后比较距离中心点的位置是否更近了

但我看了网上答案之后，发现了更好的解题方法。那就是【滑动窗口法】，这应该才是本题真正的考点。
滑动窗口法：

1. 滑动窗口在这里的应用，主要是在求落子后新的最大连续长度。而且这个滑动起点跟终点，不是从0开始，而是从引起变化的【落子节点】开始。怎么理解？这个我们在代码解读中给大家解释一下。

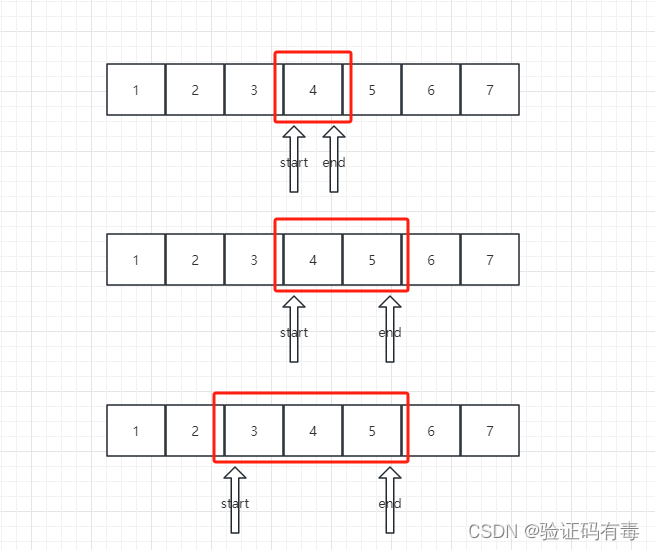
滑动窗口示意图：（随着起点、终点变化，[start, end]区间也在变化）

代码示例

暴力破解版：（但其实我不推荐，我贴出来完全是因为这是我个人自己的努力，所以为了鼓励自己就贴上，哈哈）

public class FiveInRow {/*** 白棋*/static final int WHITE_CHESS = -1;/*** 黑棋*/static final int BLACK_CHESS = 1;/*** 空白*/static final int BLANK_SPACE = 0;/*** 最大连续长度*/static final int MAX_CONTINOUS_LENGTH = 5;/*** 数组长度*/static final int MAX_LENGTH = 40;public static void main(String[] args) {// 数据准备Scanner scanner = new Scanner(System.in);int currChess = scanner.nextInt();scanner.nextLine();String nextLine = scanner.nextLine();String[] nums = nextLine.split(" ");List<Integer> chesses = new ArrayList<>();for (String num : nums) {chesses.add(Integer.parseInt(num));}// 获取最有利下子位置int bestPlace = getBestPlace(currChess, chesses);System.out.println("最佳下子位置：" + bestPlace);}/*** 找到最有利的位置** @param currChess 当前棋子颜色* @param chesses   一条线上棋子分布* @return -1-找不到可以下的位置；其他-最有利下棋处*/private static int getBestPlace(int currChess, List<Integer> chesses) {int bestPlace = -1;// 获取当前连续长度int currContinuous = getCurrContinuous(currChess, chesses);int midLocation = getMidLocation(chesses.size());// 获取下一个空白点int nearest = 99;int start = 0;int nextBlankPlace = getNextBlankPlace(start, chesses);while (nextBlankPlace != -1) {List<Integer> newChesses = new ArrayList<>(chesses);newChesses.set(nextBlankPlace, currChess);int newCurrContinuous = getCurrContinuous(currChess, newChesses);if (newCurrContinuous > currContinuous && newCurrContinuous <= MAX_CONTINOUS_LENGTH) {int interval = Math.abs(nextBlankPlace - midLocation);if (interval < nearest) {bestPlace = nextBlankPlace;nearest = interval;}}start = nextBlankPlace + 1;nextBlankPlace = getNextBlankPlace(start, chesses);}return bestPlace;}/*** 获取当前连续度** @param currChess 当前棋子颜色* @param chesses   一条线上棋子分布* @return 当前连续长度*/private static int getCurrContinuous(int currChess, List<Integer> chesses) {// 当前棋局下最大连续长度int curMaxConsecutive = 0;// 当前连续长度int consecutiveCount = 0;for (int i = 0; i < chesses.size(); ++i) {if (chesses.get(i) == currChess) {consecutiveCount += 1;} else {consecutiveCount = 0;}curMaxConsecutive = Math.max(curMaxConsecutive, consecutiveCount);}return curMaxConsecutive;}/*** 从指定位置开始检索空白位置** @param start   开始检索位置* @param chesses 一条线上棋子分布* @return 下一个空白位置*/private static int getNextBlankPlace(int start, List<Integer> chesses) {if (start >= chesses.size()) {return -1;}for (int i = start; i < chesses.size(); i++) {Integer chess = chesses.get(i);if (chess == BLANK_SPACE) {return i;}}return -1;}/*** 获取中间位置的索引** @param length 数组长度* @return 中间位置的索引*/private static int getMidLocation(int length) {return length / 2;}
}

代码解读：整体来说我还是按照了解题思路完成的

1. 多行不定长输入，我是这么完成的。我也不是很理解为什么，没有细看API源码

        // 数据准备Scanner scanner = new Scanner(System.in);int currChess = scanner.nextInt();scanner.nextLine();String nextLine = scanner.nextLine();String[] nums = nextLine.split(" ");List<Integer> chesses = new ArrayList<>();for (String num : nums) {chesses.add(Integer.parseInt(num));}

滑动窗口法：（推荐版本）
新增一个方法getBestPlaceUsingSlidingWindows替换旧方法getBestPlace

 /*** 找到最有利的位置，滑动窗口法** @param currChess 当前棋子颜色* @param chesses   一条线上棋子分布* @return -1-找不到可以下的位置；其他-最有利下棋处*/private static int getBestPlaceUsingSlidingWindows(int currChess, List<Integer> chesses) {int bestPlace = -1;// 获取当前连续长度int currContinuous = getCurrContinuous(currChess, chesses);int midLocation = getMidLocation(chesses.size());// 获取下一个空白点int nearest = 99;for (int i = 0; i < chesses.size(); i++) {Integer chess = chesses.get(i);if (chess != BLANK_SPACE) {continue;}int start = i - 1, end = i + 1;// 确定窗口真实起点while (start >= 0 && chesses.get(start).equals(currChess)) {start--;}// 确定窗口真实终点while (end < chesses.size() && chesses.get(end).equals(currChess)) {end++;}// 由于是开区间（不包含区间范围的两个节点），所以需要-1int newCurrContinuous = end - start - 1;if (newCurrContinuous > currContinuous && newCurrContinuous <= MAX_CONTINOUS_LENGTH) {int interval = Math.abs(i - midLocation);if (interval < nearest) {bestPlace = i;nearest = interval;}}}return bestPlace;}

我们解释回解题思路中，所谓【滑动起点跟终点，不是从0开始，而是从引起变化的【落子节点】开始】这段话，我们结合示例3看看，不知道大家发现没有，假设我们落子在0/1/2下标处，再求它的新的最大连续度的时候，其实真正引起连续度变化的点，就是我们落子开始的点。

例如：示例3，落子在2处
0 0 0 0 1 0 0 0 0 1 0（落子前）
0 0 1 0 1 0 0 0 0 1 0（落子后）
看，如果使用暴力解题的方法，还是会去遍历0/1坐标，有必要吗？其实可以不需要的

代码解读：滑动窗口在这里的应用，重点是里面的两个while循环，这里就不过多解释了