【C++】AVL树红黑树

AVL树

AVL树也是二叉搜索树的一种。因为对于普通的二叉搜索树，当插入的数据在有序或接近有序的情况下，二叉搜索树很可能退化成单支树，导致查找效率低下。而AVL树就很好的解决了这个问题。
首先，AVL树是一棵二叉搜索树。同时对于AVL树中每个节点，它的左右子树高度之差的绝对值不超过1。
对于有n个节点的AVL树，其搜索时间复杂度可以稳定的保持在 $O(log_2 n)$ 。
为了保证向AVL树中插入节点，仍保持其高度的平衡，可以引入平衡因子（平衡因子：右子树的高度 - 左子树的高度）来处理。

// AVL树节点的定义
template<class K, class V>
class AVLTreeNode
{
public:AVLTreeNode(const pair<K, V>& kv): _kv(kv), _left(nullptr), _right(nullptr), _parent(nullptr), _bf(0){}pair<K, V> _kv;AVLTreeNode<K, V>* _left;AVLTreeNode<K, V>* _right;AVLTreeNode<K, V>* _parent;int _bf; //  balance factor
};

因为AVL树仍是二叉搜索树，所以AVL树的插入可以分两步进行：

按照二叉搜索树的方式插入新节点
调整平衡因子

// AVL树的插入
template<class K, class V>
class AVLTree
{
private:typedef AVLTreeNode<K, V> Node;
public:AVLTree(Node* root = nullptr): _root(root){}bool Insert(const pair<K, V>& kv){/* * 1.按照二叉搜索树的方式插入新节点*/if (_root == nullptr){_root = new Node(kv);return true;}Node* parent = nullptr;Node* cur = _root;while (cur){if (kv.first  > cur->_kv.first){parent = cur;cur = cur->_right;}else if (kv.first < cur->_kv.first){parent = cur;cur = cur->_left;}else{return false;}}// 直接插入cur = new Node(kv);if (kv.first > parent->_kv.first){parent->_right = cur;}else{parent->_left = cur;}cur->_parent = parent;/** 2.调整平衡因子*/while (parent){// 新增在右，parent->_bf++; 新增在左，parent->_bf--;if (cur == parent->_right){parent->_bf++;}else{parent->_bf--;}// 调整后，parent->_bf == 0, 说明parent插入前的平衡因子是 1 or -1，说明插入前左右子树一边高一边低，插入后两边一样高，// 即插入填上了矮的一边，插入后parent所在子树高度不变，不需要继续往上调整if (parent->_bf == 0){break;}// 调整后，parent->_bf == 1 or -1, 说明parent插入前的平衡因子是0，插入前左右子树高度相等，插入后有一边变高了，// parent高度变了，需要继续往上更新else if (abs(parent->_bf) == 1){parent = parent->_parent;cur = cur->_parent;}// 调整后，parent->_bf == 2 or -2, 说明parent插入前的平衡因子是 1 or -1，已经是平衡临界值，// 插入后变成了 2 or -2，打破平衡，parent所在子树需要进行 旋转处理else if (abs(parent->_bf) == 2){// 根据节点插入位置的不同，AVL树的旋转分为四种// 新节点插入在较高右子树的右侧（右右）if (parent->_bf == 2 && cur->_bf == 1){// 左单旋RotateL(parent);}// 新节点插入在较高左子树的左侧（左左）else if (parent->_bf == -2 && cur->_bf == -1){// 右单旋RotateR(parent);}// 新节点插入在较高左子树的右侧（左右）else if (parent->_bf == -2 && cur->_bf == 1){// 左右双旋RotateLR(parent);}// 新节点插入在较高右子树的左侧（右左）else if (parent->_bf == 2 && cur->_bf == -1){// 右左双旋RotateRL(parent);}else{assert(false);}break;}// 调整后，不可能出现parent->_bf > 2 or < -2，否则一定出bug了else{assert(abs(parent->_bf) <= 2);}}return true;}
private:// 左单旋void RotateL(Node* parent){Node* subR = parent->_right;Node* subRL = subR->_left;parent->_right = subRL;if (subRL){subRL->_parent = parent;}// 保存parent的双亲节点Node* ppNode = parent->_parent;subR->_left = parent;parent->_parent = subR;// parent作为整棵树的根存在if (_root == parent){_root = subR;subR->_parent = nullptr;}// parent作为子树的根存在else{if (ppNode->_left == parent){ppNode->_left = subR;}else{ppNode->_right = subR;}subR->_parent = ppNode;}subR->_bf = parent->_bf = 0;}// 右单旋void RotateR(Node* parent){Node* subL = parent->_left;Node* subLR = subL->_right;parent->_left = subLR;if (subLR){subLR->_parent = parent;}Node* ppNode = parent->_parent;subL->_right = parent;parent->_parent = subL;if (_root == parent){_root = subL;subL->_parent = nullptr;}else{if (ppNode->_left == parent){ppNode->_left = subL;}else{ppNode->_right = subL;}subL->_parent = ppNode;}subL->_bf = parent->_bf = 0;}// 左右双旋void RotateLR(Node* parent){Node* subL = parent->_left;Node* subLR = subL->_right;// 保存subLR当前的平衡因子int bf = subLR->_bf;// 先左单旋RotateL(parent->_left);// 再右单旋RotateR(parent);// 此处对于平衡因子的调整，建议画图分析subLR->_bf = 0;if (bf == 1){parent->_bf = 0;subL->_bf = -1;}else if (bf == -1){parent->_bf = 1;subL->_bf = 0;}else if(bf == 0){parent->_bf = 0;subL->_bf = 0;}else{assert(false);}}// 右左双旋void RotateRL(Node* parent){Node* subR = parent->_right;Node* subRL = subR->_left;int bf = subRL->_bf;RotateR(parent->_right);RotateL(parent);subRL->_bf = 0;if (bf == 1){parent->_bf = -1;subR->_bf = 0;}else if (bf == -1){parent->_bf = 0;subR->_bf = 1;}else if (bf == 0){parent->_bf = 0;subR->_bf = 0;}else{assert(false);}}
private:Node* _root;
};

RotateL左单旋示意图：
在这里插入图片描述
RotateR右单旋示意图：

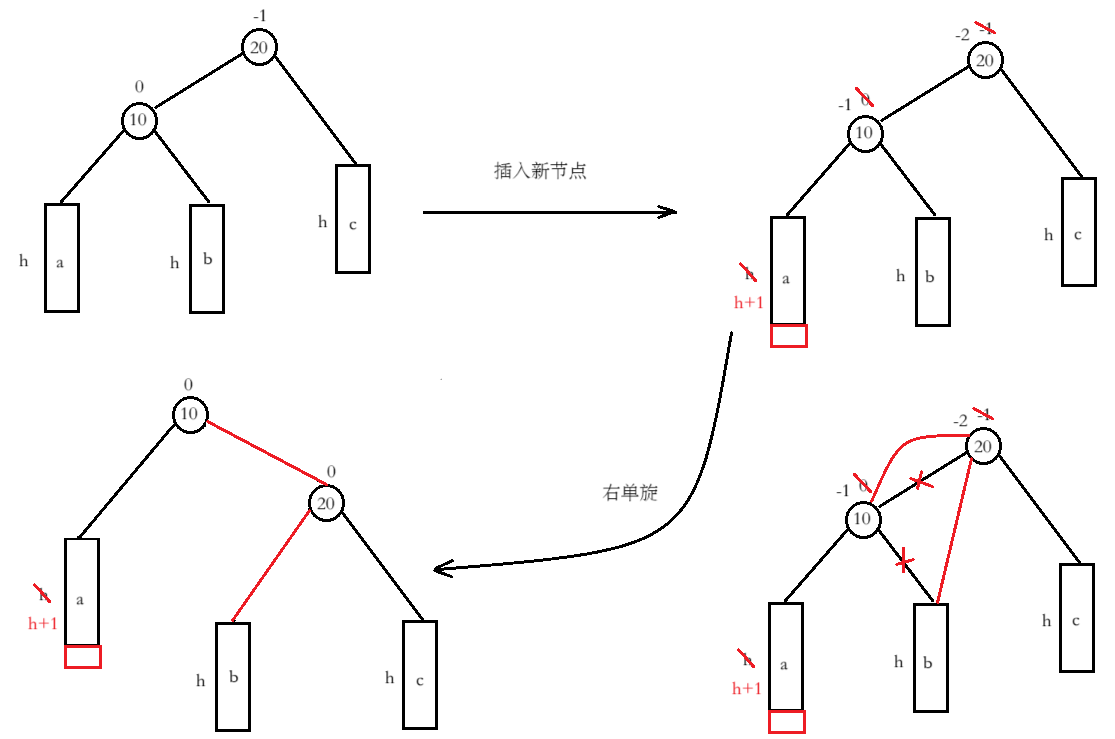
RotateLR左右双旋示意图：

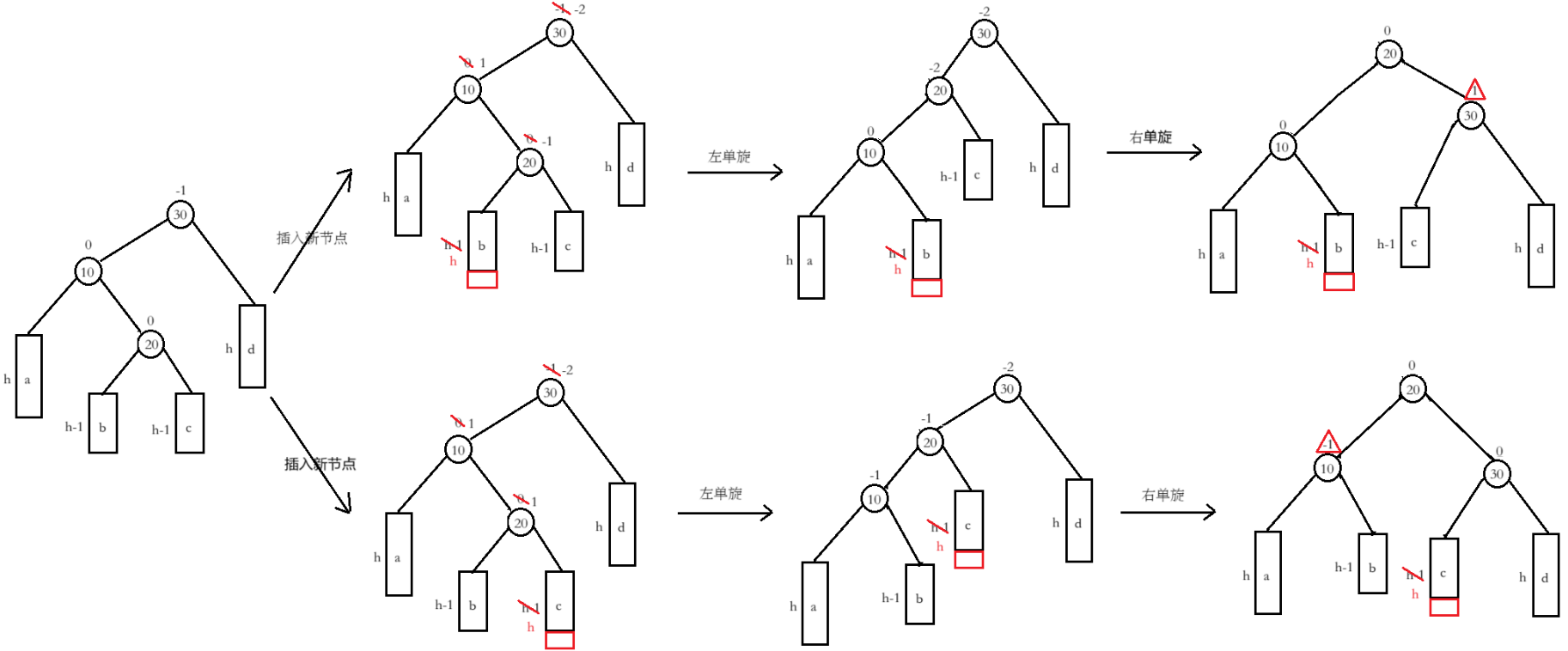
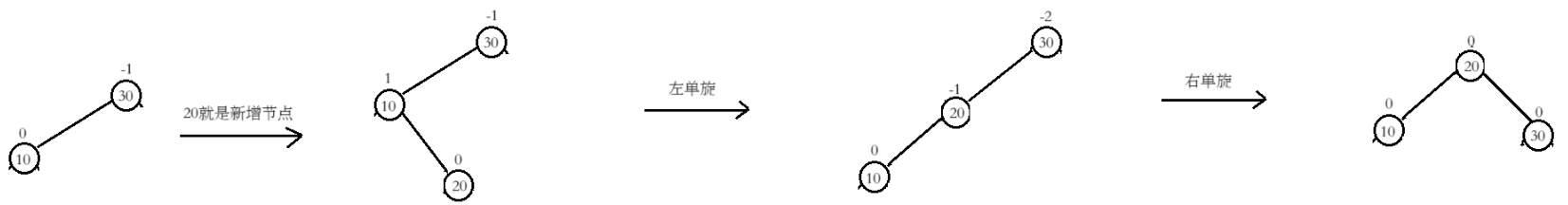
RotateRL右左双旋示意图：

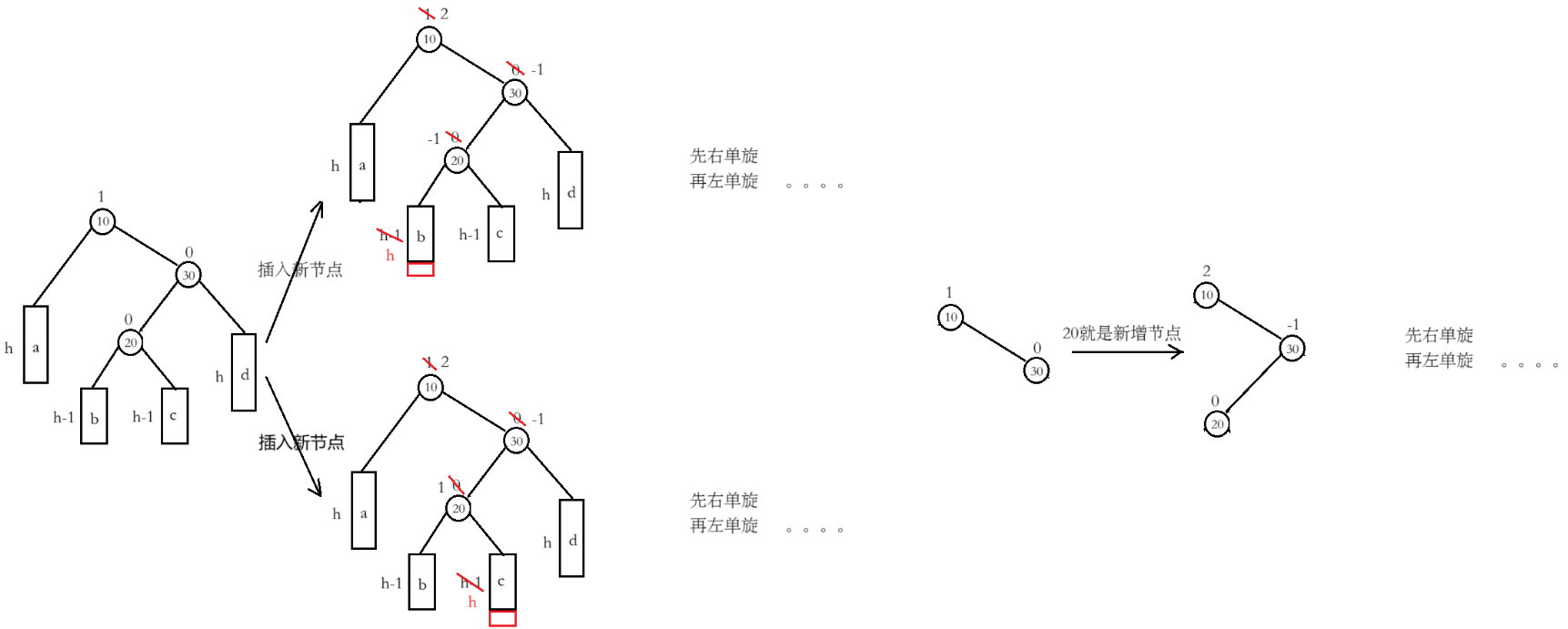
可以通过下面程序对AVL树的正确性做一个验证。

template<class K, class V>
class AVLTree
{
private:typedef AVLTreeNode<K, V> Node;
public:// 1.验证其为二叉搜索树void InOrderTraversal(){_InOrderTraversal(_root);}// 2.验证其为平衡树bool IsBalance(){return _IsBalance(_root);}
private:// 如果中序遍历为有序序列，则说明为二叉搜索树void _InOrderTraversal(Node* root){if (root == nullptr){return;}_InOrderTraversal(root->_left);cout << root->_kv.first << " : " << root->_kv.second << endl;_InOrderTraversal(root->_right);}// 要求每个节点的子树高度差的绝对值不超过1，并且高度差要和平衡因子相等bool _IsBalance(Node* root){// 空树也算AVL树if (root == nullptr){return true;}int leftHt = Height(root->_left);int rightHt = Height(root->_right);int diff = rightHt - leftHt;if (diff != root->_bf){cout << root->_kv.first << "平衡因子异常" << endl;return false;}return abs(diff) < 2&& _IsBalance(root->_left)&& _IsBalance(root->_right);}int Height(Node* root){if (root == nullptr)return 0;int leftHt = Height(root->_left);int rightHt = Height(root->_right);return max(leftHt, rightHt) + 1;}
private:Node* _root;
};

红黑树

红黑树也是二叉搜索树的一种。
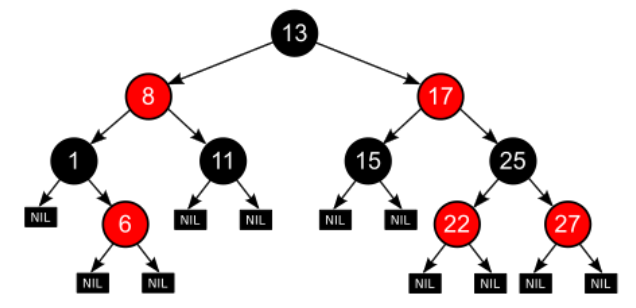
在这里插入图片描述
红黑树的性质要求如下：

每个节点不是红色就是黑色
根节点是黑色的
如果一个节点是红色的，则它的两个孩子节点必须是黑色的（或者说不允许存在父子节点同时为红色）
对于每个节点，从该节点到其后代叶节点的所有简单路径上，都只包含相同数量的黑色节点
所有的叶节点都是黑色的（叶节点指的是NIL节点）

通过以上5条性质的约束，就可以确保红黑树中 $\leq 2 \times 最短路径$ ，从而来保证了红黑树的平衡性能。
虽然红黑树的平衡性能比AVL的略差些，但插入同样的数据，红黑树旋转更少。

// 红黑树节点的定义
enum Colour
{RED,BLACK
};template<class K, class V>
class RBTreeNode
{
public:RBTreeNode(const pair<K, V>& kv): _kv(kv), _left(nullptr), _right(nullptr), _parent(nullptr){}pair<K, V> _kv;RBTreeNode<K, V>* _left;RBTreeNode<K, V>* _right;RBTreeNode<K, V>* _parent;Colour _col;
};

因为红黑树仍是二叉搜索树，所以红黑树的插入可以分两步进行：

按照二叉搜索树的方式插入新节点
检测插入新节点后，红黑树的性质是否受到破坏，并做变色或旋转调整

template<class K, class V>
class RBTree
{
private:typedef RBTreeNode<K, V> Node;
public:RBTree(Node* root = nullptr): _root(root){}bool Insert(const pair<K, V>& kv){if (_root == nullptr){_root = new Node(kv);// 根节点是黑色的_root->_col = BLACK;return true;}Node* parent = nullptr;Node* cur = _root;while (cur){if (kv.first > cur->_kv.first){parent = cur;cur = cur->_right;}else if (kv.first < cur->_kv.first){parent = cur;cur = cur->_left;}else{return false;}}// 直接插入新节点cur = new Node(kv);// 对于插入的新节点赋为红色cur->_col = RED;if (kv.first > parent->_kv.first){parent->_right = cur;}else{parent->_left = cur;}cur->_parent = parent;// 如果父节点存在且为黑，没有破坏红黑树的规则，不需要调整// 如果父节点存在且为红，破坏了性质3while (parent && parent->_col == RED){// 此时祖父节点一定存在且为黑Node* grandparent = parent->_parent;assert(grandparent);assert(grandparent->_col == BLACK);// 关键看叔叔(节点)// parent 为 grandparent 的左孩子if (parent == grandparent->_left){Node* uncle = grandparent->_right;// 情况一 uncle存在且为红if (uncle && uncle->_col == RED){// 将 parent 和 uncle 改为黑，grandparent 改为红，parent->_col = uncle->_col = BLACK;grandparent->_col = RED;// 然后把 grandparent 当成 cur，继续向上调整cur = grandparent;parent = cur->_parent;}// 情况二三 uncle不存在，或uncle存在且为黑else{// parent 为 grandparent 的左孩子，且 cur 为 parent 的左孩子if (cur == parent->_left){// 右单旋RotateR(grandparent);// 变色: parent 变黑，grandparent 变红parent->_col = BLACK;grandparent->_col = RED;}// parent 为 grandparent 的左孩子，且 cur 为 parent 的右孩子else{// 左右双旋RotateL(parent);RotateR(grandparent);// 变色: parent 变黑，grandparent 变红cur->_col = BLACK;grandparent->_col = RED;}break;}}// parent 为 grandparent 的右孩子else{Node* uncle = grandparent->_left;// 情况一 uncle存在且为红if (uncle && uncle->_col == RED){parent->_col = uncle->_col = BLACK;grandparent->_col = RED;cur = grandparent;parent = cur->_parent;}// 情况二三 uncle不存在，或uncle存在且为黑else{// parent 为 grandparent 的右孩子，且 cur 为 parent 的右孩子if (cur == parent->_right){RotateL(grandparent);parent->_col = BLACK;grandparent->_col = RED;}// parent 为 grandparent 的右孩子，且 cur 为 parent 的左孩子else{RotateR(parent);RotateL(grandparent);cur->_col = BLACK;grandparent->_col = RED;}break;}}}_root->_col = BLACK;return true;}
private:void RotateL(Node* parent){Node* subR = parent->_right;Node* subRL = subR->_left;parent->_right = subRL;if (subRL){subRL->_parent = parent;}Node* ppNode = parent->_parent;subR->_left = parent;parent->_parent = subR;if (_root == parent){_root = subR;subR->_parent = nullptr;}else{if (ppNode->_left == parent){ppNode->_left = subR;}else{ppNode->_right = subR;}subR->_parent = ppNode;}}void RotateR(Node* parent){Node* subL = parent->_left;Node* subLR = subL->_right;parent->_left = subLR;if (subLR){subLR->_parent = parent;}Node* ppNode = parent->_parent;subL->_right = parent;parent->_parent = subL;if (_root == parent){_root = subL;subL->_parent = nullptr;}else{if (ppNode->_left == parent){ppNode->_left = subL;}else{ppNode->_right = subL;}subL->_parent = ppNode;}}
private:Node* _root;
};

uncle存在且为红：
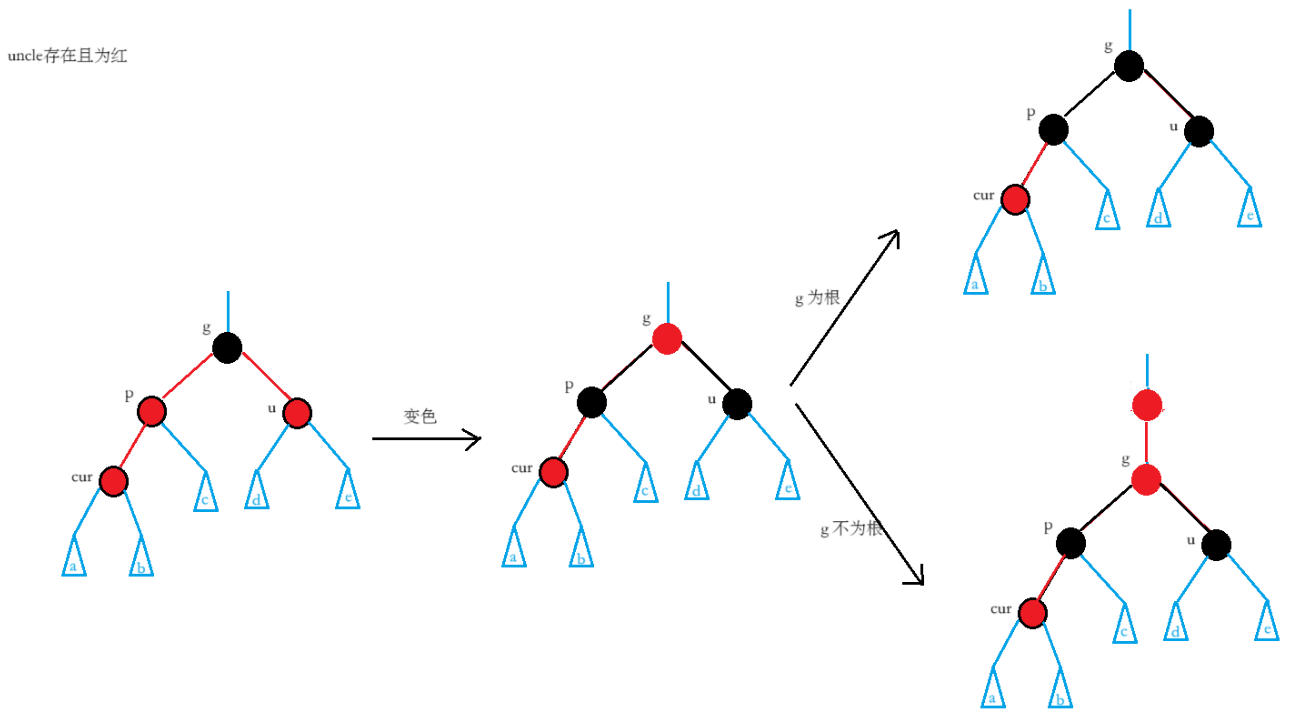
在这里插入图片描述
可以通过下面程序对红黑树的正确性做一个验证。

template<class K, class V>
class RBTree
{
private:typedef RBTreeNode<K, V> Node;
public:// 1.验证其为二叉搜索树void InOrderTraversal(){_InOrderTraversal(_root);}// 2. 通过红黑树的性质验证其平衡性bool IsBalance(){// 空树也算红黑树if (_root == nullptr){return true;}// 验证性质2if (_root->_col == RED){cout << "根节点不是黑色" << endl;return false;}// benchmark作为每条路径上黑色节点数量的基准值int benchmark = 0;Node* cur = _root;while (cur){if (cur->_col == BLACK){++benchmark;}cur = cur->_left;}return PrevCheck(_root, 0, benchmark);	}
private:// 如果中序遍历为有序序列，则说明为二叉搜索树void _InOrderTraversal(Node* root){if (root == nullptr){return;}_InOrderTraversal(root->_left);cout << root->_kv.first << " : " << root->_kv.second << endl;_InOrderTraversal(root->_right);}bool PrevCheck(Node* root, int blackNum, int benchmark){if (root == nullptr){// 验证性质4if (blackNum != benchmark){cout << "路径上黑色节点数量不相等" << endl;return false;}else{return true;}}// 验证性质3if (root->_col == RED && root->_parent->_col == RED){cout << "存在连续的红色节点" << endl;return false;}if (root->_col == BLACK){++blackNum;}return PrevCheck(root->_left, blackNum, benchmark)&& PrevCheck(root->_right, blackNum, benchmark);}
private:Node* _root;
};