---

1. AVL树的概念

AVL树是一棵二叉搜索树，但它的每个节点的左右子树的高度差的绝对值不超过1，且它的子树也是平衡二叉树。左右子树的高度差也叫平衡因子，平衡因子 = 右子树叶的高度 - 左子树的高度。

将AVL树与满二叉树对比，看看AVL的效率如何？

---

2. AVL树的实现

2.1 节点的定义

//节点
template<class K, class V>
struct AVLTreeNode
{AVLTreeNode<K, V>* _left;AVLTreeNode<K, V>* _right;AVLTreeNode<K, V>* _parent;pair<K, V> _kv;int _bf;//平衡因子AVLTreeNode(const pair<K, V> kv):_left(nullptr), _right(nullptr), _parent(nullptr), _kv(kv), _bf(0){}
};

2.2 插入

1. AVL树是二叉搜索树，所以首先按照二叉搜索树的规矩插入。插入后再考虑插入节点后，AVL树是否平衡。
2. 有个例子

（1）更新后parent的平衡因子如果是1或者-1，说明parent所在子树的高度发生变化，会影响祖先，需要沿着到root的路径往上更新。

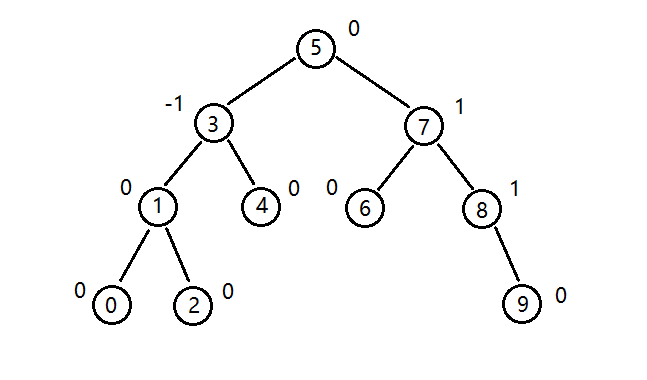
（2）更新后parent的平衡因子如果是0，说明parent所在子树的高度不变，不用继续沿着到root的路径往上更新。

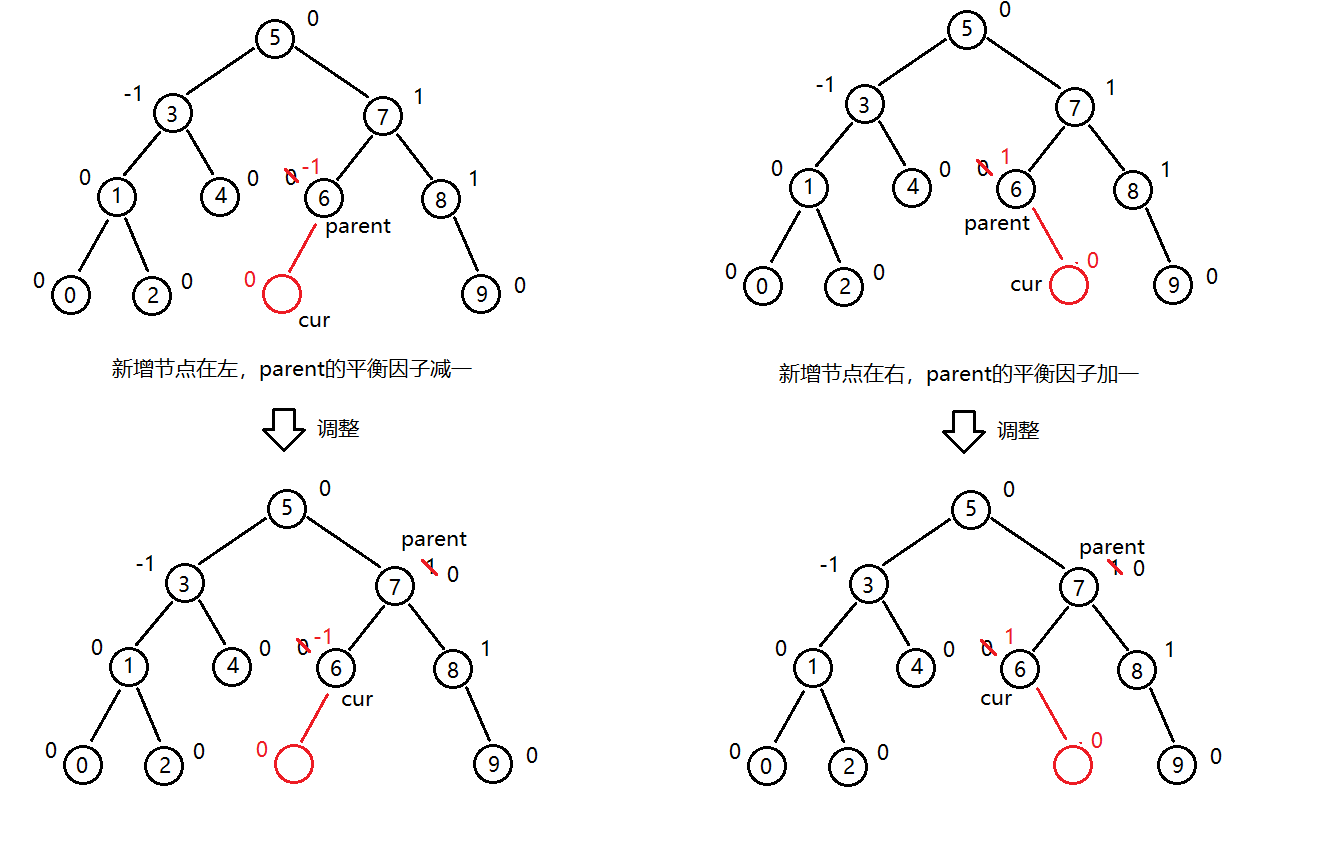
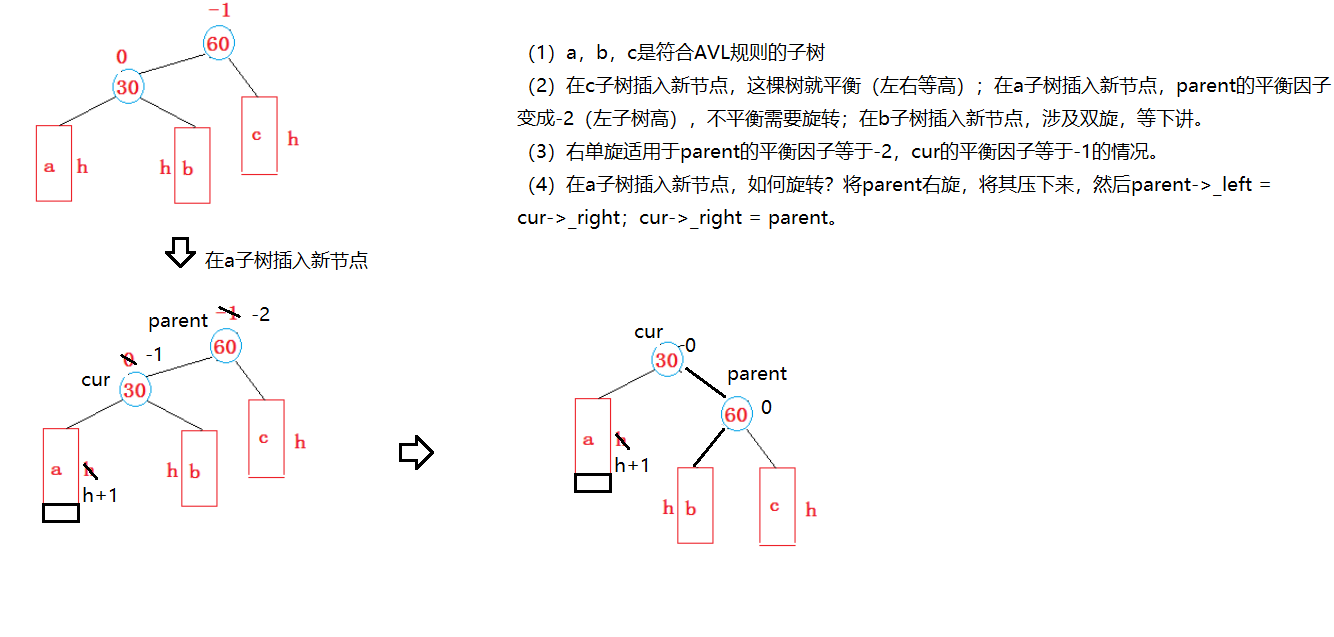
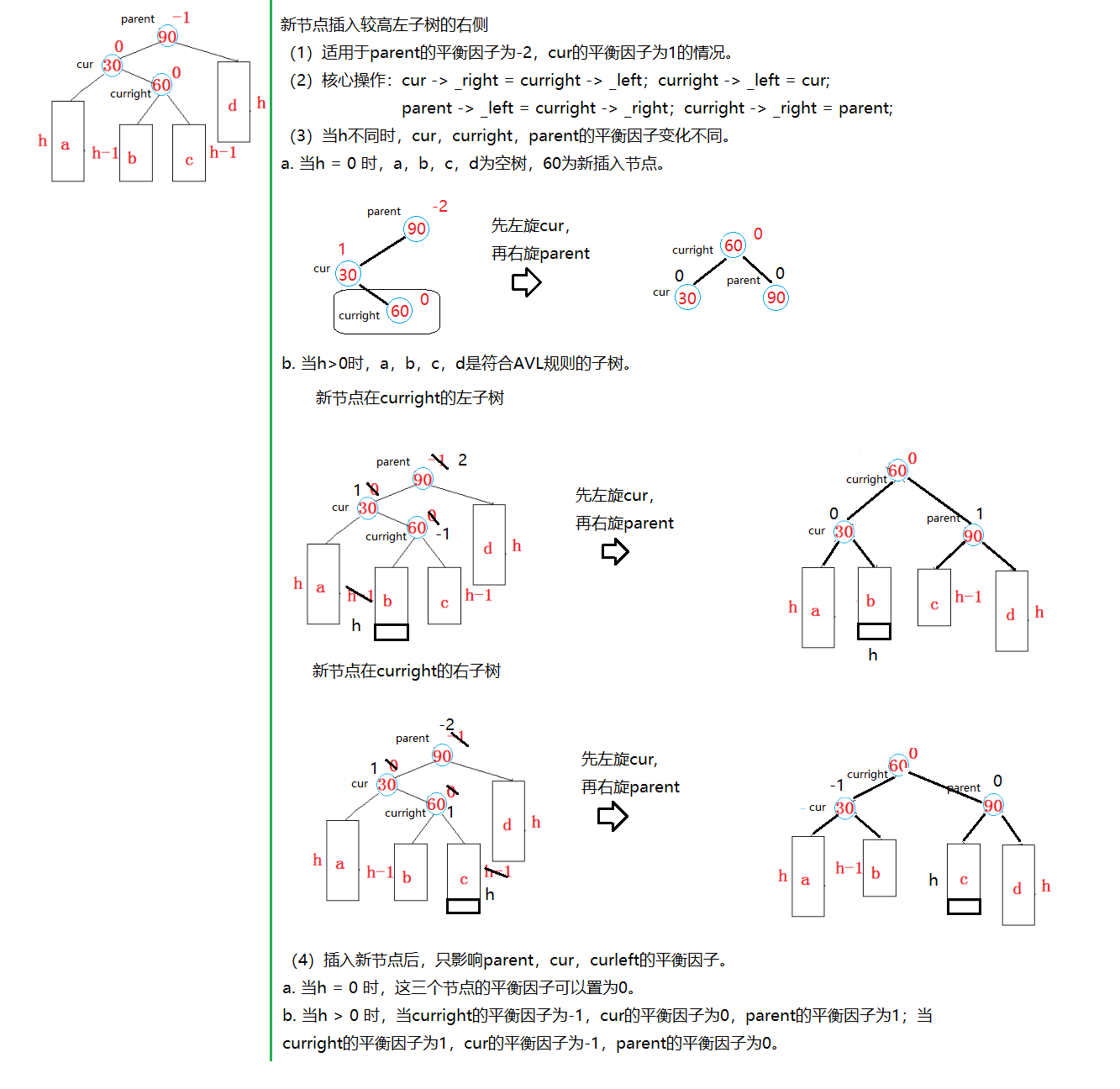
（3）更新后parent的平衡因子如果是2或者-2，说明parent所在子树的高度变化且不平衡，对parent的子树进行旋转，使其平衡。

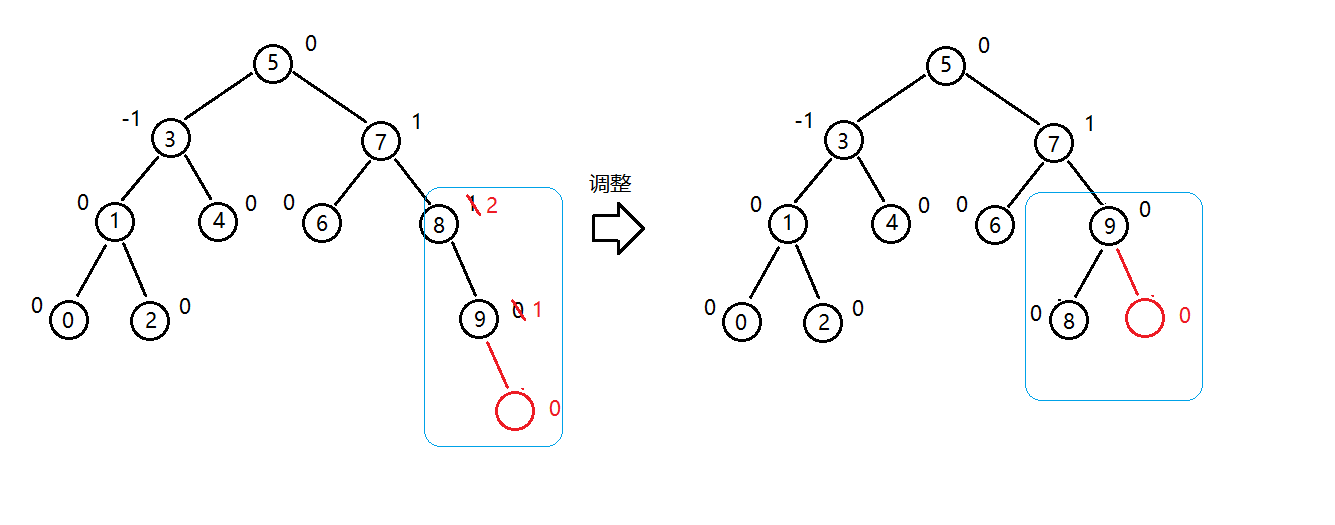
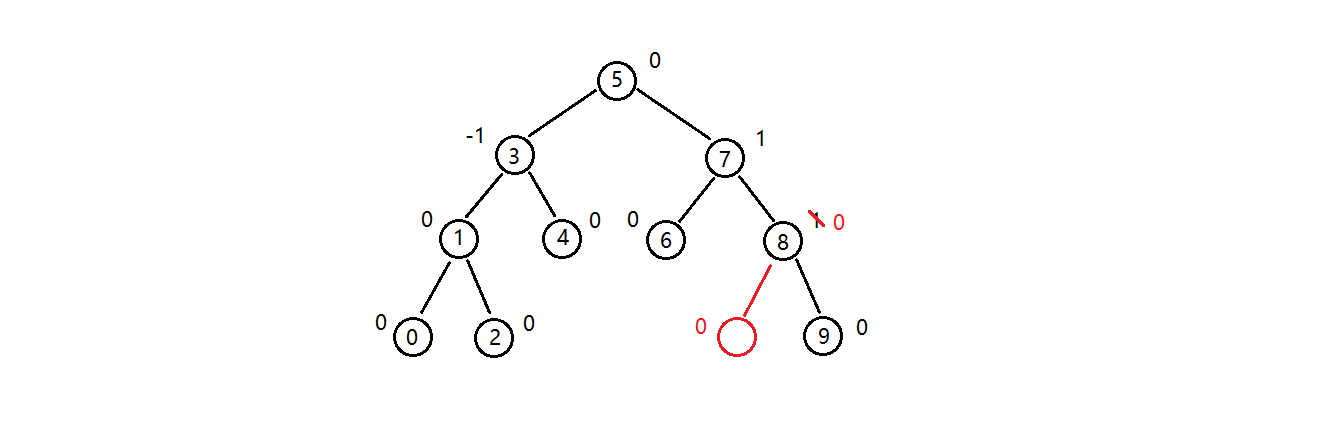
（4）如果parent是头节点，对parent进行旋转后，记得更新根节点。

3. 旋转的原理

节点的插入可以分为以下几种情况
（1）左单旋：新节点插入在较高右子树的右侧

（2）右单旋：新节点插入较高左子树的左侧

（3）双旋：新节点插入较高右子树的左侧

（4）双旋：新节点插入较高左子树的右侧

代码

template<class K, class V>
class AVLTree
{
public:typedef AVLTreeNode<K, V> Node;bool insert(const pair<K, V>& kv){if (_root == nullptr){_root = new Node(kv);return true;}Node* cur = _root;Node* parent = cur;while (cur){if (cur->_kv.first < kv.first){parent = cur;cur = cur->_right;}else if (cur->_kv.first > kv.first){parent = cur;cur = cur->_left;}else{return false;}}cur = new Node(kv);if (parent->_kv.first < cur->_kv.first){parent->_right = cur;cur->_parent = parent;}else{parent->_left = cur;cur->_parent = parent;}//更新平衡因子while (parent){if (parent->_left == cur){--parent->_bf;}else{++parent->_bf;}//如果更新完平衡因子为0，说明其左右子树等高，已经平衡if (parent->_bf == 0){break;}//不等高，继续往上更新平衡因子else if (parent->_bf == 1 || parent->_bf == -1){cur = parent;parent = parent->_parent;}//不平衡，分为四种情况else if (parent->_bf == 2 || parent->_bf == -2){//新节点插入较高右子树的右侧，需要将parent左旋if (parent->_bf == 2 && cur->_bf == 1){RotateL(parent);}//新节点插入较高左子树的左侧，需要将parent右旋else if (parent->_bf == -2 && cur->_bf == -1){RotateR(parent);}//新节点插入较高右子树的左侧，需要先将cur右旋，再将parent左旋else if (parent->_bf == 2 && cur->_bf == -1){RotateRL(parent);}//新节点插入较高左子树的右侧，需要先将cur左旋，再将parent右旋else if (parent->_bf == -2 && cur->_bf == 1){RotateLR(parent);}else{assert(false);}break;}else{assert(false);}}return true;}//左旋void RotateL(Node* parent){Node* cur = parent->_right;Node* curleft = cur->_left;parent->_right = curleft;if (curleft){curleft->_parent = parent;}cur->_left = parent;//不要忘记父节点的链接Node* pparent = parent->_parent;parent->_parent = cur;//要考虑parent的parent是否存在if (pparent){if (pparent->_left == parent){pparent->_left = cur;}else{pparent->_right = cur;}cur->_parent = pparent;}else{_root = cur;cur->_parent = nullptr;}//平衡因子置为0parent->_bf = cur->_bf = 0;}//右旋void RotateR(Node* parent){Node* cur = parent->_left;Node* curright = cur->_right;parent->_left = curright;if (curright){curright->_parent = parent;}cur->_right = parent;Node* pparent = parent->_parent;parent->_parent = cur;if (pparent){if (pparent->_left == parent){parent->_left = cur;}else{parent->_right = cur;}cur->_parent = pparent;}else{_root = cur;cur->_parent = nullptr;}parent->_bf = cur->_bf = 0;}//双旋：新节点插入较高右子树的左侧void RotateRL(Node* parent){Node* cur = parent->_right;Node* curleft = cur->_left;//先右旋，再左旋RotateR(cur);RotateL(parent);if (curleft->_bf == 0){parent->_bf = 0;cur->_bf = 0;}else if (curleft->_bf == 1){parent->_bf = -1;cur->_bf = 0;}else if (curleft->_bf == -1){parent->_bf = 0;cur->_bf = 1;}}//双旋：新节点插入较高左子树的右侧void RotateLR(Node* parent){Node* cur = parent->_left;Node* curright = cur->_right;//先左旋再右旋RotateL(cur);RotateR(parent);//更新平衡因子if (curright->_bf == 0){parent->_bf = 0;cur->_bf = 0;}else if (curright->_bf == 1){parent->_bf = 0;cur->_bf = -1;}else if (curright->_bf == -1){parent->_bf = 1;cur->_bf = 0;}}
private:Node* _root = nullptr;
};

2.3 是否是AVL树

如果一棵AVL树不平衡，那么它的左右子树的高度差的绝对值超过2，旋转出现问题。如果要判断一棵树是否是AVL树是否平衡，不能通过平衡因子判断，因为旋转出现问题，那么平衡因子也会出现问题，所以只能通过高度来判断。
代码

// 根据AVL树的概念验证pRoot是否为有效的AVL树bool IsAVLTree(){return IsAVLTree(_root);}bool IsAVLTree(Node* pRoot){//不能依赖平衡因子，容易监守自盗。如果旋转出现问题，平衡因子也会有问题//所以直接通过高度来判断if (pRoot == nullptr){return true;}int leftHeight = Height(pRoot->_left);int rightHeight = Height(pRoot->_right);//abs返回参数的绝对值return abs(leftHeight - rightHeight) < 2&& IsAVLTree(pRoot->_left) && IsAVLTree(pRoot->_right);}int Height(){return Height(_root);}int Height(Node* root){if (root == nullptr){return 0;}int leftHeight = Height(root->_left);int rightHeight = Height(root->_right);return leftHeight > rightHeight ? leftHeight + 1 : rightHeight + 1;}

---

3. AVL树与红黑树

插入时要维护其绝对平衡，旋转的次数比较多，如果需要一种查询高效且有序的数据结构，而且数据的个数为静态的(即不会改变)，可以考虑AVL树，但一个结构经常修改，就不太适合

红黑树不追
求绝对平衡，其只需保证最长路径不超过最短路径的2倍，相对而言，降低了插入和旋转的次数，
所以在经常进行增删的结构中性能比AVL树更优

1. AVL树和红黑树都是平衡二叉树。在查询效率方面，AVL树追求绝对平衡，红黑树要求最长路径不超过最短路径的两倍，AVL查询数据效率比红黑树高，但是对于CPU而言，都是属于logN这个量级的。
2. AVL树追求控制严格平衡是需要付出代价的，插入和删除已需要进行大量的旋转。红黑树不追求绝对平衡，其只需保证最长路径不超过最短路径的2倍，相对而言，降低了插入和旋转的次数，所以在插入和删除方面红黑树效率更高。
3. 综上，红黑书更优，实际运用的多。