问题描述

本题为填空题，只需要算出结果后，在代码中使用输出语句将所填结果输出即可。

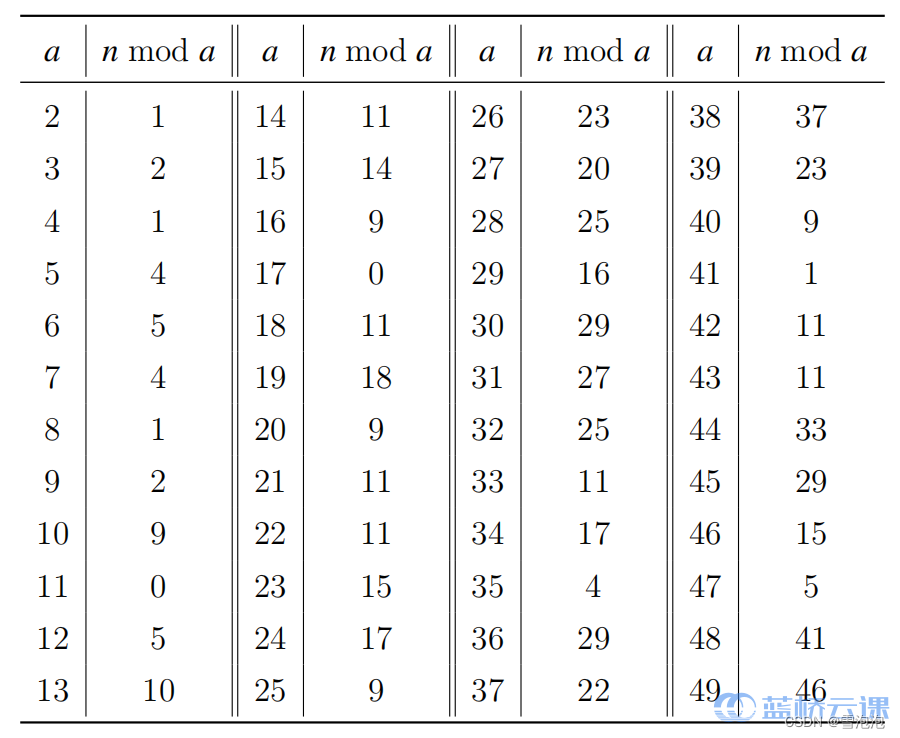
有一个不超过 10^17 的正整数  n，知道这个数除以 2 至 49 后的余数如下表所示，求这个正整数最小是多少。

运行限制

• 最大运行时间：1s
• 最大运行内存: 512M

思路：

a                mod                间隔                                                n

2                   1                     2                    3、5、7、9、11、13、15、17、19、21、23···

3                   2                     6                    5、8、11、14、17、20、23、26、29、32···

4                   1                     12                  5、9、13、17、21、25、29、33、37、41···

5                   4                     4                    9、14、19、24、29、34、39、44、49、53···

```

由上表可得出规律，间隔为x个a的公倍数。

在n中找到既满足n%a=mod又满足n%(a-1)=mod的数。

import java.util.Scanner;public class Main {public static void main(String args[]) {long lcm=2,ans=3;long[] a=new long[] {0,0,1,2,1,4,5,4,1,2,9,0,5,10,11,14,9,0,11,18,9,11,11,15,17,9,23,20,25,16,29,27,25,11,17,4,29,22,37,23,9,1,11,11,33,29,15,5,41,46};for(int i=3;i<=49;i++) {while(ans%i!=a[i]) {ans+=lcm;}lcm=lcm(i,lcm);}System.out.println(ans);}public static long gcd(long a,long b) {if(b==0) {return a;}else {return gcd(b,a%b);}}public static long lcm(long a,long b) {return a*(b/gcd(a,b));}
}