1. 题目信息

给定一个正整数 n，生成一个包含 1 到 n2 所有元素，且元素按顺时针顺序螺旋排列的正方形矩阵。

示例:输入: 3
输出:
[[ 1, 2, 3 ],[ 8, 9, 4 ],[ 7, 6, 5 ]
]

来源：力扣（LeetCode）
链接：https://leetcode-cn.com/problems/spiral-matrix-ii
著作权归领扣网络所有。商业转载请联系官方授权，非商业转载请注明出处。

2. LeetCode 59 解题

类似题目：LeetCode 885. 螺旋矩阵 III

• 创建变量top、bottom表示上下行的区间，left、right表示列的区间
  

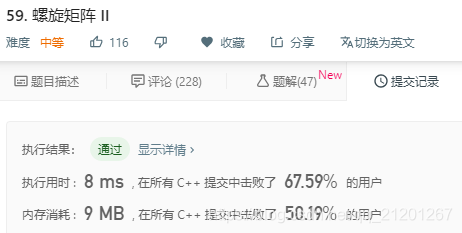
class Solution {
public:vector<vector<int>> generateMatrix(int n) {vector<vector<int> > v(n, vector<int>(n,0));if(n == 0) return {};int i, num = 0, total = n*n, top = 0, bottom = n-1, left = 0, right = n-1;while(num < total){for(i = left; i <= right; ++i)v[top][i] = ++num;++top;for(i = top; i <= bottom; ++i)v[i][right] = ++num;--right;for(i = right; i >= left; --i)v[bottom][i] = ++num;--bottom;for(i = bottom; i >= top; --i)v[i][left] = ++num;++left;}return v;}
};

3. LeetCode 54. 螺旋矩阵

给定一个包含 m x n 个元素的矩阵（m 行, n 列），请按照顺时针螺旋顺序，返回矩阵中的所有元素。

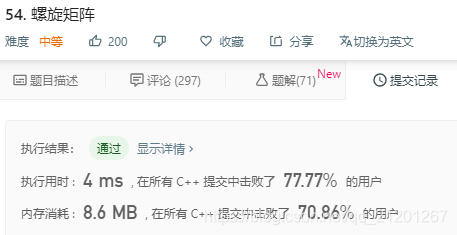
class Solution {
public:vector<int> spiralOrder(vector<vector<int>>& matrix) {if(matrix.empty()) return {};vector<int> ans;int m = matrix.size(), n = matrix[0].size();int i, num = 0, total = m*n, top = 0, bottom = m-1, left = 0, right = n-1;while(num < total){for(i = left; i <= right; ++i)ans.push_back(matrix[top][i]),num++;if(num == total)break;++top;for(i = top; i <= bottom; ++i)ans.push_back(matrix[i][right]),num++;if(num == total)break;--right;for(i = right; i >= left; --i)ans.push_back(matrix[bottom][i]),num++;if(num == total)break;--bottom;for(i = bottom; i >= top; --i)ans.push_back(matrix[i][left]),num++;if(num == total)break;++left;}return ans;}
};

4.《剑指Offer》面试题29

面试题29. 顺时针打印矩阵

class Solution {	//2020.2.22
public:vector<int> spiralOrder(vector<vector<int>>& matrix) {if(matrix.size()==0 || matrix[0].size()==0)return {};int i = 0, k = 0, count = matrix.size()*matrix[0].size();int left = 0, right = matrix[0].size()-1, up = 0, bottom = matrix.size()-1;vector<int> ans(count);while(count){i = left;while(count && i <= right){ans[k++] = matrix[up][i++];count--;}up++,i = up;while(count && i <= bottom){ans[k++] = matrix[i++][right];count--;}right--;i = right;while(count && i >= left){ans[k++] = matrix[bottom][i--];count--;}bottom--;i = bottom;while(count && i >= up){ans[k++] = matrix[i--][left];count--;}left++;}return ans;}
};