LeetCode 1522. Diameter of N-Ary Tree（递归）

文章目录

- 1. 题目
- 2. 解题

1. 题目

Given a root of an N-ary tree, you need to compute the length of the diameter of the tree.

The diameter of an N-ary tree is the length of the longest path between any two nodes in the tree. This path may or may not pass through the root.

(Nary-Tree input serialization is represented in their level order traversal, each group of children is separated by the null value.)

Example 1:
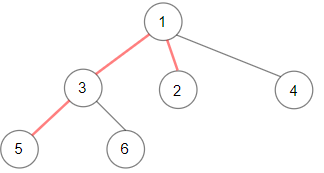
在这里插入图片描述

Input: root = [1,null,3,2,4,null,5,6]
Output: 3
Explanation: Diameter is shown in red color.

Example 2:
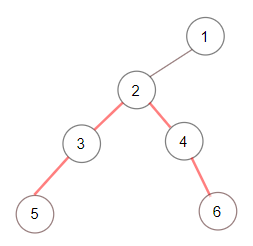
在这里插入图片描述

Input: root = [1,null,2,null,3,4,null,5,null,6]
Output: 4

Example 3:
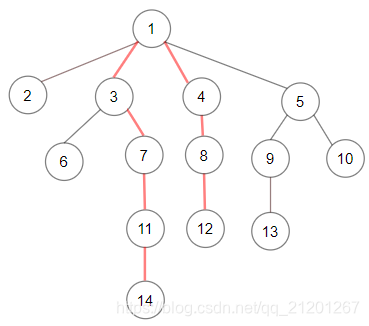
在这里插入图片描述

Input: root = [1,null,2,3,4,5,null,null,6,7,null,8,null,9,10,null,null,11,null,12,null,13,null,null,14]
Output: 7Constraints:
The depth of the n-ary tree is less than or equal to 1000.
The total number of nodes is between [0, 10^4].

来源：力扣（LeetCode）
链接：https://leetcode-cn.com/problems/diameter-of-n-ary-tree
著作权归领扣网络所有。商业转载请联系官方授权，非商业转载请注明出处。

2. 解题

/*
// Definition for a Node.
class Node {
public:int val;vector<Node*> children;Node() {}Node(int _val) {val = _val;}Node(int _val, vector<Node*> _children) {val = _val;children = _children;}
};
*/class Solution {int ans = 0;
public:int diameter(Node* root) {h(root);return ans;}int h(Node* root){if(!root) return 0;int maxdep1 = 0, maxdep2 = 0, height;for(auto c : root->children){height = h(c);if(height >= maxdep1){maxdep2 = maxdep1;maxdep1 = height;}else if(height > maxdep2)maxdep2 = height;}ans = max(ans, maxdep2+maxdep1);return max(maxdep1, maxdep2) + 1;}
};