Codeforces Round #702 (Div. 3) 全部题解

读错题意，写了半天真是心态爆炸，总的来看这次题目不难的。

A. Dense Array

http://codeforces.com/contest/1490/problem/A
在这里插入图片描述
解题思路
相邻的数字必然是倘若不满足的话是需要插入数据的，那么我们模拟插入数据即可.
$x = min(a_i, a_{i+1}), y = max(a_i, a_{i+1})$

倘若 $\leq y$ 不需要操作
否则，需要插入数据。不断的贪心插入 $2⋅x2\cdot x$ ，直到满足条件。其实这有一个公式的，推导与公式如下所示。

模拟乘以2

#include <bits/stdc++.h>
using namespace std;typedef long long LL;
typedef pair<int, int> PII;
const int N = 1010, INF = 0x3f3f3f3f;
int n, a[N];
PII b[N];
bool st[N];int cal(int t1, int t2)
{int ret = 0;while (2 * t1 < t2){ret ++;t1 *= 2;}return ret;
}int main()
{int t;  cin >> t;while (t -- ){cin >> n;LL x = 0;for (int i = 1; i <= n; i ++ ){scanf("%d", &a[i]);}double cnt;for (int i = 1, t1, t2; i < n; i ++ ){t1 = min(a[i], a[i + 1]), t2 = max(a[i], a[i + 1]);if (t1 * 2 >= t2)   continue;else{x += cal(t1, t2);}}cout << x << endl;}return 0;
}

直接上公式，没有过，应该是小数精度会被卡

#include <bits/stdc++.h>
using namespace std;typedef long long LL;
typedef pair<int, int> PII;
const int N = 1010, INF = 0x3f3f3f3f;
int n, a[N];int main()
{int t;  cin >> t;while (t -- ){cin >> n;LL x = 0;for (int i = 1; i <= n; i ++ ){scanf("%d", &a[i]);}double cnt;for (int i = 1, t1, t2; i < n; i ++ ){t1 = min(a[i], a[i + 1]), t2 = max(a[i], a[i + 1]);if (t1 * 2 >= t2)   continue;else{x += ceil(log(t2 * 1.0 / t1) / log(2)) - 1;}}cout << x << endl;}return 0;
}

B. Balanced Remainders

http://codeforces.com/contest/1490/problem/B
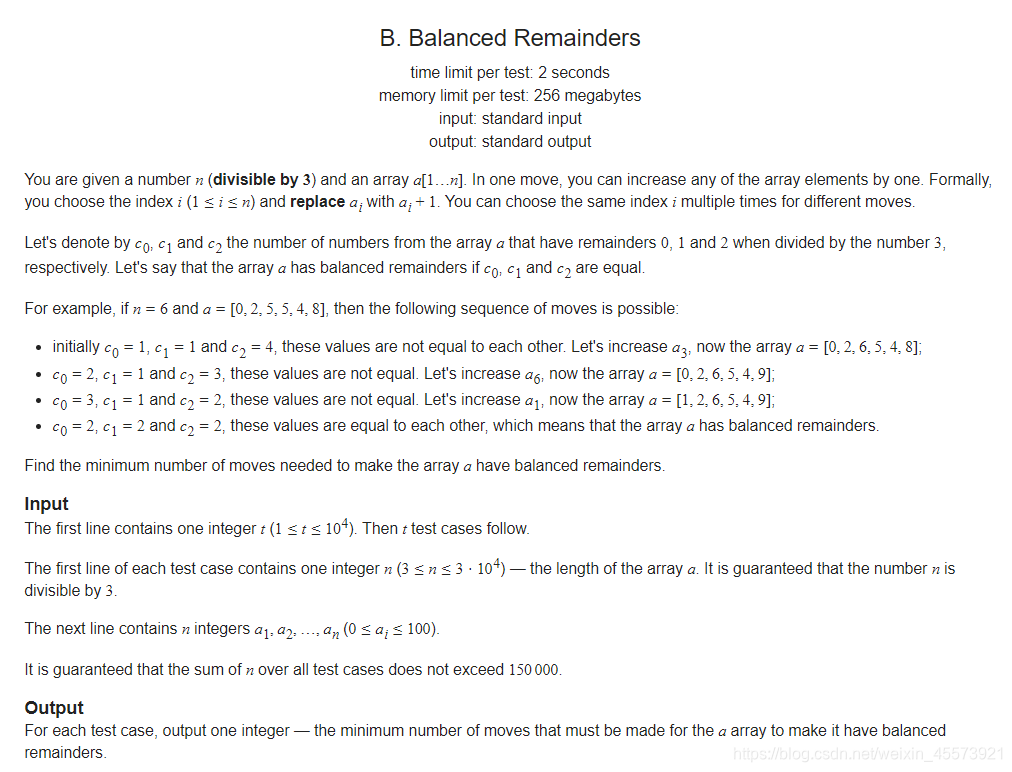
在这里插入图片描述
先统计出来 $%3\%3$ 余数为 $0, 1, 2$ 数字的数量 $c_0, c_1, c_2$ ，根据倘若多，那么一定会送走，倘若少，一定会挪进行这个性质进行模拟，而且对于特定的 $c_i$ 他给别人，或者是别人给他的交易对象是一样的，即操作是固定的。

#include <bits/stdc++.h>
using namespace std;typedef long long LL;
typedef pair<int, int> PII;
const int N = 100010, INF = 0x3f3f3f3f;
int n, a[N];
int c[5] = {0, 0, 0};int opt(int idx, int ave)
{int idx2 = (idx + 1) % 3, idx0 = (idx - 1 + 3) % 3;int need = 0;if (c[idx] < ave)	// 过少，需要向前一个索要{need = ave - c[idx];c[idx0] -= need;c[idx] += need;}else		// 太多，需要给下一个{need = c[idx] - ave;c[idx2] += need;c[idx] -= need;}return need;
}int main()
{int t;  cin >> t;while (t -- ){cin >> n;int ave = n / 3;memset(c, 0, sizeof c);for (int i = 1; i <= n; i ++ ){scanf("%d", &a[i]);c[a[i] % 3] ++;}int res = 0;for (int i = 0; i < 3; i ++ ){res += opt(i, ave);}printf("%d\n", res);}return 0;
}

C. Sum of Cubes

http://codeforces.com/contest/1490/problem/C
在这里插入图片描述
直接就是将 $i^3$ 在map上进行打表就可以了。
很关键的是预处理多处理几个，不然很容易被卡

#include <bits/stdc++.h>
using namespace std;typedef long long LL;
const int N = 100010;
LL n;
LL a[N];
map<LL, bool> m;int main()
{int t;  cin >> t;for (LL i = 1; i <= 10010; i ++ )	// 这里仅仅写到 10000就会 WA，主要是怕他多往后走了一步，查询到了0{a[i] = i * i * i;m[a[i]] = true;}// cout << m[0] << endl;while (t -- ){scanf("%lld", &n);bool flag = false;LL surp;for (int i = 1; !flag && a[i] <= n; i ++ ){surp = n - a[i];if (m[surp] == true){flag = true;}}if (flag)   puts("YES");else    puts("NO");}return 0;
}

D. Permutation Transformation

http://codeforces.com/contest/1490/problem/D
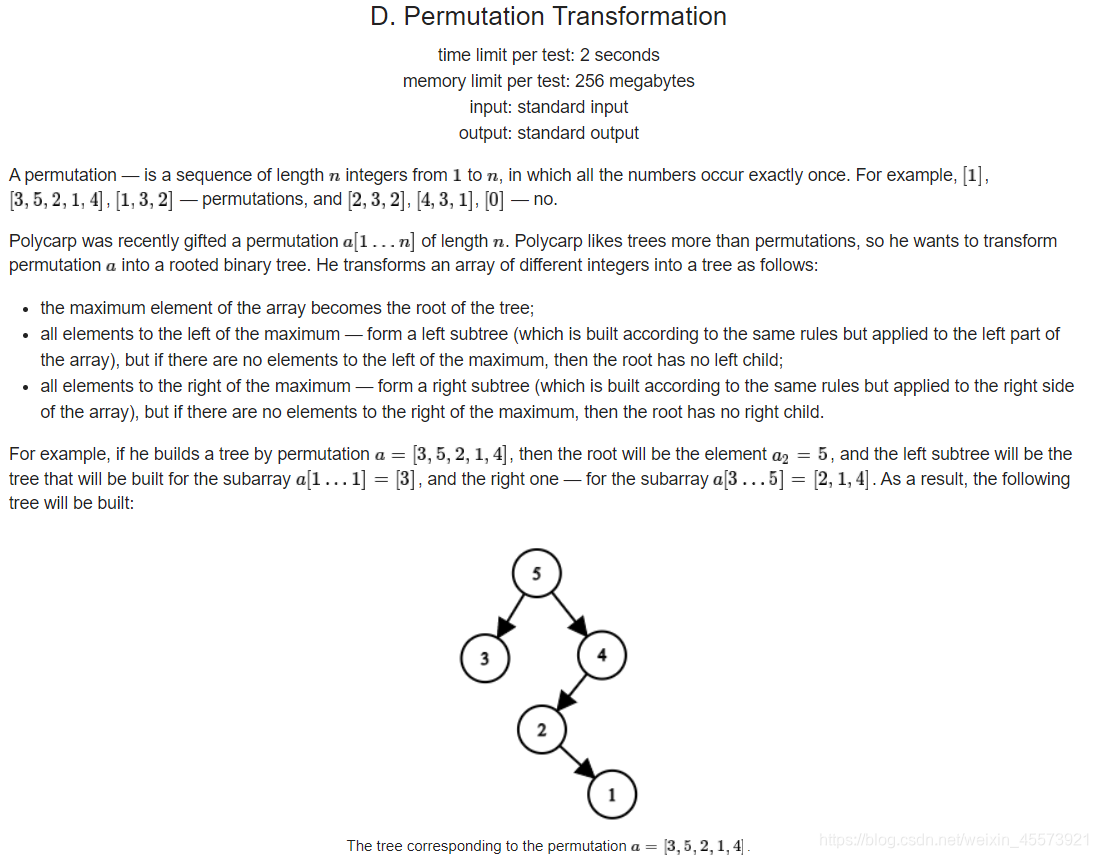
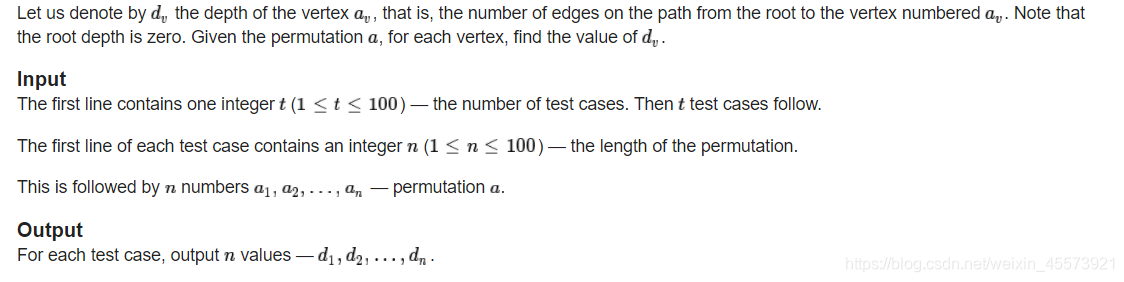
在这里插入图片描述

一个简单的模拟dfs，每次在区间找最大值作为 root 即可

#include <bits/stdc++.h>
using namespace std;const int N = 110;
int a[N], n, depth[N];void build(int fa, int l, int r)
{if (l >= r) return;int u = -1, v = -1;// 左子树for (int i = l; i < fa; i ++ ){if (u == -1 || a[u] < a[i])u = i;}depth[u] = depth[fa] + 1;build(u, l, fa - 1);// 右子树for (int i = fa + 1; i <= r; i ++ ){if (v == -1 || a[v] < a[i])v = i;}depth[v] = depth[fa] + 1;build(v, fa + 1, r);
}int main()
{int t;  cin >> t;while (t -- ){scanf("%d", &n);for (int i = 1; i <= n; i ++ )scanf("%d", &a[i]);memset(depth, -1, sizeof depth);int v;for (int i = 1; i <= n; i ++ ){if (a[i] == n){v = i;break;}}depth[v] = 0;build(v, 1, n);cout << depth[1];for (int i = 2; i <= n; i ++ )printf(" %d", depth[i]);cout << endl;}return 0;
}

E. Accidental Victory

http://codeforces.com/contest/1490/problem/E
在这里插入图片描述
说白了就是看谁可以赢。
首先我们先将他们按照各自的数值排序，得到数组 $a1,a2⋅⋅⋅,ana_1,a_2\cdot\cdot\cdot,a_n$ ，对应编号为 $idx1,idx2,⋅⋅⋅,idxnidx_1, idx_2, \cdot\cdot\cdot,idx_n$ ，我们对数组求取前缀和 $sum1,sum2,⋅⋅⋅,sumnsum_1, sum_2,\cdot\cdot\cdot,sum_n$ ，
我们从后往前看，

第 $n$ 个是最大的，肯定有机会成为winner
第 $n - 1$ 倘若可以成为 $w i n n e r$ ，当且仅当他赢过 $1 n - 2$ 个人，然后和 $a_n$ 比较，倘若他成不了 $w i n n e r$ 那么直接算法结束，比他小的人更成为不了 $w i n n n e r$
不断这样算下去即可

#include <bits/stdc++.h>
using namespace std;typedef long long LL;
typedef pair<LL, int> PII;
const int N = 200010;
vector<int> res;
LL a[N];
LL b[N];
PII c[N];
int n;bool cmp(const PII &t1, const PII &t2)
{return t1.first < t2.first;
}
int main()
{int t;  cin >> t;while (t -- ){scanf("%d", &n);for (int i = 1; i <= n; i ++ ){scanf("%lld", &a[i]);c[i].first = a[i];c[i].second = i;}sort(c + 1, c + n + 1, cmp);for (int i = 1; i <= n; i ++ )b[i] = b[i - 1] + c[i].first;res.clear();res.push_back(c[n].second);for (int i = n - 1; i >= 1; i -- ){if (b[i] >= c[i + 1].first)res.push_back(c[i].second);elsebreak;}sort(res.begin(), res.end());cout << res.size() << endl;cout << res[0];for (int i = 1; i < res.size(); i ++ )printf(" %d", res[i]);cout << endl;}return 0;
}

F. Equalize the Array

http://codeforces.com/contest/1490/problem/F
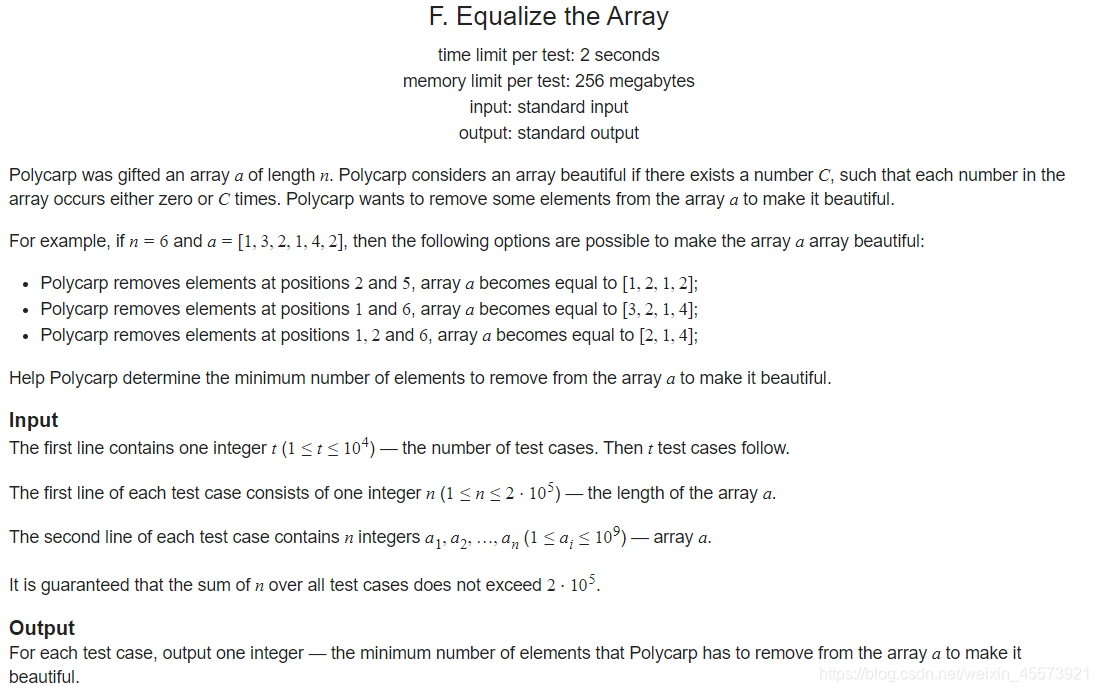
在这里插入图片描述
具体思路：
先用map将出现次数存储起来，统计出出现次数为 $i$ 的数字一共有 $cnt_i$ 个，
那么我们首先模拟一下，想让所有数字出现的次数为 $i$ 或者是 $0$ ，那么我们需要将出现次数小于等于 $i$ 的数字全部移除，需要的次数为 $cnt1⋅1+⋅⋅⋅cnti−1⋅(i−1)cnt_1\cdot1+\cdot\cdot\cdot cnt_{i-1}\cdot{(i-1)}$
需要将出现次数大于 $i$ 的数字次数缩减为 $i$ ，
需要的操作次数为$$
$⟹(i+1)⋅cnti+1+(i+2)⋅cnti+2+⋅⋅⋅+(n)⋅cntn−i⋅(cnti+1+cnti+2+⋅⋅⋅+cntn)\Longrightarrow(i+1)\cdot cnt_{i+1} + (i+2)\cdot cnt_{i+2}+\cdot\cdot\cdot+(n)\cdot cnt_n -i\cdot(cnt_{i+1}+cnt_{i+2}+\cdot\cdot\cdot+cnt_n)$
因此我们直接预处理出来 $cnt_i$ 的前缀数组和他们的加权成前缀数组即可

#include <bits/stdc++.h>
using namespace std;typedef pair<int, int > PII;
typedef long long LL;
const int INF = 0x3f3f3f3f;
const int N = 200010;
int a[N + 5];
int n;
map<int, int> m;
int cnt[N + 5];
int sum[N + 5];
LL addval[N + 5];int main()
{int t;  cin >> t;while (t -- ){m.clear();scanf("%d", &n);for (int i = 1; i <= n; i ++ ){scanf("%lld", &a[i]);m[a[i]] ++;}memset(cnt, 0, sizeof cnt);memset(sum, 0, sizeof sum);memset(addval, 0, sizeof addval);map<int, int>::iterator it;for (it = m.begin(); it != m.end(); it ++)  // 出现it->second 次数的数字进行统计{/// printf("IT: %d, %d\n",  it->first, it->second);cnt[it->second] ++;}// 计算前缀数组和累加和数组for (int i = 1; i <= n; i ++ )  // 最多出现 n 次{sum[i] = sum[i - 1] + cnt[i];addval[i] = addval[i - 1] + LL(cnt[i]) * i;}
/*printf("CNT:\n\t");for (int i = 1; i <= n; i ++ ){printf("%d ", cnt[i]);}cout << endl;
*/LL res = 1e16;LL up, down;for (int i = 1; i <= n; i ++ ){down = addval[i - 1];up = (addval[n] - addval[i - 1]) - (sum[n] - sum[i - 1]) * i;res = min(res, up + down);}cout << res << endl;}return 0;
}

G. Old Floppy Drive

http://codeforces.com/contest/1490/problem/G
在这里插入图片描述

本题是一个较为隐蔽的二分题目，需要我们贪心预处理出来可以二分的数组。
首先我们先将前缀数组给求出来，
并且贪心得到 cnt不断增加，而且前缀和也增加的数字，将其放入数组v, 具体见代码max_val部分。
那么数组 v 是一个数值（无论是val，还是opt_cnt）都不断增加的数据

倘若 $x_i$ 在数组 v 最大值的范围之内，那么直接二分找最小的cnt
否则，需要判断一下整个原数组 a 的累加和是否大于零，倘若小于等于0，无解
否则，是可以经过不断的循环找到这个数的，首先我们根据max(v)找到最小的循环次数，然后二分即可。
本题最坑的点就是 long long 开的地方很多，几乎所有的数据都要开long long

#include <bits/stdc++.h>
using namespace std;typedef long long LL;
const LL INF = 0x3f3f3f3f3f3f3f3f;
const int N = 200010;
int n, m;
LL a[N], x[N];
LL sum[N];
class Node
{
public:LL val, cnt;Node(LL _val = 0, LL _cnt = 0){val = _val, cnt = _cnt;}
}b[N];int main()
{int t;  cin >> t;while (t -- ){cin >> n >> m;LL cur_max = -INF;b[1] = Node(-INF, -1);int n2 = 1;sum[0] = 0LL;for (int i = 1; i <= n; i ++ ){scanf("%lld", &a[i]);sum[i] = sum[i - 1] + a[i];if (sum[i] > cur_max){cur_max = sum[i];b[++ n2] = Node(cur_max, i - 1);    // 注意在这里的 - 1}}for (int i = 1; i <= m; i ++ )scanf("%lld", &x[i]);// 当前的 b 数字 是 1~n val 增加， cnt增加的递增数组，下面我们的 x 仅仅只需要二分即可;/// printf("RES:\n\t");LL loop = sum[n];for (int i = 1; i <= m; i ++ ){if (b[n2].val >= x[i])  // 可以直接二分找 >= x[i] 的最小值{int l = 1, r = n2, mid;while (l < r){mid = l + r >> 1;if (b[mid].val >= x[i])r = mid;elsel = mid + 1;}printf("%lld ", b[l].cnt);}else    // 查看他的 loop{if (loop <= 0)  // 无法达到{printf("-1 ");}else{LL loopcnt = (x[i] - b[n2].val) / loop + ((x[i] - b[n2].val) % loop != 0);x[i] -= loopcnt * loop;int l = 1, r = n2, mid;while (l < r){mid = l + r >> 1;if (b[mid].val >= x[i])r = mid;elsel = mid + 1;}printf("%lld ", b[l].cnt + loopcnt * n);  // loopcnt 是乘以 n 的}}}puts("");}return 0;
}