Fibonacci

Problem Description

2007年到来了。经过2006年一年的修炼，数学神童zouyu终于把0到100000000的Fibonacci数列
(f[0]=0,f[1]=1;f[i] = f[i-1]+f[i-2](i>=2))的值全部给背了下来。
接下来，CodeStar决定要考考他，于是每问他一个数字，他就要把答案说出来，不过有的数字太长了。所以规定超过4位的只要说出前4位就可以了，可是CodeStar自己又记不住。于是他决定编写一个程序来测验zouyu说的是否正确。

 

 

Input

输入若干数字n(0 <= n <= 100000000)，每个数字一行。读到文件尾。

 

 

Output

输出f[n]的前4个数字（若不足4个数字，就全部输出）。

 

 

Sample Input

0 1 2 3 4 5 35 36 37 38 39 40

 

 

Sample Output

0 1 1 2 3 5 9227 1493 2415 3908 6324 1023

 

 

题解： n <= 100000000，数据范围很大，写大数斐波那契数列肯定会超时，这里用到了斐波那契通项公式和取对数处理。

 

先给出斐波那契通项公式：

 

关于公式的推导，给出传送门：  百度百科—斐波那契数列

我们知道了通项公式，题中要求结果大于4位的直接输出前4位，怎么才能求前四位呢？  我们要用到log取对数。

先看对数的性质,loga(b^c)=c*loga(b),loga(b*c)=loga(b)+loga(c);

假设给出一个数10234432,

那么log10(10234432)=log10(1.0234432*10^7)【用科学记数法表示这个数】=log10(1.0234432)+7;

log10(1.0234432)就是log10(10234432)的小数部分.

log10(1.0234432)=0.010063744(取对数所产生的数一定是个小数)

再取一次幂:10^0.010063744=1.023443198

这样想取前四位只要用这个数乘以1000再取整就行了

对公式取对数:

通项公式取对数后，会有误差，所以我们要对结果不超过10000的数打表。 另外最后一项小于0，对前四位不造成影响，可以直接忽略。

#include<cstdio>
#include<cstring>
#include<cmath>
double cnt=(1+sqrt(5))/2;
int a[21]={0,1};
int main()
{int n,i;for(i=2;i<21;++i)a[i]=a[i-1]+a[i-2];while(scanf("%d",&n)!=EOF){if(n<21)printf("%d\n",a[n]);else{double ans=-0.5*log10(5.0)+n*log10(cnt);ans=ans-floor(ans);//取到小数部分 ans=pow(10,ans); ans=floor(ans*1000);printf("%.0f\n",ans);}}return 0;
}

转自https://blog.csdn.net/zwj1452267376/article/details/50490990
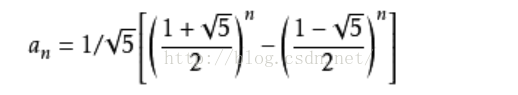
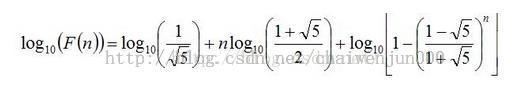