【堆排序的思路】
堆排序主要是利用了堆的性质。对于大顶堆:堆中的每一个节点的值都不小于它的孩子节点的值,具体可參考我的还有一篇博客http://blog.csdn.net/adminabcd/article/details/46880591,那么大顶堆的堆顶元素就是当前堆中全部元素中最大的。
利用这个性质。进行例如以下操作,则能够得到一个有序序列:
- 将待排序的n个元素一个一个插入堆中,那么此时堆顶元素就是全部元素中最大的
- 将堆顶元素取出,剩下的n-1个元素组成新的堆,新堆的堆顶元素是当前堆中元素中最大的,也就是全部元素中第二大的。
将堆顶元素取出。剩下的n-2个元素组成新的堆,新堆的堆顶元素是当前堆中元素中最大的。也就是全部元素中第三大的。
.
.
.
.直到全部元素取出,此时全部取出元素序列就是一个从大到小的有序序列。
【代码实现】
- 大顶堆的实现
#ifndef maxheap_h
#define maxheap_h
template<class T>
class Maxheap
{
public:Maxheap(int size);~Maxheap();bool Isempty();void push(T item); //插入操作void pop(); //删除操作T top();
private:T *heap;int currentSize;int capacity;
};
//-------构造函数初始化-------
template<class T>
Maxheap<T>::Maxheap(int size)
{if(size<1){throw"capacity must be >=1";}else{currentSize=0;capacity=size;heap=new T[capacity+1]; //heap[0]不使用}
}
//-------析构函数释放空间-------
template<class T>
Maxheap<T>::~Maxheap()
{delete []heap;
}
//--------推断堆是否为空-------
template<class T>
bool Maxheap<T>::Isempty()
{return currentSize==0;
}
//---------获取最大元素----------
template<class T>
T Maxheap<T>::top()
{return heap[1];
}
//-------插入操作-----
template<class T>
void Maxheap<T>::push(T item)
{if(currentSize==capacity)throw"Maxheap is full";else{currentSize++;int currentNode=currentSize;// 元素的插入位置初始化为最后while(currentNode>1&&heap[currentNode/2]<item) //(从下往上)进行调整{heap[currentNode]=heap[currentNode/2];currentNode=currentNode/2;}heap[currentNode]=item; //插入元素}
}//-----删除操作-------
template<class T>
void Maxheap<T>::pop()
{if(Isempty())throw"heap is empty ,cannot delete";else{T last=heap[currentSize]; //将最后一个元素初始化为根currentSize--;int currentNode=1; int child=2;while(child<=currentSize) //(从上往下)进行调整{if(child<currentSize&&heap[child]<heap[child+1])child++;if(last>=heap[child])break;else{heap[currentNode]=heap[child];currentNode=child;child=child*2;}}heap[ currentNode]=last; }
}
#endif
- 堆排序实现
#include"MAXHEAP.h"
#include<iostream>
using namespace std;
int main()
{Maxheap<int> H(100);int arr[]={50,15,30,70,6};for(int i=0;i<5;i++){H.push(arr[i]); //元素进堆}for(int i=0;i<5;i++){arr[i]= H.top();H.pop(); //取出堆顶元素。其余元素组成新的堆}cout<<"降序排序:";for(int i=0;i<5;i++){cout<<arr[i]<<" ";}cout<<endl;cout<<"升序排序:";for(int i=4;i>=0;i--){cout<<arr[i]<<" ";}cout<<endl;system("pause");return 0;
}【结果】
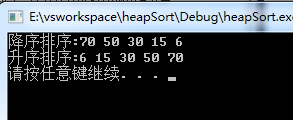
)


















