在上一篇迪瑞克斯拉算法中将功能实现了出来，完成了图集中从源点出发获取所有可达的点的最短距离的收集。
但在代码中getMinDistanceAndUnSelectNode()方法的实现并不简洁，每次获取minNode时，都需要遍历整个Map，时间复杂度太高。这篇文章主要是针对上一篇文章代码的一个优化。
其优化的过程中主要是用到了加强堆的数据结构，如果不了解的强烈建议先看加强堆的具体实现。

回顾：

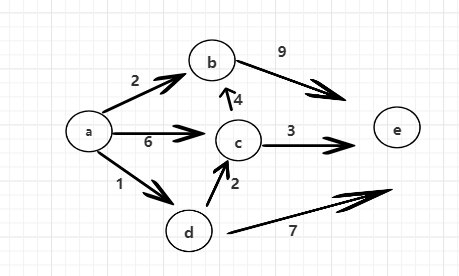
上一篇中，将确定不变的点放入Set中， 每个点之间的距离关系放入Map中，每次遍历Map获取最小minNode，并根据该点找到所有的边，算出最小距离后，放入set中，最终确定最小距离表。

优化
利用加强堆，来维护点和距离的关系，并利用小根堆的优势，让最小的点总是在最上面，需要注意的是，以往的的加强堆中，如果移除了这个元素会直接remove，但是在这里不能remove，因为要记录这个点是否在堆上，是否加入过堆，所以对于确定了的元素，value要改成 -1，用来标记当前元素已经确定，不用再动。

加强堆代码
NodeHeap中的distanceMap用来表示点和距离的关系，根据value的大小动态变化堆顶元素。
主方法是addOrUpdateOrIgnore()。
如果当前元素在堆中并且value != 1（inHeap），说明元素还没有确定，则判断进来的node和value是否大于当前堆中的node对应的value，取小的更新，如果更新，还需要改变元素在堆中位置，因为只可能越更新越小。所以要调用insertHeapify方法去改变堆结构。
如果元素还未加入过堆（!isEntered），则挂在堆尾，并insertHeapify检查是否上移。
pop方法中，如果弹出堆，正常要删除，但是不能删除，除了和最后一个元素交换，下移外，将distanceMap中对应的值改为 -1。否则无法判断该元素是否已经加入过堆，是否已经确定。

public static class NodeHeap {//Node类型的堆private Node[] nodes;//key对应的Node在堆中的位置是valueprivate HashMap<Node, Integer> heapIndexMap;//key对应的Node当前距离源点最近距离private HashMap<Node, Integer> distanceMap;//堆大小private int size;public NodeHeap(int size) {nodes = new Node[size];heapIndexMap = new HashMap<>();distanceMap = new HashMap<>();this.size = 0;}public boolean isEmpty() {return this.size == 0;}public boolean isEntered(Node head) {return heapIndexMap.containsKey(head);}public boolean inHeap(Node head) {return isEntered(head) && heapIndexMap.get(head) != -1;}public void swap(int index1, int index2) {heapIndexMap.put(nodes[index1], index2);heapIndexMap.put(nodes[index2], index1);Node tmp = nodes[index1];nodes[index1] = nodes[index2];nodes[index2] = tmp;}public void heapify(int index, int size) {int left = (index * 2) - 1;while (left < size) {int smallest = left + 1 < size && distanceMap.get(nodes[left + 1]) < distanceMap.get(nodes[left]) ? left + 1 : left;smallest = distanceMap.get(nodes[smallest]) < distanceMap.get(nodes[index]) ? smallest : index;if (smallest == index) {break;}swap(smallest, index);index = smallest;left = (index * 2) - 1;}}public void insertHeapify(Node node, int index) {while (distanceMap.get(nodes[index]) < distanceMap.get((index - 1) / 2)) {swap(distanceMap.get(nodes[index]), distanceMap.get((index - 1) / 2));index = (index - 1) / 2;}}public NodeRecord pop() {NodeRecord nodeRecord = new NodeRecord(nodes[0], distanceMap.get(0));swap(0, size - 1);heapIndexMap.put(nodes[size - 1], -1);distanceMap.remove(nodes[size - 1]);heapify(0, --size);return nodeRecord;}public void addOrUpdateOrIgnore(Node node, int distance) {if (inHeap(node)) {distanceMap.put(node, Math.min(distanceMap.get(node), distance));insertHeapify(node, distanceMap.get(node));}if (!isEntered(node)) {nodes[size] = node;heapIndexMap.put(node, size);distanceMap.put(node, distance);insertHeapify(node, size++);}}}

主方法逻辑
上来将给定的点添加到堆中，并且弹出，遍历所有的边放到加强堆中去搞。

  public static HashMap<Node, Integer> dijkstra2(Node head, int size) {NodeHeap nh = new NodeHeap(size);nh.addOrUpdateOrIgnore(head, 0);HashMap<Node, Integer> result = new HashMap<>();while (!nh.isEmpty()) {NodeRecord record = nh.pop();Node cur = record.node;int distance = record.distance;for (Edge edge : cur.edges) {nh.addOrUpdateOrIgnore(edge.to, distance + edge.weight);}result.put(cur, distance);}return result;}